Points of Concurrency Quiz

perpendicular bisector

A line, segment, or ray that is perpendicular to a side of the triangle at its midpoint is called the

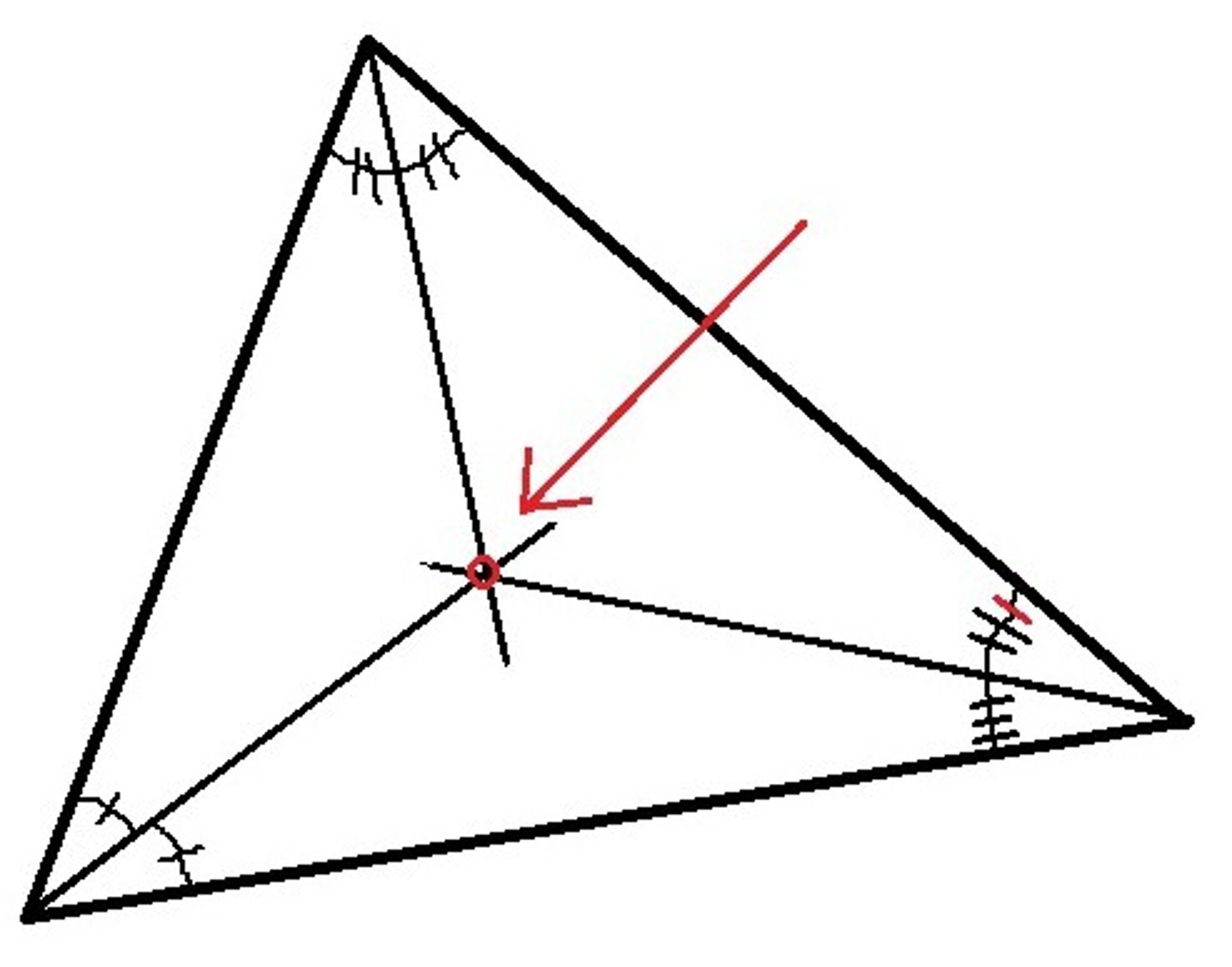
angle bisector

A ray that divides an angle into two congruent angles is called the

median

The segment that connects a vertex of the triangle to the midpoint of the opposite side of the triangle is called the

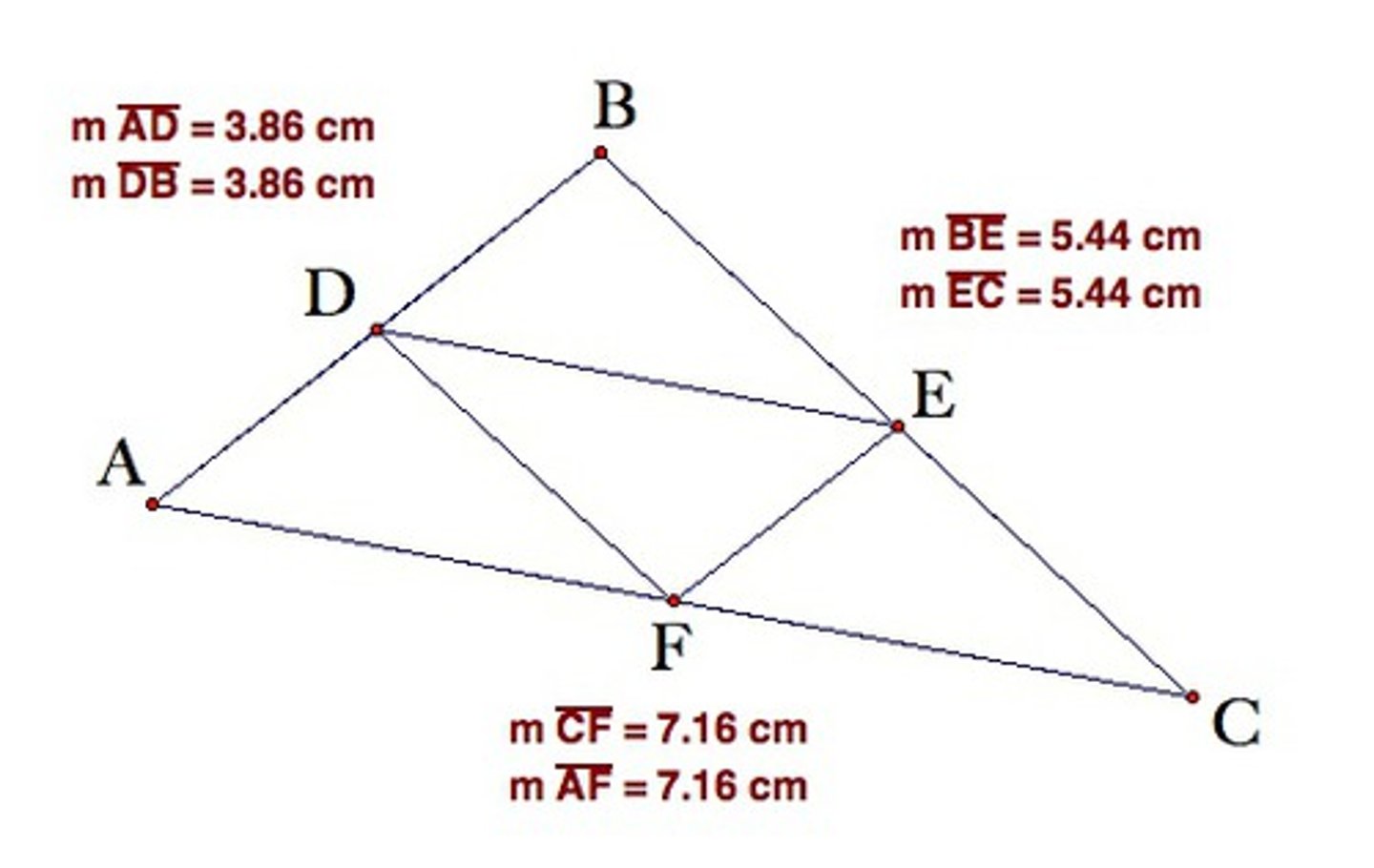
midsegment

A segment connecting the midpoints of two sides of a triangle is called the

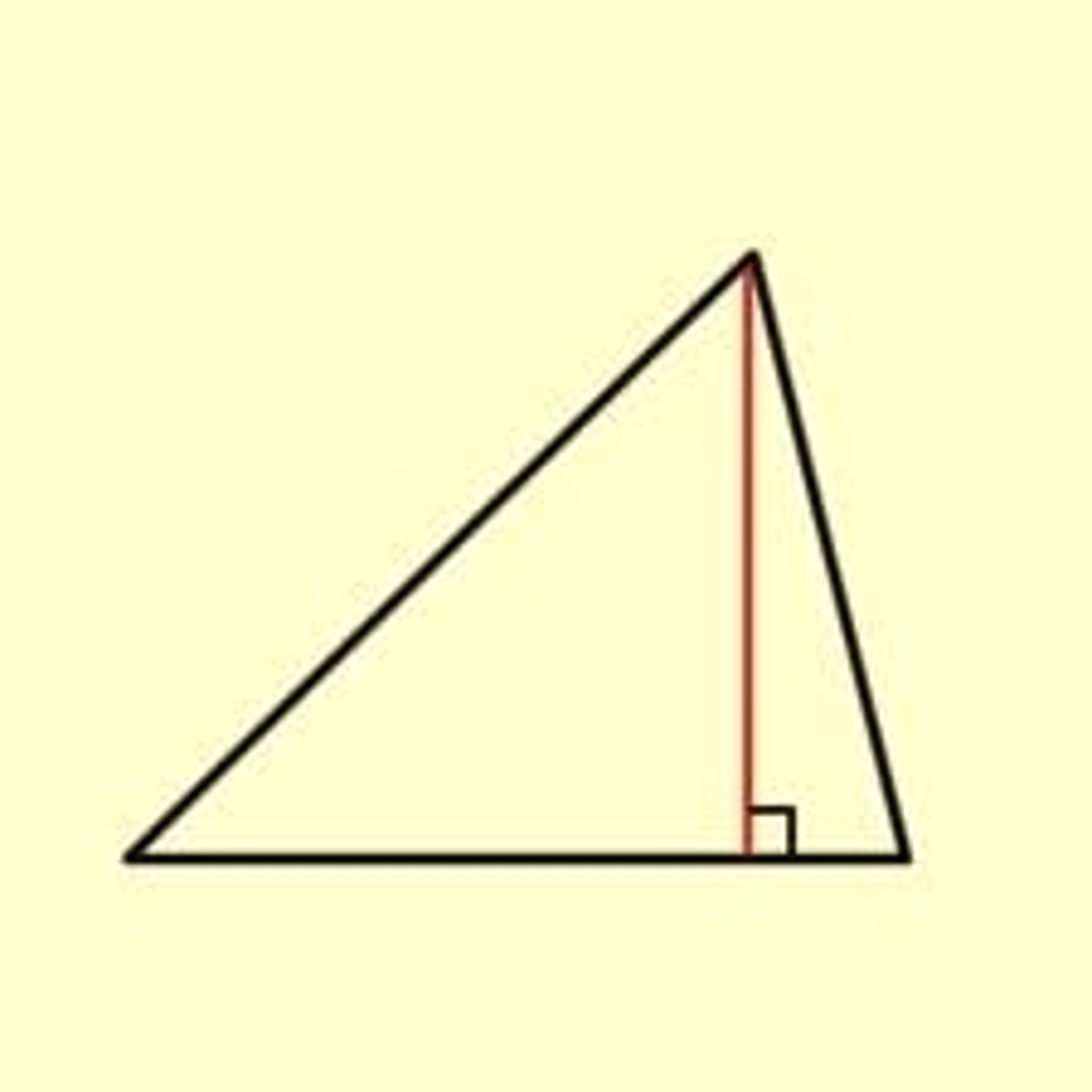
altitude

A perpendicular segment that connects a vertex of the triangle to the line containing the opposite side is called the

incenter

In a triangle, the point of concurrency of the angle bisectors is called the

circumcenter

In a triangle, the point of concurrency of the perpendicular bisectors is called the

centroid

In a triangle, the point of concurrency of the medians is called the

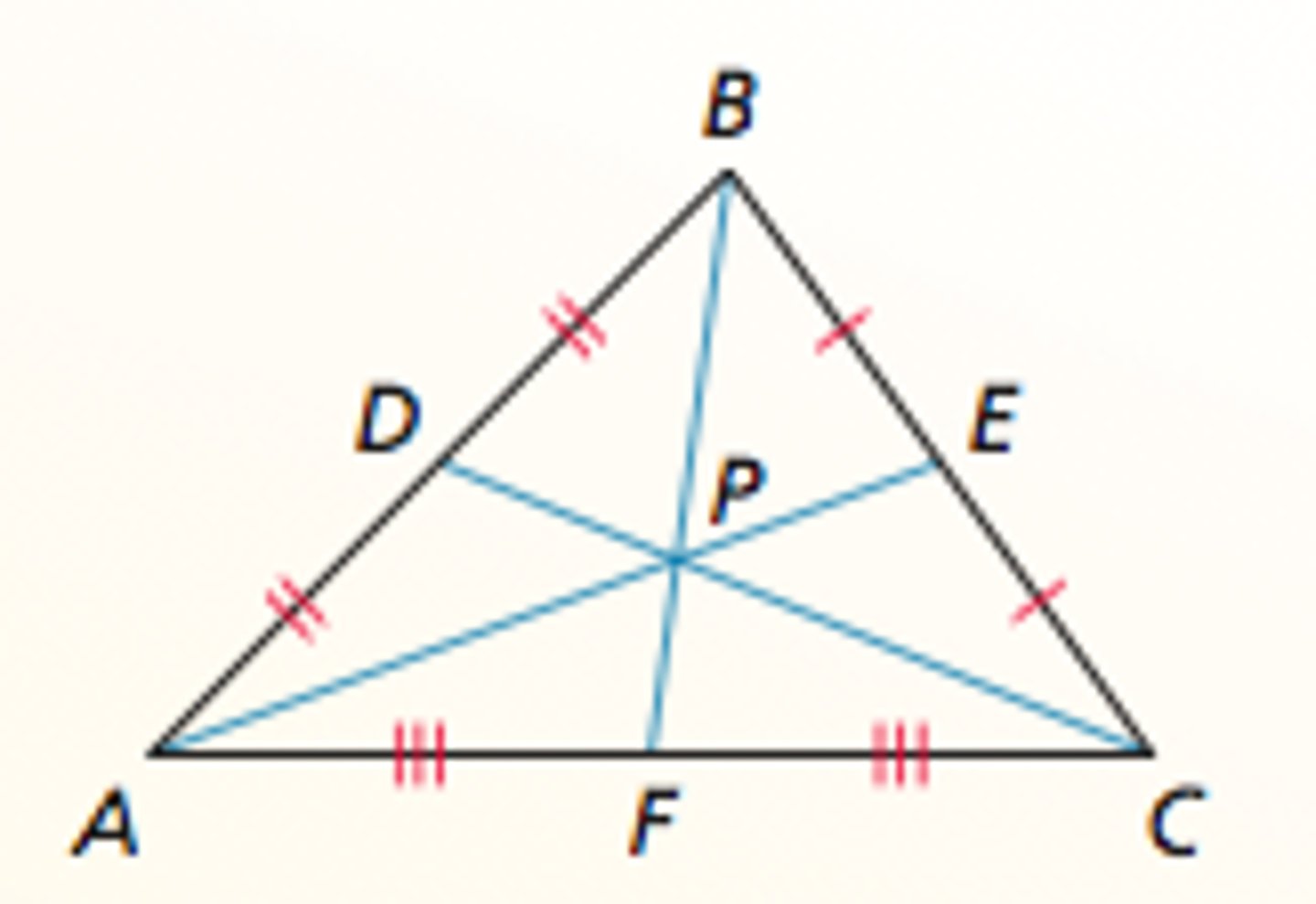
midsegment

In a triangle, 4 equal area triangles are formed

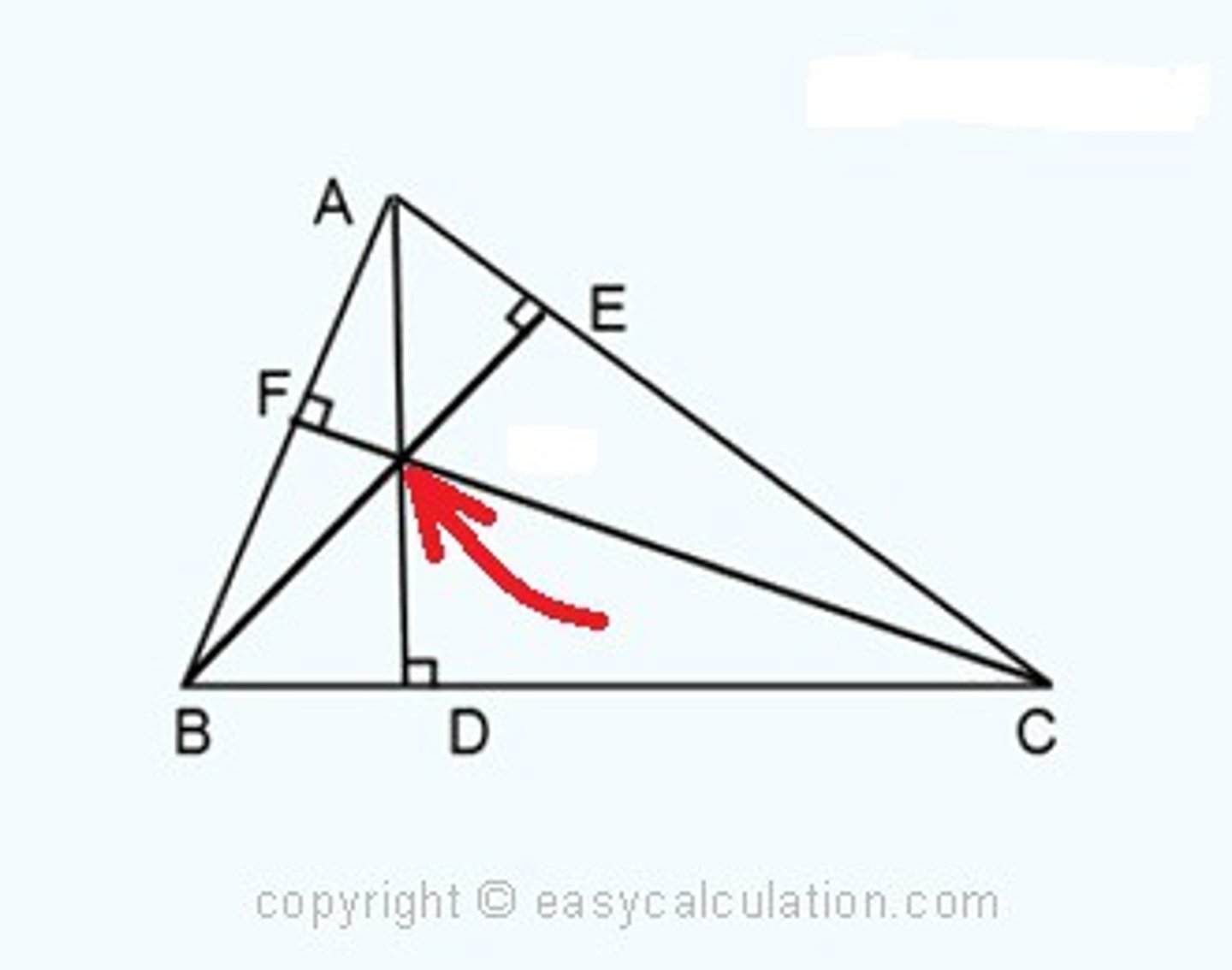
orthocenter

In a triangle, the point of concurrency of the altitudes is called the

incircle

created by bisecting each angle of the triangle

circumcenter

created by generating a perpendicular line at each midpoint of each side

centroid

created by connecting each midpoint to its opposite vertex

midsegment

created by connecting the midpoint of each side

orthocenter

created by generating a perpendicular line to a side that goes through its opposite vertex

incenter

-equidistant from the sides
-generates an inscribed circle
-always inside triangle

circumcenter

-equidistant from vertices
-generates a circumcircle
-inside (acute), on (right), and outside (obtuse) triangle

centroid

-creates 6 triangles of equal area
-identifies center of gravity
-always inside triangle

midsegment

-generates 4 congruent triangles
-each midsegment is parallel to its corresponding sides

orthocenter

-inside (acute), on (right), and outside (obtuse) triangle

circumcircle

The circle that passes through the vertices of the triangle is called the

inscribed circle

The circle that touches each side of the triangle at one point is called the