Lecture 2: bounded rationality

rational choice theory; prospect theory; regret theory; paradigm of inter-temporal choice

Discuss the different types of models for thinking

• Descriptive models: these are models of how the processes of decision making operates, irrespective of the outcome (if it was good or bad)

• Normative models: these models evaluate a decision in terms of the goals of the decision maker, decisions can be good or bad (good if it reaches this goal)

Discuss the rational choice theory, and which theoretical approach it takes.

Rational choice theory is a normative model, where rational decision maximises expected value. A range of information is integrated together to choose the most useful option.

• It assumes the decision maker has full access to all alternative thoughts

• Knows the probability of their outcomes

• Knows the value of their outcomes

Discuss the differences between rational choice theory and bounded rationality

• Rational choice theory suggests decision maker integrates: all alternatives, probabilities of outcomes, value of outcomes.

• Bounded rationality suggests were have limited cognitive capacity (limited outcome information); human behaviour can be impulsive, conditioned, imitating, and random.

Discuss a key problem of rational choice theory

Choice under certainty: when given the choice between two or more alternatives we choose the one with highest utility, if this outcome is uncertain we multiply probability by value. However, we don’t always rationally choose the most likely option.

• Expected Value = Probability x Value

Discuss the St. Petersburg Paradox introduced by Bernoulli (1738)

The St. Petersburg Paradox states that someone will flip a coin and should it land on ‘heads’ after a certain period of throws will give someone money, as the probability decreases the value to pay doubles (to infinity).

• According to Rational Choice Theory the expected value of this would be infinite, meaning someone should be willing to pay a potentially infinite sum.

• Demonstrates RCT failing to make a rational choice.

Discuss how the St. Petersburg Paradox opposes Rational Choice Theory.

The St. Petersburg Paradox suggests a potential infinite expected value.

• Bernoulli (1738) suggests expected utility of a decision is not the same as expected value (this then suggests each additional unit of wealth in the paradigm has less worth than the previous one)

• Therefore, decreasing the expected utility of additional currency.

• Despite the paradox being trivial, it shows the RC Theory fails to cover general situations.

Discuss the expected utility theory

The expected utility theory is another normative theory (clearly defining what the rational choices are). Where it differs is that expected utility might deviate from expected value, allowing for situational dependency of an outcome.

• Weak ordering: for any set of choices we must always be able to set a preference of one or neither

• Transitivity: choices must be transitive (e.g., if X > Z, and Z > Y, then this must be true: X > Y)

Discuss research by Kahneman and Tversky (1979, 1992) into risk-seeking and risk-aversive behaviours.

Research by Kahneman and Tversky (1979; 1992) investigated how expected value does not necessarily lead to the same expected utility.

• Participants would be given two options (either with equal expected value, or distorted one way)

Results: participants gain counter normative (in regards to rational choice theory) responses with greater risk taking when there is an uncertain gain, and greater risk aversion when there is an option for certain loss.

Discuss the prospect theory and what theoretical approach it takes.

The prospect theory introduced by Kahneman and Tversky is a descriptive model, aiming to account for deviations from rational choice theory (which is accurate sometimes).

• made up of two components: utility and probability.

Discuss what Tversky and Kahneman (1981) introduced.

Tversky and Kahneman (1981) introduced the value function which is for graphing gains and losses; utility function is nonlinear.

• The x-axis reflects losses (-) and gains (+).

• The y-axis reflects utility

• Function for gains and losses are asymmetric, which is why we treat losses more seriously than gains

Discuss how changes to small and large value changes influence the value function

• Small changes considerably increase gains and losses

• Changes to higher values have small influences on utilities.

Discuss what the π function is and its links to prospect theory.

The π function aims to explain why people prefer certainty for gains (certainty effect) and gambling for losses.

• According to the prospect theory we do not treat probabilities as they are stated

• Instead these are distorted by π (therefore objective p becomes subjective π).

• Probabilities near 0 are overweighted, whereas probabilities near to 1 (but not 1 itself; not fully certain) are underweighted.

Discuss the issue of π functions plotting extreme probabilities.

Extreme probabilities, as in incredibly low (tending to 0) and incredibly high (tending to 1, but not certainty itself) are overweighted and underweighted, respectively.

• Low probability: things like winning the lottery are incredibly unlikely, but this function exaggerates its likelihood

• High probability: things like 1-p are likely, but this function exaggerates the risk of it not happening.

Discuss the regret theory and what it aims to address.

The Regret Theory aims to complete the prospect theory, which does not give psychological reasons for the deviations from normative expectations that it shows from π functions.

• According to the Regret Theory we compare the outcomes, even after the decision has been made. Argues we will feel regretful if the decision we make does not lead to the highest payoff (even when the prospect was better), or we feel rejoice if we result in the better outcome.

Discuss what inter-temporal choice is, and which choice people tend to make.

Inter-temporal choice is a delayed reward decision (immediate gain now, or longer term gain, which could be better or worse).

• Research has demonstrated people are more likely to choose an immediate gain option (even though it has less value than a delayed one)

Discuss the model introduced by Samuelson (1937) to address inter-temporal choices

The Discounted Utility Model (Samuelson, 1937) suggests discount function (such that the time discount on the strength of the delayed reward) is an exponential function.

Discuss what this formula is representing.

This formula represents exponential discounting

• v = subjective discounted value

• k = the discount rate

• d = the delay

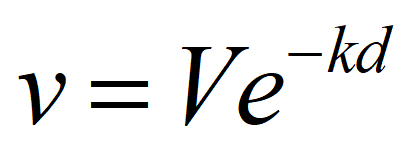
Discuss what this formula is representing.

This formula represents hyperbolic discounting

• v = subjective discounted value

• k = the discount rate

• d = the delay

This accommodates dynamic inconsistencies in exponential functions for discounting.

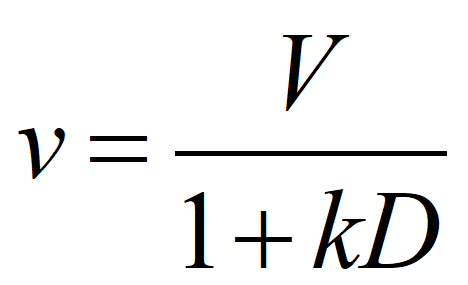
Discuss why people (and other animals) discount the future?

• Interest rates: if you take an immediate gain you can then invest that, and potential receive a higher rate of return than the delayed reward

• Uncertainty: future is uncertain (e.g., bank holding your investment could collapse, or you may not survive the many months to receive the better food reward)

Discuss how Loewenstein (1996) explains why we discount the future.

Loewenstein (1996) suggest temporal and physical proximity of options can reduce aversive states (e.g., hunger) disproportionately increase attractiveness of those options.

• They argue people with low discount rates are differing the way they anticipate the regretfulness of an outcome, rather than exhibiting self-control.

• This model predicts discount rates should be different when making decisions on behalf of other people.

Discuss how Ziegler and Tunney (2012) explained how people discount the future.

Ziegler and Tunney (2012) aimed to test the emotion-based theories prediction (we have different discount rates when making decisions for other people).

• Results: demonstrated that discount rate varies as a function (as a measure of social proximity), greater impulsivity when making decisions for ourselves. Except decisions for close social groups, strangers are given our least impulsive decisions.

Discuss research by Stillwell and Tunney (2011) into discounting as impulsivity

Research by Stillwell and Tunney (2011) tested 9454 Facebook users around the world to determine relationship between discounting and smoking.

• Results: showed that daily smokes discount future rewards more so than irregular or none smokers.

Discuss the ultimatum game.

The Ultimatum Game is a task where two players decide how to split some money, one player is given a sum and has to share this, if the other player declines, both players lose all the money, they cannot communicate prior.

• RCT suggests the responder should always accept the minimum given often (maximises expected utility), although not supported empirically.