Voltage and Current Laws

MEE1008: Dynamic Systems 1 - Lecture 2

Kirchhoff’s Current Law

At any node (junction) in an electrical circuit, the sum of the currents flowing into that node is equal to the sum of currents flowing out of that node.

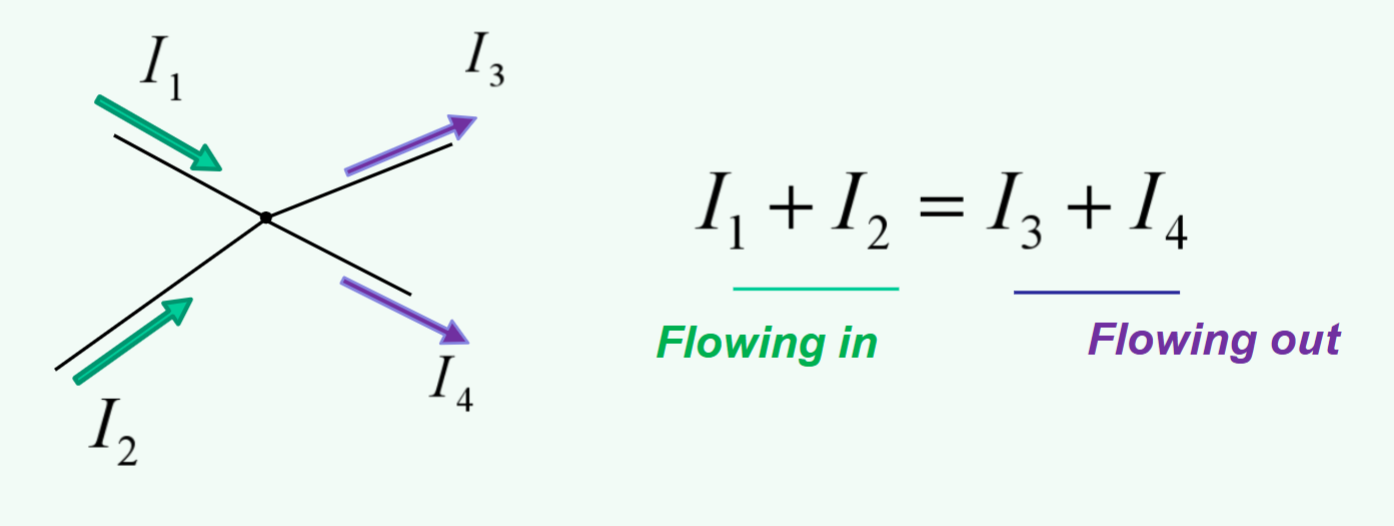
Current flowing in the opposite direction in a juncition

Is represented with a minus algebraically.

More complex junction calculations

Where n: conductors in and k: conductors out.

The fundamental property of charge results in Kirchhoff’s current law

Kirchhoff’s current law is a consequence of charge conservation principle - the net charge in the wires and components of a closed circuit is constant.

Ohm’s law

The current (I) through a conductor between two points is directly proportional to the voltage (V) across the two points. The constant of proportionality is the conductor resistance (R).

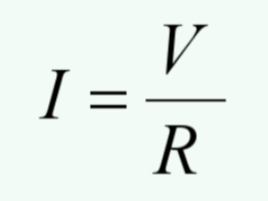
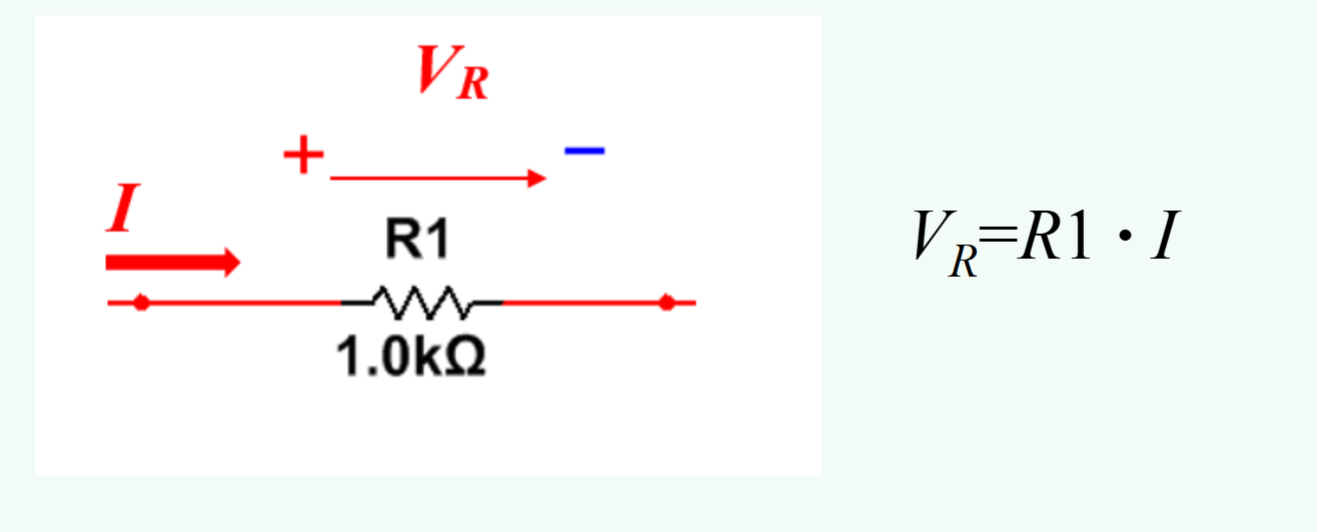
Ohm’s law with voltage drops

Electric current (I) from an external source flows through the resistor R1. There is voltage drop VR across this resistor which relates to current I and resistance R1.

Ohm’ Law: Voltage

Describes voltage generated by active source, e.g. battery.

Ohm’s Law: Voltage Drop

The decrease of electric potential due to resistive load.

Devices that don’t obey Ohm’s Law

Diode, battery.

Kirchhoff’s Voltage Law

For a closed loop series path the algebraic sum of all the voltages around any closed loop in a circuit is equal to zero.

Take into account the polarities and signs of the sources and voltage drops around the loop.

Another Kirchhoff’s Voltage Law formulation

For a closed loop series circuit the algebraic sum of all the voltage drops equals to the sum of voltage rises (active sources).