A.5. Special Relativity (HL)

Formulae and Definitions (HL Only)

Reference Frame

A reference frame is a set of coordinate axes and clocks at all points in space. A non-accelerating frame is called an inertial frame of reference.

Galilean Distance Transformation

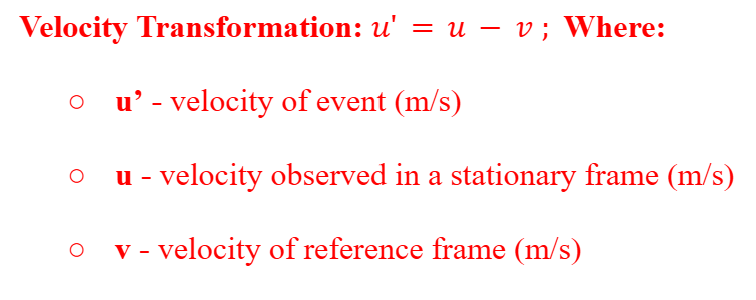
Galilean Velocity Transformation

Galilean Time Transformation

Postulates of Special Relativity

• All laws of physics remain the same in all inertial frames.

• The speed of light in a vacuum is constant in all frames.

Lorentz Factor

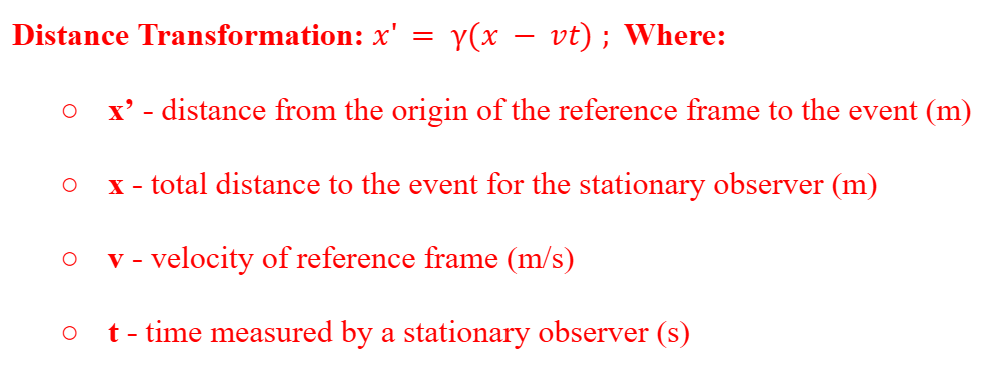
Relativistic Distance Transformation

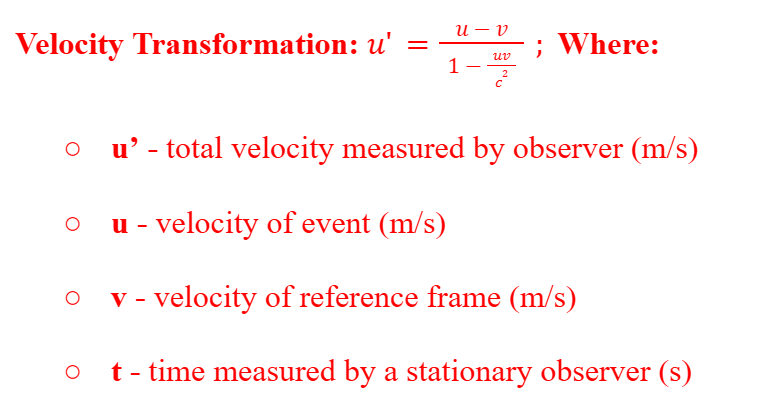
Relativistic Velocity Transformation

Relativistic Time Transformation

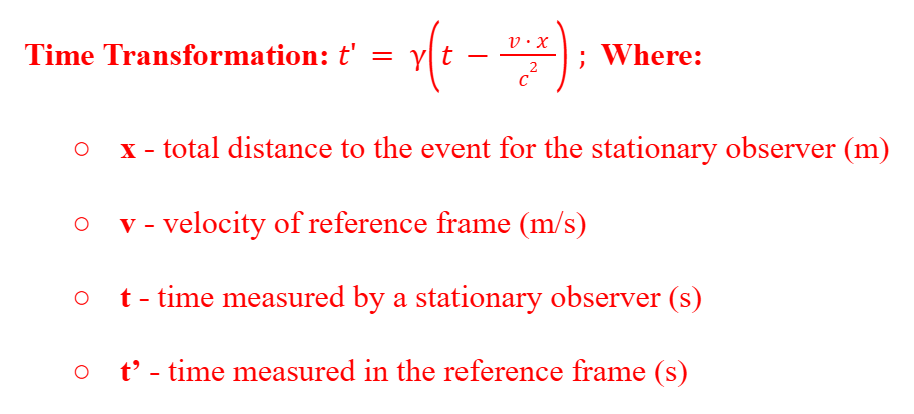
Spacetime Interval

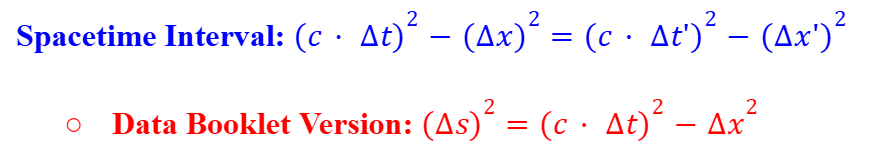
Spacetime Coordinate Differences

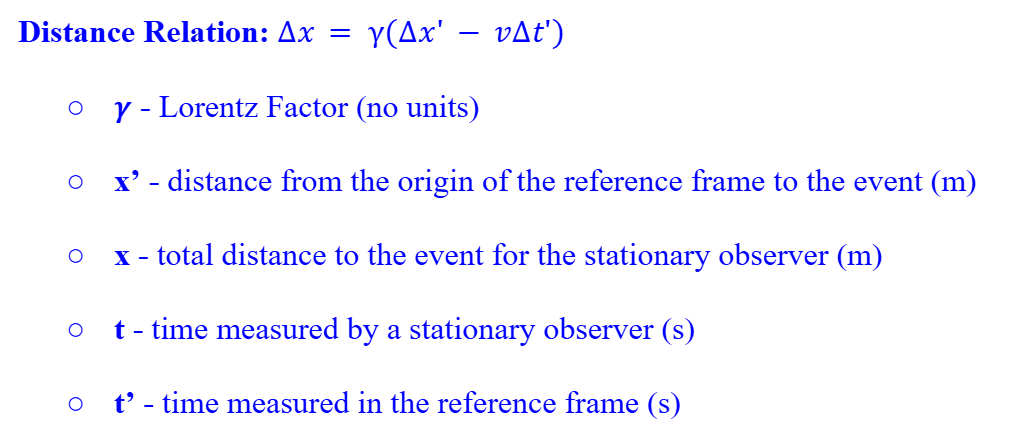
Distance Relation

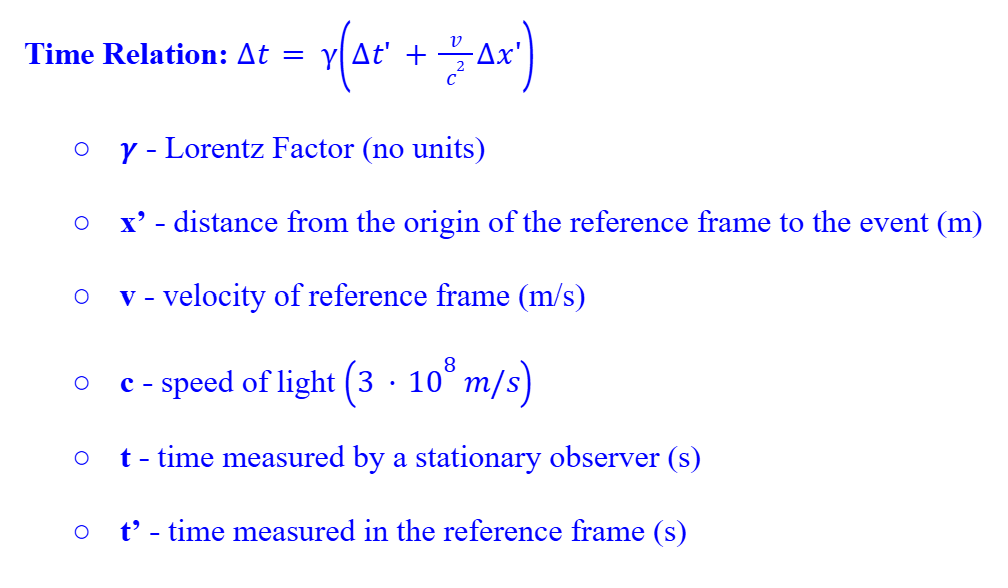
Time Relation

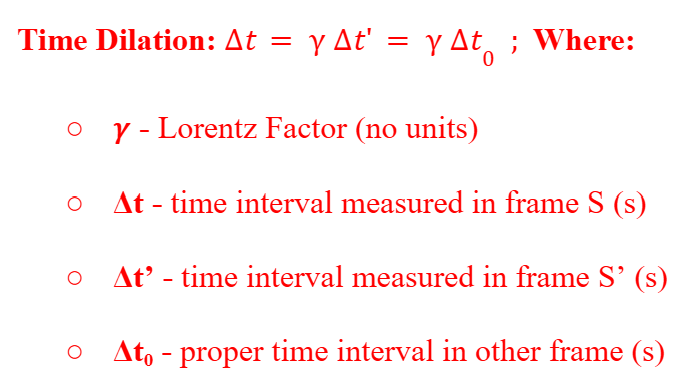
Time Dilation

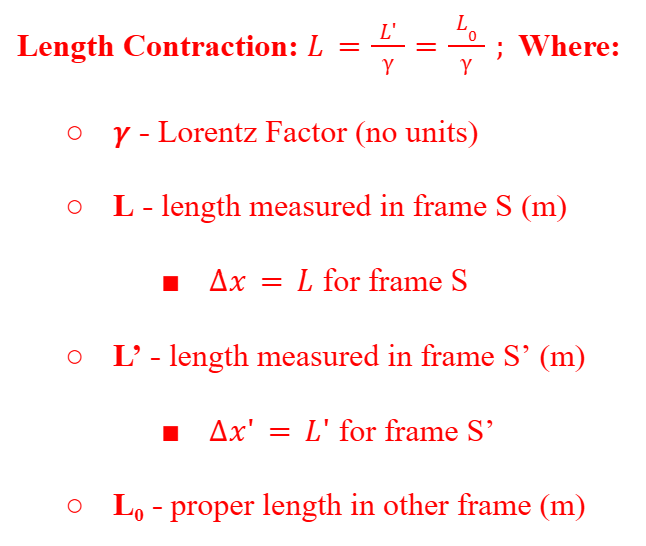
Length Contraction

Proper time and Proper Length

• A proper time interval is the time between two events that take place at the same point in space.

• A proper length is the length measured when the object is at rest. The object's rest frame is the reference frame where the object is at rest. Only the lengths in the direction of motion are contracted.

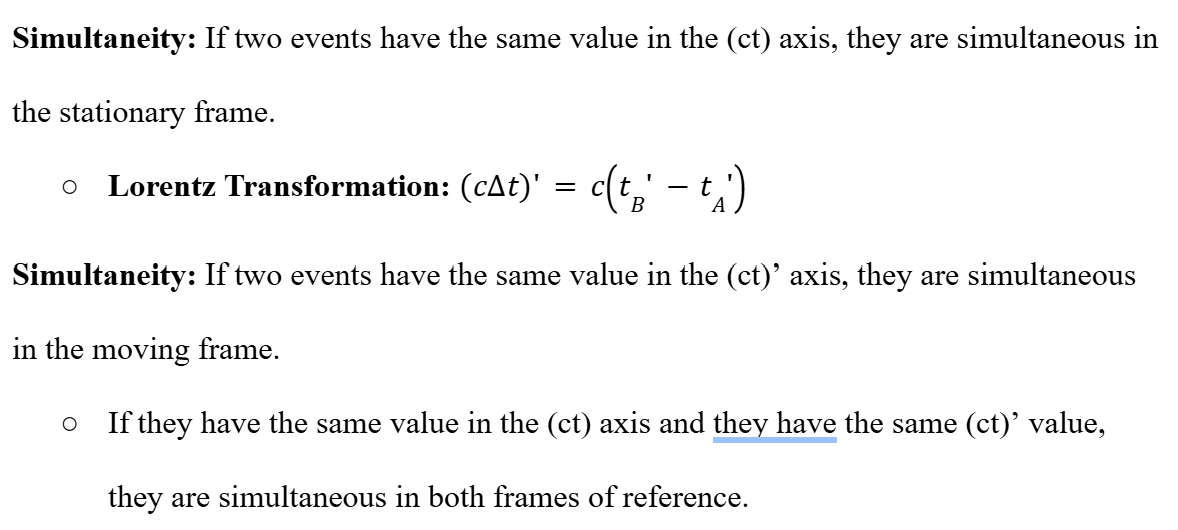
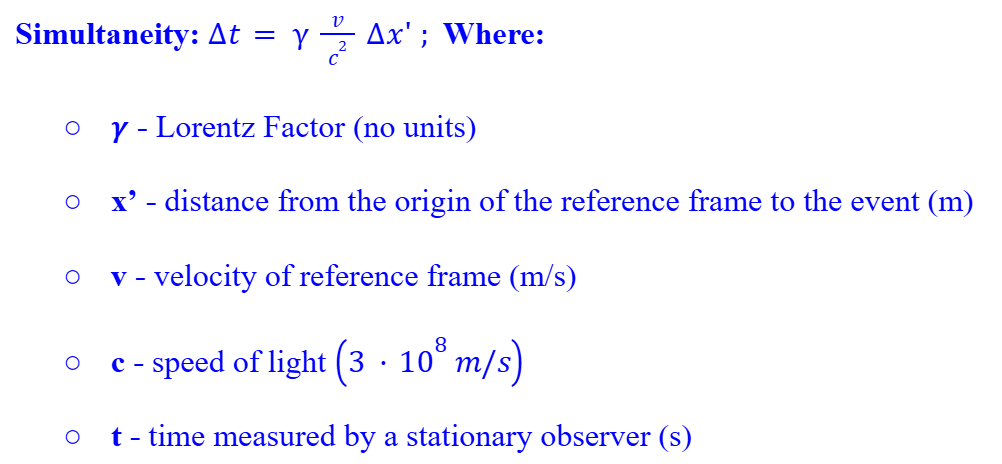
Simultaneity

If two events occur simultaneously in frame S’ with a time interval of 0, then the following equation is the time interval in frame S.

If x' = 0, the events will not be simultaneous in other frames.

If x = 0 i.e. the simultaneous events occur at the same point in space, thus they are simultaneous in all frames of reference.

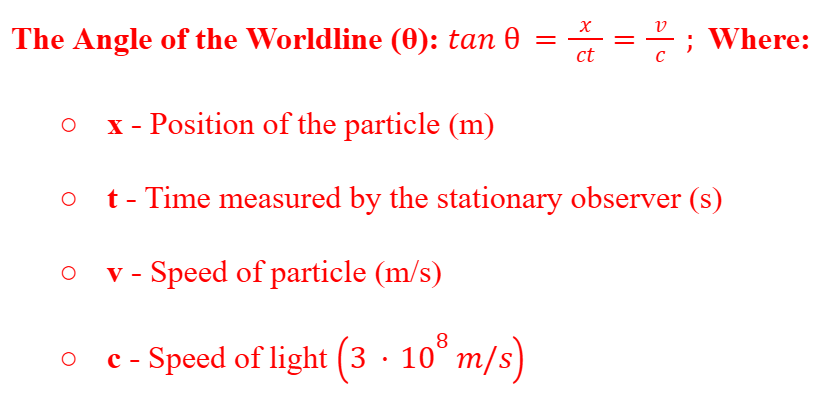
Angle of the Wordline

The angle of the wordline cannot exceed 45^o.

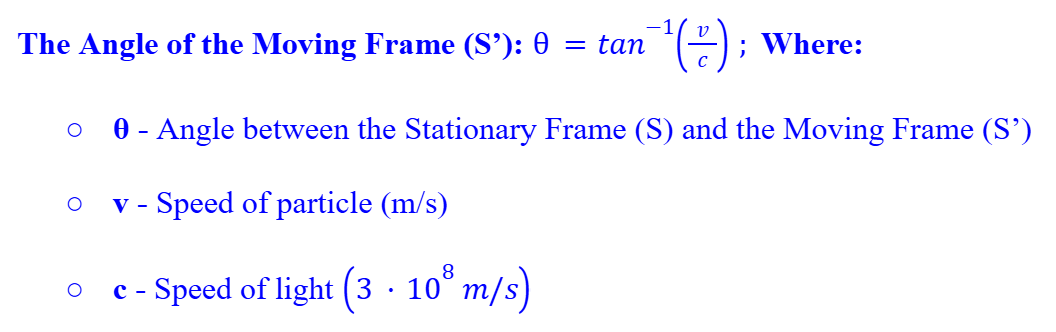
Angle of Moving Frame

The angle of the moving frame cannot exceed 45^o.

Causation in Spacetime Diagrams

An event ‘E’ can cause event ‘L’ if the time separating ‘E’ and ‘L’ is greater than or equal to the travel time of a photon from the position of ‘E’ to ‘L’.

Scale Relation of Axes in Different Frames

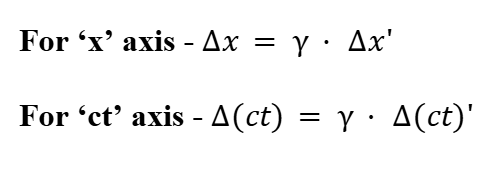
Length Contraction in Spacetime Diagrams

• Draw a line parallel to the (ct)’ axis at the start and end of the length in the moving frame and extrapolate it towards the x-axis in the stationary frame to find the proper length.

• Draw a line parallel to the (ct) axis at the start and end of the length in the stationary frame and extrapolate it towards the (x’)-axis in the moving frame to find the contracted length. 

• Lengths are only contracted when in the direction of the velocity.

Time Dilation in Spacetime Diagrams

• Draw a line parallel to the x-axis from the start and end points of (ct)’ in the moving frame and extrapolate it towards the (ct) axis to find the proper time.

• Draw a line parallel to the (x’)-axis from the start and end points of (ct) in the stationary frame and extrapolate it towards the (ct)’ axis to find the dilated time.

Simultaneity in Spacetime Diagrams