Chapter 4: Specific factors and Income Distribution

The specific factors model

Assumes an economy that produces 2 goods and where labour (the mobile factor) can move freely between these two industries.
Some resources are fixed (specific) and can only be used for production in ONE of the industries.

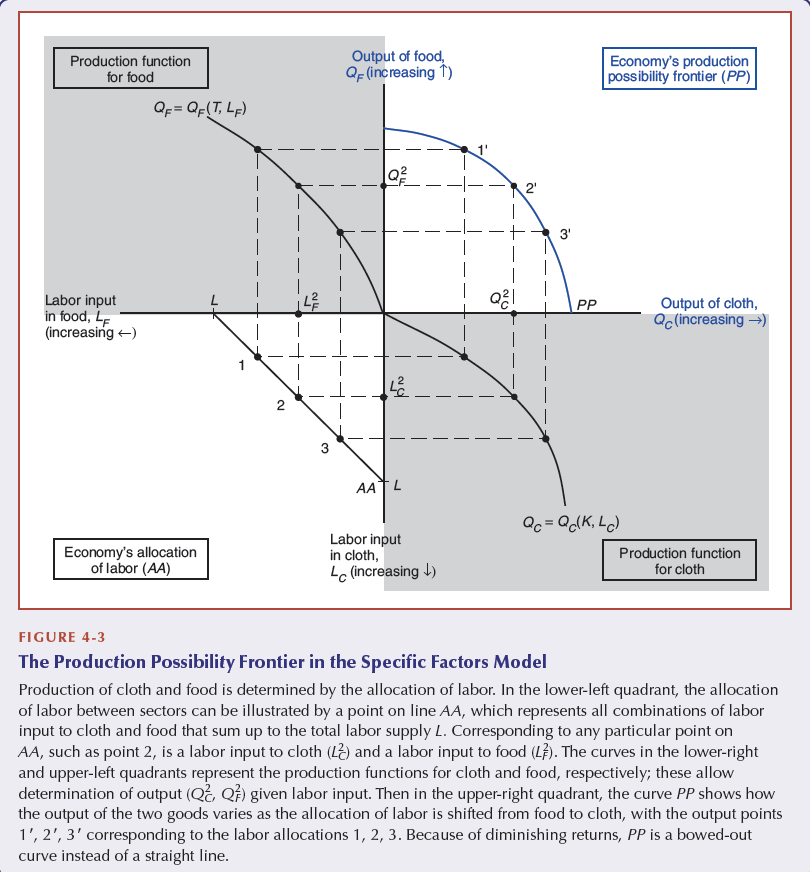
Production functions

The book example uses 3 factors, Capital (K), Labour (L), and Land (T)
One good (cloth, C) only uses labour, but not land and food use land, but not capital.

The production function (for cloth) is given by: Q_c=Q_c(K,L_c)
The production function (for food) is given by: Q_f=Q_f(T,L_c)

Where Lc+Lf=L

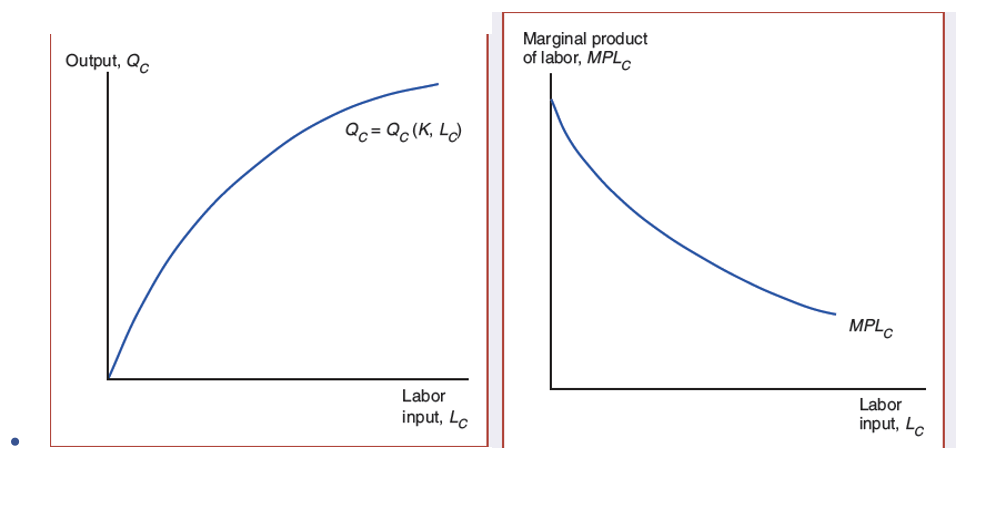
Diminishing returns to scale

If only one resource is increased (let's say L) without increasing the other (K), the output per unit of input will increase less and less as more workers have less to work with

Marginal Product of Labour (MPL)

the output generated by increasing the input of labour by one

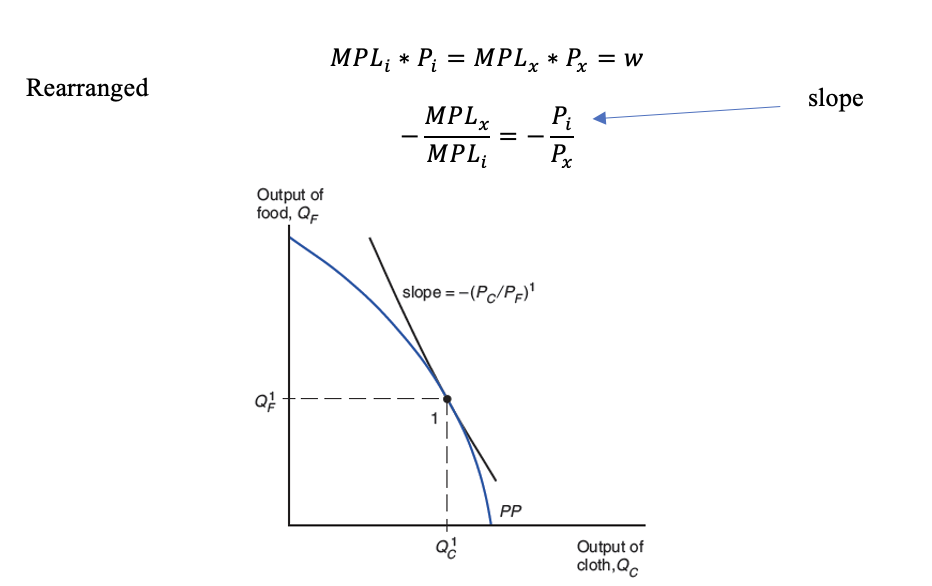
Slope of PP curve

given by =-MPLf/MPLc (when it is food in terms of cloth)
the opportunity cost of marginal labour productivity

wage rate of labour

given by MPL_i*P_i=w_i
employers will hire up until this point
if the price decreases, more workers hired

Allocation of labour

Equilibrium

Where the the slope of the curve equals =-Pi/px

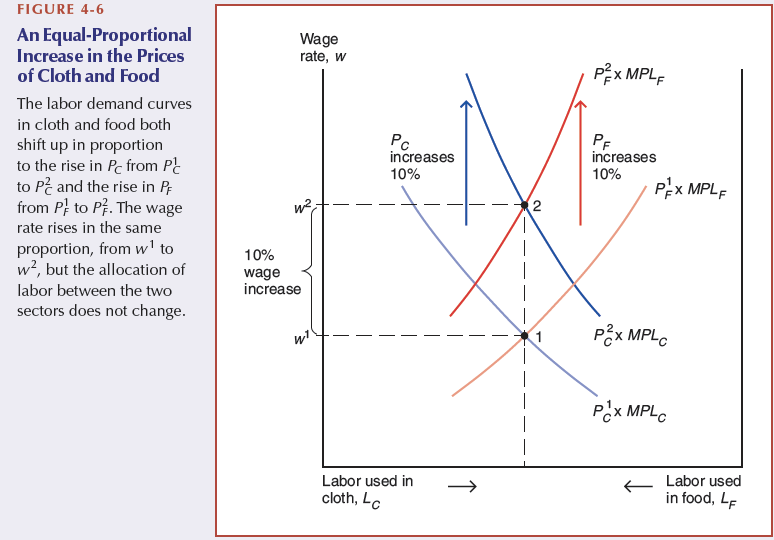
Equal-proportional increases in prices

wages increase, but labour allocation stays the same

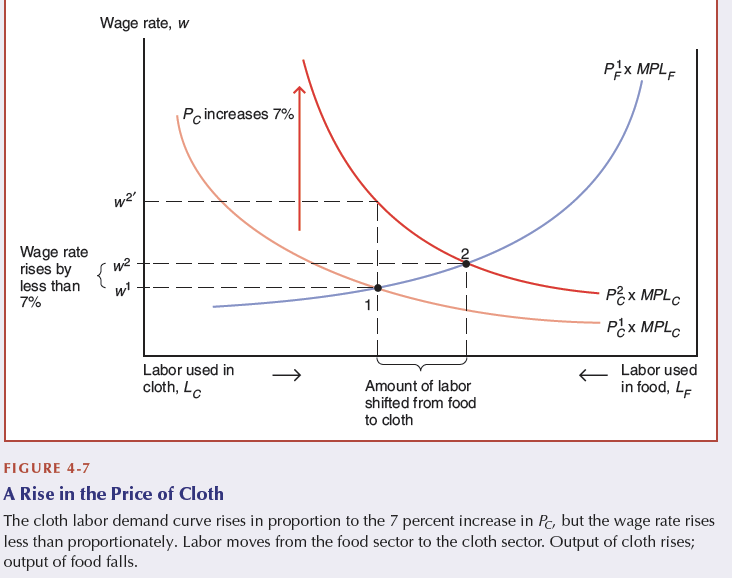
Changes in relative prices

Labour moves from one sector to another

The relationship between output and relative prices

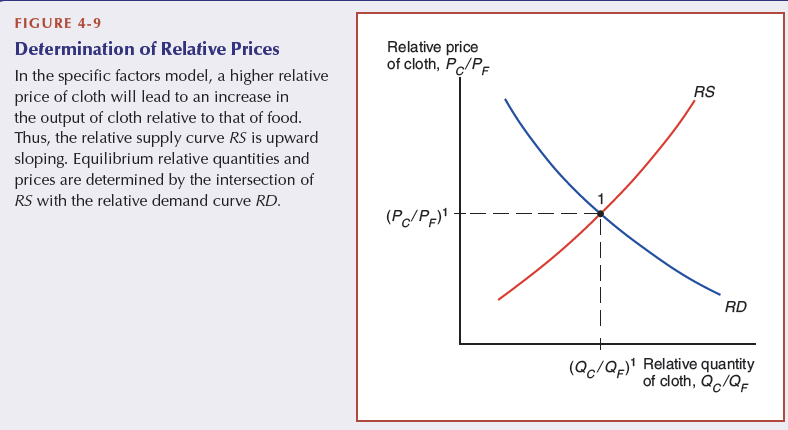
Determination of relative prices

Who gains from trade in the specific factors model?

The factor specific to the sector whose relative price increases is definitely better off.

The factor specific to the sector whose relative price decreases is definitely worse off.

The change in welfare for the mobile factor is ambiguous.

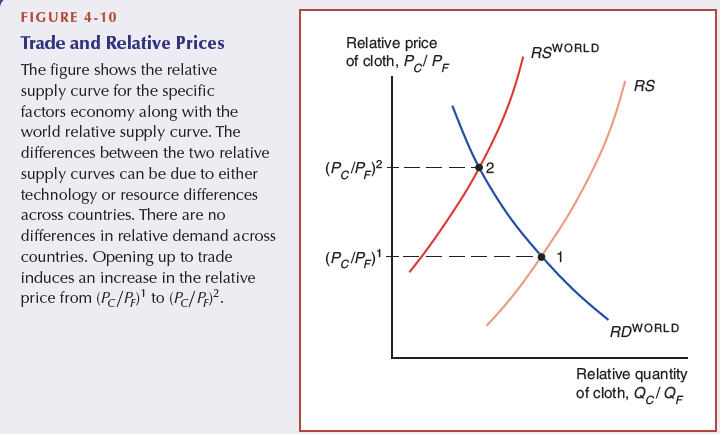
Trade and relative prices

For trade to take place:

Free trade P ≠ autarky P

in free trade, Home will export the good where the relative prices have increased and will export the good where the relative price have decreased

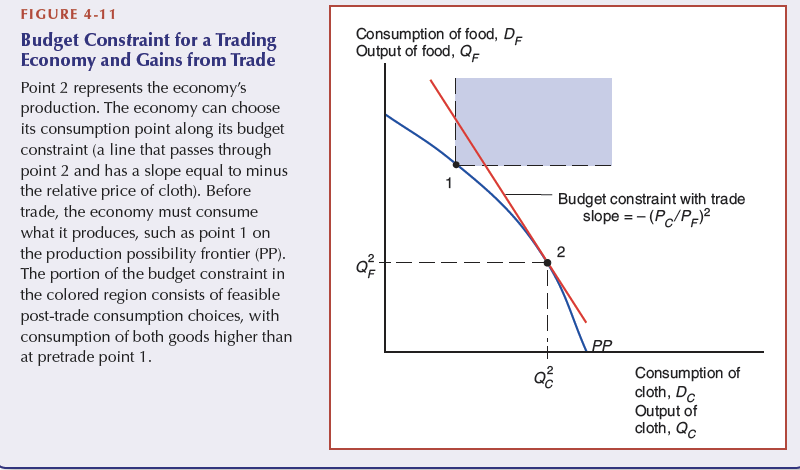
Aggregate gains from trade

In absence of trade, the output, Q, must equal the consumption (demand), Di = the demand for a good.

Qi=Di

Free trade allows different consumption patterns. But a country CAN NOT spend more than they earn. The value of production must be equal

Pi * Di + Px * Dx = Pi * Qi + Px * Qx

can be rearranged to show the imports of "i" in an open economy when demand exceeds production. The budget constraint equation is rewritten from the equation above:

Di - Qi = (Px/Pi) x (Qx/Dx)

A country must export more of the other good to afford the imports:

(Px/Pi) x (Qx/Di)

shows the relative price of the export good and the amount that exceeds own consumption

Budget constraint slope

=-(Px/Pi)

Losers from trade

The immobile factor owners in the (now) import-industry

Real world: Low-skilled workers in developed countries have a hard time transitioning into high-skilled labour that is demanded in their export industry. The people who are losing from trade, will protest it >< winners are less vocal

→ Solution? safety net for low-skilled workers

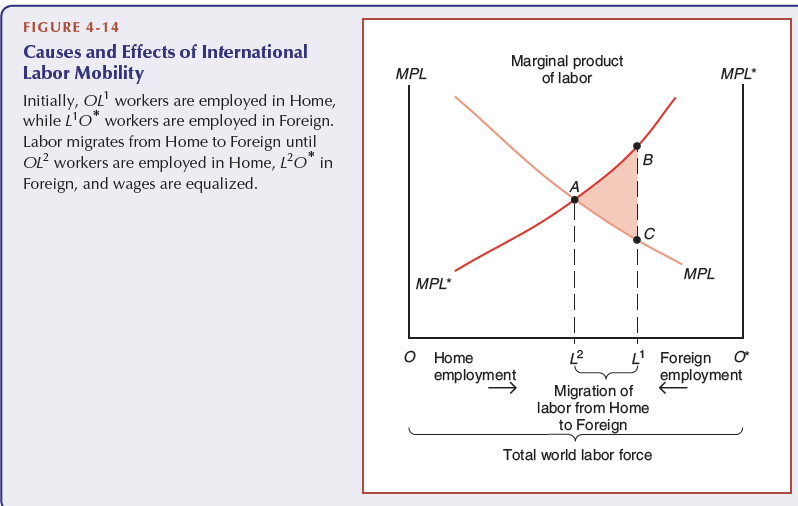
International labour mobility