(10)Linear Kinetics

Overview

1. Newton’s Laws of Motion

   1. Law of Inertia

   2. Law of Acceleration

   3. Law of Reaction

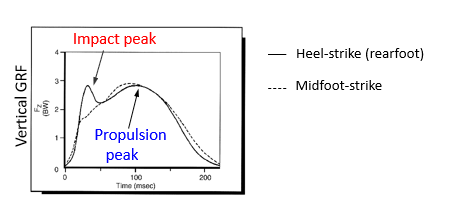
      • Ground Reaction force

2. Law of Gravitation

   • Weight

3. Friction

4. Momentum and Impulse

   • Momentum-impulse relationship

5. Mechanical work, power and energy

   • Work-energy relationship

1st Law: Law of Inertia

A body remains at current state of motion (constant velocity) unless acted upon by an external force

1st Law: Inertia (statics)

• the tendency of an object to keep the current state of motion

• Difficulty in changing the state of motion

• Proportional to mass of the object

  • Mass=measure of inertia in linear motion

2nd law: Law of Acceleration

An external force applied to a body causes acceleration

F=ma

2nd Law: Acceleration

Acceleration

• proportional to force

• inversely proportional to mass (linear inertia)

• a=F/m

Direction of a= Direction of F

If F=0 —→ a=0

• constant v

• no change in state of motion

• law of inertia

• a= F/m=0

3rd Law: Law of Reaction

For every force (action), there is an equal and opposite reaction

3rd law: Reaction & Examples

Reaction

• Same magnitude

• opposite direction

Examples

• Bullet vs Gun

• Fist Fighting

• Propulsion in swimming

• Hockey Players

Ground Reaction Force

GRF

• reaction supplied by the ground

• passive force

Important for human motion

• major external force that accelerates the body

  • speeds up

  • slow down

• the only external force one can voluntarily control

  • weight is always constant and downwards

Examples of GRF

• High jump

  • ax=Fx/m < 0

    • slow down of rightward (+) Velocity

  • ay=Fy/m>0

    • slowdown of downward (-) velocity

    • speed up of upward (+) velocity

• Running

Law of Gravitation

• All bodies are attracted to one another due to their masses

• G= Gravitational constant= 6.672×10^-11 Nm²/kg²

Gravitation

• proportional to masses

• inversely proportional to (separation distance)²

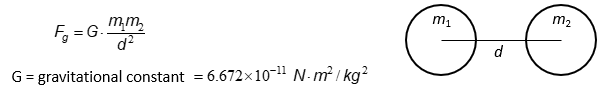
Weight

• Gravity Exerted to a body by the earth

• Weight= (mass)(gravitational acceleration)= mg

• Direction- downward

• passes through the COM of the body

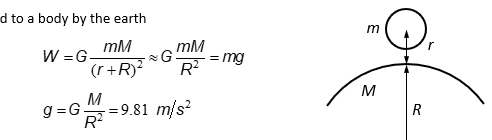
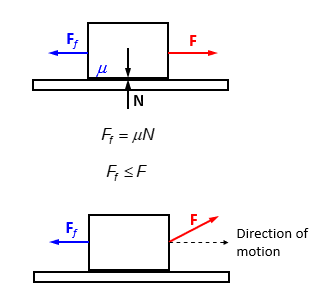
Friction

Friction

• force acting at the area of contact between two surfaces

• Source of horizontal GRF

Magnitude

• proportional to

  • normal reaction force (N)

  • friction coefficient (Mu)

• Cannot exceed your force

Direction

• opposite that of motion or motion tendency

Sliding vs Rolling Friction- Nature of interaction

Sliding friction

• friction due to relative motion of the surfaces

Rolling Friction

• friction due to temporary deformations of the surfaces

Sliding vs rolling

• sliding friction>> rolling friction

• Beneficial to convert sliding to rolling for smaller friction

EXAMPLES

• Wheels

• how they built the pyramid of giza (rolling the stones over logs to get to the location

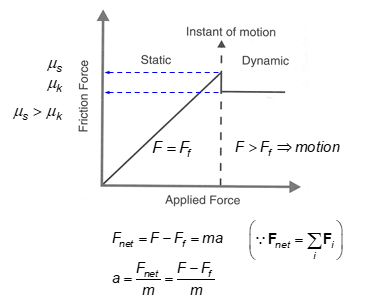
Static vs Kinetic (Dynamic) Friction — State of motion

Static Friction

• F_f experienced with no motion (F=F_f)

• Max Static friction= force required to initiate motion

Kinetic (Dynamic) friction

• F_f experienced during motion (F>F_f)

• force required to maintain motion once initiated

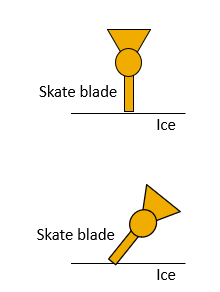
Friction Strategies

Minimize friction as resistance

• Lubrication- synovial fluid (mu=0.01)

• Skating-gliding

• tire inflation and paving

Maximize friction as source of propulsion

• source of horizontal GRF

• cleats: Prevent slipping

• Skating: Push off

Momentum

• Amount of motion

• (inertia)(velocity) M=mv

• important in giving and receiving impact, collision, etc

• Vector quantity

  • direction=direction of velocity

• Unit: kg*m/s

Principle of momentum conservation

• If no external force, total momentum remains constant

• a=Fnet/m = 0

• a=delta v/ delta t= 0

• v= constant M=mv= constant

• from netwons first law

• example: Hockeyplayer

Impulse

• The accumulated effect of force exertion over a period of time I=F(bar)*delta t

• vector quantity: direction=direction of force

• Unit: Ns (same as momentum)

• causes change in Momentum

  • momentum impulse relationship

  • I= Delta M= M2-M1=mv2-mv1= m(v2-v1)= m*delta v

Impulse strategies

• Giving impulse ( Batting, throwing, etc.)

  • maximize impulse by maximizing the momentum of the apparatus (batting)

  • elongating elapsed time (throwing)

  • I=F(bar)*delta t= delta M apparatus

• receiving impulse (landing, catching, etc.)

  • reduce impulse if possible

  • reduce impulse force/pressure by increasing time/area

  • F(Bar)= I/delta t P(bar)= F(Bar)/A

Mechanical Work

• Force applied against a resistance

• accumulated effect of force exertion over a displacement

• work=(force)(displacement)

  • W= F(bar)*d*cos theta

• Scalar

• Unit: J (joule)=Nm