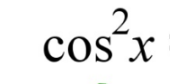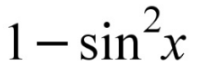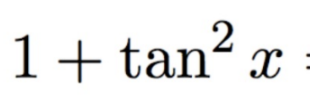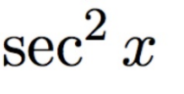Techniques of Integration

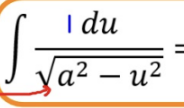
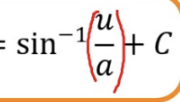
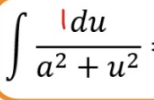
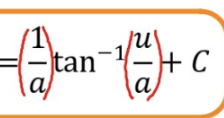
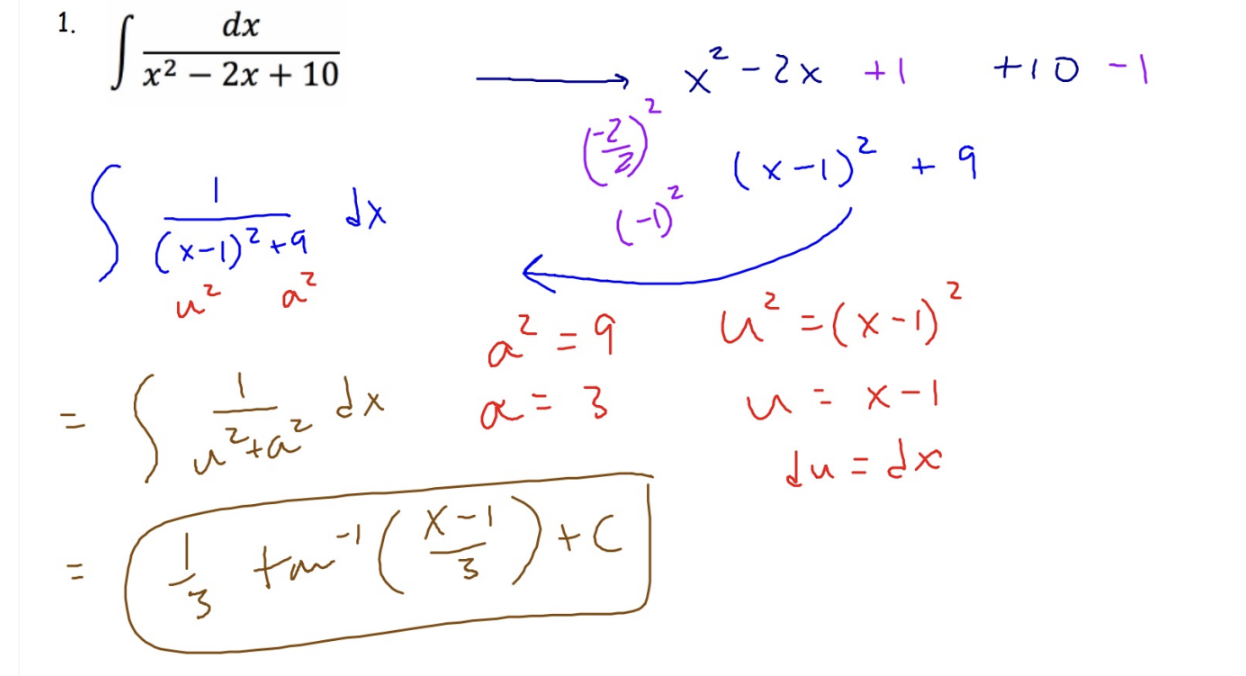
Completing the Square

• Use this method when there’s a constant in the numerator & a quadratic in the denominator

• Most common forms leads us to the inverse trig integrals of sin and tan but not always

Separation

Sometimes we need to split the integral into 2 separate integrals to find the antiderivative (one term in the denominator and 2 or more terms in numerator)

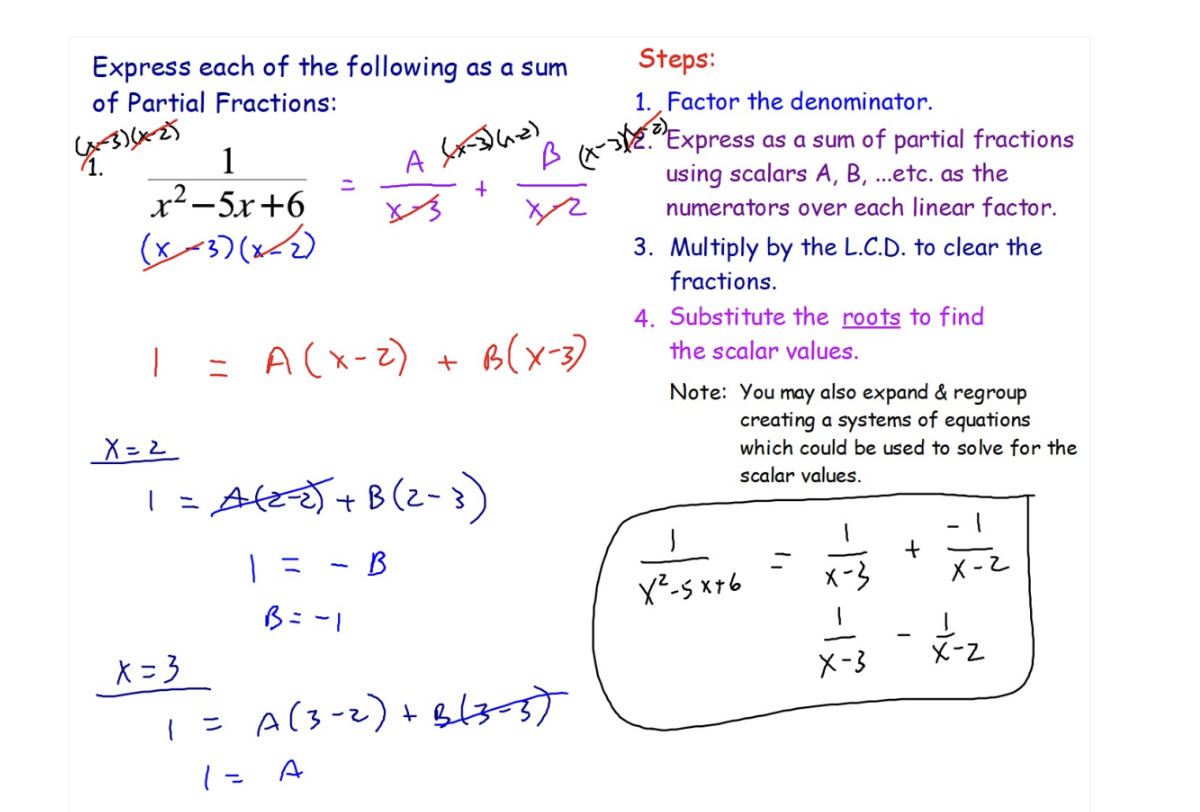
Partial Fractions: Decomposition (Case I: Linear Factors)

The following method only works for Proper Rational Expressions where the degree of the numerator is less than the denominator

Steps/ Example of Partial Fractions: Decomposition (Case I: Linear Factors)

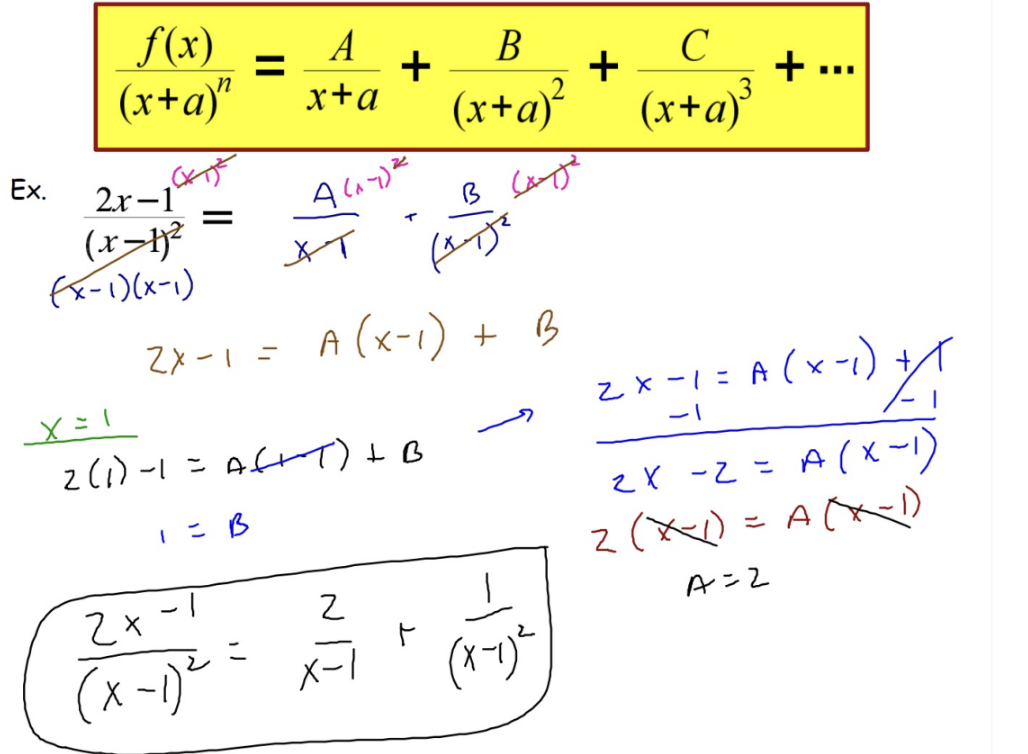
Partial Fractions: Decomposition (Case II: Repeated Linear Factors)

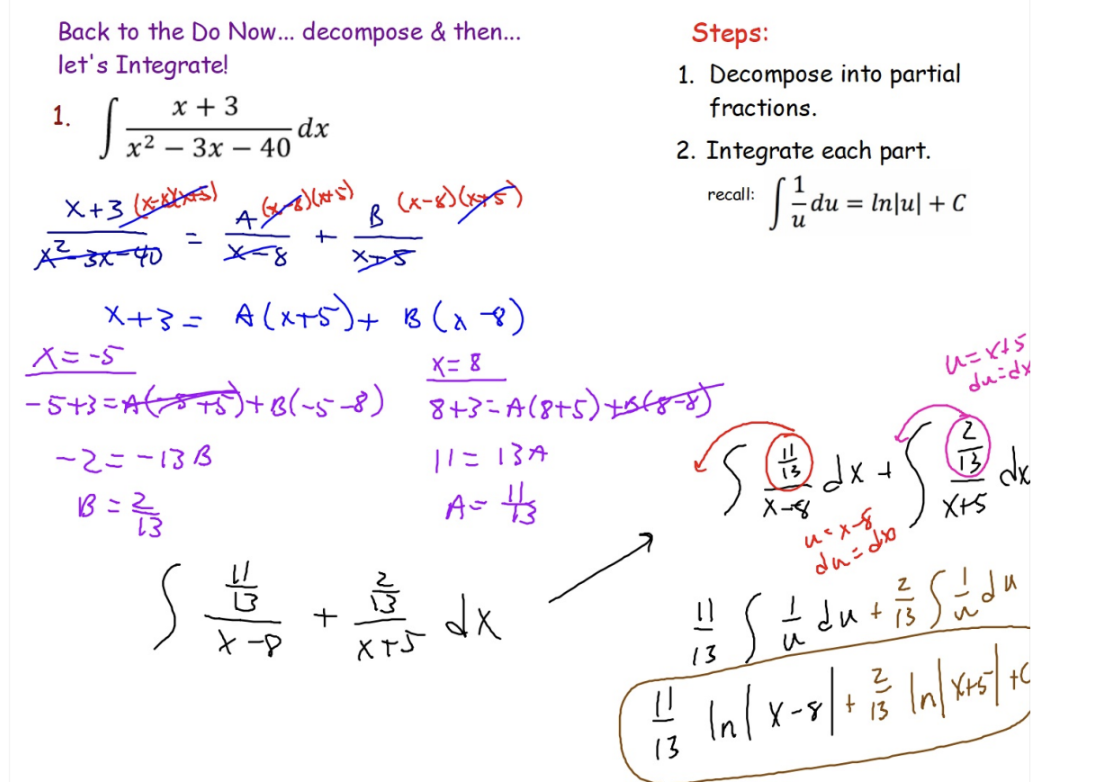
Partial Fractions: Integration

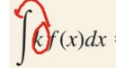
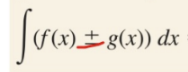
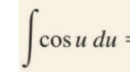
Power Formula

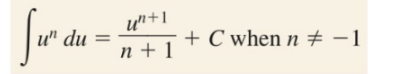
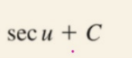
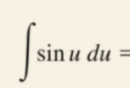
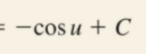
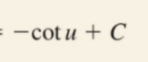
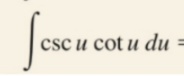
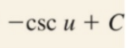
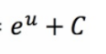
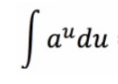
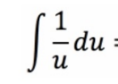
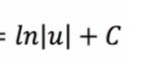
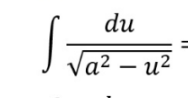
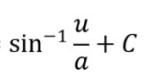
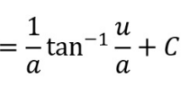
Partial Fractions: Integration (Long Division)

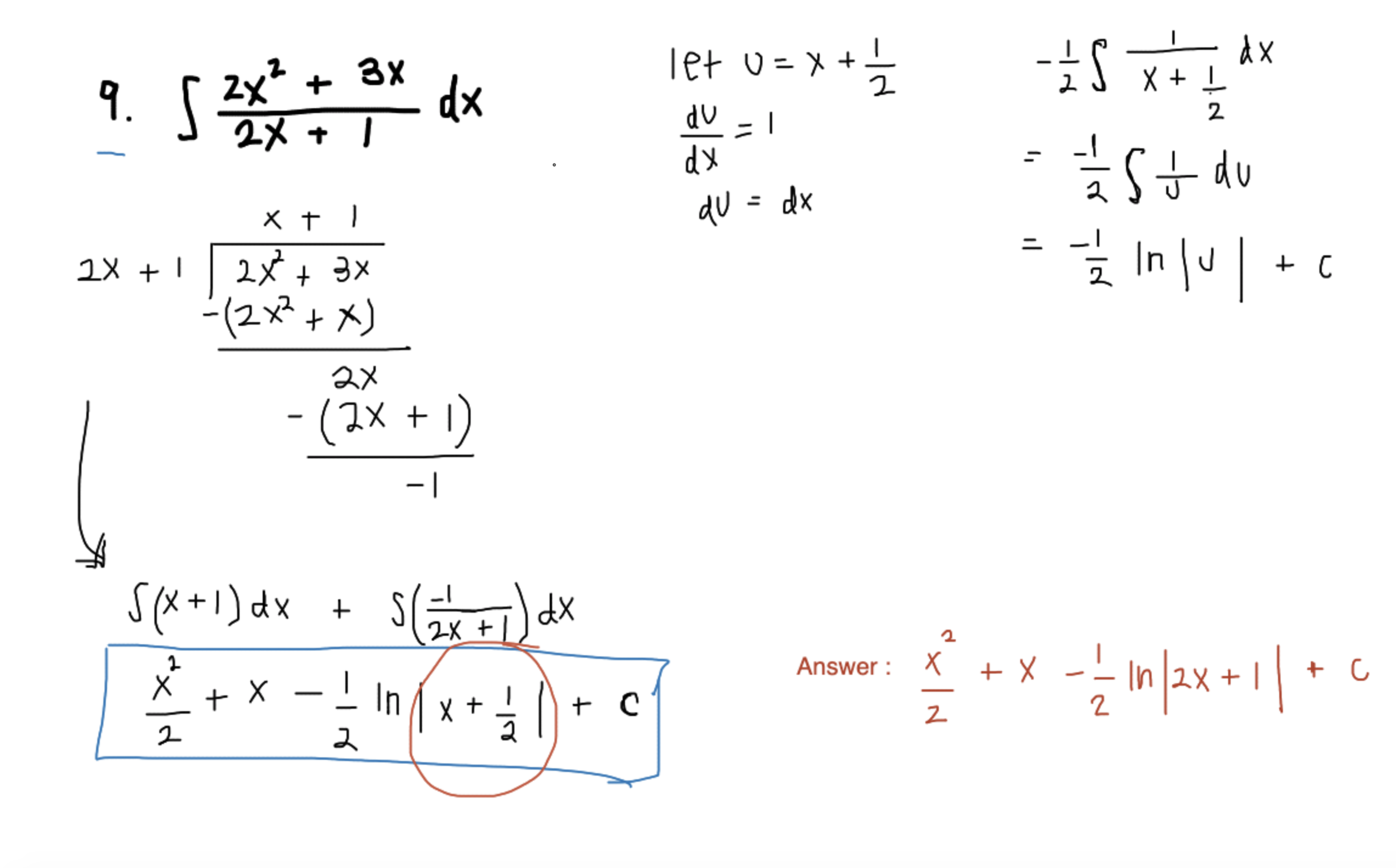
For improper rational expressions (degree of numerator is greater than or equal to degree of denominator) long division is 1st required before you integrate

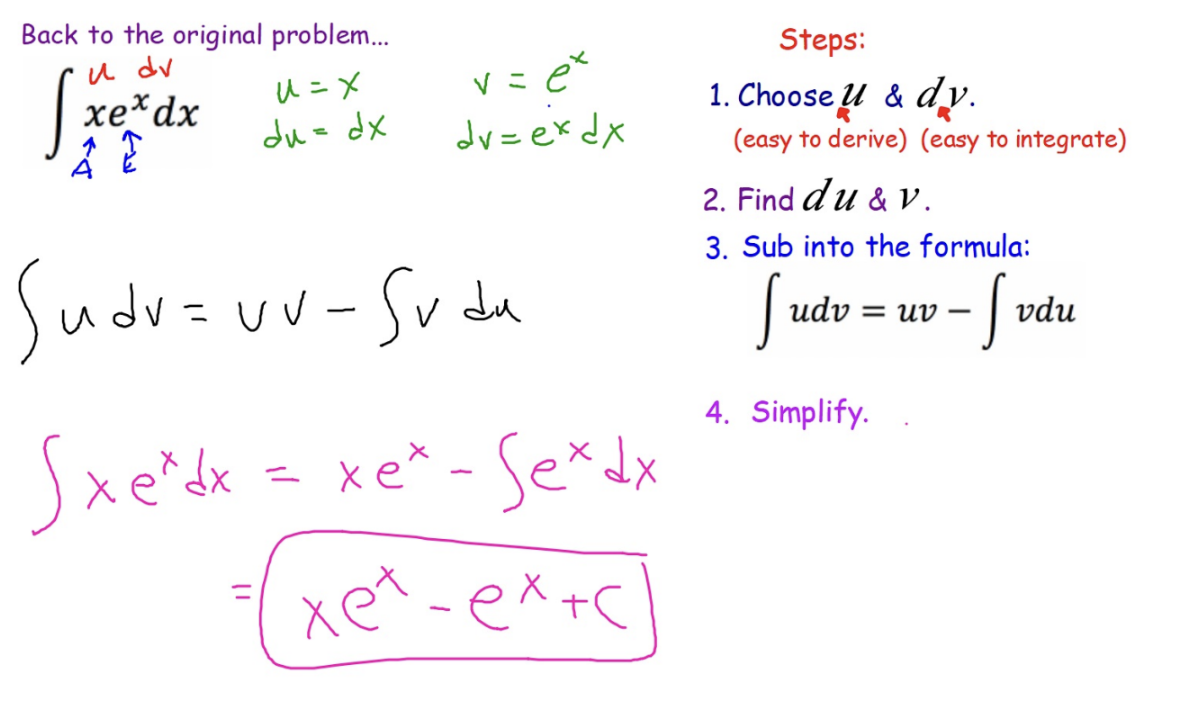
Integration by parts

formula: integral of udv= uv- integral of vdu

Tabular Method

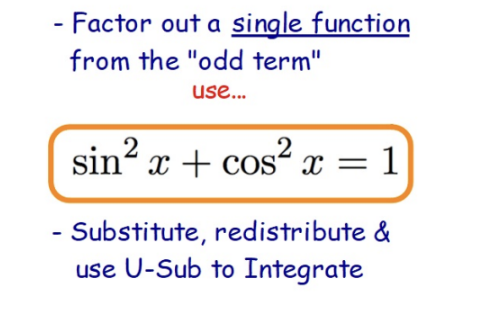
Trig Identity Substitution (odd integers)

Trig Identity Substitution (even integers)