Nth Term Test for Divergence

The Nth Term Test for Divergence is an essential tool for identifying whether an infinite series diverges. Here's a concise guide based on your detailed content:

Purpose:
To check if an infinite series diverges by examining the behavior of its general term a​_n as n→∞.

Test Statement:

Why This Test Works:

• For a series to converge, the general term a_n must approach 0 as n→∞.

• If a_n does not approach 0, the series cannot converge, because the sum of infinitely large terms cannot settle to a finite value.

Steps to Apply:

1. Identify the General Term: Write the formula for a_n​.

2. Compute the Limit: Use algebraic techniques or calculus to evaluate lim_⁡n→∞a_n​.

3. Interpret the Result

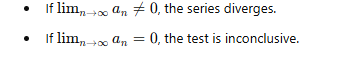
Key Limitations:

• Divergence Only: This test cannot prove convergence. A limit of zero is necessary for convergence but not sufficient.

• Further Testing Required: When lim_⁡n→∞a_n= 0, use additional tests (e.g., Comparison Test, Ratio Test, Integral Test).

Examples:

Example 1: Divergence

Series:

Example 2: Inconclusive

Series:

Common Misunderstandings:

Conclusion:

The Nth Term Test is a quick first step in determining whether an infinite series diverges. It simplifies analysis but must be supplemented with other tests for a complete understanding of series behavior.