Sequences & Series

• Sequence: An infinite succession of numbers that follow a pattern

  • Usually denoted with a subscript using aₙ

  • Usually the terms are generated by a formula, ex. aₙ=(n-1)/n beginning with n=1 is 0, 1/2, 2/3, etc.

    • n is always an integer

• Officially:

  • “A sequence has a limit L if for any ε>0 there is an associated positive integer N such that |aₙ - L| < ε for all n ≥ N. If so, the sequence converges to L and we write lim (n → ∞) aₙ = L”

  • If a sequence has no finite limit, it diverges.

• Infinite Series

• An infinite series is an expression of the form a₁ + a₂ + a₃… + aₖ + …. The numbers a₁, a₂, a₃, etc. are the terms of the series

  • In other words, an infinite series is a sequence of terms where the terms are added up, there is a pattern to the order of the terms, and there are an infinite number of terms.

• As n increases, the partial sum includes more and more terms of the series. So if aₙ approaches a limit as n approaches ∞, then this limit is likely to be the sum of all of the terms in the series, thus a convergence.

  • If the sequence of partial sums converges to a limit S, then the series is said to converge and S is called the sum of the series.

  • If the sequence of partial sums diverges (doesn’t have a limit), then the series is said to diverge which means that a divergent series has no sum.

Geometric Series

• Used in the form of ∞Σ(n=1) a*r^(n-1)

  • These show up often on the AP exam: often asked to determine whether the series converges or diverges, and if it converges, to what limit.

  • If |r|<1, the series converges

  • If |r|>1, the series diverges

• For example, the series (1/2)+(1/2^2)+(1/2^3) converges, while 2+2^2+2^3 diverges.

• If a geometric series converges, you can figure its sum out by doing the following:

  • Multiply the expression by r

    • aₙ = a + ar + ar^2 + ar^3… + ar^(n-1) → raₙ = a + ar + ar^2 + ar^3… + ar^(n-1)

  • Subtract the second expression from the first

    • aₙ - raₙ = a - ar^n

  • Factor out aₙ from the left side

    • aₙ(1-r)=a-ar^n

  • Divide through by (1-r)

    • aₙ=(a(1-r^n))/(1-r)

• If we want to find the sum of the first n terms of a geometric series, we use the following formula:

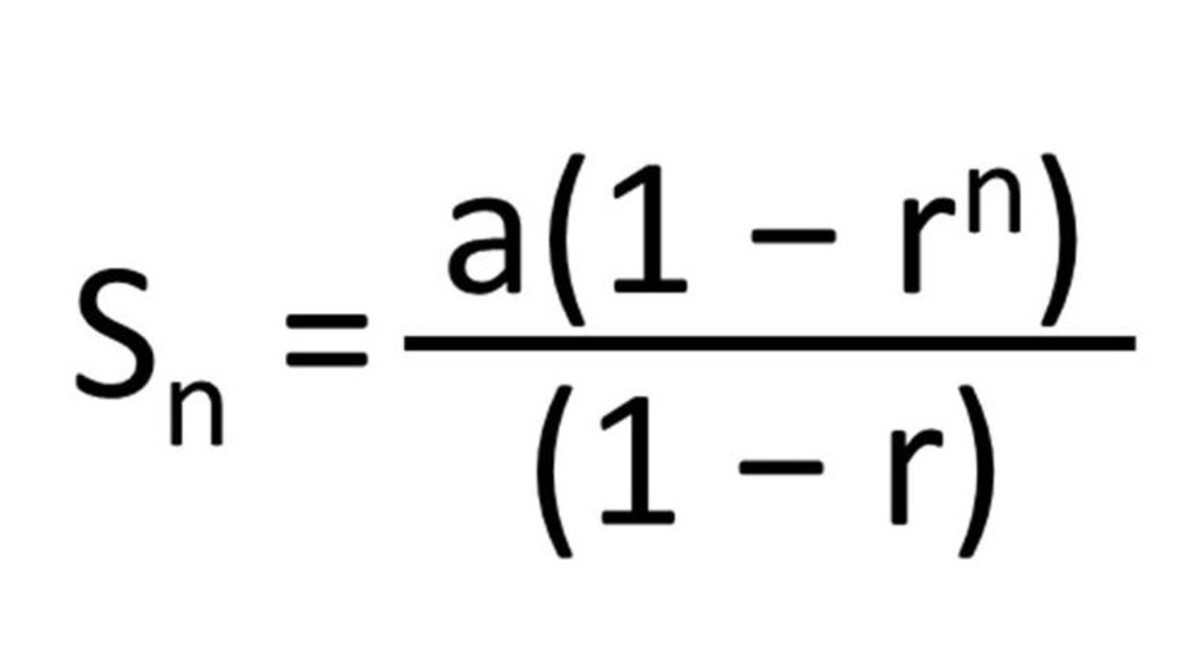
• For an infinite geometric series, if it converges, lim (n→∞) r^n = 0, and its sum is found this way:

  • S = (a)/(1-r)

• The nth Term Test For Divergence

• The nth term test means we find the limit of the series and see if we get zero or not

  • If the limit = L (any number other than 0), the series diverges

  • If the limit = 0, we need to choose a different test

• Integral Test for Convergence

  • If f is positive, continuous, and decreasing for x≥1, and aₙ=f(n), then

Either both converge or both diverge.

• In other words, if you want to test convergence for a series with positive terms, evaluate the integral and see if it converges.

Harmonic Series & P-series

Harmonic Series: (1/n) pattern

• Might look as though it is converging, but it diverges

• The sums are not approaching a limit, so as the sequence of partial sus diverges, so does the sequence of partial sums for the harmonic series

p-Series

• A series of the form (1/n^p) converges if p>1 and diverges if 0<p<1

Tests for Convergence

Comparison Tests for Convergence

• Let 0 ≤ aₙ ≤ bₙ for all n

  • If the summation of bₙ from 1 to ∞ converges, then the summation of aₙ from 1 to ∞ converges

  • If the summation of aₙ from 1 to ∞ diverges, then the summation of bₙ from 1 to ∞ diverges

• Essentially, if all of the terms of a series are less than those of a convergent series, then it too converges. If all of the terms of a series are greater than those of a divergent series, then it too diverges

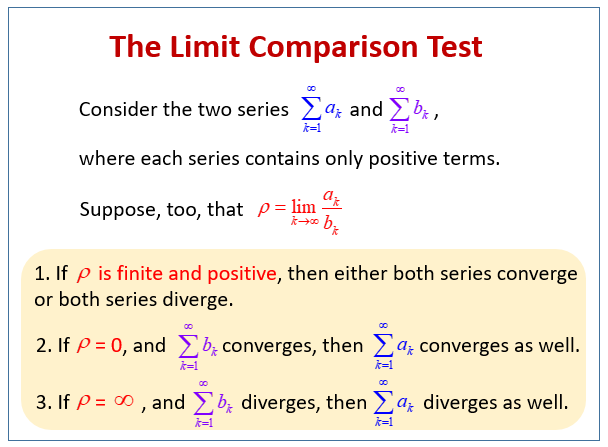
Limit Comparison Test

Alternating Series Test for Convergence

• Used for terms that alternate between positive and negative

• The series using (-1)^(n+1) bₙ converges if the following conditions are all satisfied

  • bₙ > 0 (which means that the terms must alternate in sign)

  • bₙ > bₙ₊₁ for all n

  • bₙ → 0 as n → ∞

Absolute Convergence Theorem:

• The series Σaₙ converges absolutely if the corresponding series Σ|aₙ| converges

Ratio Test for Convergence

Determining Absolute or Conditional Convergence

• Sometimes, an alternating series will converge, but when we look at the absolute value of the terms, it doesn’t.

• An alternating series that is not absolutely convergent is called conditionally convergent

Alternating Series Error Bound

• When summing an alternating series with a finite number of terms, the sum will not be the same as the sum with an infinite number

• If we want to find the error, we need to evaluate | S-Sₙ |

• Can put a bound on the error: a number that the error is less than, which is simply the absolute value of the next term in the alternating series

  • If summing the first 10 terms in an alternating series, the error will be less than the absolute value of the eleventh term

Finding Taylor Polynomial Approximations of Functions

• We can approximate a function on an interval centered at x=a by using a Taylor series (if centered about x=0, it’s a Maclurin series).

• In a Taylor polynomial, the terms are found from thee derivatives of f as follows:

  • If f can be differentiated n times at a, then the nth Taylor polynomial about x=a is

  • pₙx = f(a) + f’(a)(x-a) + (f’’(a)/2!)(x-a)^2+ (f’’’(a)/3!)(x-a)^3+… + (f^(n)(a)/n!)(x-a)^n(x-a)^n

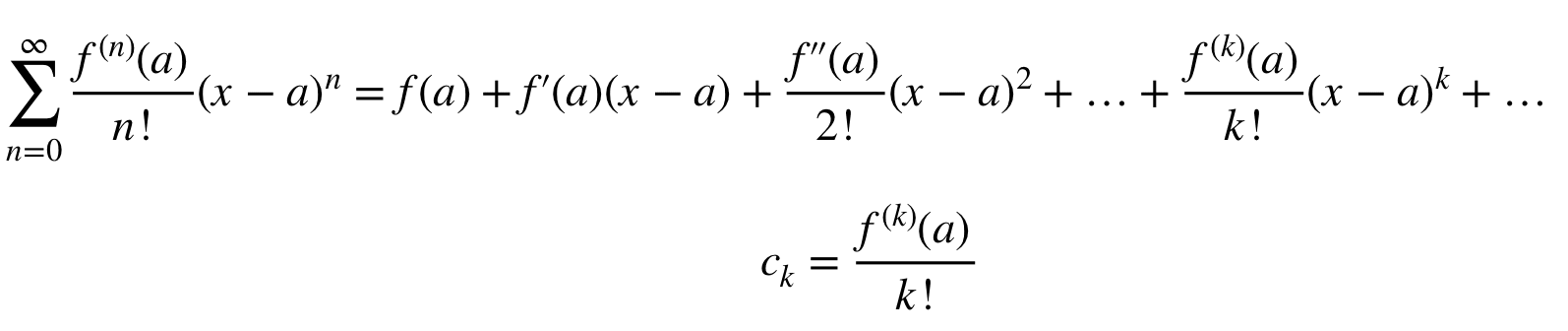
Lagrange Error Bound

• The “error bound” of a Taylor polynomial, aka the Lagrange error

  

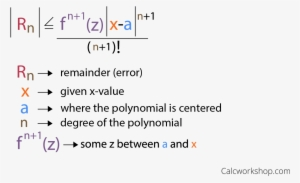
• If you are finding an nth degree Taylor polynomial, a good approximation to the error bound is the next nonzero term in a decreasing series.

Radius and Interval of Convergence of Power Series

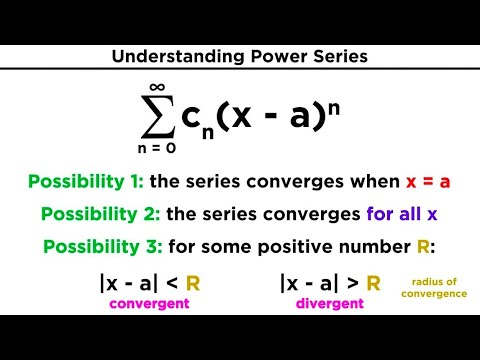
• Going from n = 0 to n = ∞ instead of n = 1 to n = ∞

• Radius of convergence: R.

  • If the series converges only for x=0, the radius of convergence is 0

  • If the series converges absolutely for all x, the radius is all x

  • Set of all values of X (-R, R) is the interval of convergence

Representing Functions as Power Series

• We can use differentiation and integration of power series to find the power series of other functions

• Can approximate the integral using its Maclurin and approximate the solution using the aforementioned series

• Find a new Taylor series from a known Taylor series.