How to Determine Extrema Using the Candidates Test
The Candidates Test is a reliable method for finding the absolute maximum and minimum values of a continuous function on a closed interval. By systematically evaluating both critical points and endpoints, the method ensures no potential extrema are overlooked.
Steps for the Candidates Test
Verify Continuity
Ensure the function f(x) is continuous on the closed interval [a,b].Why? The Extreme Value Theorem guarantees the existence of absolute extrema only for continuous functions on a closed interval.
Find Critical Points
Critical points are where the derivative f′(x) is zero or undefined.Compute f′(x).
Solve f′(x)=0.
Identify points where f′(x) does not exist.
Keep only the critical points within [a,b].
Evaluate the Function at Critical Points and Endpoints
Calculate f(x) for:Each critical point within [a,b].
The endpoints a and b.
Why? Absolute extrema can occur at critical points or endpoints.
Compare All Values
The largest value is the absolute maximum.
The smallest value is the absolute minimum.
Confirm and Interpret Results
Verify the results in the context of the problem. In practical applications, connect extrema to their real-world implications (e.g., profit, cost, efficiency).
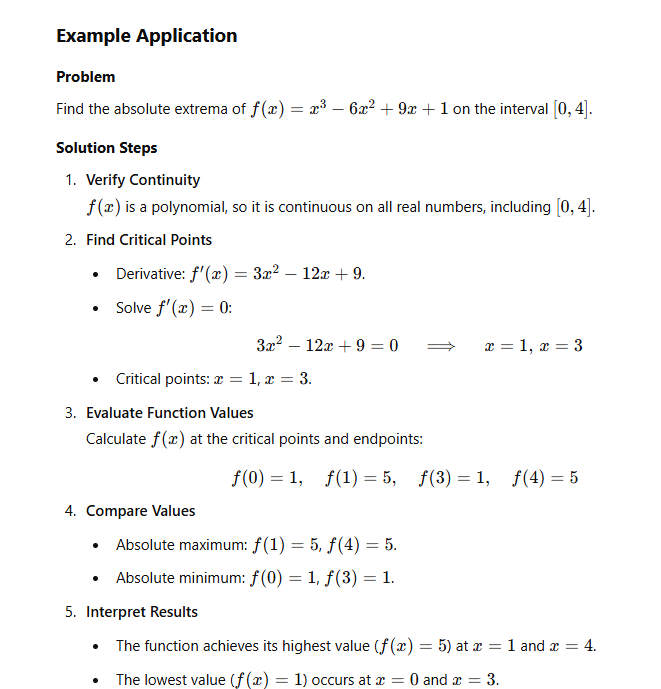
Why the Candidates Test Matters
Simplicity: No need for second derivatives or complex analysis; straightforward evaluation of finite points suffices.
Reliability: Guarantees absolute extrema are found by considering all critical points and endpoints.
Applicability: Works well in practical optimization problems across disciplines such as engineering, business, and physics.
Conclusion
The Candidates Test is a systematic, efficient method for identifying absolute extrema of continuous functions on closed intervals. By leveraging the Extreme Value Theorem and focusing on critical points and endpoints, the method ensures complete and accurate results. Whether solving theoretical calculus problems or optimizing real-world systems, mastering the Candidates Test equips you with a powerful tool for tackling diverse challenges.