Chapter 3 - Electric Force, Field, and Potential

Electric Charge

• Units of charge: Coulombs (C)
  • One proton has a charge of 1.6 x 10^-19 C
  • One electron has a charge of -1.6 x 10^-19
• When an object has more protons than electrons, it’s positively charged
• When an object has more electrons than protons, it’s negatively charged
• Like charges repel and opposite charges attract
• Quanta = the smallest package of a proton or electron that charge comes in
• Atomic Structure
  • Atoms have protons (and neutrons) in the middle and electrons zipping around outside
  • Electrons are easier to remove and in static electricity, we assume only electrons are being removed/added
• Law of conservation of charge - The initial charge of the system will always equal the final charge of the system
• Conductors vs insulators
  • Generally, metals are good conductors and nonmetals are insulators
  • Conductors - allow charge to move easily through them
  • Insulators - don’t allow charge to move easily through them (held in place)
• There are 3 ways to charge an object:
  • Charging by Friction - rubbing two objects like a fuzzy towel and iron rod results in electrons jumping from one object to the other
  • Remember that net charge of the towel-rod system is still the same
  • Charging by Contact or Conduction
  • When a charged object comes in contact with a neutrally charged object, the electrons disperse so that both objects have the same charge sign
    • Bigger objects end up with more charge because they have more room
  • Insulators don’t allow as much charge to disperse through contact as conductors do
  • Induced Charge, Polarization, and Induction
  • Induced charge - a neutrally charged object becomes polarized (electrons clump up on one side of the object and positive charges pile on the other side)
  • In AP Physics 2 questions, a grounding wire is often included
    • The grounding wire essentially serves as an escape route for charges to escape from the polarized object
• Charge Distribution
  • On conductors, excess charges are pushed to the outside of the object to get away from each other
  • On insulators, excess charges stay where they are and don’t disperse

Electric Fields

• Field: a property of a region that can apply force to objects found in that space

• Electric fields affect charged particles only

  • Charged particles in electric fields experience an electric force

    

  \

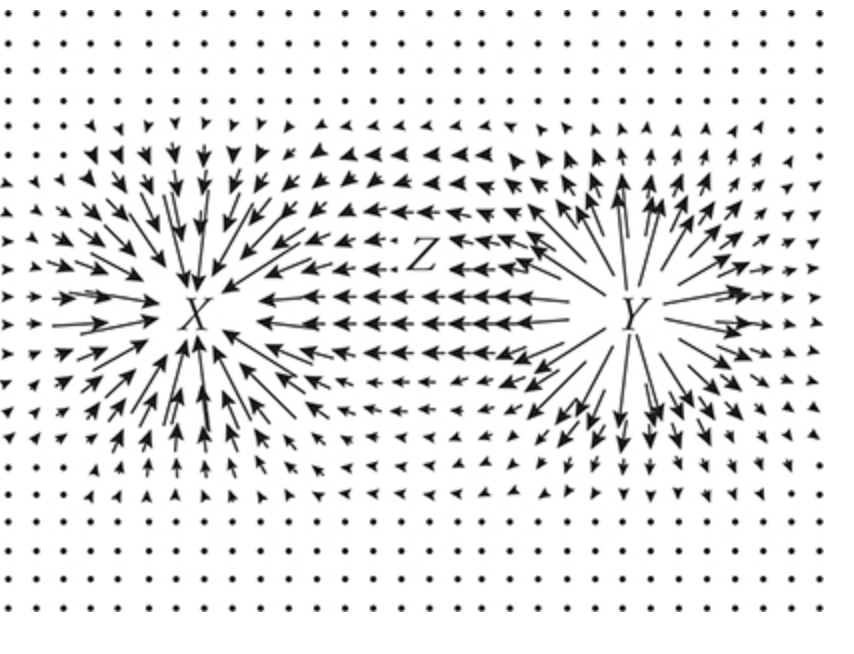
• Electric fields are drawn as arrows because they’re vectors

  • The longer the arrows, the greater the magnitude of the electric field

• Units of electric fields: N/C (Newtons/Coulomb)

• F = qE

  • F: electric force
  • q: charge
  • E: electric field

• The direction of the force on a positive charge is the same direction as the electric field

  • The direction of the force on a negative charge is the opposite direction as the electric field
  • Typically, when using the equation F = qE, we solve for the magnitude and find the direction of the electric force and/or field afterward

Electric Potential

• Electric potential: Electric potential energy per unit charge (provided by an electric field)

  • Units: 1 V = 1 J/C
  • Electric potential is scalar (only have magnitudes)
  • “Zero of electric potential” = “ground” = a theoretical distance at which two charged particles are infinitely far away from each other and therefore don’t affect each other
  • ==ΔU = qΔV==
  • ΔU = difference in electric potential energy
  • q = charge
  • ΔV = difference in electric potential

• Equipotential lines: Lines on which a charged particle would have the same potential

  

  \

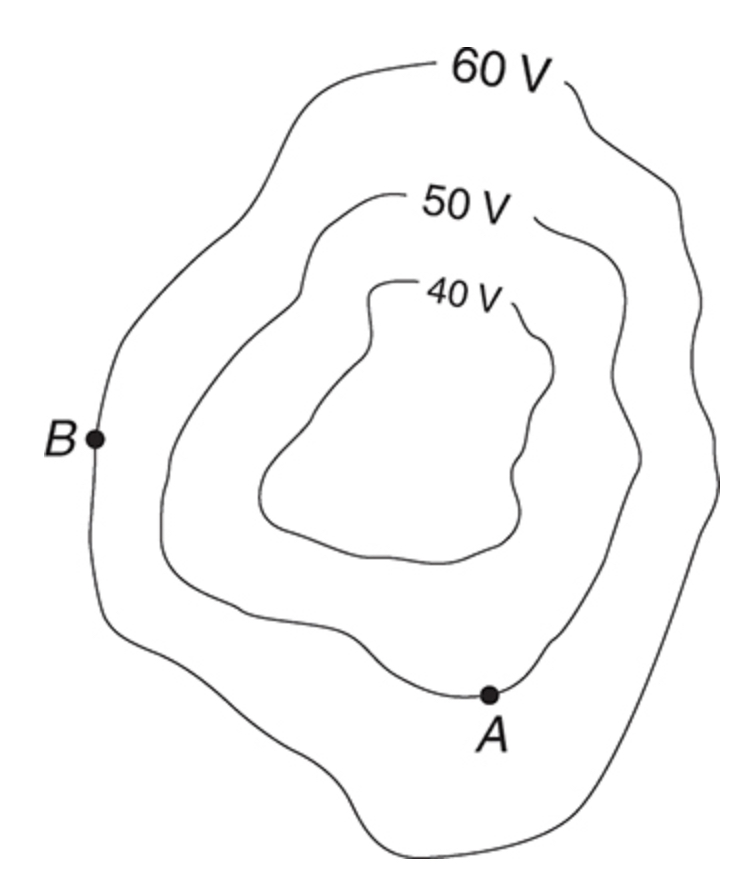
  • Equipotential lines are drawn perpendicular to the electric field lines
  • It takes energy to move a charge to another equipotential line
  • Positive charges are naturally pulled to areas of negative potential
  • Negative charges are naturally pulled to areas of positive potential
  • Remember that energy is conserved so U + K is constant
  • U is electric potential energy and K is kinetic energy

Electrostatics

• Parallel Plates
  • There are 2 metal plates that are parallel - one is positively charged and the other is negatively charged
  • This creates a uniform electric field with the arrows pointing from the positive plate to the negative plate
  • ==E = ΔV/Δr==
  • E = the magnitude of the electric field
  • ΔV = the magnitude of the voltage difference between plates
  • Δr = the distance between plates
  • Parallel plates can be used to make capacitors (a device that stores charges and will be further explored in circuits)
  • ==ΔV = Q/C==
    • ΔV = the voltage across plates
    • Q = charge on each plate
    • C = the capacitance of the capacitor
  • ==C = kεA/d==
    • C = capacitance
    • k = dielectric constant - shows how good of an insulator you have between plates
    • ε = “vacuum permittivity” = 8.85 x 10^-12 C/Vm
• Point charges
  • ==E = q/(4πεr) = kq/r==
  • k = Coulomb’s Law Constant = 9 x 10^9 Nm^2/C^2
  • The electric field produced by a positive charge points away from the charge
  • The electric field produced by negative charge points toward the charge
  • ==V = kq/r==
  • ==F = kqq/r^2==
  • Where the two q’s are the charges of two point charges
  • k = Coulomb’s Law Constant
  • r = the distance between the two point charges
• Electric Field around a point charge or conducting sphere
  • ==E = kq/r^2==
  • To solve for the magnitude of the electric field
  • Inside a conducting sphere, the electric field is 0
    • Net force on any charge inside a conducting sphere is 0

\