Chapter 5 - Magnetism and Electromagnetic Induction
Magnetic Fields
All magnets have South and North poles
- If you took a magnet such as a rectangular-shaped bar magnet, you are not separating the poles - you are creating two magnets that still have North and South poles
Magnetism and static electricity
- Similarities:
- Magnets and charges exert equal and opposite forces on each other
- Magnetic and electric fields extend into infinity and get weaker with increased distance
- Differences:
- Magnetic fields only affect moving charges whereas electric fields can affect both stationary and moving charges
- The force exerted by magnetic fields is perpendicular to both the velocity of the charge and the direction of the magnetic field
- Magnetic field lines are loops instead of lines like in electric fields
Magnetic Field lines
Magnetic field lines are loops that point away from the North and toward the South
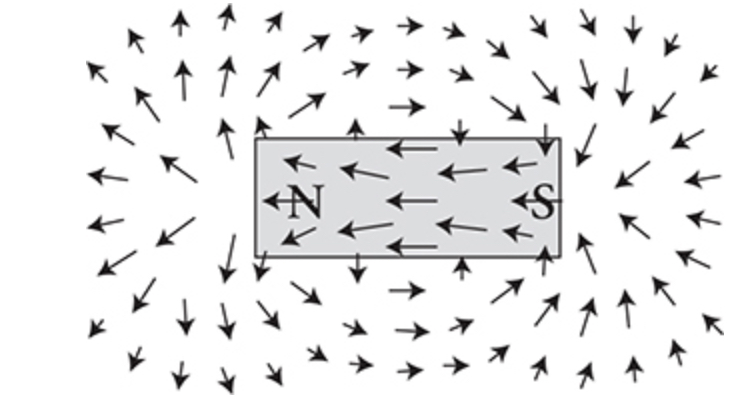
Iron filings gather on these magnetic field lines, creating patterns visible to the human eye
Like electric field lines, longer arrows indicate larger field strength
What creates magnetic fields?
- Moving charges
- For bar magnets, these charges are the electrons circling the nucleus of atoms
- In wires, current serves as moving charges
3-D nature
Magnetic fields are 3D which is often hard to show on paper
In the exam, a dot with a circle (the circle is most often there but it could just be a dot) around it indicates a magnetic field coming out of the page (think of an arrow head coming at you)
An X indicates a magnetic field going into the page (think of the back of an arrow)
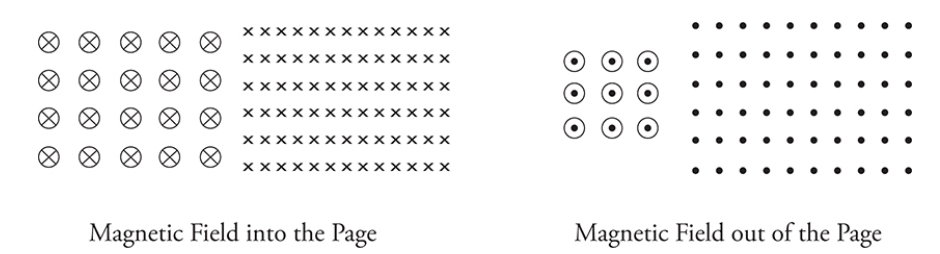
Applications of Magnetic Fields
Dipoles of the Earth
- The magnetic south pole is actually the geographic north pone and vice versa
The magnetic field in a straight wire with current
The magnetic field forms circles in the plane perpendicular to the length of the wire
Picture washers on a wire - those represent circles of the magnetic field
The right-hand rule
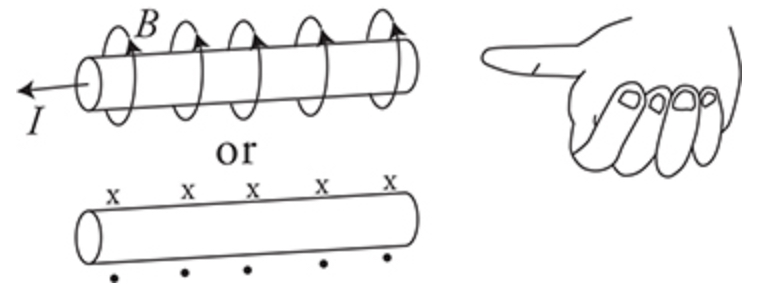
Grasp a pencil with your right hand
Your fingers will curl around the pencil in the same direction the magnetic field curls
- If you imagine a wire with current pointing left, the magnetic field will be represented with X’s on top of the wire and dots below the wire (also known as counterclockwise)
Your thumb will point in the direction of the current
==B = 𝜇I/(2πr)==
B: magnetic field
𝜇: vacuum permeability (4π x 10^-7)
I: Current
r: distance between enter of wire to where you’re trying to find the field strength
Solenoid
- Solenoid: a coil of wire created by wire looped circularly multiple times
- Solenoids hooked up to a battery creates a dipole magnetic field like a bar magnet
Force on a moving charge
If the velocity of a moving particle is perpendicular to the magnetic field, a magnetic force is exerted on the moving charge
==F = qvBsin(θ)==
F: magnetic force
q: charge of particle
v: velocity
B: magnetic field
θ: angle between velocity and magnetic field vectors
Right hand rule - “flat finger” rule
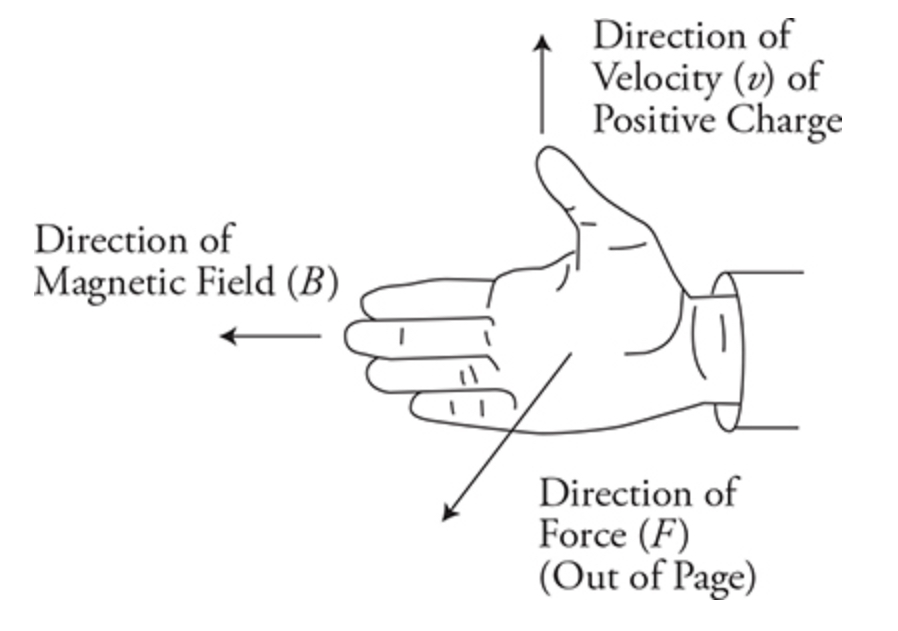
Fingers point in the direction of the magnetic field
Thumb points in the direction of the velocity for the positive charge
Palm points in the direction of the force
The right hand rule works for positive particles but for negative particles, the same rules apply if you use your left hand
When acceleration is perpendicular to the velocity, as is the case because the magnetic force is perpendicular to the velocity, the acceleration is centripetal
Force on a current-carrying wire from an outside magnetic field
- ==F = ILsin(θ)B==
- F: magnetic force
- L: length of the wire
- B: magnetic field
- I: current
- θ: angle between the current and magnetic field
The force between two parallel wires
- To solve problems like this, find the directions of the magnetic field around Wire B and determine the effects on Wire A
- The forces on the wires are equal and opposite in direction
- ==B = 𝜇I/(2πr)==
- B: Magnetic field from wire B
- I: Current from wire B
- r: distance between wires A and B
- ==F = ILsin(θ)B==
- F: magnetic force on wire A from wire B
- I: current through wire A
- L: length of wire (lengths of wire A and B are the same)
- B: magnetic field from wire B
Mass Spectrometer
Remember that magnetic forces give charges a centripetal acceleration
- This means the magnetic force only changes the direction of the charge without altering the magnitude of the velocity
- The path of the charge then becomes circular
Fc = Fb
- Fc: centripetal force
- Fb: magnetic force
==mv^2/r = qvB==
- m: mass of the particle
- v: velocity
- r: radius of the circular path
- q: charge
- B: magnetic field
- Therefore, ==r = mv/(qB)==
If part of the velocity is parallel to the field (theta is not 90 degrees), the charge will take a helical path
Mass Spectrometer: a device used to determine the charge to mass of a particle by arcing them in a magnetic field and finding the radius of its path
Magnetic Flux
- Magnetic Flux: a measure of the magnetic field passing through an area
- Measured in Webers
- Magnetic field strength (magnetic flux density) multiplied by area is equal to magnetic flux
- ==ɸ = Bcos(θ)A==
- ɸ: magnetic flux
- B: magnetic field
- θ: angle between the magnetic field and the “window” of magnetic flux we’re measuring
Electromagnetic Induction
- Electromotive force
- ==ε = Blv==
- ε: electromotive force
- B: magnetic field
- l: length of the wire in the magnetic field
- v: velocity of the wire
- The movement of a wire through a magnetic field can produce an electromotive force
- Other ways to use electromagnetic induction:
- Changing magnetic field strength
- Changing the flux area of a loop
- Turning the loop
- ==ε = -N(Δɸ/Δt)==
- N: number of turns in the wire around the loop
- For rectangular loops:
- ==ε = BLv==
- ε: electromotive force
- L: length of the rectangle side that is entering the magnetic field
- B: magnetic field
- v: velocity
- Lenz’s Law: The direction of the induced current opposes any change in flux
- If we move a loop with zero magnetic field near a magnetic field coming out of the page, the induced current will create a magnetic field into the page within the loop to oppose the increased magnetic field out of the page
- When the loop stops moving and is completely in the region with the magnetic field, there is no induced emf with no changing flux
- Uses for electromagnetic induction:
- Generation of electricity
- In microphones and speakers
- To run motors
- In MRIs
- On credit cards
- Point is: electromagnetic induction is very important in everyday use
Magnetic Behavior
- Ferromagnetism
- Ex: iron, nickel. and cobalt
- Localized regions called domains are inside this material
- In an external magnetic field, the domains align, amplifying it
- Domains can grow enough to create a permanent magnet
- Magnets strongly attract ferromagnetic materials
- Paramagnetism
- Unlike ferromagnetic materials, paramagnetic materials don’t form permanent magnets
- Magnets weakly attract paramagnetic materials
- The domains still align with the external magnetic field
- Diamagnetism
- Internal properties align opposite to the external field - cancel out that part of the magnetic field
- Ex: water, graphite
- Magnets weakly repel diamagnetic materials