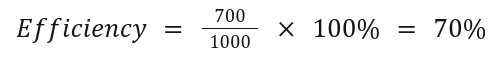Types of Energy in Physics to Know for AP Physics 1 (2025)
1. Kinetic Energy (KE)
Definition:
Energy an object possesses due to its motion.
Formula:
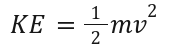
m: Mass (in kilograms, kg)
v: Velocity (in meters per second, m/s)
Key Concept:
Kinetic energy depends on the square of velocity, meaning small increases in speed lead to significant increases in energy.
Doubling an object's velocity increases its kinetic energy by four times
Importance:
Critical in analyzing motion, collisions, and momentum in physics.
Applications:
Vehicle safety (e.g., analyzing collisions and braking distances).
Sports (e.g., calculating energy in projectiles like a baseball or soccer ball).
Engineering (e.g., turbines and engines).
Example Problem:
A 5 kg object moves at 10 m/s. Find its kinetic energy.
Solution:
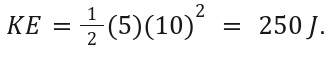
2. Gravitational Potential Energy (PE)
Definition:
Energy stored in an object due to its height in a gravitational field.
Formula:
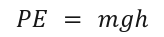
m: Mass (kg)
g: Gravitational acceleration (9.8 m/s2)
h: Height (m)
Key Concept:
Directly proportional to mass and height.
Importance:
Plays a key role in energy conservation and systems like roller coasters, pendulums, and hydroelectric power.
Applications:
Hydropower (e.g., water stored in dams).
Roller coasters (e.g., analyzing height and speed transitions).
Space science (e.g., calculating escape velocity for rockets).
Example Problem:
A 2 kg object is 5 m above the ground. Find its gravitational potential energy.
Solution:
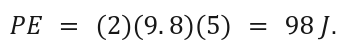
3. Elastic Potential Energy (PE)
Definition:
Energy stored in elastic materials when stretched or compressed.
Formula:
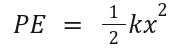
k: Spring constant (N/m)
x: Displacement from equilibrium position (m)
Key Concept:
Increases quadratically with displacement.
Importance:
Essential in analyzing springs, elastic bands, and other systems where energy is stored and released.
Applications:
Suspension systems in vehicles.
Archery (e.g., energy stored in a stretched bowstring).
Trampolines (e.g., energy during compression and release).
Example Problem:
A spring with k = 200 N/m is compressed by 0.1 m. Find its potential energy.
Solution:
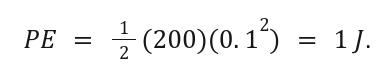
4. Thermal Energy
Definition:
The total kinetic energy of particles in a substance, linked to its temperature.
Key Concepts:
Higher temperatures result in greater particle motion.
Plays a key role in phase changes (e.g., melting, boiling).
Importance:
Vital in understanding heat transfer methods (conduction, convection, radiation) and thermodynamics.
Applications:
Heat engines (e.g., steam engines, car engines).
Climate science (e.g., understanding global temperature changes).
Daily life (e.g., cooking, insulation systems).
Applications:
Engine efficiency, refrigeration, and climate systems.
5. Mechanical Energy
Definition:
Total energy in a system from both kinetic (KE) and potential (PE) energy.
Formula:
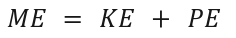
Key Concept:
Remains constant in a closed system (ignoring friction or external forces).
Importance:
Used in energy conservation laws to analyze physical systems like pendulums and roller coasters.
Applications:
Pendulum motion (e.g., energy transformation between KE and PE).
Amusement park rides (e.g., analyzing energy in roller coasters).
Example Problem:
A 3 kg object at a height of 4 m has a speed of 2 m/s. Find its total mechanical energy.
Solution:
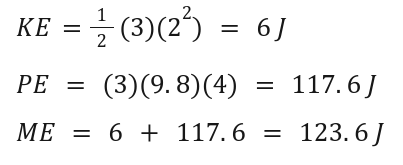
6. Work-Energy Theorem
Definition:
Work done on an object equals the change in its kinetic energy.
Formula:
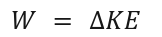
Key Concept:
Links force, displacement, and energy changes.
Importance:
Simplifies motion problems by focusing on energy changes rather than forces.
Applications:
Launching a rocket (work increases kinetic energy).
Braking a car (negative work decreases kinetic energy).
Example Problem:
A force does 50 J of work on a stationary object. What is its kinetic energy after the work is applied?
Solution:
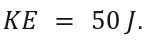
7. Conservation of Energy
Definition:
Energy cannot be created or destroyed, only transformed between forms.
Key Concepts:
Total energy in a closed system remains constant.
Examples include conversions between kinetic, potential, and thermal energy.
Importance:
Fundamental principle in physics and engineering.
Applications:
Power generation (e.g., potential energy of water in dams to electrical energy).
Space exploration (e.g., converting chemical energy of fuel to kinetic energy of rockets).
Example Problem:
A pendulum at its highest point has PE = 100 J. At its lowest point, what is its kinetic energy (ignoring friction)?
Solution:
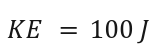
8. Energy Transformations
Definition:
Conversion of energy from one form to another (e.g., potential to kinetic).
Key Concepts:
Observed in systems like pendulums, roller coasters, and power plants.
Energy loss as heat or sound often accompanies transformations.
Importance:
Critical for analyzing real-world systems and energy efficiency.
Applications:
Renewable energy systems (e.g., solar panels converting light to electrical energy).
Daily life (e.g., batteries powering devices).
9. Power (P)
Definition:
The rate at which work is done or energy is transferred.
Formula:
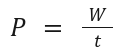
W: Work (J)
t: Time (s)
Key Concept:
Measured in watts (W, where 1 W = 1 J/s).
Applications:
Electrical systems (e.g., power ratings of appliances).
Sports (e.g., analyzing athletes’ performance).
Example Problem:
A motor does 500 J of work in 10 s. Find its power output.
Solution:
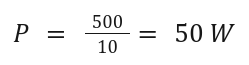
10. Efficiency
Definition:
A measure of how much useful energy or work output is achieved compared to energy input.
Formula:
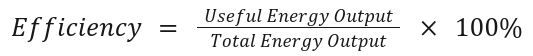
Key Concept:
No machine is 100% efficient due to energy losses as heat or friction.
Applications:
Evaluating machine performance (e.g., engines, electric generators).
Energy systems (e.g., renewable energy plants).
Example Problem:
A machine uses 1000 J of energy and produces 700 J of useful work. Find its efficiency.
Solution: