Triangles
Vocab:
- 30-60-90 Triangle - A triangle with a 30, 60, and 90 degree angle.
- 45-45-90 Triangle - A triangle with a two 45 degree angles and one 90 degree angle.
- Acute Triangle - A triangle with three acute angles.
- Base Angle - An angle of an isosceles triangle opposite one of the equal sides, i.e. opposite one of the legs.
- Base of an Isosceles Triangle - The side of an isosceles triangle unequal to the other two sides, the legs.
- Equiangular Triangle - A triangle whose angles are all equal.
- Equilateral Triangle - A triangle whose sides are all equal (of equal length).
- Hypotenuse - The side of a right triangle opposite the right angle. The Pythagorean Theorem states that the square of the hypotenuse is equal to the sum of the square of the other two sides.
- Isosceles Triangle - A triangle with at least two equal sides.
- Legs of a Right Triangle - The two sides of a right triangle opposite the two oblique angles
- Legs of an Isosceles Triangle - The two sides of an isosceles triangle that are equal.
- Obtuse Triangle - A triangle with one obtuse angle.
- Pythagorean Theorem - The theorem that states that the length of the hypotenuse squared is equal to the sum of the squares of the lengths of the legs of a right triangle; c2 = a2 + b2.
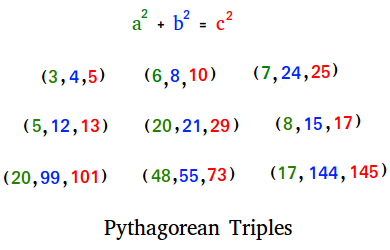
- Pythagorean Triple - A set of three integers a, b, and c such that c2 = a2 + b2
- Right Triangle - A triangle with one right angle.
- Scalene Triangle - A triangle with no equal sides.
- Vertex Angle - The angle in an isosceles triangle opposite the base.
| Length Of Hypotenuse | c = |
|---|
| Length Of A Leg | a = |
|---|
Every triangle has six main parts, its three sides and three angles. A triangle can be classified by either of these criteria--sides or angles. In addition to falling into a certain category based on sides or angles, triangles can also qualify as special triangles by meeting more specific requirements. Special triangles such as equilateral, isosceles, and right triangles, have very precise properties and characteristics that help us draw conclusions about unknown figures.
Isosceles:
An isosceles triangle is a triangle with at least two equal sides, meaning that the lengths of those sides are equal. A new set of terms accompanies the isosceles triangle. The equal sides are called legs. The third side is the base. The angle opposite the base is the vertex angle. The angles opposite the legs are called the base angles.
Sides a and b are the legs. Side c is the base. Angle C is the vertex angle. Angles A and B are the base angles.
The beauty of an isosceles triangle is that, just like the sides, the base angles are equal. From this we learn that if two angles of a triangle are equal, the sides opposite them are also equal, and the triangle is an isosceles triangle. We also know the converse: if two sides of a triangle are equal, their opposite angles are equal, and the triangle is isosceles.
Equilateral:
An equilateral triangle is a triangle whose sides are all equal. It is a specific kind of isosceles triangle whose base is equal to each leg, and whose vertex angle is equal to its base angles.
The angles of an equilateral triangle are all equal. This is true because of the special property of isosceles triangles. Every equilateral triangle is also an isosceles triangle, so any two sides that are equal have equal opposite angles. Therefore, since all three sides of an equilateral triangle are equal, all three angles are equal, too. So, every equilateral triangle is also equiangular.
Right:
A triangle with one right angle is called a right triangle. The side opposite the right angle is called the hypotenuse of the triangle. The other two sides are called legs. The other two angles have no special name, but they are always complementary. The total angle sum of a triangle is 180 degrees, and the right angle is 90 degrees, so the other two must sum to 90 degrees.
The triangle above has side c as its hypotenuse, sides a and b as its legs, and angle C as its right angle. Angles A and B are complementary.
There are two types of right triangles that every mathematician should know very well. One is the right triangle formed when an altitude is drawn from a vertex of an equilateral triangle, forming two congruent right triangles. The angles of the triangle will be 30, 60, and 90 degrees, giving the triangle its name: 30-60-90 triangle. The ratio of side lengths in such triangles is always the same:
The other common right triangle results from the pair of triangles created when a diagonal divides a square into two triangles. This ratio holds true for all 45-45-90 triangles. 45-45-90 triangles are also often called isosceles right triangles.
One last characteristic to note is that the legs of a right triangle are also altitudes of the triangle. Therefore, the area of a right triangle is one-half the product of the lengths of its legs.
Pythagorean theorem:
One of the most interesting and well-known formulas in math is the Pythagorean Theorem, which only holds true for right triangles. The formula says that the length of the hypotenuse squared is equal to the sum of the squares of the lengths of the legs:
| c2 = a2 + b2 |
|---|
If a triangle is right, then this formula holds true. Conversely, if this formula holds true, then you know that the triangle for which it works is a right triangle. With this formula, given any two sides of a right triangle, you can calculate the length of the third side. One way to picture it can be seen below.
In the figure above, the area of the square with side length c is equal to the sum of the areas of the squares with side lengths a and b. This is a physical interpretation of the Pythagorean Theorem.
The three sides of a right triangle can be of any length, provided that they obey the laws of the Pythagorean Theorem. However, only certain groups of three integers can be the lengths of a right triangle. Such groups of integers are called Pythagorean triples. Any multiple of one of these groups of numbers also can be a Pythagorean triple. You can check any of them for yourself. All of these groups of three integers, these Pythagorean triples, satisfy the Pythagorean Theorem.