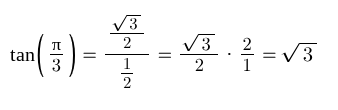AP Precalculus Guide
Unit 1
1.1 - Change in Tandem
A function is a mathematical relation that maps a set of input values to a set of output values such that each input value is mapped to exactly 1 output value
Positive Function - the output values are above 0
Negative Function - the output values are below 0
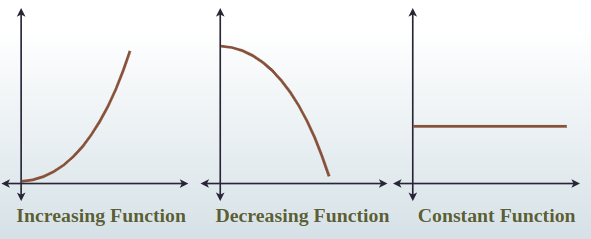
Increasing function if…
Verbally: as the input values increase, the output values always increase
Analytically: for all a and b in the interval ___, if a < b, then f(a) < f(b)
Decreasing function if…
Verbally: as the input values increase, the output value always decreases
Analytically: for all a and b in the interval ___, if a < b then f(a) > f(b)
Zero: when the output value is zero
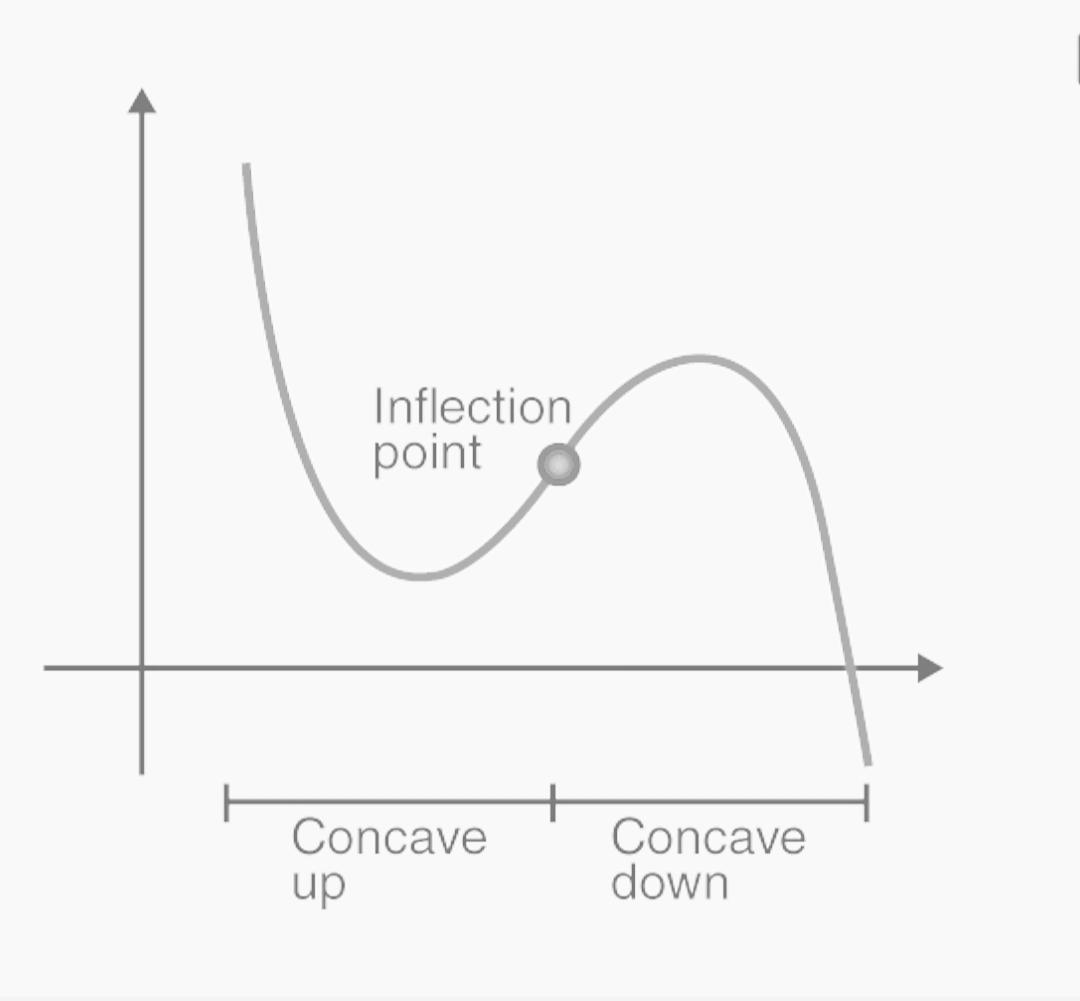
Concave up: bowl facing UP → slope increasing
Concave down: bowl facing DOWN → slope decreasing
Point of Inflection: point where the concavity changes
Justification on stating whether or not the function is increasing or decreasing…
x | 4 | 6 | 7 | 10 |
f(x) | 1 | 1.01 | 1.04 | 1.06 |
The function f is increasing on the interval 4 < x < 10 or (4, 10) because for all a and b values, if a < b, then f(a) < f(b)
1.2 - Rates of Change
rate of change = slope
Rate of Change of a point
Ex. Estimate the rate of change at x = 1 for the function f(x) = -½x² + 3x - ½
Get as close as you can to the point (at least 3 decimal places)
(1, 2) & (1.001, 2.0019995)
Now calculate the slope
(2.0019995 - 2) ÷ (1.001 - 1) = 1.9995 ≈ 2
Positive ROC - indicates that as one quantity increases or decreases the other quantity does the same (same as if it were to say a function is increasing)
Negative ROC - indicates that as one quantity increases, the other decreases (same as if it were to say a function is decreasing)
1.3 - Rates of Change in Linear and Quadratic Functions
Average Rate of Change = Slope of a SECANT Line (calculates overall change in a quantity across a given interval)
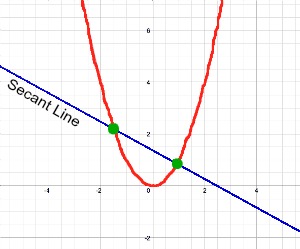
average rate of change = f(a) - f(b) / a - b
The average rate of change for a linear function is constant. Regardless of the input-value interval length, the average rate of change always stays the same
Does not matter where you pick your points, the slope is always the same
x | 15 | 16 | 17 | 18 | 19 |
f(x) | 18 | 20 | 22 | 24 | 26 |
NOTE: linear functions only have an increase/decrease in the y values, meanwhile quadratics have an increase/decrease in the average rate of change (the amount of differences is the degree)
The amount of differences you get from this indicates the degree. 1 is linear, 2 is quadratic, 3 is cubic, 4 is quartic, 5 is quintic, etc.
The average rate of change for a quadratic doesn’t stay the same. For consecutive equal-length input-value intervals, the average rate of change of the rate of change of a quadratic function is constant.
here the x is going up by one (15 +1 = 16; 16 +1 = 17)
x | 15 | 16 | 17 | 18 | 19 |
f(x) | 18 | 20 | 20 | 18 | 14 |
The rate of change of the rate of change: what is the change in the slope/ROC (This will always be 0 for linear functions)
here the bottom is varying (18 + 2 = 20; 20 + 0 = 20; 20 - 2 = 18; 18 - 4 = 14)
Then you would do that again to find a commonality (+2, 0, -2, -4) → ( 0 - 2 = -2; -2 - 0 = -2; -4 - (-2) = -2)
Which means the rate of change of the rate of change is -2
The function is concave down because the rate of change is decreasing over equal length input intervals
Example problem on quiz:
The function p is given by p(x) = g(x + 1) - g(x). If p(x) = 2, which of the following statements must be true?
g(x + 1) - g(x) is really the slope (y2 [which is f(x + 1)] - y1 [which is f(x)]) / 1
p(x) = 2 means that the slope is 2
This means the graph always has a positive slope and that because p is positive and constant, g is increasing
Confusing Terms
Positive Rate of Change | Negative Rate of Change |
The independent variable increases, the dependent variable also increases | As the independent variable increases the dependent variable decreases |
Increasing Rate of Change | Decreasing Rate of Change |
The rate of change (slope) itself is increasing Ex. (car speed goes from 20 to 30 in one hour and 30 to 70 in the next hour) | The rate of change (slope) itself is decreasing Ex. (car speed increases from 30 to 40 in 1 hour and then to 45 in the next hour, the acceleration is decreasing) |
Positive Average Rate of Change | Negative Average Rate of Change |
Whether the rate of change between an interval or 2 points (secant line) is positive | Whether the rate of change between an interval or 2 points (secant line) is negative |
Increasing Average Rate of Change | Decreasing Average Rate of Change |
The rate of change over an interval itself is increasing Ex. (In a 3 hour period, a car goes 10 mph at 1 hour, 15 mph a 2 hours and 20 mph at 3 hours, the aroc of the speed is increasing) | The rate of change over an interval itself is decreasing Ex. (In a 3 hour period, a car goes 20 mph at 1 hour, 15 mph a 2 hours and 10 mph at 3 hours, the aroc of the speed is decreasing) |
Increasing + Positive ROC | Increasing + Negative ROC |
→ The function is increasing → The graph is concave up | → The function is decreasing → The graph is concave up |
Decreasing + Positive ROC | Decreasing + Negative ROC |
→ The function is increasing → The graph is concave down | → The function is decreasing → The graph is concave down |
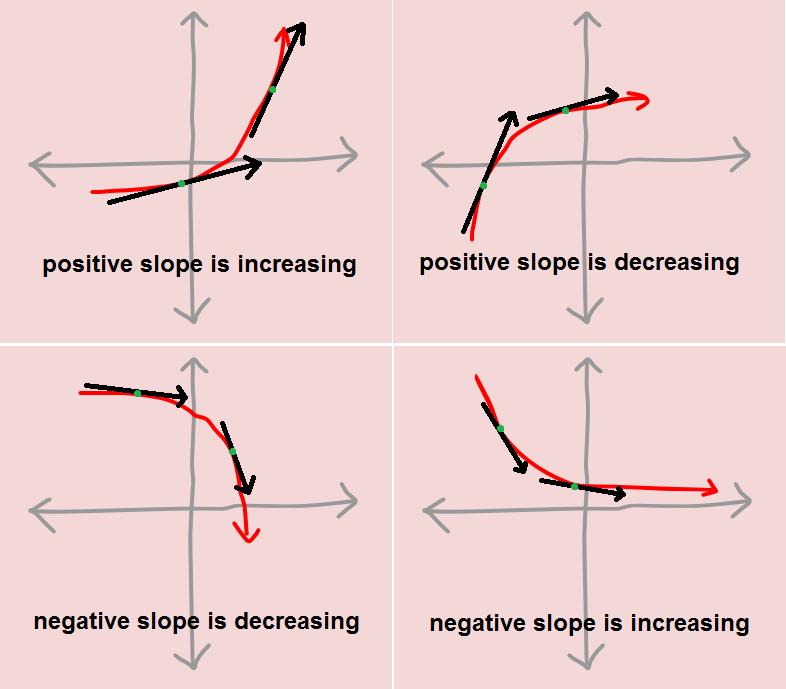
Increasing / Decreasing ROC → The graph is concave Up / Down
Positive / Negative ROC → The function is Increasing / Decreasing (± slope; imagine a positive or negative linear line)

1.4 - Polynomial Functions and Rates of Change
Polynomial
p(x) = anxn + an-1xn-1 + an-2xn-2 + … + a1x2 + a1x + a0
anxn is the leading term | n is the degree | an is the leading coefficient
Local / Relative Maximum - if the polynomial switches from increasing to decreasing
Local / Relative Minimum - if the polynomial switches from decreasing to increasing
an included endpoint of a polynomial with a restricted domain may also be the local minimum or maximum
Global Maximum - the greatest of the local maximums (If it goes to infinity, there is none)
Global Minimum - the least of the local minimums
Two Zeros = if you have 2 zeros of a polynomial, there must be at least 1 local extrema between the two
Even degree = absolute extreme
Positive leading coefficient = absolute minimum
Negative leading coefficient = absolute maximum
1.5A - Polynomial Functions and Complex Zeros & 1.5B - Even and Odd Polynomials
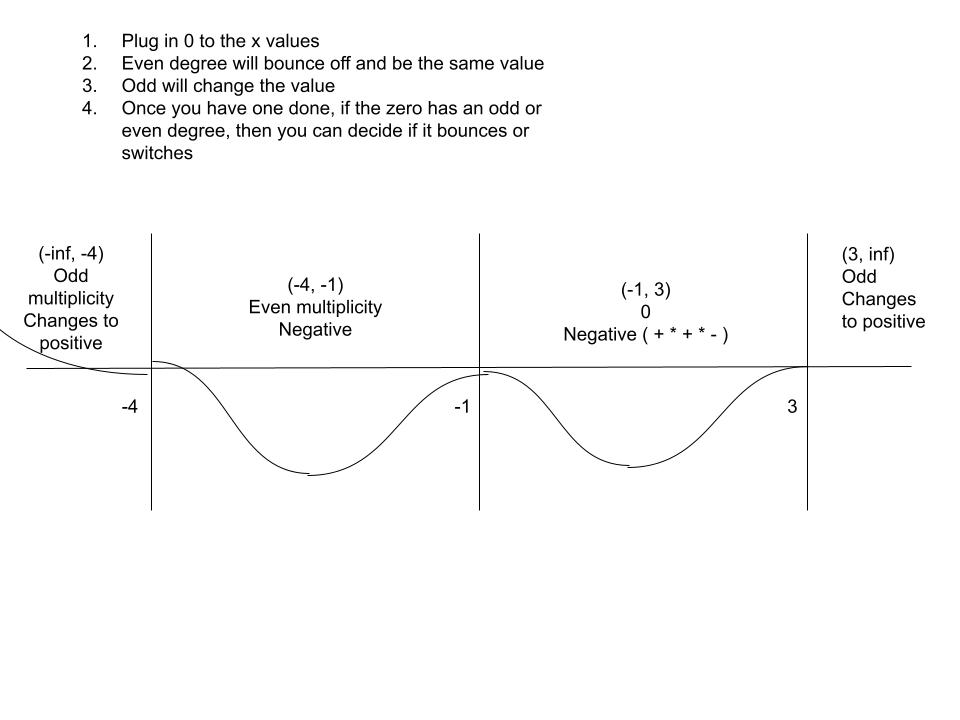
If the real zero, a, of (x - a), has an even multiplicity, then the graph will bounce off the x-axis
Example:
Given the function: (x + 1)2(x - 3)3(x + 4)1
Complex Zeros - non-real zeros that will always come in pairs (Ex. 3 ± 2i)
a ± bi
Even functions = symmetrical over the y-axis
has the property f(-x) = f(x)
You can prove that the function is even by substituting in -x and see if you get the same original function
Ex. f(x) = x6 - 4x2 → f(-x) = (-x)6 - 4(-x)2 → f(-x) = x6 - 4x2
Odd functions = symmetrical over the origin
has the property f(-x) = -f(x)
1.6 - Polynomial Functions and End Behavior
The left or right side of a polynomial will either go up or down.
The limit expresses the end behavior of a function.
The Left Side
The x values are approaching negative ∞
lim x → -∞ f(x)
The Right Side
The x values are approaching positive ∞
lim x → ∞ f(x)
Left Side | Right Side | |
Up | limx → -∞ f(x) = ∞ | limx → ∞ f(x) = ∞ |
Down | limx → -∞ f(x) = -∞ | limx → ∞ f(x) = -∞ |
Left side x → -∞ | Right Side x → ∞ | |
Even degree and Positive leading coefficient | limx → -∞ f(x) = ∞ | limx → ∞ f(x) = ∞ |
Even degree and Negative leading coefficient | limx → -∞ f(x) = -∞ | limx → ∞ f(x) = -∞ |
Even degree means that the left and right side will behave the same
Odd degree means that the left and right side will behave opposite
1.7 - Rational Functions & End Behavior
Rational Function - the ratio of two polynomials where the polynomial in the denominator cannot equal 0
Usually can be described as:
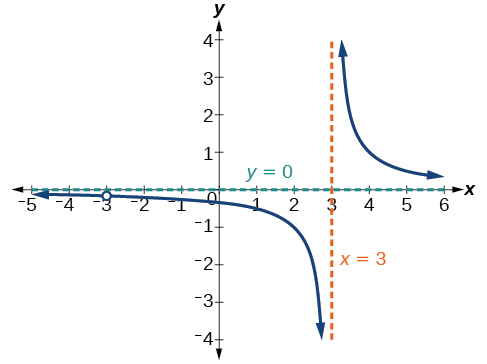
Labeling some of the properties of the function:
Domain: (-∞, -3) U (-3, 3) U (3, ∞) There is a hole and vertical asymptote
limx → -∞ f(x) = 0 | limx → ∞ f(x) = 0
0 is the horizontal asymptote because both limits approach this number
For inputs with a larger magnitude, the polynomial in the denominator dominates the polynomial in the numerator
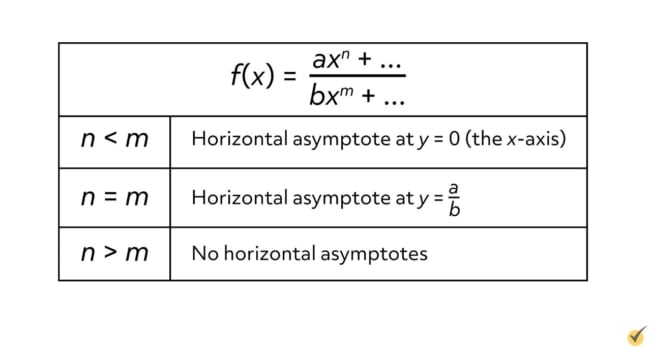
Ways to remember:
BOBO BOTNA EATS DC
BOBO - Bigger on bottom = 0 (BOB0)
BOTNA - Bigger on top = No Asymptote (BotNA)
EATS DC - Exponents Are The Same = Divide Coefficients (EATSDC)
1.8 - Rational Functions & Zeros
When there is an unfactored polynomial in a rational function, try to factor both numerator and denominator to make it easier to see holes and asymptotes
This is a helpful equation when working with a polynomial that is both factored on the numerator and denominator: (x-int)(hole) / (hole)(vertical asymptote)
Example function: (x + 3)(x - 2) / (x + 4)(x - 2)
X intercepts (zeros) will be any factors on the numerator that aren’t holes
(x + 3); so that means that there is an x-intercept at x = -3
Holes will appear on both the numerator and denominator (This is the value you cannot plug into the equation)
(x - 2) / (x - 2); meaning that x = 2 does not exist
Vertical asymptotes are any factors that aren’t holes left in the denominator
(x + 4); so that means that there is a vertical asymptote at x = -4
1.9 - Rational Functions & Vertical Asymptotes

Finding the limit as x approaches a number/asymptote
2- means approaching the vertical asymptote on the left side from left to right
limx → 2- f(x) = -∞
2+ means approaching the vertical asymptote on the right side from right to left
limx → 2+ f(x) = ∞
Order of dominance
Hole > 0
If there is a zero with a hole, then there will be no zero, but a hole instead
Ex. (x + 1)(x + 1) / (x + 1)(x + 2) means that there will be a hole at x = -1 instead of a zero because there is a hole of (x + 1)
Vertical Asymptote > Hole
If there is a vertical asymptote the same as a hole, then that hole will be a vertical asymptote
Ex. (x + 1) / (x + 1)(x + 1) means that there is a vertical asymptote at x = -1 instead of a hole because there is a vertical asymptote of (x + 1)
1.10 - Rational Functions & Holes
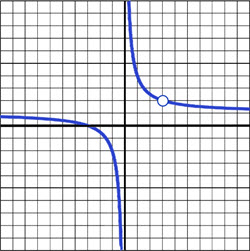
Writing limits for holes:
From the left:
As x approaches 3 from the left, f(x) approaches 2
limx → 3- f(x) = 2
From the right:
As x approaches 3 from the right, f(x) approaches 2
limx → 3+ f(x) = 2
Using a Table to Determine Limits
x | 2.9 | 2.99 | 3 | 3.01 | 3.1 |
f(x) | 2.0345 | 2.0033 | undefined | 1.9967 | 1.9677 |
As you can see, the closer we get to 3, the closer the values get to 2

1.11A - Equivalent Representations & Binomial Theorem & 1.11B - Polynomial Long Division & Slant Asymptotes
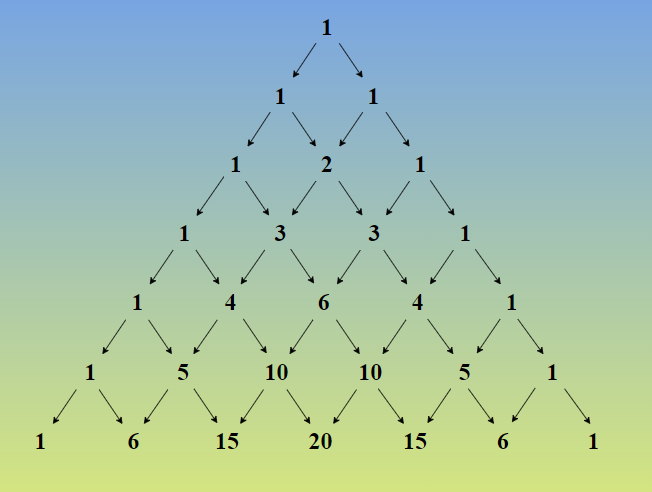
Using pascal’s triangle, you can expand binomials. The rows of pascal’s triangle start from 0 (the top) and so on.
For example, if you had (x + 3)4 then the row you would use would be → 1 4 6 4 1
Binomial Theorem:
The formula is (x + y)n where n would be the row #
(a+b)n = C1anb0 + C2an - 1b1 + C3an - 2b2 + … + Cn - 1a1bn - 1 + Cna0bn
C is the coefficient corresponding to the row in pascal’s triangle
n is the power
Using the numbers in pascal’s triangle, expand the polynomial
As the power of x decreases, the power of y increases
Ex. (x + 5)3
1x350 + 3x251 + 3x152 + 1x053 → x³ + 15x² + 75x + 125
Slant Asymptote - When the degree of the numerator is one higher than the degree of the denominator
End behavior of slant asymptote:
Finding the asymptote of the slant asymptote with long division or synthetic division will give you the linear equation. The end behavior of that function (in this case, y = x + 1) is the end behavior of the rational function
limx → ∞ f(x) = ∞
limx → -∞ f(x) = -∞
Long Division
Long division can be used to divide any polynomial by another one
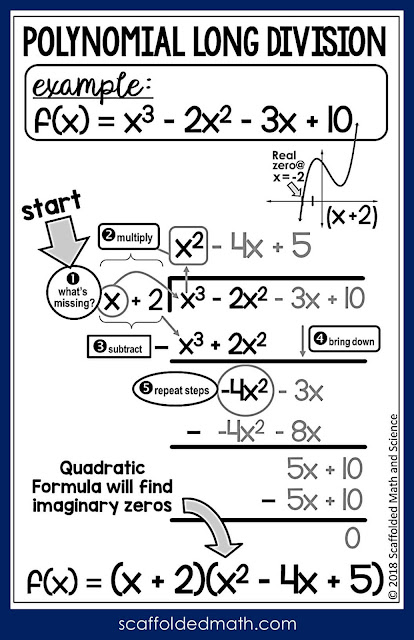
Synthetic Division:
Synthetic division can only be used when dividing a polynomial by a factor like (ax + b)

1.12 - Transformations of Functions
Vertical Translations (anything done outside of the function)
f(x) + d → up by d units
f(x) - d → down by d units
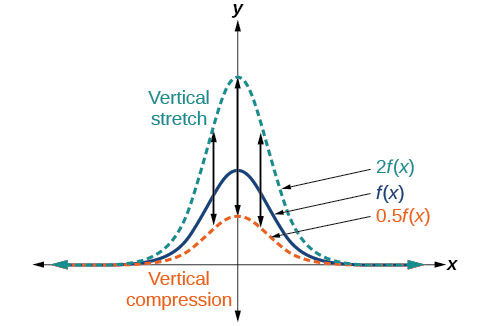
Vertical Dilations (anything done outside of the function)
a × f(x)
When doing a vertical dilation, only the y-values change, the x-values do not
Multiply the y-values by a
a > 1: vertical stretch
a < 1: vertical shrink
Horizontal Translations (anything done inside the function)
f(x - d) → right by d units
f(x + d) → left by d units
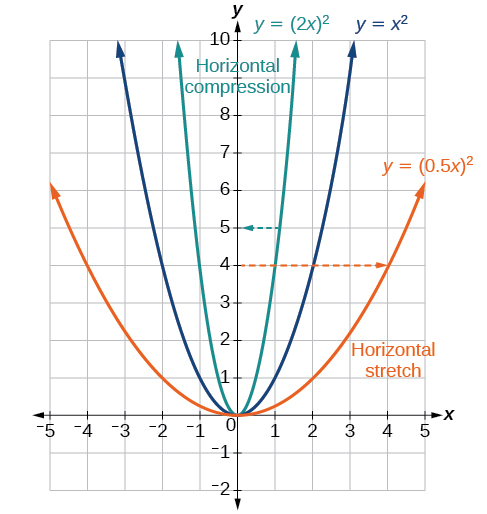
Horizontal Dilations (anything done inside the function)
f(bx)
b is inverted (if it was f(3x) that would be a horizontal dilation of 1/3)
When doing a horizontal dilation, only the x-values change, the y-values do not
Multiply the x-values by the inverse of b
1/b > 1: horizontal shrink
1/b < 1: horizontal stretch
Reflections
-f(x) → reflect over x-axis → vertical change
f(-x) → reflect over y-axis → horizontal change
Transformations Numerically
x | 0 | 1 | 2 | 3 | 4 |
f(x) | -20 | -12 | 0 | 8 | 14 |
Ex. let g(x) = 3f(x - 2) + 1, find g(3)
g(x) = 3f(x - 2) + 1 → set up equation
g(3) = 3f(3 - 2) + 1 → plug in the 3 for x in the modified equation
g(3) = 3f(1) + 1 → simplify
g(3) = 3(-12) + 1 → find what f(1) is and substitute that in
g(3) = -35 → solve
Transformations through domain & range
Ex. Given the graph for f has a domain of [-4, 3] and a range of (3, 9]. Let g(x) = -f(x + 5) + 2.
f(x)
Domain: [-4, 3]
Range: (3, 9]
g(x)
Domain: [-4 - 5, 3 - 5] → [-9, -2]
f(x + 5) → move left 5 → subtract 5 from the domain
Range: (3 * -1, 9 * -1] → (-3 + 2, -9 + 2] → (-1, -7]
Do the inversions and dilations first then the translations
Transformations Algebraically
Ex. Given f(x) = x² - 3x + 2, let g(x) = f(x - 3) + 2, find g(x)
g(x) = f(x - 3) + 2 → set up equation
[(x - 3)² - 3(x - 3) + 2] + 2 → substitute the input values in g(x) for x in f(x)
(x² - 6x + 9 - 3x + 9 + 2) + 2 → distribute
(x² - 9x + 20) + 2 → combining like terms
g(x) = x² - 9x + 22 → solved
1.13 - Function Model Selection
Perimeter will be linear
Area will be quadratic
Volume will be cubic
Restricted Domain & Range
Restricted domain and range is give between brackets and is usually given in a word problem or between a piece of a function
Piecewise Functions
Range of a piecewise function
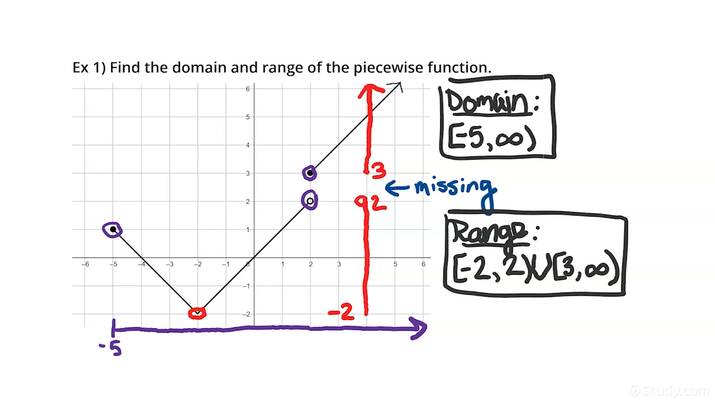
1.14 - Function Model Construction
Types of Regression
Linear
Quadratic
Cubic
Quartic
Exponential
Logarithmic
Logistic
Sine
Inversely Proportional
When something is inversely proportional, the equation y = k / x is used
k is some constant
Ex. The number of workers at a job is inversely proportional to the time it takes to complete a task. If there are 15 workers and it takes 20 minutes to do a task, then how many workers would it take to complete a task in 3 minutes?
n = k / t → number of workers = k / time
15 = k / 20 → set up equation to solve for k
k = 15 × 20 = 300 → solve for k
n = 300 / 3 → input k into new equation to solve for workers
n = 100 workers → it would take 100 workers to complete a task in 3 minutes
Piecewise Functions Algebraically

Piecewise functions can be shown like the image above. The function on the left is in the bounds of the inequality on the right.
Unit 2
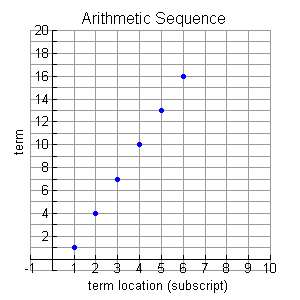
2.1 - Change in Arithmetic and Geometric Sequences
Sequence → an ordered list of numbers; each listed number is a term
Ex. {1, 3, 5, 7, 9, …} (… means it keeps going continuously)
1 would be the first term not the zero term
Arithmetic Sequences
Linear
If each successive term in a sequence has a common difference (constant ROC), the sequence is arithmetic
Equation for the nth term → an = a0 + dn
a0 is the initial value (zero term) and d is the common difference

Equation for any term → an = ak + d(n - k)
ak is the kth term of the sequence
Geometric Sequence
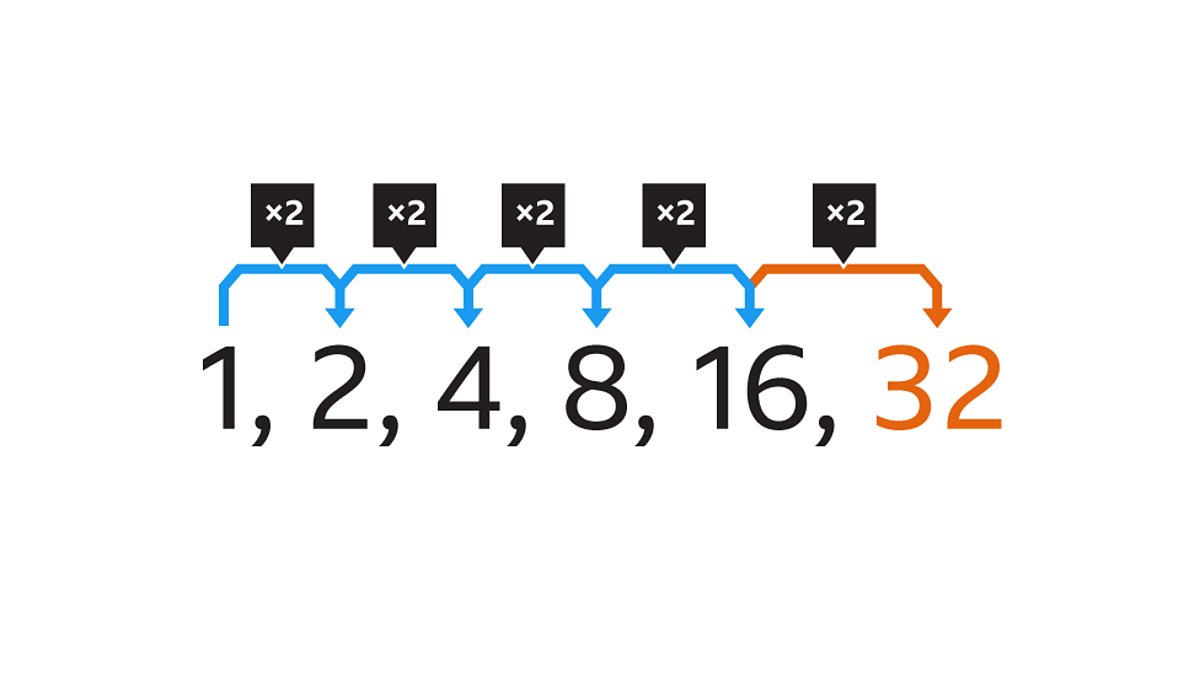
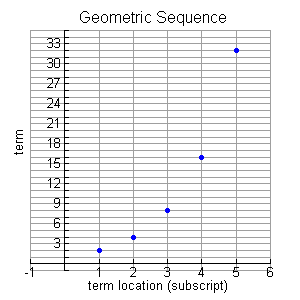
Exponential
If each successive term in a sequence has a common ratio (constant proportional change) the sequence is geometric
If dividing is involved (for example, {4, 2, 1, …} is ÷2 each time), then the ratio will be multiplied by a fraction (÷2 → ×1/2)
Equation for the nth term → gn = g0rn
g0 is the initial value (zero term) and r is the common ratio
Equation for any term → gn = gkr(n - k)
gk is the kth term of the sequence
2.2 - Change in Linear and Exponential Functions
Linear Functions vs Arithmetic Sequences
They are basically the same, except when using a sequence vs a function, the domain and points are discrete, meaning the domain only includes specific points and not all numbers in between
The point (x1, y1) of a linear function is similar to (k, ak) of an arithmetic sequence
Slope-intercept form f(x) = b + mx | Arithmetic Sequence an = a0 + dn |
Point-slope form f(x) = y1 + m(x - x1) | A.S. for the kth term an = ak + d(n - k) |
Exponential Functions vs Geometric Sequences
Again, they are basically the same except with discrete points for the sequence
The point (x1, y1) of an exponential function is similar to (k, gk) of a geometric sequence
Exponential Function f(x) = abx | Geometric Sequence gn = g0rn |
Shifted Exponential Function f(x) = y1r(x - x1) | G.S. for the kth term gn = gkr(n - k) |
How each output value changes analytically
Arithmetic → Over equal-length input value intervals, if the output values of a function change at a constant rate, then the function is linear (adding the slope)
Geometric → Over equal-length input value intervals, if the output values of a function change proportionally, then the function is exponential (multiplying the ratio)
“Over equal-length input intervals” means that the x-values of the function or a table are increasing by a constant amount (ex. 1, 2, 3, 4 or 2, 4, 6, 8)
Finding whether a set of points is linear or exponential
x | 5 | 6 | 7 |
f(x) | 8 | 16 | 32 |
Make sure the function is increasing over equal-length input intervals
Test to see whether the function is linear or exponential
Linear functions change by adding or subtracting a constant amount
Exponential functions change by multiplying or dividing a constant amount
This example is increasing x2 from 8-16 and 16-32
So this function is exponential because over equal-length intervals of 1, f(x) proportionally changes by a ratio of 2
If this was linear you could say, the function is linear because over equal-length input intervals of ___, f(x) has a constant rate of change of ___
Finding a common difference/ratio from a set of points
Common Difference
Given the points (a, b) & (c, d), you can find the common difference using the slope formula →
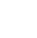
Common Ratio
1 | 2 | 3 |
4 | ? | 16 |
Find how many times r would have to be multiplied to get from 4 to 16 (in this case it is 2)
This means we would have to multiply 4 ⋅ r ⋅ r to get 16 → 4r² = 16
Then, you solve for r → r² = 16/4 = 4 → r = √4 = 2
So r = 2
OR… given the points (a, b) & (c, d), you can find the common ratio using this equation →
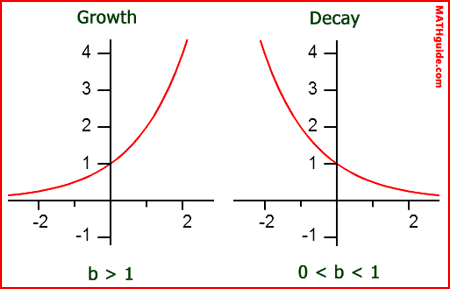
2.3 - Exponential Functions
General form of an exponential function → f(x) = abx
a = initial value
a ≠ 0 & b > 0
Exponential growth → when a > 0 & b > 1
Exponential decay → when a > 0 & 0 < b < 1
Determining exponential function based on output values
Ex. 3 × 3 × 3 × 3 × 6
a, the input value, will be the odd one out: 6
the rest are the common ratio multiplied by however many times
f(x) = 6(3)x where x = 4
Exponential Functions depending on parameters
a > 0; b > 1
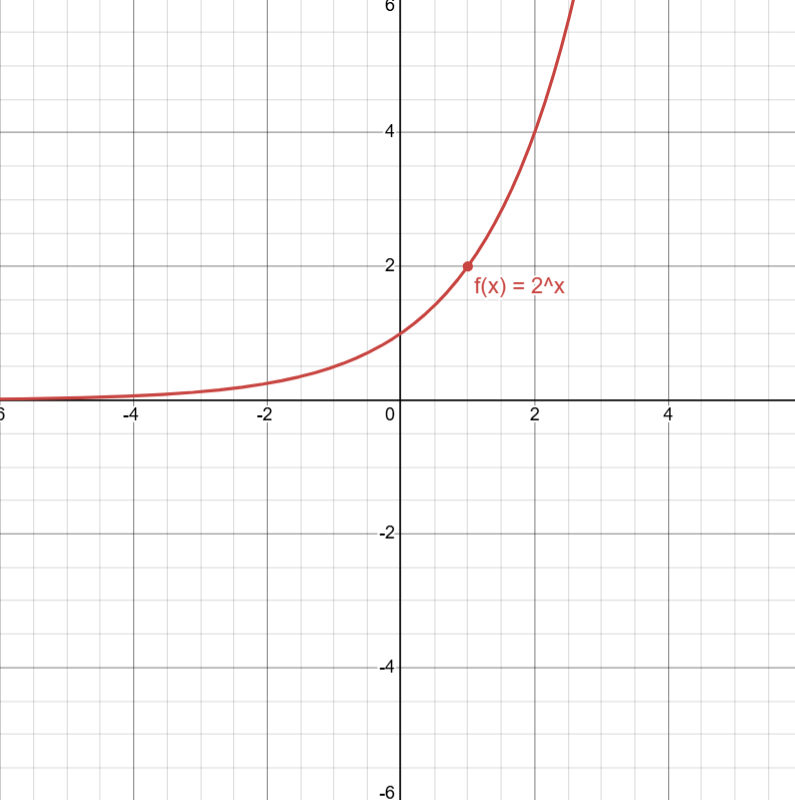 | a > 0; 0 < b < 1
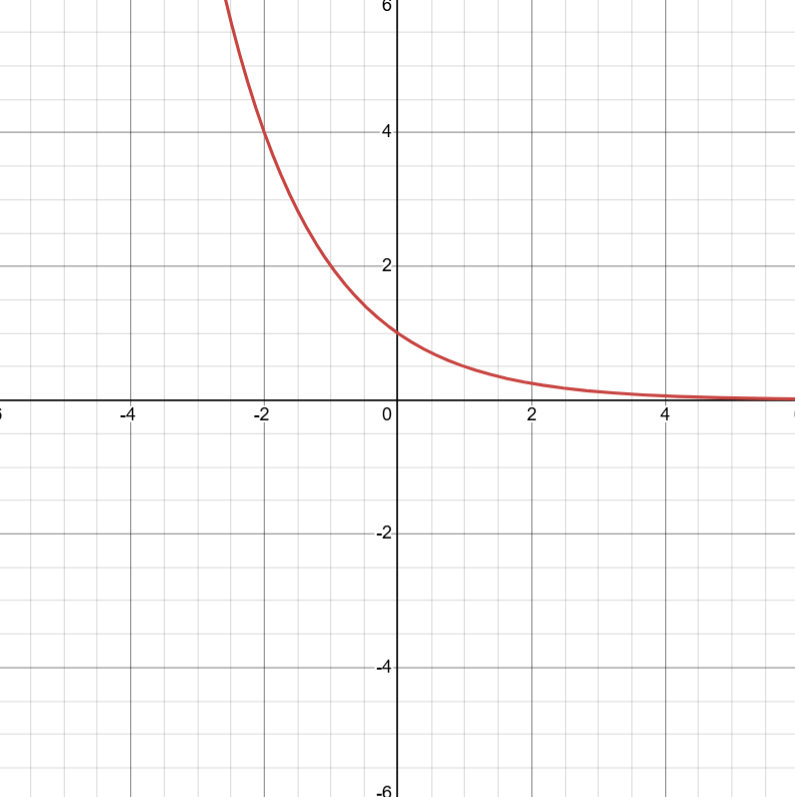 |
a < 0; b > 1
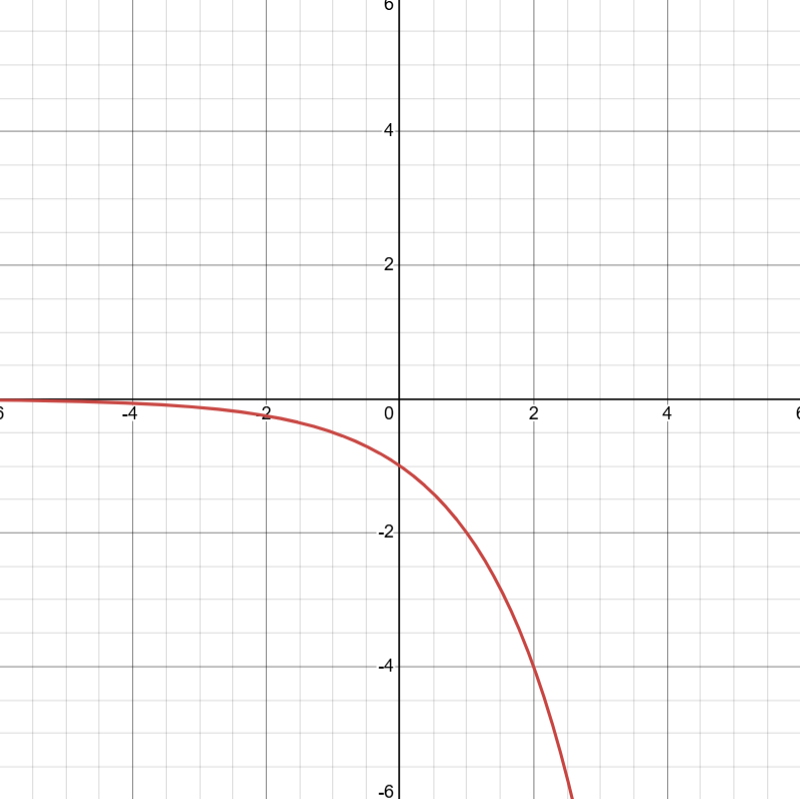 | a < 0; 0 < b < 1
 |
Characteristics of Exponential Functions
They are always increasing or decreasing
There is no extreme (except on closed intervals)
Their graphs are always concave up or concave down
They have no inflection points
If the input values increase or decrease without bound, the end-behavior can be expressed as
limx → ±∞ abx = 0
limx → ±∞ abx = ±∞
Additive Transformation of an Exponential Function
g(x) = f(x) + k
If the output values of g are proportional over equal-length input-value intervals, then f is exponential
Meaning that if g(x) has proportional output values, then f(x) will always be exponential
But if f(x) is proportional, that doesn’t mean g(x) will be proportional

2.4 - Exponential Function Manipulation
Negative Exponent Property → b-n = 1/bn
Product Property → bn * bm = bn + m
Product Property → (bm)n = bmn
Transformations of Exponential functions

Horizontal Translations & Vertical Dilations
ac(b)x = bkbx = b(x + k)
Through the product property, when doing a horizontal translation, it is the same as doing a vertical dilation
Every horizontal translation of an exponential function, f(x) = b(x + k), is equivalent to a vertical dilation
Ex. 1(2)(x + 3) → 1 × 23(2x) → 8(2)x
Horizontal translation left by 3 units / Vertical dilation of 8 units
Horizontal Dilations
Every horizontal dilation of an exponential function, f(x) = b(cx), is equivalent to a change of base
(bc)x → bc and c ≠ 0
Ex. 23x → (23)x → 8x
The kth Root of b
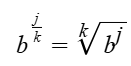
2.5 - Exponential Function Context & Data Modeling
Finding the exponential function in the form f(x) = abx + k through a table
x | 0 | 1 | 2 | 3 | 4 |
f(x) | 6 | 9 | 15 | 27 | 51 |
Find the ratio (b) (in this case b=2)
Find the differences
9-6 = +3; 15-9= +6; 27-15= +12; 51-27= +24
Then, you can find the ratio
3 × 2 = 6 × 2 = 12 × 2 = 24
Then find a and k by setting up a system of equations
6 = a(2)0 + k → 6 = a + k
9 = a(2)1 + k → 9 = 2a + k
9 = 2a + k
-6 = -a - k
a = 3
6 = 3 + k → k = 3
The number e
e ≈ 2.718…
The natural base e is often used as the base in exponential functions that model contextual scenarios
Percent Increase:
Start with the initial amount a and grow with a % increase
y = a(1 + %increase)x
Percent Decrease:
Start with the initial amount a and decay with a % decrease
y = a(1 - %decrease)x
Half-Life:
An initial amount a that shrinks by half every h
f(t) = a(1/2)t/h
Doubling time:
An initial amount a that doubles every d
f(t) = a(2)t/d
Equivalent Forms
If t represents the number of weeks, then the base of f(t) = (1.01)t indicates that the quantity increases by a factor of 1.01 every week
If you want the equation for every year, then that equation would look like this → (1.0152)t/52
This is because every 52 weeks, t = 52/52, means that it would account for 1 year
since we’re counting years, 1.0152 means that in 1 year, we’d have (1.01 × 1.01 × 1.01 … 1.01) 52 times per year
So when t = 52 weeks / 52 (one year), 1.01 would’ve increased 52 times
If you wanted an equation for every day, then it would look like this → (1.011/7)t
This is because once t is > 7, the function will equal 1.01 which is the same as if it where (1.01)1 weeks
2.6 - Competing Function Model Validation
The only way to know what model fits an equation best is to use context clues
Residual Value - a measure of how much a regression curve vertically misses a data point
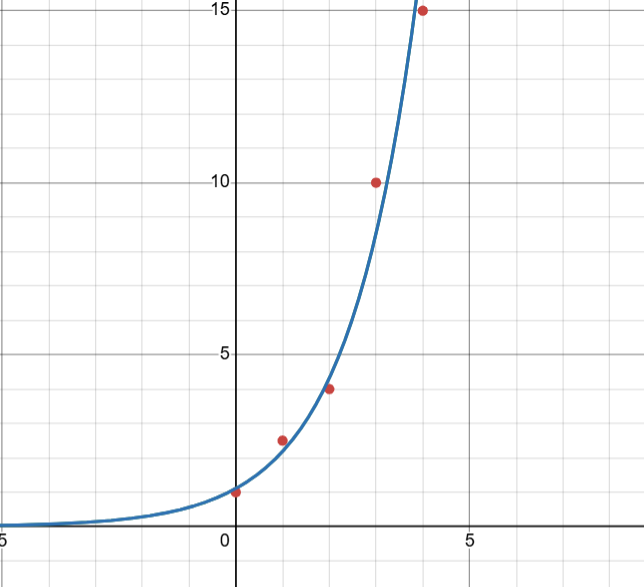
Residuals → Actual - Predicted (AP)
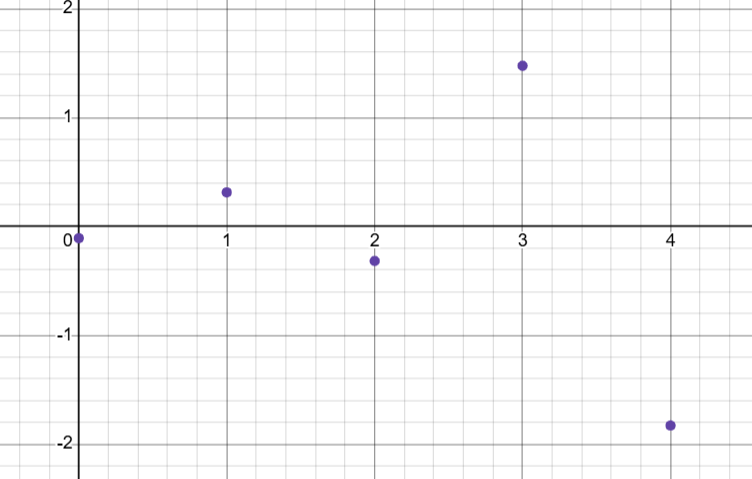
The difference between the predicted and actual values is the error
Error → Predicted - Actual (PA)
A positive error means that the model overestimated (you can see the y=0 line is above the point)
A negative error means that the model underestimated (you can see the y=0 line is below the point
Residual Plot Patterns
If there is a clear pattern in the residual plot, it is a bad regression model
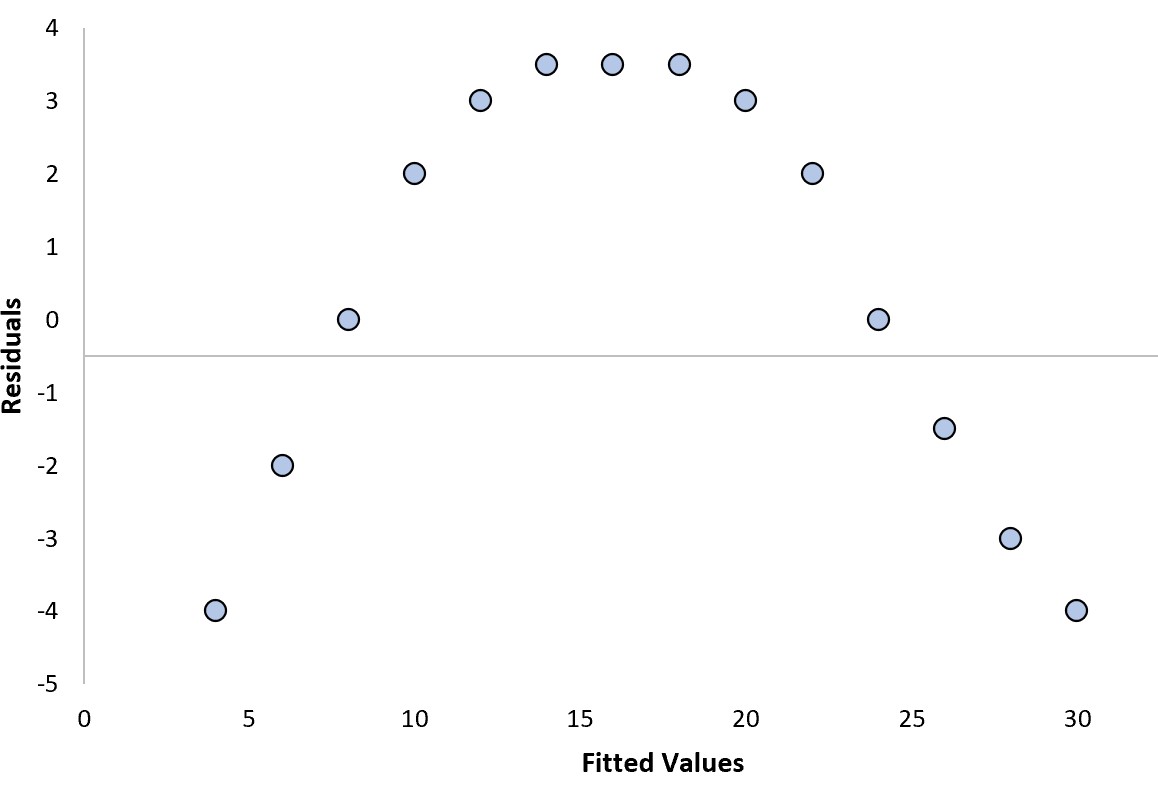
2.7 - Composition of Functions
The composition of functions means we take one function and substitute it into the other function
f(g(x) or f ∘ g(x)
Ex. f(x) = 2x + 3; g(x) = 5x²; find f(g(5))
5(5)² → 5(25) → 125
2(125) + 3 = 253
Identity Function
When f(x) = x
then f(g(x)) = g(x)
Finding a Composed Function
f(x) = x² - 1 & g(x) = √x ; find f(g(x))
h(x) = (√x)² -1
h(x) = x - 1
Domain of a Composed Function
When finding the domain of a function h(x) = f(g(x)), there are 2 things to consider
because g(x) is the input, we can only use x-values in the domain of g
any restrictions on the domain of f(g(x)) must be included
Ex. problem above in Finding a Composed Function
g(x) is the input, so it can only be from [0, ∞)
and there are no restrictions in x - 1, so the domain is [0, ∞)
Decomposition of Functions
if there is a radical, one function can simply be √x (this applies with 1/x as well)
Ex. h(x) = √x³ + 1 ; find f(g(x))
f(x) = √x
g(x) = x³ + 1
Ex2. h(x) = 1/(x² + 1) ; find g(f(x))
f(x) = x² + 1
g(x) = 1/x
2.8 - Inverse Functions
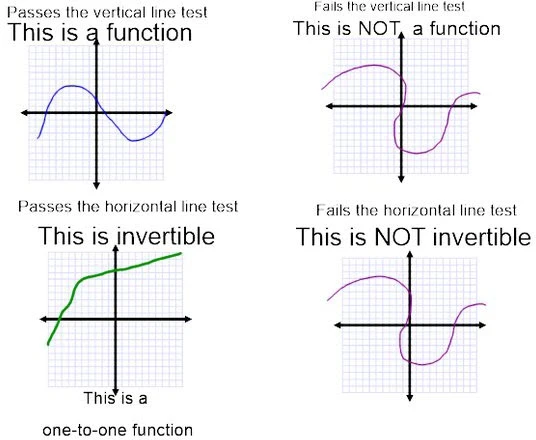
The inverse of a function is a reverse mapping of a function where (x, y) → (y, x)
They should reflect across the line y=x
If the graph turns (has a max/min) then it is no longer invertible because there will be output values that are the same for different input values
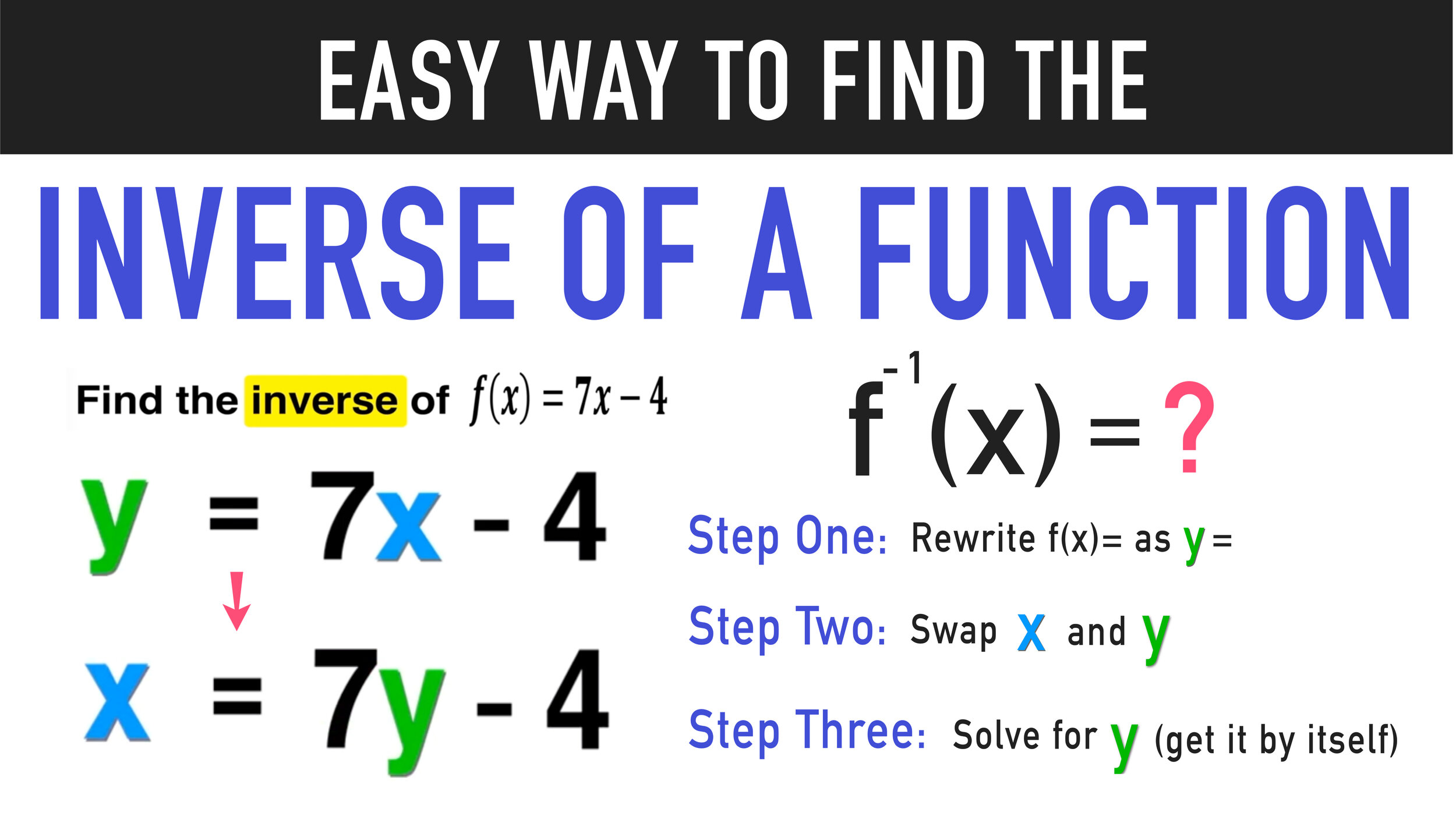
Find the Inverse of a function
instead of (for example) y = mx + b, switch the x and y so it is → x = my +b
solve for y
Finding the domain and range
The domain and range swap for inverse functions
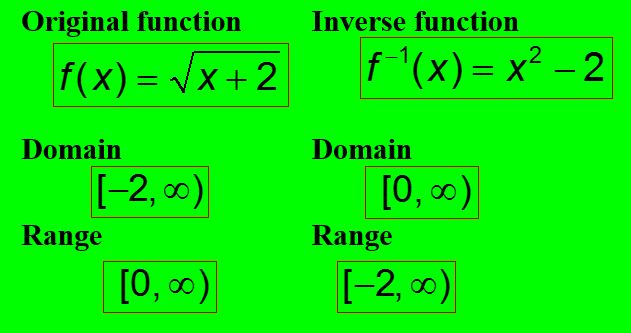
Composition of f and f-1
f(f-1(x)) = f-1(f(x)) = x
If both functions, when composed with each other, cancel out, then they are inverses of each other
2.9 - Logarithmic Expressions
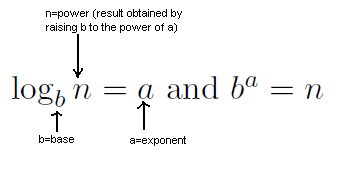
A logarithm is a way to find an unknown power using the base and a number
Ex.
log2(64) = 6 | 26 = 64
2.10 - Inverses of Exponential Functions
The inverse of an exponential function can be modeled through a logarithmic function.
Exponential vs. Logarithmic
Exponential | Logarithmic |
x values will be linear (1, 2, 3, 4) | x values will change proportionally (3, 9, 27, 81) |
y values change proportionally (2, 4, 8, 16) | y values are linear (1, 2, 3, 4) |
Power Rule → 2log5(x) = log5(x2)
Raising to a log power
ac(logb(x))
if a and b are the same and c = 1, then you can cancel out to get x
2log2(x) = x
You can also cancel out to get x if the bases are the same in this scenario → log2(2x) = x
Using these two, you can find if an exponential function and a logarithm function are inverses by plugging each function into each other (ex. f(g(x)) = x = g(f(x)))
2.11 - Logarithmic Functions
Logarithmic functions have inverse properties as exponential functions
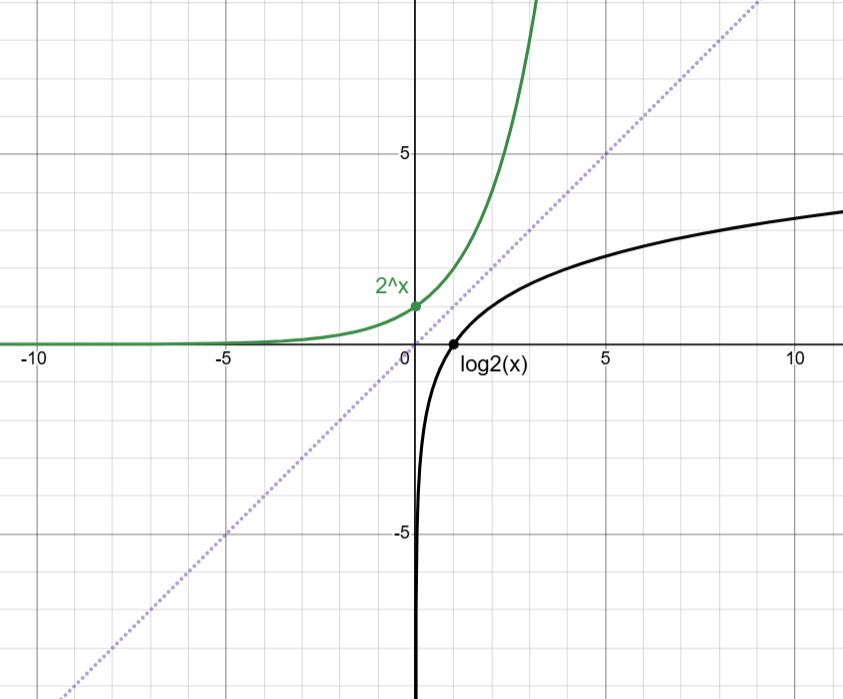
Exponential
x-intercept: NA
y-intercept: (0, 1)
asymptote: y = 0
increasing: (-∞, ∞)
decreasing: NA (only when 0 < b < 1)
domain: (-∞, ∞)
range: (0, ∞)
Logarithmic
x-intercept: (0, 1)
y-intercept: NA
asymptote: x = 0
increasing: (-∞, ∞)
decreasing: NA (only when 0 < b < 1)
domain: (0, ∞)
range: (-∞, ∞)
Transformations of Logarithm Graphs
Horizontal Translations
logb(x ± h)
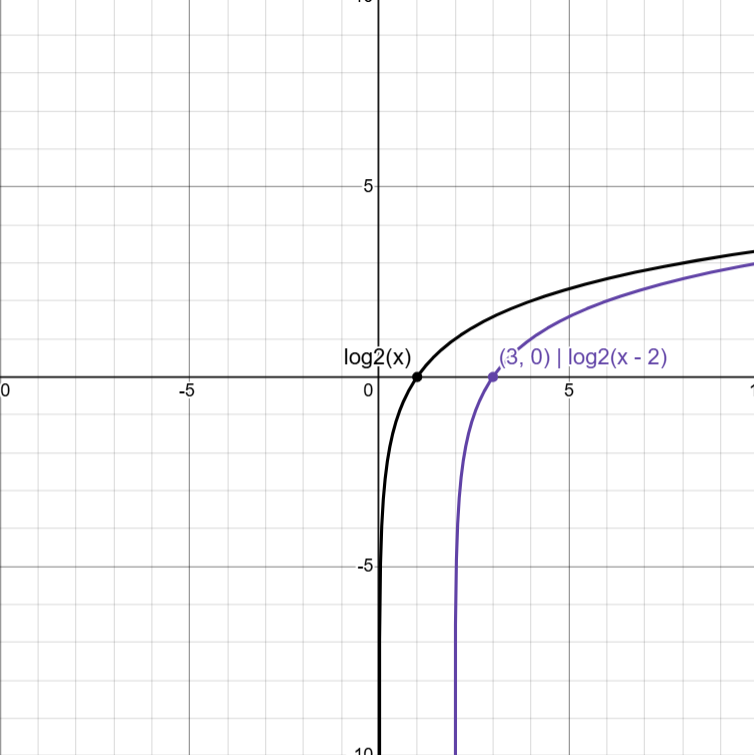
CHANGES DOMAIN
Vertical Translations
logb(x) + k
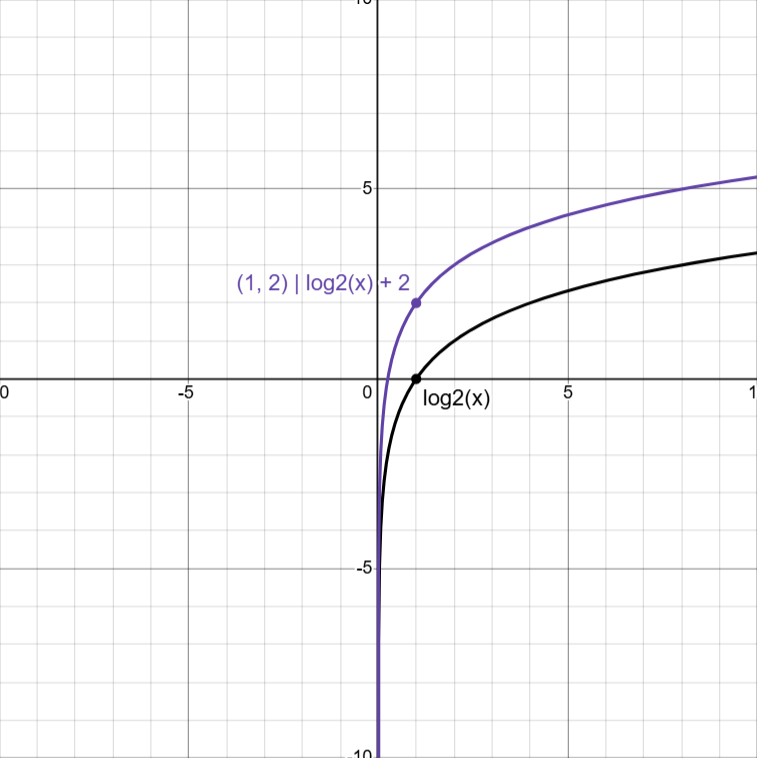
Horizontal Dilation
logb(cx)
Compresses x-values by 1/c

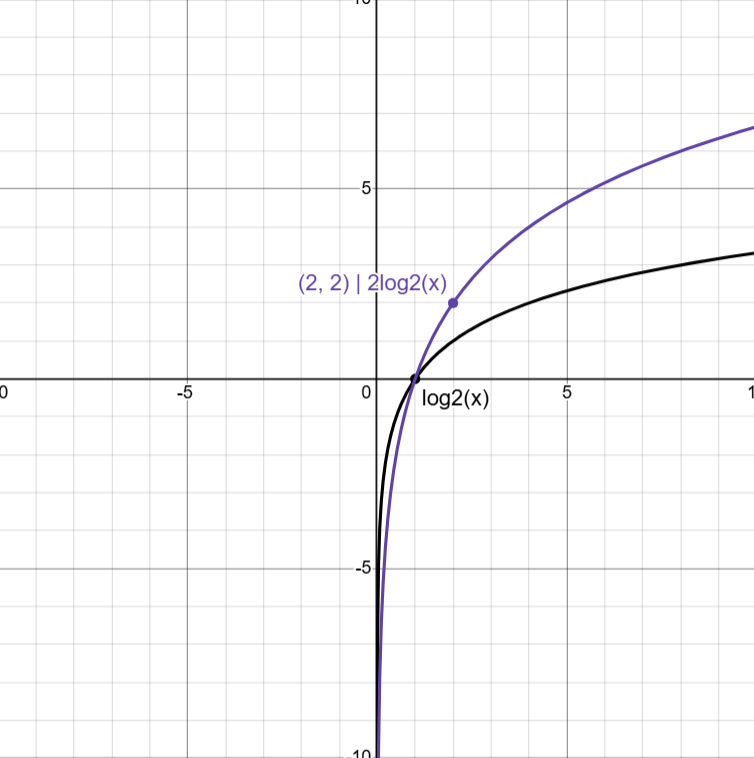
Vertical Dilation
alogb(x)
Extends y-values by a
Horizontal Reflection
logb(-x)
Reflection over y-axis
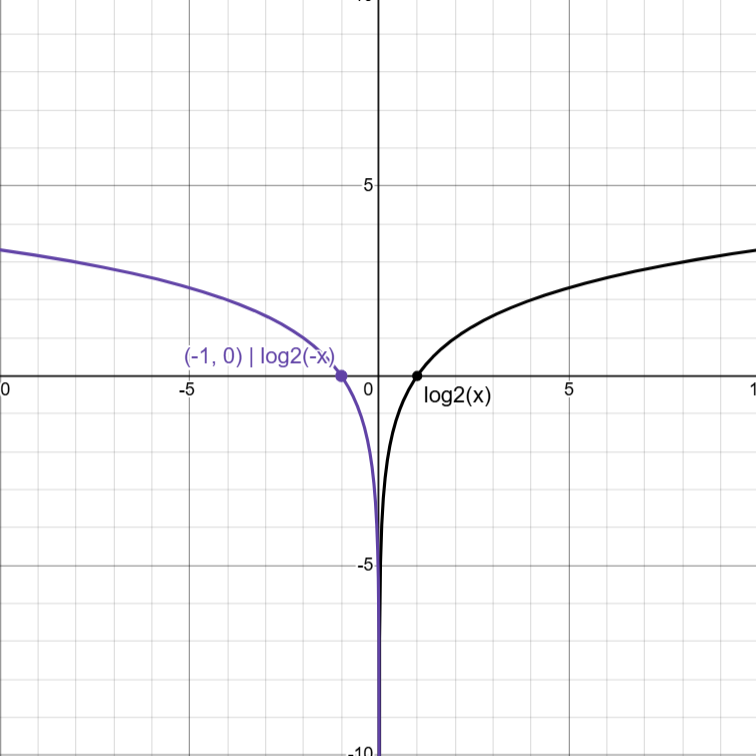
CHANGES DOMAIN
Vertical Reflection
-logb(x)
Reflection over x-axis
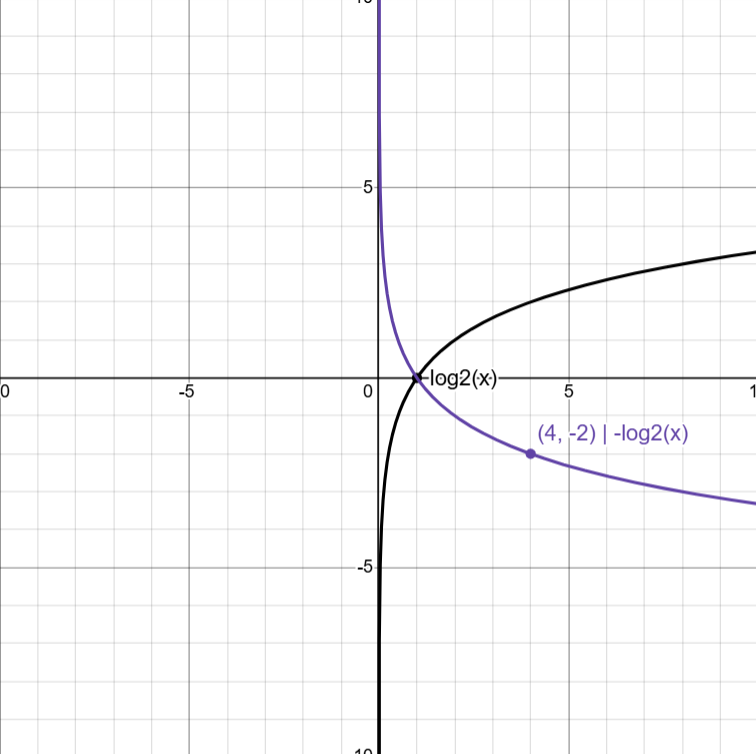
2.12 - Logarithmic Function Manipulation
Product Property → logb(xy) = logbx + logby
Quotient Property → logb(x ÷ y) = logbx - logby
Power Property → logbxk = klogbx
Change Base Formula
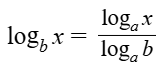
Finding transformations through manipulation
f(x) = log2(4x)
f(x) = log24 + log2x
f(x) = log2(22) + log2x
f(x) = 2 + log2x
This means that the function f(x) has a vertical translation of 2
2.13 - Exponential & Logarithmic Equations & Inequalities
Logarithmic Equations
If you have logs with the same base on each side, you can cancel them
log2(x + 3) = log2(5) → x + 3 = 5
You can also use logs to solve exponential equations
2(3)x - 3 = 18 → 3x - 3 = 9 → 3x - 3 = 32 → x - 3 = 2
Inequalities
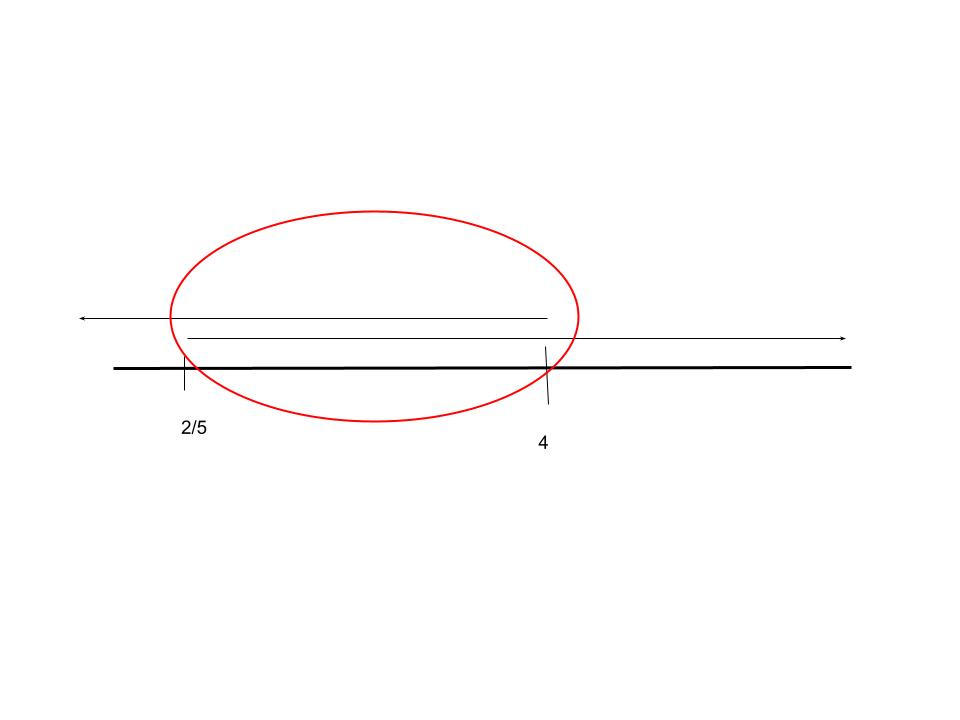
First, you need to find out what restrictions there are in the inequality (making sure they are positive)
log(x + 2) + log(3) > log(5x - 2)
x + 2 > 0 → x > -2 (won’t work because it is below zero)
0 < 5x - 2 → x > 2/5
Then, you need to solve the equation
log(3x + 6) > log(5x - 2) → 3x + 6 > 5x - 2 → 6 > 2x - 2 → 8 > 2x → x < 4
Then you follow along with the restrictions and your output to get → 2/5 < x < 4
2.14 - Logarithmic Function Context and Data Modeling
Usually, when given datasets, if the x-values are proportional and the y-values are linear, then the table is logarithmic
x | 1 | 3 | 9 | 27 | 81 |
y | 3 | 5 | 7 | 9 | 11 |
You can use your calculator to use natural log regression to find the equation for these points
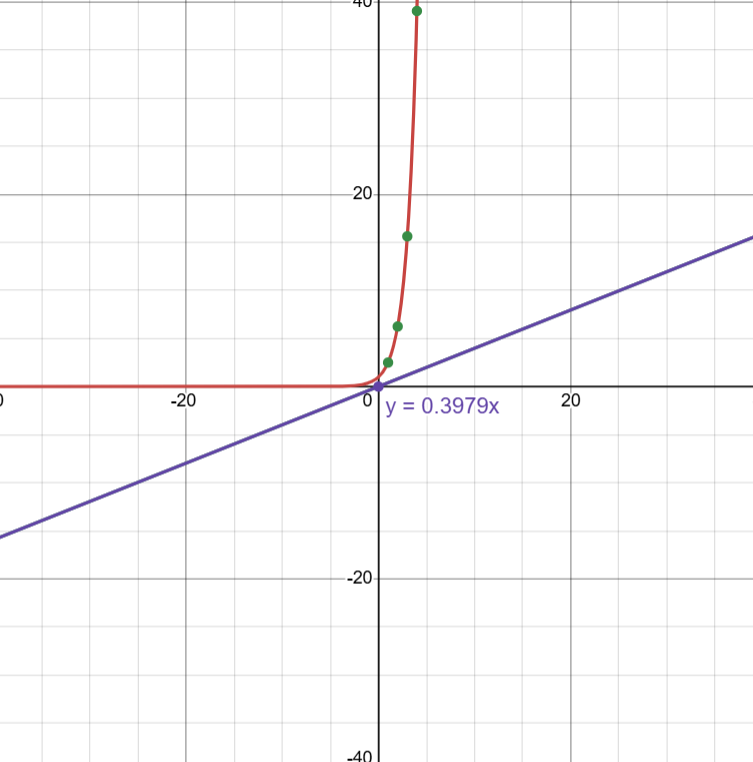
2.15 - Semi-Log Plots
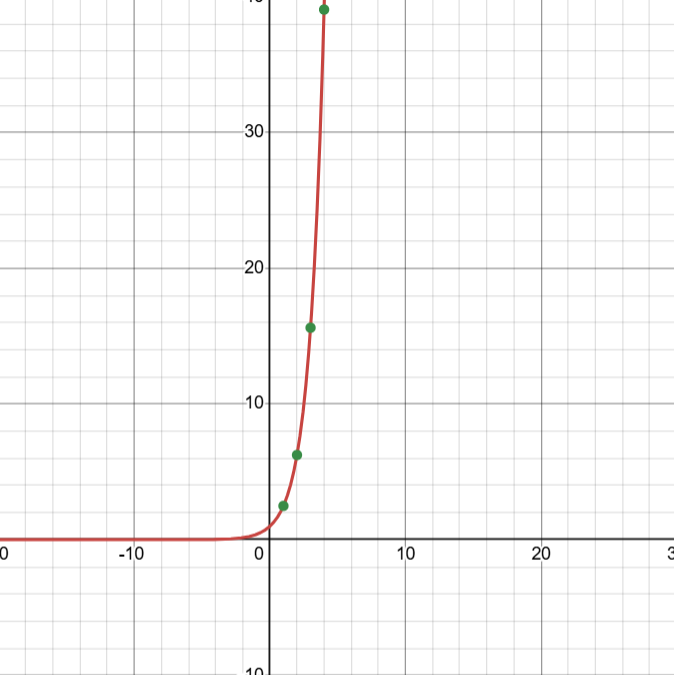
Taking these points…
x | 1 | 2 | 3 | 4 |
y | 2.5 | 6.25 | 15.625 | 39.0625 |
… you get this exponential graph…
However, when you switch to a semi-log plot, the y-values appear as bases of 10 (100, 101, 102) and the graph appears linear…
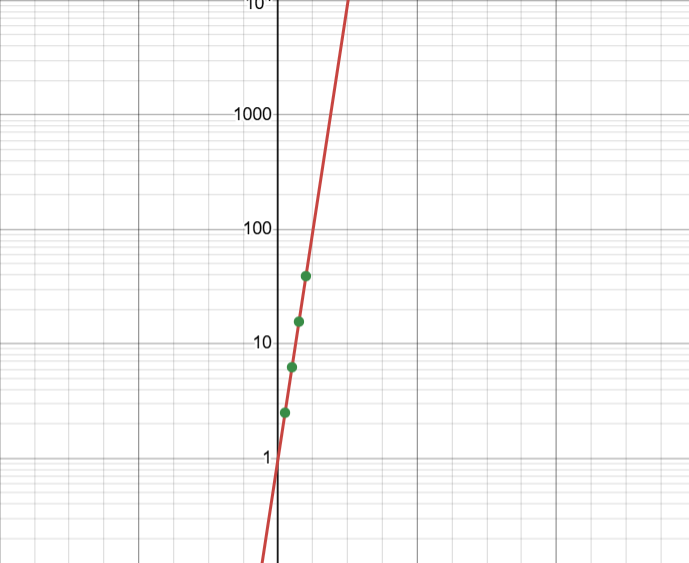
Taking the log the equation gives this straight, linear line:
y = 1(2.5)x → log(y) = log(1) + log(2.5x) → log(y) = 0 + xlog(2.5) → y = 0.3979x
This is the same as taking the log of each of the y-values of the original function.
Unit 3
3.1 - Periodic Phenomena

Periodic Functions have a repeating pattern over successive equal-length intervals
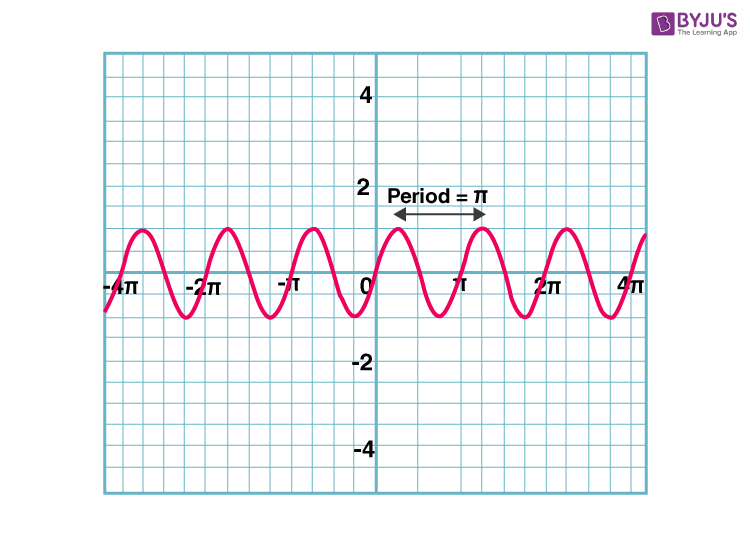
The space in between each pattern repeat is the period
The period in the function is the smallest positive value of k such that f(x + k) = f(x)
3.2A - Radians & 3.2B - Sin, Cosine, and Tangent
Angles in standard position will always start on the x axis, going from the origin out towards positive infinity
the Initial Side will always be on the positive x-axis between Quadrants I & IV
the Terminal Side will be the other ray of the angle
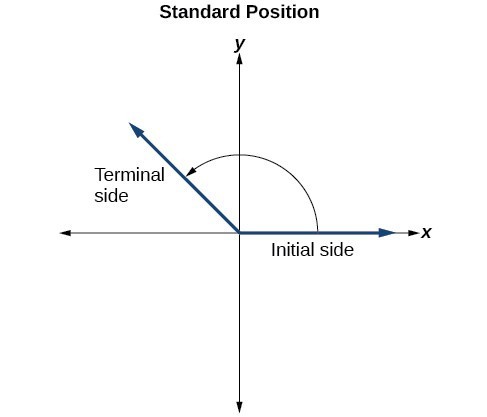
Negative Angles are made like this →

Radians → a unit of angle, equal to an angle at the center of a circle whose arc is equal in length to the radius
Example
π = 180 degrees
3π/2 = 270 degrees
π/2 = 90 degrees
0 / 2π = 0 / 360 degrees (in the unit circle, 360 degrees = 2π because the circumference C = 2πr would be C = 2π(1))
When the rays of an angle touch the circle, the arc of the circle is subtended by the angle
θ (the angle measure in radians) is thus = s (the arc length subtended by the rays) / r (the radius)
θ = s/r
A radian could also be defined as when the angle creates an arc length on a circle that is equal to the radius of the circle; so θ = 1 and s = r
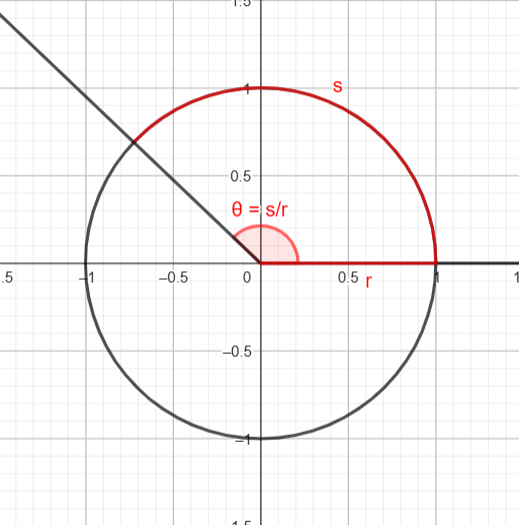
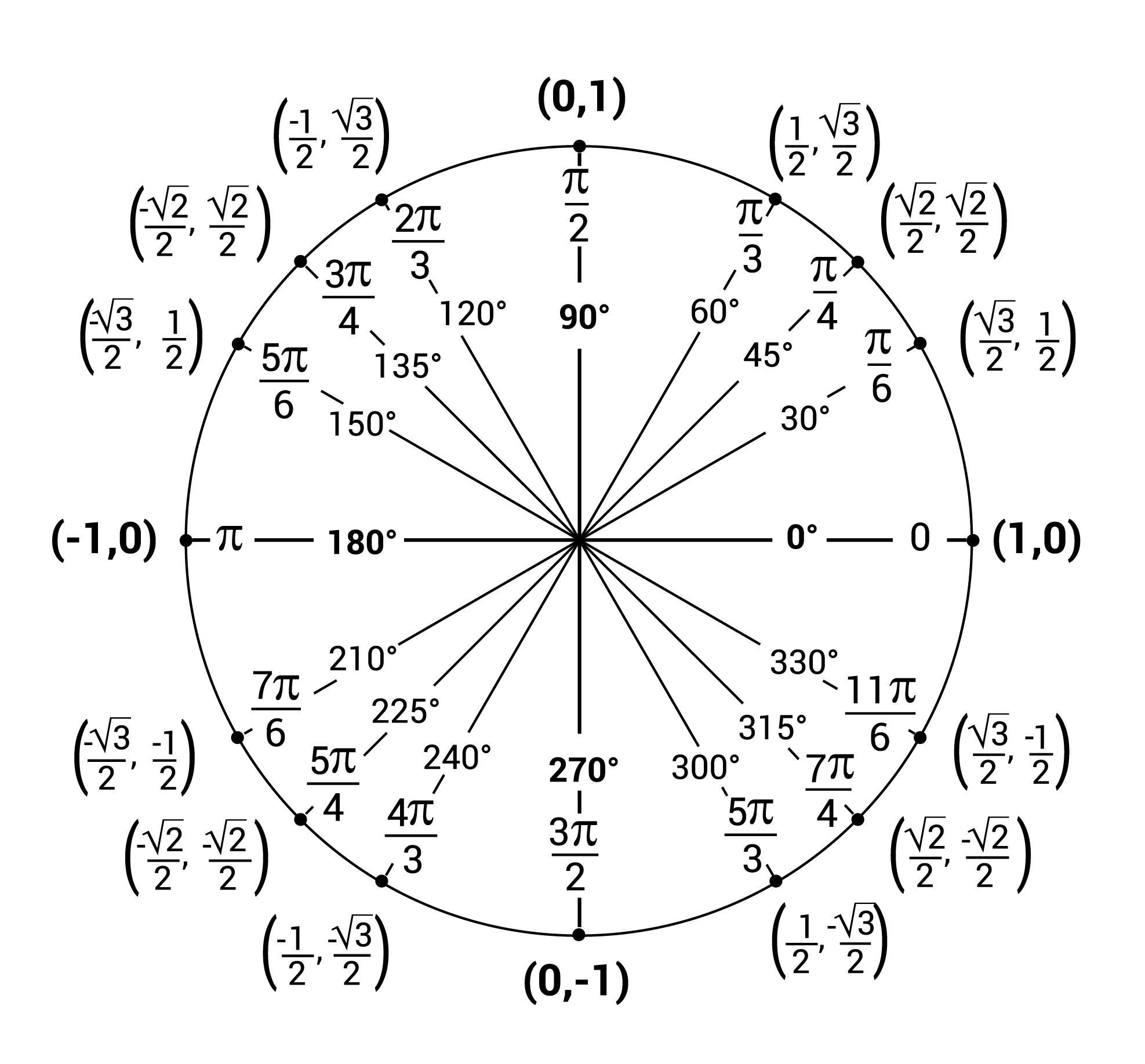
The Unit Circle
The unit circle is a circle with a radius of 1
because it has a radius of 1 → θ = s
REMEMBER THE UNIT CIRCLE
One Revolution = 2π
½ Revolution = π
¼ Revolution = π/2
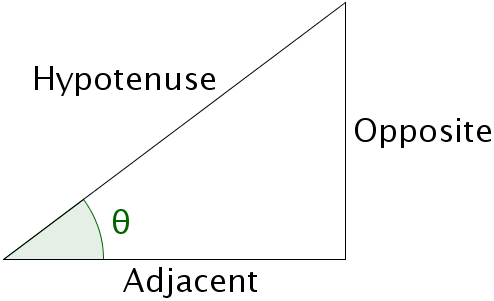
Sine, Cosine, Tangent
Remember → SOHCAHTOA
sin θ = opposite / hypotenuse
cos θ = adjacent / hypotenuse
tan θ = opposite / adjacent
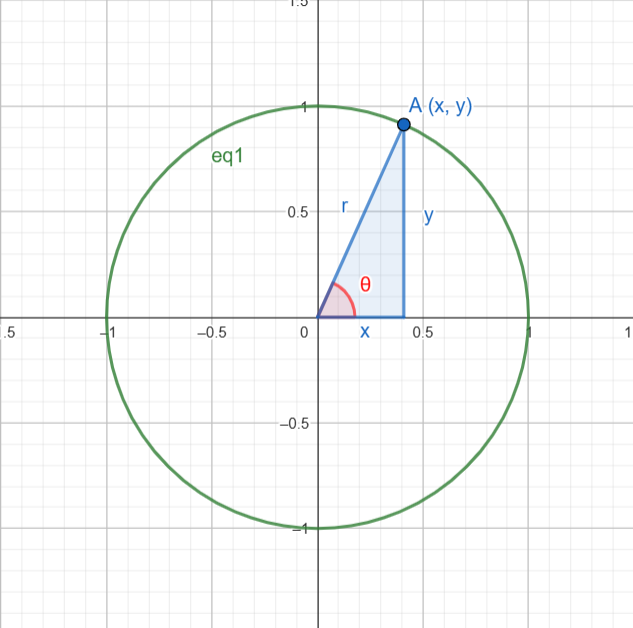
SIN θ
The sine of the angle is the ratio of the vertical displacement of A from the x-axis to the distance between the origin and point A
In the unit circle, when r = 1, sin θ = y
sin θ = y / r
COS θ
The cosine of the angle is the ratio of the horizontal displacement of A from the y-axis to the distance between the origin and point A
In the unit circle, when r = 1, cos θ = x
cos θ = x / r
TAN θ
The tangent of the angle is the slope
tan θ = y / x = sin θ / cos θ
3.3 - Sine and Cosine Function Values
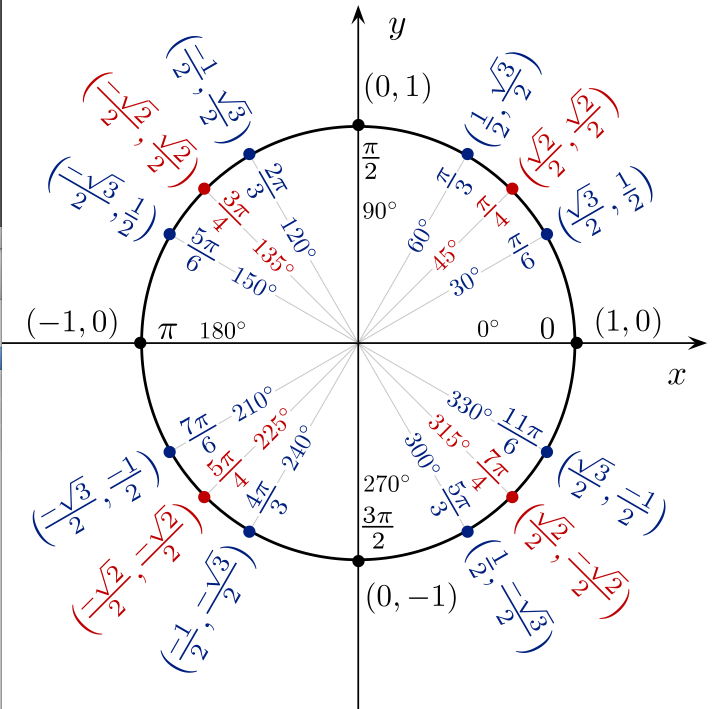

45, 45, 90 & 30, 60, 90 Triangles
By knowing the unit circle, we can find the coordinates for the sine and cosine values of π/4 (45 degree angle)
sin(π/4) = 1/√2 = √2/2
cos(π/4) = 1/√2 = √2/2
These coordinates appear on the unit circle!
Another example with another value of π/4
sin(5π/4) = -√2/2
cos(5π/4) = -√2/2
The same goes with values of π/6, the sine and cosine of those values will always be equal to the x and y values inside the unit circle
In the example, the triangles are the same within the unit circle:
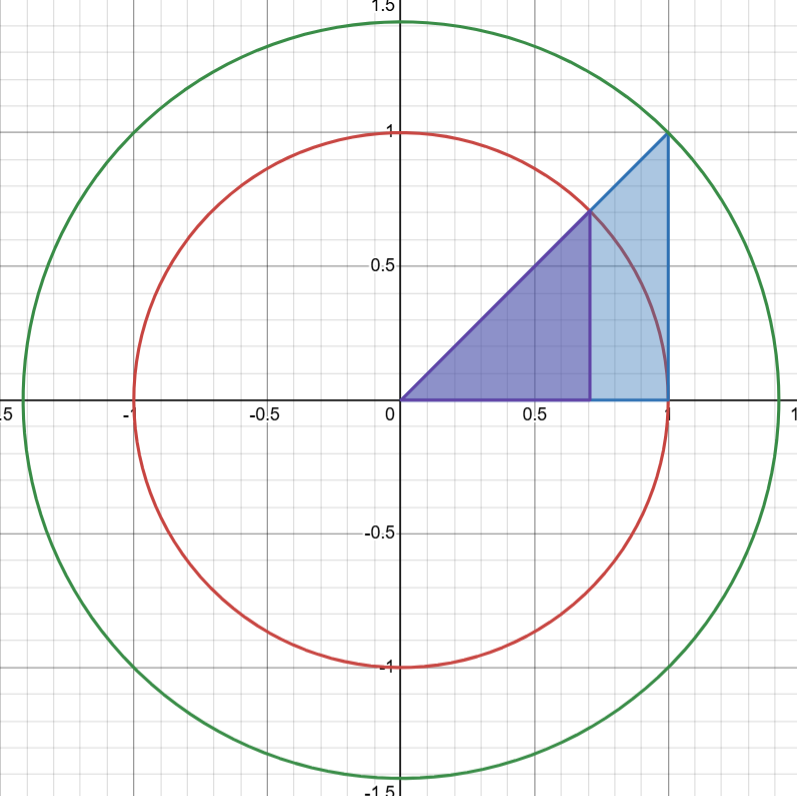
3.3 - Continued
Because…
cos θ = x / r
sin θ = y / r
This means…
x = r × cos θ
y = r × sin θ
This allows us to find any coordinate point if we know the angle in standard position and the circle’s radius
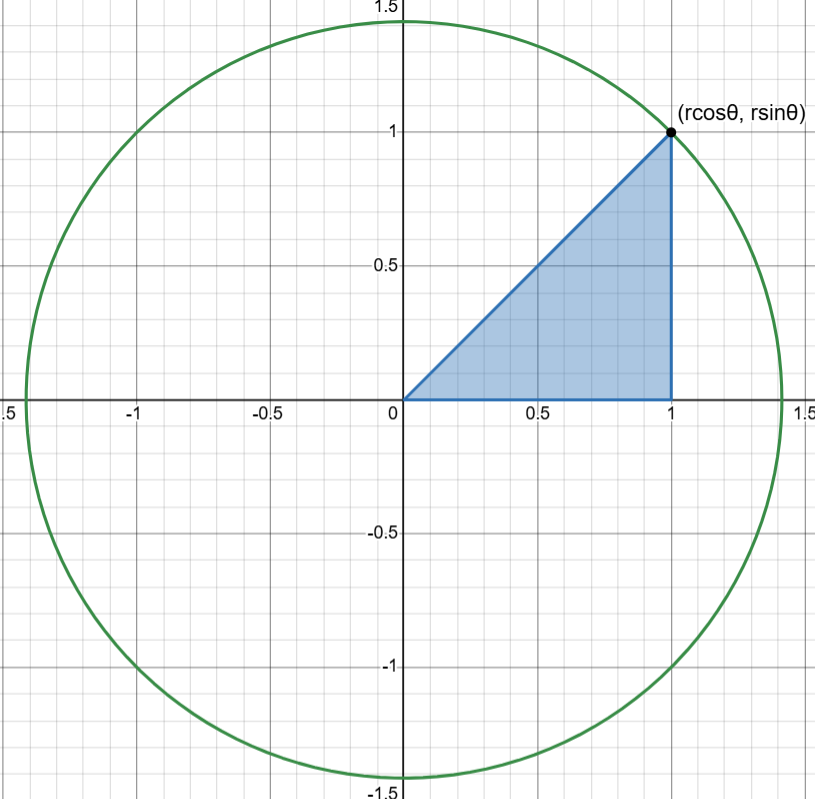
Example
θ = 5π/6, r = 6
x = 6cos(5π/6) → 6(-√3/2) → -3√3
y = 6sin(5π/6) → 6(1/2) → 3
so the coordinates of the point of intersection of the terminal ray and the circle is (-3√3, 3)
3.4 - Sine and Cosine Function Graphs
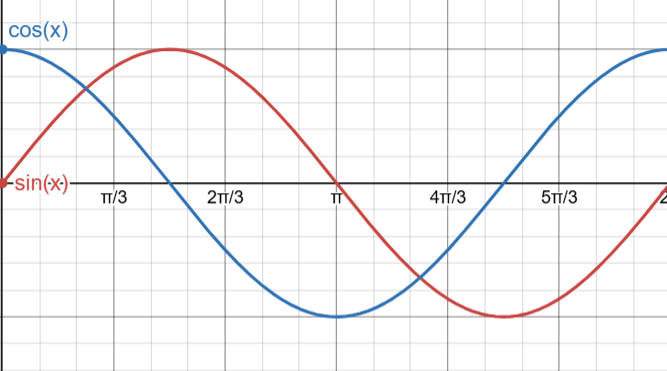
Here is an image of the sine and cosine graphs.
sin(x)
sinθ increases
0 → π/2 & 3π/2 → 2π
sinθ decreases
π/2 → 3π/2
sinθ is concave up
π → 2π
sinθ is concave down
0 → π
cos(x)
cosθ increases
π → 2π
cosθ decreases
0 → π
cosθ is concave up
π/2 → 3π/2
cosθ is concave down
0 → π/2 & 3π/2 → 2π
3.5 - Sinusoidal Functions
cos θ = sin(θ + π/2)
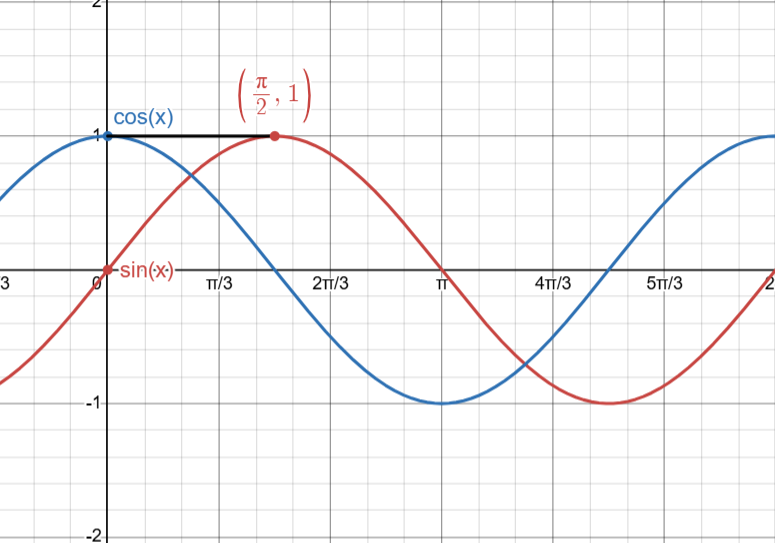
Standard Equations
y = a cos θ
y = a sin θ
a is a vertical stretch or shrink (if negative, reflect over x-axis)
Amplitude → | a |; half the difference between the maximum and minimum values
Midline → a horizontal line halfway between the max and min values
Period → the reciprocal of frequency (ex. 2π); the change in θ values required for the function to complete one full cycle
Frequency → the reciprocal of period (ex. 1/2π); the number of cycles the graph completes per one radian
3.6 - Sinusoidal Function Transformations
Standard Equations
y = a cos(b(x - c)) + d
y = a sin(b(x - c)) + d
a is the vertical stretch or shrink (- = reflect over x-axis)
b is the horizontal dilation (- = reflect over y-axis)
0 < b < 1 → horizontal stretch
b > 1 → horizontal shrink
b can be found by taking the period and setting it equal to 2π/|b|
Ex.
period = 20
2π/b = 20 → 20b = 2π → b = 2π/20 → b = π/10
d is the vertical shift
the midline is y = d
-c = a shift right; +c = a shift left
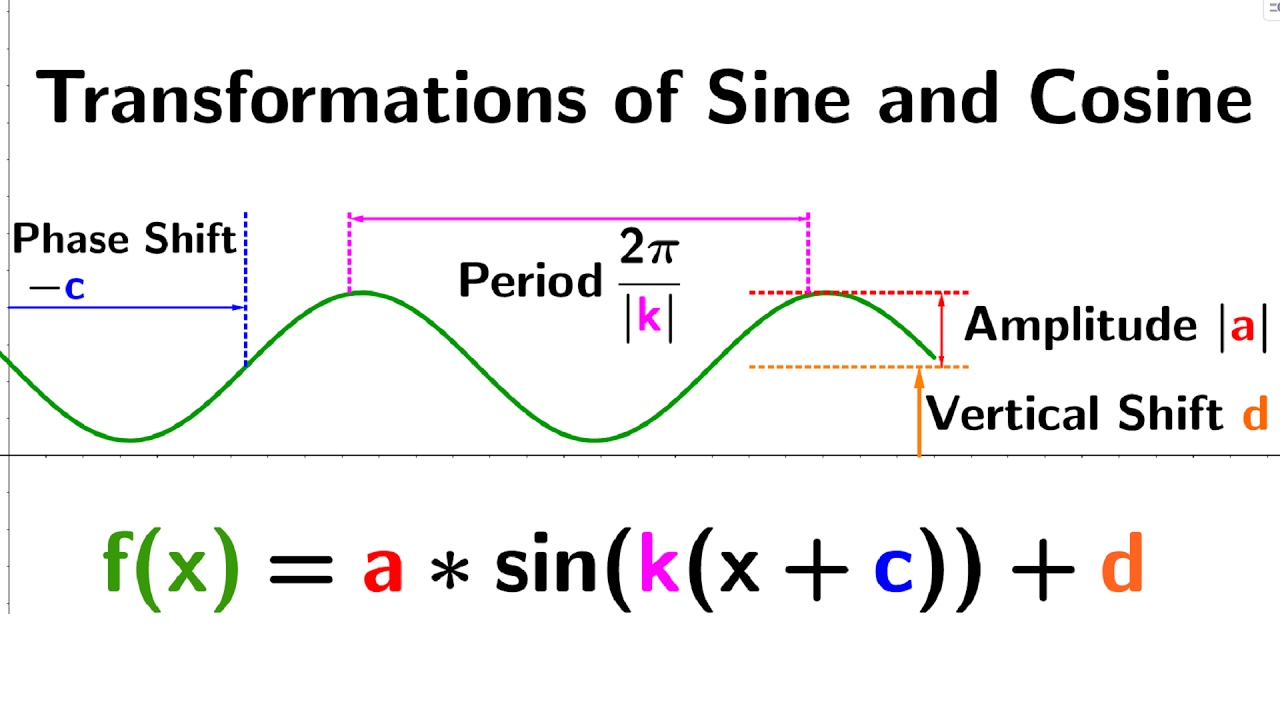
Period → 2π ÷ |b|
Frequency → |b| ÷ 2π
3.7 - Sinusoidal Function Context and Data Modeling
Given theses datapoints…
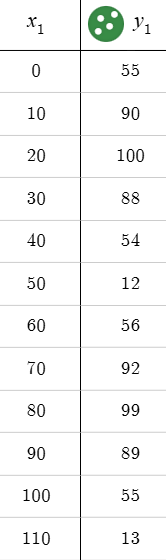
How do we model a sinusoidal function through them?
Remember: a * sin(b(x - c)) + d
Creating a Sinusoidal Function Manually
Estimate the period and frequency
we know that
max = (20, 100)
min = (50, 12)
Other max values would be → (80, 99)
Other min values would be → (110, 13)
By taking both max values and subtracting them, we get the period
80 - 20 = 60
And therefore the frequency = 1/60
Estimate the vertical shift / midline
The midline is calculated in the middle of both extremas
(100 + 12) / 2 = 56
y = 56
d = 56
Estimate the amplitude
amplitude can be found by taking one extrema and subtracting it from the midline
a = 100 - 56 = 44
Find the horizontal shift
Sine Functions
For sine functions, we know that the period is 60
We know that we will hit another min value for every half period (30)
The next min value before 20 would be at -10 (20-30=-10)
The sine function starts at the midpoint between a min and a max, so finding the middle of these two points would be at x = (-10+20)/2 = 5
This means the graph is shifted 5 to the right
c = 5
Cosine Functions
For cosine functions, you take the maximum point and subtract it from x = 0
So our c value would be 20
Make the function
To find b, take the period and set it equal to 2π/|b| (60 = 2π/b → b = 2π/60)
Putting it all together
Sine function

Cosine function
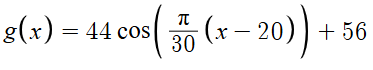
You can also do this with a calculator to like we did with previous functions earlier!
3.8 - The Tangent Function
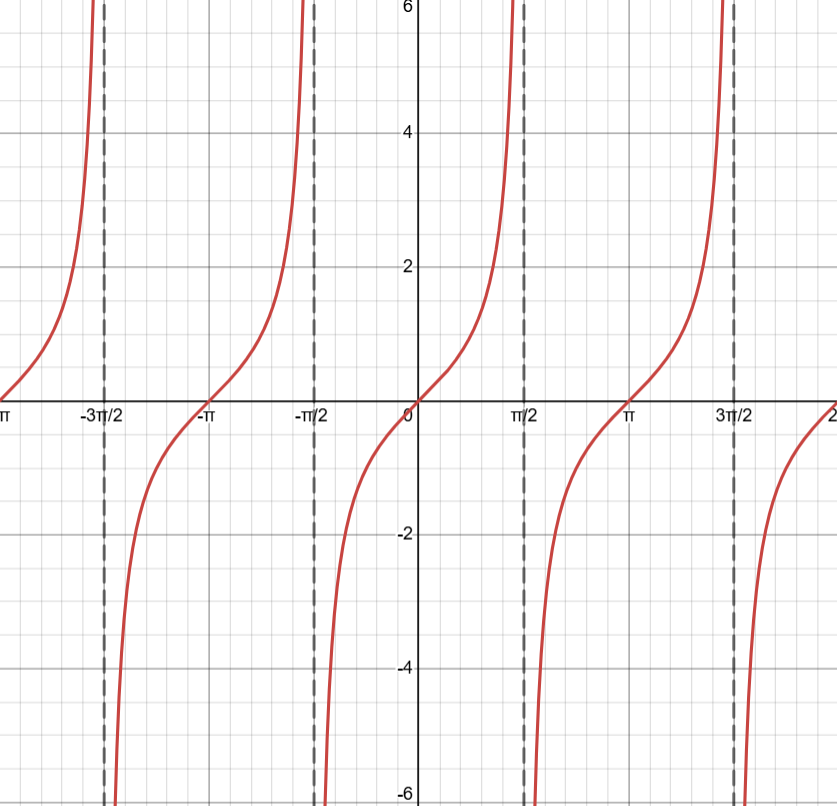
the tangent function gives the slope of the terminal ray
tan θ = sin θ / cos θ = y / x
Example:
Properties of tan(x)
the slope starts at 0
it gets large and approaches ∞
at π/2, the slope is undefined
every ½ revolution of the circle, the tan function repeats
the period of tan(x) is π
the period of tan(bx) = π/|b|
Vertical Asymptotes
for tan(θ) without a b value, a vertical asymptote appears at every…
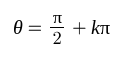
where k is an integer
you start at π/2 and π is the period
for tan(bθ) with a b value, a vertical asymptote appears at every…

where k is any integer
Manipulations of tan(x)
f(x) = a * tan(b(x + c)) + d
a →creates a vertical dilation by a factor of |a|; if a < 0, there is a reflection over the x-axis
b → creates a horizontal dilation and changes the period by a factor of |1/b|; if b < 0, there is a reflection over the y-axis
c → creates a horizontal shift by -c units
d → creates a vertical shift by d units
 Knowt
Knowt