Gen.-physics-2-Repaired
Electric Charge
- Atom
is the basic building block of matter.
- Subatomic particles
Protons are positively charged (+e)
Electrons have negative charges (-e).
Neutrons have no charge or are electrically neutral
Electric Charge
- An electric charge determines the electric interaction and magnetic interaction between subatomic
particles and other charged particles.
- This interaction between charges is summarized in the phrase “like charges repel, unlike charges attract.
A glass rod and a rubber rod are used to demonstrate the interaction between electric charges. It was found out that after rubbing the rubber rod with fur and the glass rod with silk, both pairs of objects acquired charges. Two glass rods are found to repel each other, whereas a glass rod is found to be attracted to the rubber rod.
🞇 In an atom, the subatomic particles provide the net charge. An
electrically neutral atom contains an equal number of protons and electrons.
🞇 An atom that has an imbalance in the number of protons and
electrons is called an ion. Cations are positive (more protons than electrons), and anions are negative ( more electrons than protons).
🞇 As a derived SI (or International System of Units) quantity, an electric charge is represented by the symbol
“q” and measured using the unit
coulomb (C).
🞇 1 coulomb = 6.242 x 10^18 e
Law of Charges
🞇 Like charges repel each other, and unlike charges attract
each other.
🞇 Macroscopically, a body is electrically charged if the number of positive charges
it has is not equal to the number of the negative charges. In other words, the charge of a particle depends on the sum of its electrical charges.
What is the charge of each atom if it comprises the following particles?
- 5 electrons and 6 protons
- 14 protons and 11 electrons
- 5 electrons and 5 protons
- 25 protons and 26 electrons
Compute the charge of the following ions in Coulomb.
a. Ion with a charge of -2e
a. Ion with a charge of +5e
a. Ion with a charge of -3e
- Ion with a charge of -7e
- Ion with a charge of +6e
Conductors
Conductors
🞇 are materials that allow electrical charges to move from one material to another.
🞇 Conductors may be charged through different methods— rubbing, conduction, and induction.
Charging by Rubbing
🞇 An electrically neutral body can gain a charge by rubbing or friction.
🞇 Consider two different uncharged bodies. Because of the difference in their material compositions, the nuclei of their atoms pull their electrons with different strengths.
🞇 Rubbing these two bodies will force their atoms to interact with one another, resulting in the “ripping off” of electron(s)from the body with a weaker electron hold.
🞇 The “ripped off” electrons are then transferred to the other body. After rubbing, one of the bodies will have more electrons, and the other one will have fewer. Thus, both of them will now be electrically charged.
Triboelectric series
🞇 is a list of common materials that were experimented on and found to behave in a predictable way.
🞇 When these materials are rubbed together, those that appear first in the list tend to lose their electrons, making them positive.
🞇 Those latter in the list tend to gain electrons, making them negative.
🞇 In other words, if you rub any two of the materials in the series, the material in the upper part of the list will be positive, and the other material in the lower part will be negative.
🞇 Using the information presented in table 1.1, determine the acquired charges of the bodies in each of the following pairs if
they are rubbed together.
🞇 a. wood and Styrofoam
🞇 wood: +
🞇 Styrofoam: -
- glass and leather glass: -
leather: +
- rubber and rubber rubber: none
rubber: none
- dry hand and PVC dry hand: +
PVC: -
Charging by Conduction
Charging by Conduction
🞇 During charging by conduction, both objects acquire the same type of charge.
🞇 If a negative object is used to charge a neutral object, then objects become charged negatively.
Charging by Induction.
🞇 A negatively charged balloon is placed near (but without physical contact) a neutral tin can. As a result, the positive
charges of the can move to the left, near the negatively charged balloon because of attraction. Meanwhile, the negative charges move to the right side of the tin can, away from the balloon because of repulsion. The excess negative charges at the right side of the tin can are known as induced charges.
Electric Dipoles
Electric Dipoles
🞇 When you bring a neutrally charged body A near a
strongly negative body B, its positive charges will be
drawn near B, and the negative charges will be pushed to the other side. This resulting condition polarizes the body and forms a dipole.
🞇 Polarization is the process wherein an electrically neutral body becomes polar by the rearrangement of its molecules. As the molecules realign or move, particles with similar charges group together and move toward the opposite ends of the body.
🞇 Hence, the body becomes positive at one end and negative on the other, making it a dipole.
🞇 Point dipoles refer to atoms bearing a positive side and a negative side. In such atoms, the electrons
converge or gather on one side and the protons on the other.
🞇 Molecular dipoles. This type of dipole involves a
molecule having a negatively charged side and a
positively charged side. The electronegative atoms of the molecule form the negative end of the molecule, and the electropositive ones are responsible for the positive end.
Another way to classify dipoles is whether they are permanent or temporary(instantaneous). An instantaneous or a temporary dipole is an atom or a molecule with most of its negative charges shifted only to one side as a result of their random movement.
MODULE 2
ELECTROSTATIC FORCE, ELECTRIC FIELD, AND ELECTRIC FLUX
At the end of this module, I can :
🞇 Define electrostatic force using Coulomb's Law.
🞇 Calculate the electrostatic force between two charges using Coulomb's Law.
🞇 Compute the Electric Field at a given distance from a given source charge.
🞇 a push or a pull pertains to force.
🞇 electric charges exert a force on each other as they interact.
🞇 The act of repelling implies pushing and the act of attracting suggests pulling.
Electrostatic Force
Electrostatic Force
🞇 The attraction and repulsion between electric charges comes from a force known as electrostatic force.
🞇 This force can be computed using
Coulomb’s law for electrostatics.
Example 1:
🞇 What is the electrostatic force of attraction between a -6.0 × 10^-6 C charge and a 4.0 × 10^-6 C charge if they are separated by a distance of 3
meters(m)?
0.02 N
Example 2:
🞇 Two identically charged one-peso coins are 1.5 m apart on a table.
What is the charge of one of the coins if each of them experiences a repulsive force of 2.0 N?
2.23 x 10^-5 C
TRY THIS!!!
🞇 Compute the force of attraction between a +1.60 x 10^-19 C and -2.09 x 10^-18C
charge if they are 4.0 x 10^-10 m apart.
0.00000001879 N
TRY THIS!!!
🞇 Calculate the repulsive force between a
-0.71783 electron charge and a -1.49 x
10^-8 C charge if a distance of 2.01 x 10^-20 m separates them.
TRY THIS!!!
🞇 Two ball bearings with same charges are 87.00787 inches apart
on the floor. What are their charges if they are attracted to each other with a force of 8.11 N
?
Electric Field and Its Representation
🞇 An electric field is a region of space where an electric charge experiences a force.
🞇 An electric field is a measurable effect generated
by any charged object.
🞇 An electric field coexists with every electrostatic charge; it associates with each point in space the electrostatic force experienced per unit of electric
charge, by an extremely small (or infinitesimal) test charge at that point.
Electric fields
🞇 predict the behavior of the charges present in any location in space.
🞇 Physicists compute the value of an electric field because of its direct relation with electrostatic force.
🞇 Mathematically, the electric field can be
computed using the equation:
🞇 E - is the electric field,
🞇 Q is the source charge
🞇 r is the distance from the source charge where the electric field is being measured.
🞇 The unit used to measure electric field is newton per coulomb (N/C).
Source and Test Charge
🞇 The charge Q which is producing the
electric field, is called a source charge
and the charge q, which tests the effect the charge of source charge, is called a test charge.
Source charge
🞇 is the charge from where the electric field comes from.
Test Charge
🞇 is a single charge whose behavior is measured or determined based on the
presence of external factors or stimuli. Its presence is arbitrary. For easier computation, a unit of 1 C is used.
Example :
🞇 Calculate the electric field that a test charge will experience on the following distances from the source charge of
+5.02 × 10-13 C.
🞇 a. Distance from source charge:
2.04 × 10-3 m.
Source charge will experience an electric field of
approximately 1 084.43 N/C
🞇 b. Distance from source charge:
1.55 × 10-12 m.
🞇 The source charge will experience an electric field
of approximately 1.88 × 1021 N/C.
Answer the following: Copy and Answer.
🞇 I. Calculate the electric field experienced by a test charge given the following conditions:
- Source charge: +3.1397 × 1015 e
Distance from source charge: 2.33 × 10-5 m
- Source charge: -4.33 × 10-12 C
Distance from source charge: 4.6 × 10-19 m
Answer the following: Copy and Answer.
🞇 I. Calculate the electric field experienced by a test charge given the following conditions:
- Source charge: +4.05 × 10-5 C
Distance from source charge: 3.23 × 10-7 m
- Source charge: -6.53 × 10-10 C
Distance from source charge: 3.7 × 10-15 m
- Source charge: +5.45 × 10-12 C
Distance from source charge: 6.8 × 10-17
- Source charge: -3.44 × 10-16 C
Distance from source charge: 4.43 × 10-12
Copy and Answer:
- What is the electrostatic force of attraction between a -6.0 × 10^-6 C charge and a 4.0 × 10^- 6 C charge if they are separated by a distance of 3 meters(m)?
- 2. Two ball bearings with opposite charges are
1.11 m apart on the floor. What are their charges if they are attracted to each other with a force of
5.11 N ?
- Calculate the repulsive force between a -2.23 x 10^-6 C charge and a -2.49 x 10^-5 C charge if a distance of 3.55 x 10^-21 m separates them.
Continuation….
🞇 II. Compute the electrostatic force experienced by
-4.43 × 10-18 C charge from the following electric
fields:
a.
E = 5 465 N/C
b.
E = 9.5 × 1015 N/C
E = 8.97 × 1024 N/C
Electric Field Lines
🞇 An electric field can be graphically represented using electric field lines. The
density or thickness of these lines is directly proportional to the strength of the electric field at any region in space.
🞇 If the field lines are close to each other, the electric field is stronger.
Electric field lines are drawn based on the charge being considered.
🞇 Positive charges have field lines drawn from them.
🞇 Negative charges have field lines drawn to them.
Electric field lines from the positive charge going to the negative charge.
Draw electric lines for the following charges.
- d.
+ + + + - -
b.
- -
c.
+ + -
Electric Potential
Work and Potential Energy
🞇 Energy is defined as the capacity to do work. A body with energy can do work,
whereas a body without it cannot do work.
Potential energy
🞇 is one of the many forms of energy.
🞇 It is the energy of a body due to its position and normally converted into useful work.
🞇 This is why it is known as the energy at rest.
Gravitational potential energy (GPE)
🞇 a type of potential energy, is due to a
body’s elevation from the ground.
🞇 A body placed higher above the ground can do greater work as it moves downward from its initial position.
Electric Potential
🞇 Electric potential is the amount of electric potential energy per unit charge. This is
equivalent to the amount of work needed to move a charge from one reference point to another.
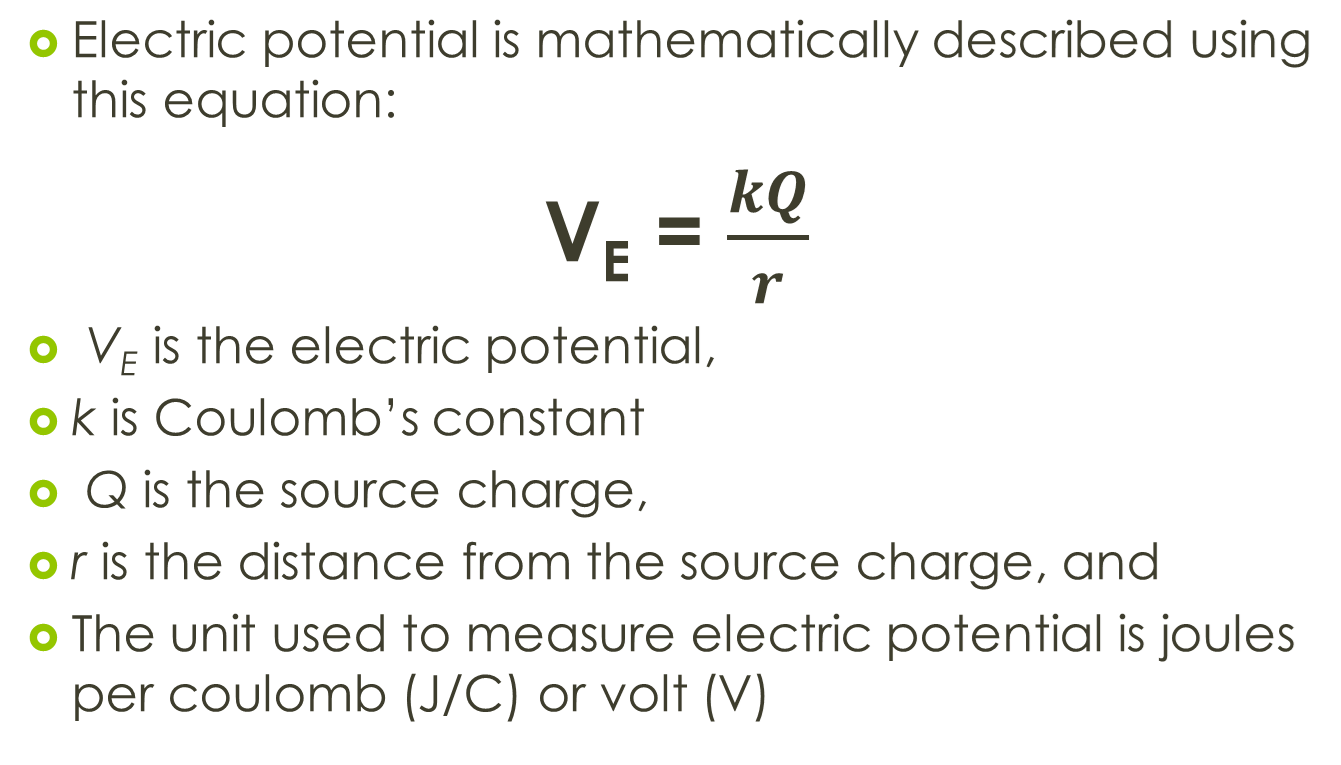 Electric Potential
Electric Potential
🞇
Example:
🞇 a. Compute the electric potential from a source charge of +5.02 × 10-13 C if a test charge will be placed 2.08 × 10-3 m from it.
🞇 The electric potential is approximately 2.17 V
🞇 b. How much is the electric potential from the
+4.02 × 10-15 C source charge if a test charge
will be placed 4.55 × 10-12 m from it.
🞇 The electric potential is approximately
7.94 × 108 V.
Compute the following electric potentials.
Source Charge | Distance from Source Charge | Electric Potential |
+4.31 x 103 C | 7.11x 10-12 m | |
-2.33 x 10-13 C | 6.45x 10-15 m |
🞇 Module 4: Voltage, Current,
and Resistance
Current: Flow of Electrical Charges
🞇 Normally, electrons move to any direction.
🞇 If this flow is regulated and made to move continuously in one direction, then the flow becomes an electric current.
![]() 🞇
🞇
Example 1:
🞇 a. Compute the current produced by a
+6.5 × 10-18 C charge flowing in 15 s.
🞇 The amount of current is approximately
4.33 × 10–19 A.
🞇 b. A steady current of 0.6 A flows through a wire. How much charge passes through the wire in 1 minute
(min)?
🞇 In 1 min, a charge of 36 C will pass through the wire.
Example:
🞇 How much current is flowing when 29.3 Coulombs of charges pass a point in 7.84 seconds?
🞇 The amount of current is approximately 3.
74A.
Solve for the following. Copy and
Answer.
- Compute the current produced by a
+4.40 × 10 14 e charge flowing in 21 min.
- How much current is flowing when 4.32 x 10-15 e of charges pass a point in 7.84 seconds?
- How much current is flowing when 33.3 Coulombs of charges pass a point in 2 hours?
- Calculate the total DC resistance of a 100 metre roll of 2.5mm2 copper wire if the resistivity of copper is 1.72 x 10-8 Ω metre.
- A 20 metre length of cable has a cross-sectional area of 1mm2 and a resistance of 5 ohms. Calculate the conductivity of the cable.
Resistance and Resistivity
🞇 An electrical conductor is any material that allows the free flow of electric current.
🞇 A conductor possesses characteristics that either enhance or limit the flow of current passing through it. The limitation to
current flow is referred to as resistance.
🞇 Resistance and electric current are inversely proportional. So a greater
amount of resistance on a conductor results in a lower amount of current passing through the conductor, whereas a lower resistance means less restrictions, allowing more current to flow through the conductor.
Electrical resistivity
🞇 is an intrinsic property of the material that describes how it resists the electric current flowing through it.
🞇 Higher electrical resistivity means higher overall resistance of the material, whereas lower resistivity indicates the material’s lower
resistance.
🞇 Consequently, current flow is reduced by an increase in the electrical resistivity of the material, whereas a decrease in the resistivity
allows more current to flow through the material.
electrical conductivity
🞇 an increase in the electrical conductivity of the material results in a lower resistance
offered by the material and a higher current flow through it. On the other hand, decreasing the electrical conductivity of the material increases its resistance and lowers the flow of current through it.
Factors or property of the material that affects the resistance and current flow
🞇 Temperature
🞇 Length of Conductor
🞇 Cross-sectional area of conductor
Temperature
🞇 If the conductor has a higher temperature, its resistance increases and the amount of current that can flow through it decreases.
🞇 On the other hand, if the conducting material has a lower temperature, the resistance decreases, thus allowing more current to flow through it
Length of the conductor
🞇 Longer conductors provide more resistance to the flow of current, which means less current can flow through it.
🞇 Shorter conductors provide less resistance, thus allowing more current to flow.
Cross-sectional area of the conductor
🞇 “Fat” conductors allow more charges to pass
through them, which means more current can flow.
More current flow also means lower resistance offered by the conductor.
🞇 “Thin” conductors, on the other hand, have limited space for current to flow through them, making resistance higher.
![]() 🞇
🞇
Sample problem1:
🞇 Compute the resistance of a conductor given a resistivity of 10.4 Ω-m, length of 4 m, and cross- sectional area of 7.85 × 10-3 m2.
🞇 The resistance of the conductor is approximately 5
299.36 Ω
- A conductor has a diameter of 2.59 mm. How many meters of this material are needed to yield a resistance of 1 Ω? The resistivity of the conductor is
1.77 × 10-8 Ω-m.
🞇 To obtain a resistance of 1 Ω, approximately
297.66 m of the conductor is needed.
Sample problem #2.
🞇 Solve for the resistance of a conductor given a resistivity of 15.3 Ω-m, length of 6 m, and cross-sectional area of 2.85 × 10-2 m2.
Sample Problem #3.
🞇 A copper rod is 3 m long and 4 mm in diameter. Compute its resistance if
the resistivity of the material is 1.76 × 10-8 Ω-m.
Electromotive Force
🞇 Electromotive force , it is the potential energy given to a unit charge to make it flow through a conductor or around a complete circuit.
🞇 The EMF acts like a charge pump that causes charges to flow through a circuit.
🞇 As a measurable quantity, EMF is measured using the unit volt (V)
Potential Difference
🞇 Similar to electromotive force is the potential difference (PD) across a circuit.
🞇 Potential difference is an actual consideration of the potentials in the circuit. The existence of PD also identifies the flow of
charges through the circuit. Without this difference, there will be no electric potential, thus making the flow of charges through the conductor impossible.
🞇 Both the EMF and PD are measured in terms of voltage (V).
Ohm’s Law
🞇 In 1827, Georg Simon Ohm discovered the relationship among voltage, current, and resistance.
🞇 He found out that electricity acts similarly to water in
a pipe. Through this observation, he was able to
summarize the relationship among EMF or voltage (V ), electric current (I ), and resistance (R) through the Ohm’s law.
🞇 In equation form, Ohm’s law is stated as follows:
V = IR
Example 3:
🞇 a. Using Ohm’s law, solve for the electric current of a conductor given a voltage of 25 V and a resistance of 10 Ω.
🞇 The current is approximately 2.5 A
🞇 b. An electric water heater uses 15 A of current when plugged to a 220-V outlet.
🞇 What is the resistance provided by the appliance?
🞇 The electric water heater provides a
resistance of approximately 14.67 Ω.
Electric Circuits
Electric Circuit.
🞇 The pathway for the current to move to and from the source and the appliance is called an electric circuit.
🞇 A functional circuit has to be “closed” or
must form a closed loop.
Circuits
🖉 In order for electricity to flow we need:
🖉 Power source
🖉 Closed circuit
🖉 Two type of circuits:
🖉 Series circuit
🖉 Parallel circuit
Closed circuits
🞇 Closed circuits allow the current to flow from the source of the current to the load where the current is needed.
Open circuits
🞇 an “open” circuit does not form a closed loop; the resulting circuit would then be nonfunctional.
🞇 Open circuits have gap(s) where current cannot flow.
🞇 Thus, electric current cannot be delivered
to the load where it is needed
The Series Circuit
Series Circuit
🖉 In a series circuit there is only one path for the electrons to flow.
🖉 In other words all the components are in series with each other
🖉 Because there is only one path each charge will go
![]() through each resistor
through each resistor
The Series Circuit
🞇 In a series circuit, all components are connected using a single pathway.
🞇 The current is the same for all the components along this circuit.
🞇 The total voltage is the sum of the individual voltages across the circuit.
🞇 the total resistance of the circuit is the sum of the individual resistances of each circuit load.
These relationships are summarized by the following formulas:
🞇 Vtotal = V1 + V2 + V3 + . . .+ Vn
🞇 Itotal = I1 = I2 = I3 = . . . = In
🞇 Rtotal = R1 + R2 + R3 + . . .+ R
Calculating Resistance in Series Circuits
![]() 🖉The rule for calculating Series Circuits is to…
🖉The rule for calculating Series Circuits is to…
🖉Add up the values of each individual in the series.
🖉R1 + R2 + R3…
🖉5 + 5 + 10
🖉20 Ω (ohms)
Calculating Resistance in Series Circuits
1
0
2
0
🖉Add up the values of each individual in the series.
🖉R1 + R2 + R3…
🖉10 + 20 + 10
🖉40 Ω (ohms)
The Parallel Circuit
🞇 Parallel circuits use branches to allow current to pass through more than one path, unlike in the series circuit.
🞇 Thus, the individual voltages in a parallel circuit are the same as the total voltage.
🞇 The current in each load is not the same
as the total current in the circuit.
Parallel Circuit
🖉 In a Parallel circuit there are multiple pathways for charge to flow.
🖉 Each device is placed on it’s own separate
branch
🖉 Current goes through each of the branches at the
![]()
![]() same time
same time
The Parallel Circuit
🞇 The total current is the sum of the individual currents across the resistors.
🞇 The reciprocal of the total resistance in this type of circuit is equal to the sum of
the reciprocals of the individual resistances.
🞇 Always remember that the total resistance is always less than the individual resistances
The following formulas for a parallel circuit:
🞇 Vtotal= V1 = V2 = V3 = . . . = Vn
🞇 Itotal = I1 + I2 + I3 + . . .+ In
Calculating Resistance in Parallel Circuits
🖉 The rule for calculating
Series Circuits is to…
🖉 Add up the values of each
individual in the circuit.
🖉 1 1 1 1
-- = -- + -- + -- Rt R1 R2 R3
🖉 1 1 1 1
-- = -- + -- + --
Rt 4 4 2
🖉 1
-- = .25 + .25 + .5
Rt
🖉 1
-- = 1
Rt
🖉 1
-- = 1
Rt
🖉 1
-- = 1
Rt
🖉 1
-- = Rt
1
🖉 Rt = 1 Ω (ohms)
2
0
Calculating Resistance in Parallel Circuit
🞇 The rule for calculating
Series Circuits is to…
🞇 Add up the values of each
individual in the circuit.
🞇 1 1 1 1
-- = -- + -- + -- Rt R1 R2 R3
10
00
🞇 1 1 1 1
-- = -- + -- + --
Rt 100 200 1000
🞇 1
2
0
-- = .01 + .005 + .001 Rt | 1 | 2 | ||
🞇 | 1 | 0 | 0 | |
🞇 | -- = .016 Rt 1 -- = .016 Rt | 0 | 0 |
- 1
-- = Rt
.016
- Rt = 62.5 Ω
(ohms)
 Knowt
Knowt
