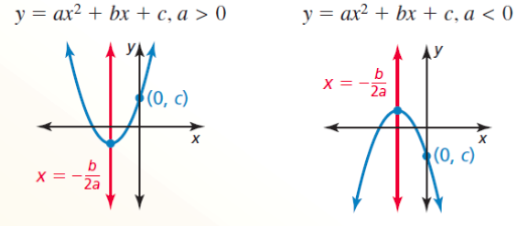Lesson 1: Transformations of Quadratic Functions

• Quadratic Functions

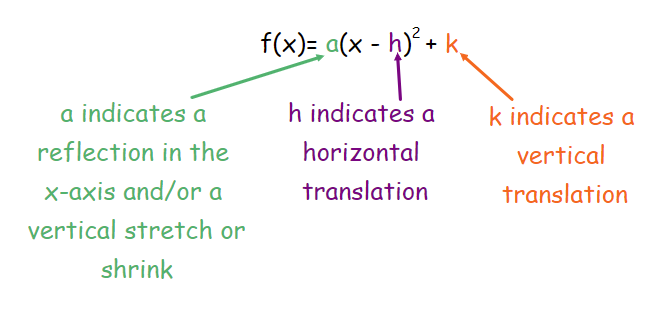
  • A quadratic function is a function that can be written in the form f(x) = a(x - h)2 + k, where a ≠ 0

  • The U-shaped graph of a quadratic function is called a parabola

• Translations

  • Horizontal Translation

    • f(x) = x2

    • f(x - h) = (x - h)2

    • 

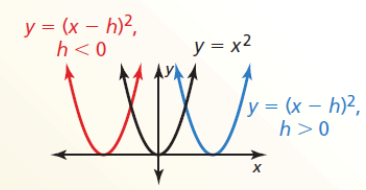
    • Shifts left when h < 0

    • Shifts right when h > 0

  • Vertical Translation

    • f(x) = x2

    • f(x) + k = x2 + k

    • 

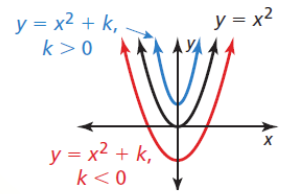
    • Shifts down when k < 0

    • Shifts up when k > 0

• Reflections

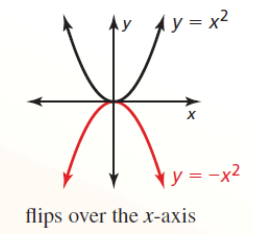
  • Reflection in the x-axis

    • f(x) = x2

    • -f(x) = -(x2) = -x2

    • 

  • Reflection in the y-axis

    • f(x) = x2

    • f(-x) = (-x)2 = x2

    • 

• Stretches and Shrinks

  • Horizontal Stretches and Shrinks

    • f(x) = x2

    • f(ax) = (ax)2

    • 

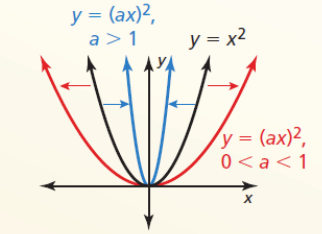
    • Horizontal stretch (away from y-axis) when 0 < a < 1

    • Horizontal shrink (towards y-axis) when a > 1

  • Vertical Stretches and Shrinks

    • f(x) = x2

    • a * f(x) = ax2

    • 

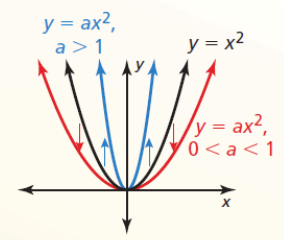
    • Vertical stretch (away from x-axis) when a > 1

    • Vertical shrink (towards x-axis) when 0 < a < 1

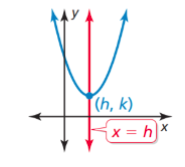
• Vertex Form

  • The lowest part on a parabola that opens up or the highest point on a parabola that opens down is called the vertex

  • The vertex form of a quadratic function is f(x) = a(x - h)2 + k, where a ≠ 0 and the vertex is (h, k)

  • 

Lesson 2: Characteristics of Quadratic Functions

• Quadratic Function Forms

  • Axis of Symmetry - a line that divides a parabola into mirror images and passes through the vertex; the axis of symmetry is the vertical line x = h or x = -b/2a

  • 

  • Standard Form - a quadratic function written in the form f(x) = ax2 + bx + c

  • Intercept Form - a quadratic function in the form f(x) = a(x - p)(x - q)

• Properties of the Standard Form

  • 

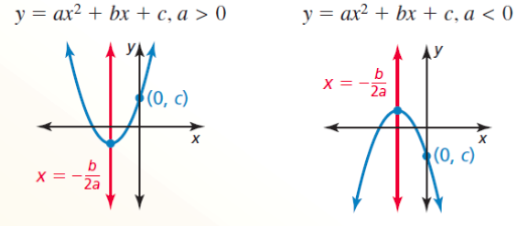
  • The parabola opens up when a > 0 and opens down when a < 0

  • The graph is narrower than the graph of f(x) = x2 when |a| > 1 and wider when |a| < 1

  • The axis of symmetry is x = -b/2a and the vertex is (-b/2a, f(-b/2a))

  • The y-intercept is c; the point (0, c) is on the parabola

• Minimum and Maximum Values

  • For the quadratic function f(x) = ax2 + bx + c, the y-coordinate of the vertex is the minimum value of the function when a > 0 and the maximum value when a < 0

  • 

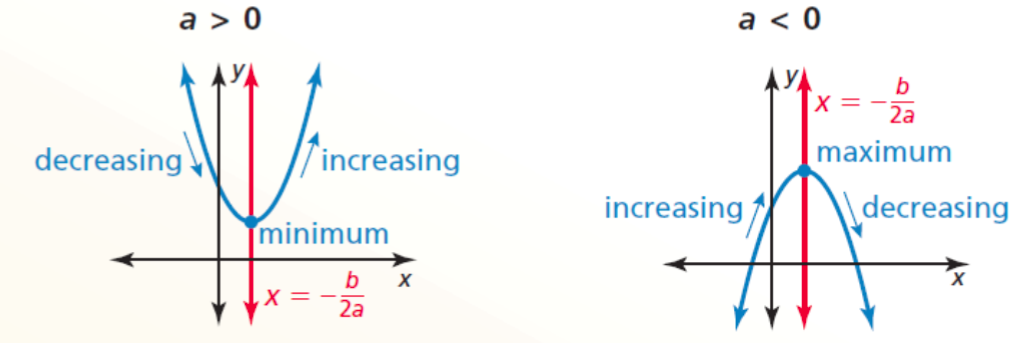
  • a > 0

    • Minimum value: f(-b/2a)

    • Domain: all real numbers

    • Range: y ≥ f(-b/2a)

    • Decreasing to the left of x = -b/2a

    • Increasing to the right of x = -b/2a

  • a < 0

    • Maximum value: f(-b/2a)

    • Domain: all real numbers

    • Range: y ≤ f(-b/2a)

    • Increasing to the left of x = -b/2a

    • Decreasing to the right of x = -b/2a

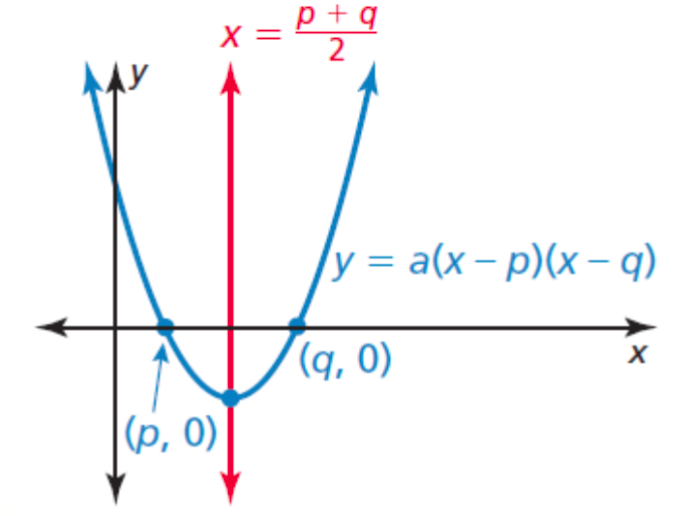
• Properties of the Intercept Form

  • Because f(p) = 0 and f(q) = 0, p and q are the x-intercepts of the graph of the function

  • The axis of symmetry is halfway between (p, 0) and (q, 0); x = (p + q)/2

  • The parabola opens up when a > 0 and opens down when a < 0

  • 

Lesson 3: Modeling with Quadratic Equations

• Writing Quadratic Equations

  • Given a point and the vertex (h, k), use vertex form: y = a(x - h)2 + k

  • Given a point and x-intercepts p and q, use intercept form y = a(x - p)(x - q)

  • Given three points, write and solve a system of three equations in three variables