Chapter 5: Forces
5.1-Contact and Non-Contact Forces
Vectors have magnitude and direction
Force is a vector quantity-vector quantities have a magnitude and a direction
Lots of physical quantities are vector quantities: force, velocity, displacement, acceleration, momentum,etc
Some physical quantities only have magnitude and no direction, these are called scalar quantities:speed, distance, mass, temperature, time, etc
Vectors are usually represented by an arrow-the length of the arrow shows the magnitude and the direction of the arrow shows the direction of the quantity
Velocity is a vector, but speed is a scalar quantity.
Both bikes are travelling at the same speed, but they have different velocities because they are travelling in different directions
Forces can be contact or non-contact
A force is a push or a pull on an object that is caused by it interacting with something
All forces are either contact or non-contact forces
When two objects have to be touching for a force to act, that force is called a contact force
If the objects do not need to be touching for the force to act, the force is a non-contact force
When two objects interact, there is a force produced on both objects.
An interaction pair is a pair of forces that are equal and opposite and act on two interacting objects(basically Newtons third law)
Examples:
The sun and the earth are attracted to each other by the gravitational force.
This is a non-contact force.
An equal but opposite force of attraction is felt by both the sun and earth.
A chair exerts a force on the ground, whilst the ground pushes back at the chair with the same force.
Equal but opposite forces are felt by both the chair and the ground
5.2-Weight, Mass and Gravity
Gravitational force is the force of attraction between masses
Gravity attracts all masses, but you only notice it when one of the masses is really big, like a planet
This has two important effects:
On the surface of a planet, it makes all things fall towards the ground
It gives everything a weight
Weight and mass are not the same
Mass is just the amount of stuff in an object.
For any given object this will have the same value anywhere in the universe
Weight is the force acting on an object due to gravity, the pull of gravitational force on the object.
Close to Earth, this force is caused by the gravitational field around the Earth
Gravitational field strength varies with location.
It’s stronger the closer you are to the mass causing the field, and stronger for larger masses
The weight of an object depends on the strength of the gravitational field at the location of the object.
This means that the weight of an object changes with its location
For example, an object has the same mass whether it’s on Earth or on the Moon-but its weight will be different. A 1kg mass will weigh less on the Moon, because the gravitational field strength is less
Weight is a force measured in newton
Weight is measured using a calibrated spring balance
Mass is not a force, and is measured in kilograms with a mass balance
Mass and weight are directly proportional
You can calculate the weight of an object if you know its mass and the strength of the gravitational field:
Weight(N)=Mass(kg) x Gravitational Field Strength(N/kg)
For Earth, g=9.8N/kg and for the moon its around 1.6N/kg.
Increasing the mass of an object increases its weight.
If you double the mass, the weight doubles meaning they are directly proportional(W=M)
5.3-Resultant Forces and Work Done
Free body diagrams show all the forces acting on an object
Size of the line shows the relative magnitudes
Need to be able to describe all forces happening on something
A resultant force is the overall force on a point or object
In most situations there are at least 2 forces acting on an object
If you have a number of forces acting at a single point, you can replace them with a single force
This single force is called the resultant force
If the forces all act along the same line, the overall effects is found by adding those going in the same direction and subtracting any going in the opposite direction
If a resultant force moves an object, work is done
To make something move a force must be applied
The thing applying the force needs a source of energy
The force does work to move the object and energy is transferred from one store to another
Whether energy is transferred usefully or is wasted you can still say that work is done
You can find out how much work has been done using: W=Fs
One joule of work is done when a force of one newton causes an object to move a distance of one metre. You need to be able to convert joules to newton metres:1J=1Nm
5.4-Calculating Forces
Use scale drawings to find resultant forces
Draw all the forces acting on an object, to scale, ‘tip-to-tail’
Then draw a straight line from the start of the first force to the end of the last force-this is the resultant force
Measure the length of the resultant force on the diagram to find the magnitude and the angle to find the direction of the force
An object is in equilibrium if the forces on it are balanced
If all of the forces acting on an object combine to give a resultant force of zero, the object is in equilibrium
On a scale diagram, this means that the tip of the last force you draw should end where the tail of the first force you draw begins
You might be given forces acting on an object and told to find a missing force, given that the object is in equilibrium.
To do this, draw out the forces you do know, join the end of the last force to the start of the first force.
This line is the missing force so you can measure its size and direction
You can split a force into components
Not all forces act horizontally or vertically-some act at awkward angles
To make these easier to deal with, they can be split into two components at right angles to each other
Acting together, these components have the same effect as the single force
You can resolve a force by drawing it on a scale grid. Draw the force to scale, and then add it horizontal and vertical components along the grid lines.
Then you can just measure them
5.5-Forces and Elasticity
Stretching, compressing or bending transfers energy
When you apply a force to an object you may cause it to stretch, compress or bend
To do this, you need more than one force acting on the object, otherwise the object would simply move in the direction of the applied force instead of changing shape
An object has been elastically deformed if it can go back to its original shape and length after the force has been removed
Objects than can be elastically deformed are called elastic objects
An object has been inelastically deformed if it doesn’t return to its original shape and length after the force has been removed
Work is done when a force stretches or compresses an object and causes energy to be transferred to the elastic potential energy store of the object.
If it is elastically deformed.
ALL this energy is transferred to the object’s elastic potential energy store
Extension is directly proportional to force
If a spring is supported at the top and then a weight is attached to the bottom, it stretches
The extension of a stretched spring is directly proportional to the load of force applied
F=ke
The spring constant depends on the material that you are stretching-a stiffer spring has a greater spring constant
The equation also works for compression
But this stops working when the force is great enough
There’s a limit to the amount of force you can apply to an object for the extension to keep on increasing proportionally
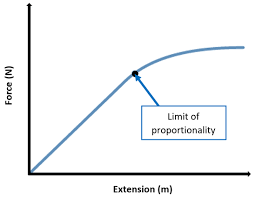
The graph shows force against extension for an elastic object
There is a maximum force above which the graph curves, showing that extension is no longer proportional to force.
This is known as limit of proportionality and is shown on the graph at the point marked P
You might see graphs with these axis the other way round-extension force graphs.
The graph still starts with a straight part, but starts to curve upwards once you go past the limit of proportionality, instead of downwards
5.6-Investigating Springs
You can investigate the link between force and extension
Set up the apparatus and make sure you can extra masses, then measure the mass of each and calculate the weight using W=mg
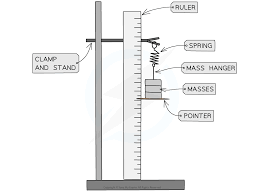
Measure the natural length of the spring with a millimetre ruler clamped to the stand.
Make sure you take the reading at eye level and add a marker to the bottom of the spring to make the reading more accurate
Add a mass to the spring and allow it to come to rest.
Record the mass and measure the new length of the spring.
The extension is the change in length
Repeat this process until you have enough measurements, no fewer than 6
Plot a force-extension graph of your results.
It will only start to curve if you exceed the limit of proportionality.
You can work out energy stored for linear relationships
As long as a spring is not stretched past its limit of proportionality, the work done in stretching a spring can be found using: Ee=1/2ke2
For elastic deformation, this formula can be used to calculate the energy stored in a spring’s elastic potential energy store. It’s also the energy transferred to the spring as it’s deformed
5.7-Moments
A moment is the turning effect of a force
A force, or several forces, can cause an object to rotate.
The turning effect of a force is called its moment.
The size of the moment of the force is given by:
M=Fd, Moment of a force=force x distance
The force on the spanner causes a turning effect or moment on the nut.
A larger force or a longer distance would mean a larger moment
To get the maximum moment you need to push at right angles to the spanner.
Pushing at any other angle means a smaller distance, and so a smaller moment
Levers make it easier for us to do work
Levers increase the distance from the pivot at which the force is applied. Since M=Fd this means less force is needed to get the same moment.
This means levers make it easier to do work
Gears transmit rotational effects
Gears are circular discs with ‘teeth’ around their edges
Their teeth interlock so that turning one causes another to turn, in the opposite direction
They are used to transmit the rotational effect of a force from one place to another
Different sized gears can be used to change the moment of the force.
A force transmitted to a larger gear will cause a bigger moment, as the distance to pivot is greater
The larger gear will turn slower than the smaller gear
5.8-Fluid Pressure
Pressure is the force per unit area
Fluids are substances than can flow because their particles are able to move around
As these particles move around, they collide with surfaces and other particles
Particles are light, but they still have a mass and exert a force on the object they collide with.
Pressure is force per unit area, so this means the particles exert a pressure
The pressure of a fluid means a force is exerted normal, at right angles, to any surface in contact with the fluid
You can calculate the pressure at the surface of a fluid by using:
p=F/A
Pressure in a liquid depends on depth and density
Density is a measure of the compactness of a substance. For a given liquid, the density is uniform and it doesn’t vary with shape or size.
The density of a gas can vary though
The more dense a given liquid is, the more particles it has in a certain space.
This means there are more particles that are able to collide so the pressure is higher
As the depth of the liquid increases, the number of particles above that point increases.
The weight of these particles adds to the pressure felt at that point, so liquid pressure increases with depth
You can calculate the pressure at a certain depth due to the column of liquid above using:
P=HPG, pressure x height of column in liquid x density of liquid x gravitational field strength
You could write the answer in standard form so its easier to read
5.9-Upthrust and Atmospheric Pressure
Objects in fluids experience upthrust
When an object is submerged in a fluid, the pressure of the fluid exerts a force on it from every direction
Pressure increases with depth, so the force exerted on the bottom of the object is larger than the force acting on the top of the object
This causes a resultant force upwards, known as upthrust
The upthrust is equal to the weight of fluid that has been displaced by the object
An object floats if its weight=upthrust
If the upthrust on an object is equal to the object’s weight, then the forces balance and the object floats
If an object’s weight is more than the upthrust, the object sinks
Whether or not an object will float depends on its density
An object that is less dense than the fluid it is placed in weighs less than the equivalent volume of fluid.
This means it displaces a volume of fluid that is equal to its weight before it is completely submerged
At this point, the object’s weight is equal to the upthrust, so the object floats
An object that is denser than the fluid it is placed in is unable to displace enough fluid to equal its weight.
This means that its weight is always larger than the upthrust, so it sinks
Atmospheric pressure decreases with height
The atmosphere is a layer of air that surrounds Earth.
It is thin compared to the size of the Earth
Atmospheric pressure is created on a surface by air molecules colliding with the surface
As the altitude increases, atmospheric pressure decreases
This is because as the altitude increases, the atmosphere gets less dense, so there are fewer air molecules that are able to collide with the surface
There are also fewer air molecules above a surface as the height increases.
This means that the weight of the air above it, which contributes to atmospheric pressure, decreases with altitude
5.10-Distance, Displacement, Speed and Velocity
Distance is Scalar, Displacement is a Vector
Distance is just how far an object has moved. It’s a scalar quantity, so it doesn’t involve direction.
Displacement is a vector quantity.
It measures the distance and direction in a straight line from an object’s starting point to its finishing point.
The direction could be relative to a point, then 5m south, your displacement is 0m but the distance travelled is 10m
If you walk 5m north, then 5m south, your displacement is 0m but the distance travelled is 10m
Speed and velocity are both how fast you’re going
Speed and velocity both measure how fast you’re going, but speed is a scalar and velocity is a vector
Speed is just how fast you’re going with no regard to the direction.
Velocity is speed in a given direction
This means you can have objects travelling at a constant speed with a changing velocity.
This happens when the object is changing direction whilst staying at the same speed.
An object moving in a circle at a constant speed has a constantly changing velocity, as the direction is always changing
If you want to measure the speed of an object that’s moving with a constant speed, you should time how long it tales the object to travel a certain distance.
You can then calculate the object’s speed from your measurements using this formula:
distance travelled= speed x time
Objects rarely travel at a constant speed. For these cases, the formula above gives the average speed during that time
You need to know some typical everyday speeds
Whilst every person, train, car is different, there is usually a typical speed that each object travels at.
Remember these typical speeds for everyday objects
Lots of different things can affect the speed something travels at.
For example, the speed at which a person can walk, run or cycle depends on their fitness, their age, the distance travelled and the terrain as well as many other factors
It’s not only the speed of objects that varies.
The speed of sound changes depending on what the sound waves are travelling through, and the speed of wind is affected by many factors
Wind speed can be affected by things like temperature, atmospheric pressure and if there are any large buildings or structures nearby
5.11-Acceleration
Acceleration is how quickly you’re speeding up
Acceleration is definitely not the same as velocity or speed
Acceleration is the change in velocity in a certain amount of time
You can find the average acceleration of an object using:
Acceleration=Change in velocity / time
Deceleration is just negative acceleration
You need to be able to estimate acceleration
You might have to estimate the acceleration of an object.
To do this, you need the typical speeds from the previous page:
First, give a sensible speed for the car to be travelling at
Next, give it a time
Put these numbers into the acceleration equation
The question asked for the deceleration so you can lose the minus sign
Uniform acceleration means a constant acceleration
Constant acceleration is sometimes called uniform acceleration
Acceleration due to gravity is uniform for objects in free fall.
It’s roughly equal to 9.8m/s2 near the Earth’s surface and has the same value as gravitational field strength
You can use this equation for uniform acceleration
v2-u2=2as, Final velocity-initial velocity = 2 acceleration x distance
5.12-Distance-Time and Velocity-Time Graphs
You can show journeys on distance-time graphs
If an object moves in a straight line, its distance travelled can be plotted on a distance-time graph
Gradient=speed. This is because speed = distance / time
Flat sections are where it’s stationary, it’s stopped
Straight uphill sections mean it is travelling at a steady speed
Curves represent acceleration or deceleration
A steepening curve means it’s speeding up(increasing gradient)
A levelling off curve means it’s slowing down
If the object is changing speed you can find its speed at a point by finding the gradient of the tangent to the curve at that point
You can also show them on a velocity-time graph
How an object’s velocity changes as it travels can be plotted on a velocity-time graph
Gradient = acceleration, since acceleration is change in velocity / time
Flat sections represents travelling at a steady speed
The steeper the graph, the greater the acceleration or deceleration
Uphill sections are acceleration
Downhill sections are deceleration
A curve means changing acceleration
The area under any section of the graph is equal to the distance travelled in that time interval
If the section under the graph is irregular, it’s easier to find the area by counting the squares under the line and multiplying the number by the value of one square
5.13-Terminal Velocity
Friction is always there to slow things down
If an object has no forces propelling it along it will always slow down and stop because of friction
Friction always acts in the opposite direction to movement
To travel at a steady speed, the driving force needs to balance the frictional forces
You get friction between two surfaces in contact, or when an object passes through a fluid
Drag increases as speed increases
Drag is the resistance you get in a fluid. Air resistance is a type of drag.
The most important factor by far in reducing drag is keeping the shape of the object streamlined.
This is where the object is designed to allow fluid to flow easily across it, reducing drag.
Parachutes work in the opposite way-they want as much drag as they can get
Frictional forces from fluids always increase with speed.
A car has much more friction to work against when travelling at 70mph compared to 30mph.
So at 70mph the engine has to work much harder just to maintain a steady speed.
Objects falling through fluids reach a terminal velocity
When a falling object first sets off, the force of gravity is much more than the frictional force slowing it down, so it accelerates.
As the speed increases the friction builds up.
This gradually reduces the acceleration until eventually the frictional force is equal to the accelerating force.
It will have reached its maximum speed or terminal velocity and will fall at a steady speed
Terminal velocity depends on shape and area
The accelerating force acting on all falling objects is gravity and it would make them all fall at the same rate if it wasn’t for air resistance.
This means that on the Moon, where there’s no air, hamsters and feathers dropped simultaneously will hit the ground together.
However, on Earth, air resistance causes things to fall at different speed, and the terminal velocity of any object is determined by its drag in comparison to its weight.
The frictional force depends on its shape and area.
The most important example is the human skydiver.
Without his parachute open he has quite a small area and a force of W=mg pulling him down.
5.14-Newton’s First and Second Laws
A force is needed to change motion
Newton’s first law says that a resultant force is needed to make something start moving, speed up or slow down
If the resultant force on a stationary object is zero, the object will remain stationary.
If the resultant force on a moving object is zero, it’ll just carry on moving at the same velocity
So when a train or car or bus or anything else is moving at a constant velocity, the resistive and driving forces on it must all be balanced.
The velocity will only change if there’s a non-zero resultant force actin on the object.
A non-zero resultant force will always produce acceleration in the direction of the force
This acceleration can take five different forms: starting, stopping, speeding up, slowing down and changing direction
On a free diagram, the arrows will be unequal
Acceleration is proportional to the resultant force
The larger the resultant force acting on an object, the more the object accelerates-the force and the acceleration are directly proportional.
You can write this as F=a
Acceleration is also inversely proportional to the mass of the object-so an object with a larger mass will accelerate less than one with a smaller mass
There’s a formula that describes Newton’s second law:
F=ma, Resultant force, mass x acceleration
You can use this to get an idea of the forces involved in everyday transport, large forces are needed to produce large acceleration
5.15-Inertia and Newton’s Third Law
Inertia is the tendency for motion to remain unchanged
Until acted upon by a resultant force, objects at rest stay at rest and objects moving at a steady speed will stay moving at that speed.
This tendency to continue in the same state of motion is called inertia
An object’s inertial mass measures how difficult it is to change the velocity of an object
Inertial mass can be found using Newton’s Second Law of F=ma.
Rearranging this gives m=F/a, so inertial mass is just the ratio of force over acceleration
Newton’s third law: equal and opposite forces act on interacting objects
When two objects interact, the forces they exert on each other are equal and opposite
If you push something, say a shopping trolley, the trolley will push back against you, just as hard
And as soon as you stop pushing, so does the trolley
The important thing to remember is that the two forces are acting on different objects
It can be easy to get confused with Newton’s third law when an object is in equilibrium.
A book resting on the ground is in equilibrium.
The weight of the book is equal to the normal contact force.
But this is NOT Newton’s third law because the two forces are different types, and both acting on the book
5.16-Investigating Motion
You can investigate the link between force and extension
Set up the apparatus and make sure you can extra masses, then measure the mass of each and calculate the weight using W=mg

Measure the natural length of the spring with a millimetre ruler clamped to the stand.
Make sure you take the reading at eye level and add a marker to the bottom of the spring to make the reading more accurate
Add a mass to the spring and allow it to come to rest.
Record the mass and measure the new length of the spring.
The extension is the change in length
Repeat this process until you have enough measurements, no fewer than 6
Plot a force-extension graph of your results.
It will only start to curve if you exceed the limit of proportionality.
You can work out energy stored for linear relationships
As long as a spring is not stretched past its limit of proportionality, the work done in stretching a spring can be found using: Ee=1/2ke2
For elastic deformation, this formula can be sued to calculate the energy stored in a spring’s elastic potential energy store. It’s also the energy transferred to the spring as it’s deformed
5.17-Stopping Distances
Many factors affect your total stopping distance
In an emergency, a driver may perform an emergency stop.
This is where maximum force is applied by the brakes in order to stop the car in the shortest possible distance.
The longer it takes to perform an emergency stop, the higher the risk of crashing into whatever’s in front.
The distance it takes to stop a car in an emergency is found by:
Stopping distance = Thinking Distance + Braking Distance
Where the THINKING DISTANCE is how fast the car travels during the driver’s reaction time.
And the BRAKING DISTANCE is the distance taken to stop under the braking force.
Typical car barking distances are: 14m at 30mph, 55mm at 60mph and 75m at 70mph
Thinking distance is affected by:
Your SPEED-the faster you’re going the further you’ll travel during the time you take to react
Your REACTION TIME-the longer your reaction time the longer your thinking distance
Braking distance is affected by:
Your Speed-for a given braking force, the faster a vehicle travels, the longer it takes to stop
The WEATHER or ROAD SURFACE-if it is wet or icy, or there are leaves or oil on the road, there is less grip, and so less friction, between a vehicles tyres an the road, which can cause tyres to skid
The CONDITION of your TYRES-if the tyres of a vehicle are bald, then they cannot get rid of water in wet conditions. This leads to them skidding on top of the water.
How good your BRAKES are-if brakes are worn or faulty, they won’t be able to apply as much force as well-maintained brakes, which could be dangerous when you need to brake hard
You need to be able to describe the factors affecting stopping distance and how this affects safety=especially in an emergency
Speed limits are really important because speed affects the stopping distance
Braking relies on friction between the brakes and wheels
When the brake pedal is pushed, this causes brake pads to be pressed onto the wheels.
This contact causes friction, which causes work to be done,
The work done between the brakes and the wheels transfers energy from the kinetic energy stores of the wheels to the thermal energy stores of the brakes.
The brakes increase in temperature
The faster a vehicle is going, the more energy it has in its kinetic stores, s the more work needs to done to stop it.
This means that a greater braking force is needed to make it stop within a certain distance
A larger braking force means a larger deceleration.
Very large decelerations can be dangerous because they may cause brakes to overheat or could cause the vehicle to skid
You can estimate the forces involved in accelerations of vehicles using typical values:
Assume the deceleration is uniform, and rearrange v2-u2-2as to find the deceleration
Then use F=ma with m=1000kg
5.18-Reaction Times
Reaction times vary from person to person
Everyone’s reaction time is different, but a typical reaction time is between 0.2 and 0.9 s. This can be affected by tiredness, drugs or alcohol. Distractions can also affect your ability to react
You can measure reaction times with the ruler drop test
You can do simple experiments to investigate your reaction time, but as reaction time are so short, you haven’t got a chance of measuring one with a stopwatch
One way of measuring reaction times is to use a computer-based test. Another is the ruler drop test:
Sit with your arm resting on the edge of a table.
Get someone else to hold a ruler so it hangs between your thumb and forefinger, lined up with zero.
You may need a third person to be at eye level with the ruler to check it’s lined up
Without giving any warning, the person holding the ruler should drop it.
Close your thumb and finger to try to catch the ruler as quickly as possible
The measurement on the ruler at the point where it is caught is how far the ruler dropped in the time it takes you to react
The longer the distance, the longer the reaction time
You can calculate how long the ruler falls for because acceleration due to gravity is constant, roughly 9.8m/s
It’s pretty hard to de this experiment accurately
Make sure it’s a fair test-use the same ruler and same person
You could try to investigate some factors affecting reaction time
Do lots of repeats and calculate mean reaction time with distractions, which you can compare to the mean reaction time without distractions
5.19-More on Stopping Distances
Leave enough space to stop
The figure below for typical stopping distance are from the Highway Code

To avoid an accident, drivers need to leave enough space between their car and the one in front so that if they had to stop suddenly they would have time to do so safely.
‘Enough space’ means the stopping distance for whatever speed they’re going at
Speed limits are really important because speed affects the stopping distance so much
Speed affects braking distance more than thinking distance
As a car speeds up, the thinking distance increases at the same rate as speed.
The graph is linear
This is because the thinking time stays pretty high constant-but the higher the speed, the more distance you cover in that same time
Braking distance, however, increases faster the more you speed up,.
The work done to stop the car is equal to the energy in the car’s kinetic energy store.
So as speed doubles, the kinetic energy increase 4-fold, and so the work done to stop the car increases 4-fold. Since W=Fs and the braking force is constant the braking distance increases 4-fold.
Stopping distance is a combination of these two distances so the graph of speed against stopping distance for a car looks like this:
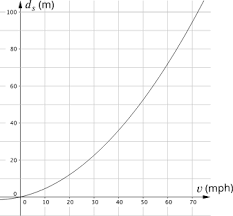
You need to be able to interpret graphs like this for a range of vehicles-they’re all a similar shape
5.20-Momentum
Momentum=Mass x Velocity
Momentum is mainly about how much ‘oomph’ an object has. It’s a property that all moving objects have
The greater the mass of an object, or the greater its velocity, the more momentum the object has
You can work out the momentum of an object using:
p=mv momentum = mass x velocity
Momentum before = momentum after
In a closed system, the total momentum before an event is the same as after the event.
This is called conservation of momentum
In snooker, balls of the same size and mass collide with each other.
Each collision is an events where the momentum of each ball changes, but the overall momentum stays the same
Before: The red ball is stationary, so it has zero momentum.
The white ball is moving with a velocity so has a momentum of p=mv
After: The white ball hits the red ball, causing it to move.
The red ball now has momentum.
The white continues moving, but at a much smaller velocity.
The combined momentum of the red and white ball is equal to the original momentum of the white ball, mv
If the momentum before an event is zero, then the momentum after is zero
5.21-Changes in Momentum
You can use conservation of momentum to calculate velocities or masses
Momentum is conserved in a closed system.
You can use this to help you calculate things like velocity or mass of objects in an events
Example question:
Calculate the momentum of the pellet
The momentum before the gun is fired is zero.
This is equal to the total momentum after the collision
The momentum of the gun is 1.5 x v
Rearrange the equation to find the velocity of the gun.
The minus sign shows the gun is travelling in the opposite direction to the bullet
Forces cause a change in momentum
You know that when a non-zero resultant force acts on a moving object it causes its velocity to change. This means that there is a change in momentum
You also know that F=ma and that a= change in velocity / change in time
So F=m x v-u/t which can also be written as: F = mchangeinv/changeint
The force causing the change is equal to the rate of change of momentum
A larger force means a faster change of momentum
Likewise, if someone’s momentum changes very quickly the forces on the body will be very large, and more likely to causes injury
This is why cars are designed to slow people down over a longer time when they have a crash- the longer it takes for a change in momentum, the smaller the rate if change in momentum, and so the smaller the force.
Smaller forces means the injuries are likely to be less severe
Cars have many safety features, such as:
Crumple zones crumple on impact, increasing the time taken for the car to stop
Seat belts stretch slightly, increasing the time taken for the wearer to stop
Air bags inflate
 Knowt
Knowt
