USNCO

Iodimetry(Direct Iodometry): A reducing analyte is titrated directly with iodine (I2) to produce iodide (I-)

/

H2S + I2 —> 2H+ + S + 2I-

Iodometry(Indirect): An oxidizing analyte is added to excess I- to produce I2, which is then titrated with standard S2O3 2- solution

Cr2O7 2- + 14 H+ + GI- —> 2Cr3+ (green) + 3I2 + 7H2O

I2 + 2S2O3 2- —> 2I-(colorless) + S4O6 2-

Endpoint (Starch indicator): Blue (starch + I3-) to colorless (no I3-)

I2(s) is only slightly soluble in water but adding iodide, I-< produces the triiodide ion (I3-) in solution, thus KI is almost always added.

I2(s) + I-(aq) —> I3-

• Common compounds titrated - Ascorbic acid

Question:

• Test 8, Q 10;

• Key Points:

  • The reaction involves the reduction of dichromate ions in an acidic solution.

  • The blue color indicates the presence of triiodide ions (I3-), formed when iodine (I2) interacts with starch.

  • The disappearance of the blue color signifies the endpoint of the titration, indicating that all iodine has reacted with thiosulfate.

Solubility in water —> should be polar, as polar solutes tend to dissolve well in polar solvents due to similar intermolecular forces.

• Forming more hydrogen bonds = increasing force

• Test 8, Q 14 —> A has more hydrogen bonds than D, which leads to a higher solubility in water compared to the other options.

Phase diagram (water, sulfur)

• Sulfur phase diagram: 2 types of solids; rhombic and monoclinic

  • Rhombic: Stable form at room temperature, characterized by a distinct crystal structure.

  • Monoclinic: More stable at higher temperatures, exhibiting different physical properties and solubility behavior.

  • Test 8,Q 17: C incorrect because at 119 C, the monoclinic sulfur can exist on the line

    

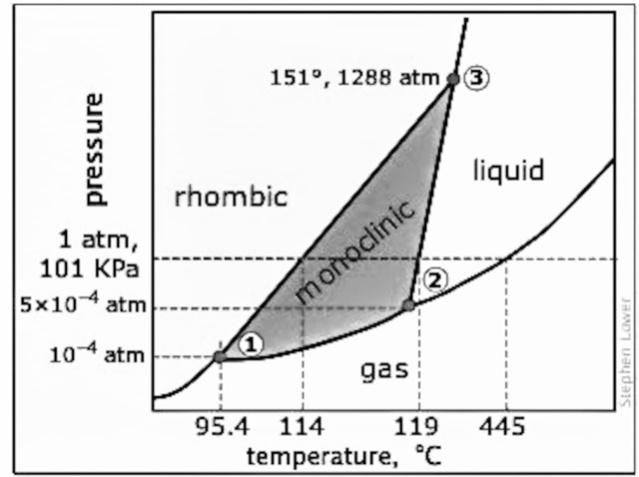
• Equilibrium means that 2 phases will exist simultaneously (the boundary between 2 phases)

• Density —> slope is positive, then the density of the solid is greater than that of the liquid or monoclinic sulfur.

Descriptive Lab

• Refluxing

• Types of Chromatography

States of Matter

Freezing point depression constant

• Molality (m) = moles of solute/kg of solvent

• ΔTf = Kf × m × i

Types of Solids

1) Ionic Solids

2) Molecular Solids

3) Covalent Network Solids

4) Metallis Solids

Ideal Solutions

• Raoult’s Law: P(solution) = X1P1 + X2P2

  • P1 and P2 are vapor pressures of the two components

• Vapor Pressure Lowering Equation: deltaP/P = xsolute

  • deltaP/P is the relative vapor pressure lowering

  • x_solute = mole fraction of the solute

Ideal Gases

Unit Cells

1) Simple Cubic

• 1 atom

• Coordination number: 6

• Packing efficiency: 52% (48% is empty space)

• Edge length (x) = 2 * radius (R)

• 8 atoms at the corner. of the cube —> 1/8 × 8 = 1

• Volume = x³ = 8R³

2) Body centered Cubic

• 2 atoms

• CN: 8

• PE: 68%

• x = 4/sqrt(3) * R

• 8 atoms at the corner and one at the middle —> 1/8 × 8 + 1 = 2 atoms

3) Face Centered Cubic

• 4 atoms

• CN: 12

• PE: 74%

• x = sqrt8 * R

• 8 atoms at the corner, 4 on the sides, and one in the middle —> 1/8 × 8 + ½ * 4 + 1 = 4

4) Hexagonal close-packed

• CN: 12

• PE: 74%

Coordination number: the number of atoms every atom is touching

Packing efficiency: how much of the unit cell is actually consists of the volume of the atoms

Relationship between edge length and atomic radius

Test 8, Q 18

Good video to watch:

Thermochemistry:

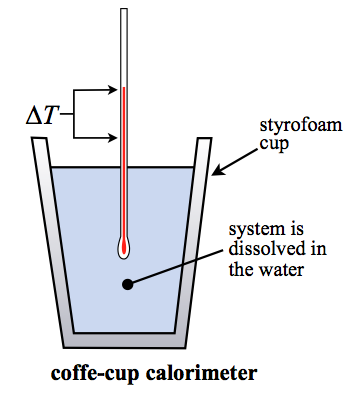
Coffee cup calorimeter and Bomb calorimeter (info 31)

1) Hess’s Law

2) Bond Dissociation Energy

• Bonds broken (reactants) - bonds formed (products)

3) Heat of formation

• Hf of products - Hf of reactants

4) Directly measure (Coffee cup and Bomb)

Important Equations (also combines it with electrochem):

ΔG = ΔH - TΔS = -RT lnK = nKE

Arrhenius: K = Ae^(-Ea/RT)

Entropy (ΔS): A measure of how disordered a system is

• Positive - becoming more disordered; negative - becoming less disordered

• Going from solid to liquid or liquid to gas —> +ΔS

• Going from less moles to more moles —> +ΔS

• Increase in complexity or disorder —> +ΔS

  • Molecule is more flexible —> +ΔS

  • For single molecules —> bigger molecule = higher entropy because it has more e- surrounding it

• Mixing of substances —> +ΔS

• Test 8, Q 24

Important formulas

• ΔE = Q

1) Coffee Cup Calorimeter - Insulated system, reaction in a solution

• Constant volume and pressure (ΔV = ΔP = 0)

  • Cannot expand or contract

  • Liquids are incompressible

  • ***If it involved a liquid or a solid, it maintains a constant volume and pressure.

• Work = 0

• ΔH = ΔE = Q

2) Piston Calorimeter - Gas Forming Reactions

• Piston changes volume (variable volume but constant P)

  • Ex: Gasoline engine

  • ΔV≠0, ΔP = 0

• ΔH = ΔE + PΔV = Q - PΔV + PΔV = Q —> ΔH = Q

3) Bomb Calorimeter - Gas forming reaction

• Constant V but varying P

  • ΔV = 0, ΔP ≠ 0

  • Measuring temp (C) of H2O

• ΔE = Q

• ΔH = Q + VΔP

*High Specific Heat = lower thermal conductivity

Heat of Formation

• Formation reaction: synthesis of a compound from its elements in standard conditions (25 C, 1 atm, 1M)

• Example: Formation reaction of Ethanol (C2H5OH)

  • 2 C(s) + 3H2(g) + ½ O2(g) —> C2H5OH (l) ΔHf = -277.7 kJ/mol

  • C2H5OH (l) ΔHf = -277.7 kJ/mol

  • C2H5OH (g) ΔHf = -235.7 kJ/mol

    • Different heat of formation for gas and liquid. It takes energy to turn vaporize the liquid into a gas (Heat of vaporization)

    • Hf (l) + Hvap = Hf(l)

    • -277.7 + x = -235.7 —> x = 42.6 kJ/mol

Hrxn = ∑n_products * H_f - ∑n_reactants * H_f

Solid Ice: C = 2.09

Water: 4.184

Gas: 2.02

Heat of vaporization: 2260 J/g

Heat of Fusion: 334 J/g

H_vap>H_Fus because it takes more energy to completely break the bonds between water molecule to bring them from a liquid to a gas. When going from solid to liquid, the bonds are only partially broken/loosened.

Energy

• ΔE = Q + (-PΔV)

  

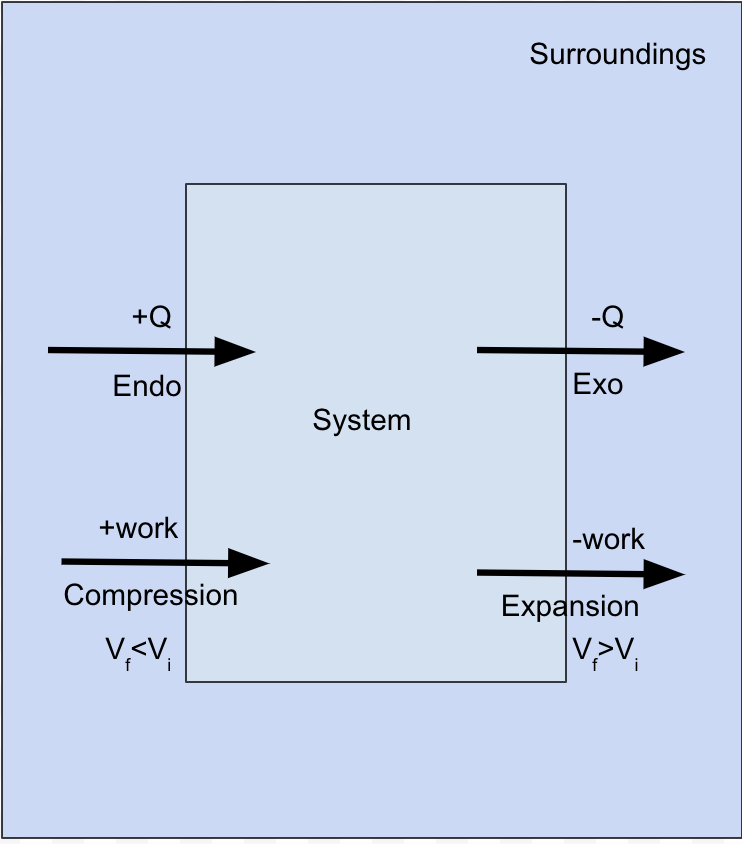
Increase ΔE = putting heat into system and compressing it

Decrease ΔE = releasing heat and expanding

UNITS: 1 L* atm = 101.3 Joules = 101.3 × 10^-3 kJ

w = -Pe*tΔV

when ∆H° > ∆E°, there is a net increase in gas moles

• ∆H = ∆E + ∆(PV) = ∆E + ∆(nRT)

3 laws of Thermodynamics

Kinetics

The Rate Law (Method of Initial Rates)

• Relation between initial concentration of reactants and initial rate of reaction

• Experimentally determined by data analysis

A + B —> C (Rate law only dependent on reactants)

rate = k[A]^x * [B]^y

• k = specific rate constant, depends on temp, Ea, and presence of catalysts

• Rate = M/time

• x, y = orders, x+y = overall order

Integrated Rate Law (IRL)

[A] —> Product, -Δ[A]_o/Δt = k[A]_o (derivative rate law/DRL)

rate law: Rate = k[A],

Half life: A_o = A_f * ½ ^(t/t_1/2)

Formulas:

lnK = -Ea/R * 1/T

Equilbrium:

A ⇌ bB + cC

Ksp = [B]^b [C]^c = (bx)^b * (cx)^c

Acid-Base:

pH = pKa + log [A-]/[HA]

kb = [BH+][OH-]/[B]

Ka = [H+][A-]/[HA]

Ionization

Dissociations for a diprotic and monoprotic acid

Listing acids in order of decreasing acidity

HW 18 Q 27: In which of the following are the carboxylic acids lised in order of decreasing acidity, from most acidic to  least acidic? 

1.CHF2CH2CH2CO2H 2. CH3CF2CH2CO2H 3. CH3CH2CF2CO2H 4. CH3CH2CH2CO2H

The acidity of a carboxylic acid is determined by the stability of its conjugate base. More stable conjugate bases lead to stronger acids. Electron-withdrawing groups (like fluorine) near the carboxyl group stabilize the conjugate base by withdrawing electron density. The closer the electron-withdrawing group is to the carboxyl group, the stronger the acid. 

Titration Curves:

A plot of volume of titrant added vs. pH

Strong Acid + Strong Base —> the starting point is very low pH (near 1) and then it jumps to a very high pH (very basic)

• Equivalence point = halfway between the jump - the moles of acid = the moles of the base

HCl present as H+ and Cl-

Adding NaOH —> goes in as Na+ and OH-

Cl- and Na+ are spectator ions, they don’t change anything, but the H+ and the OH- ions neutralize each other and form H2O

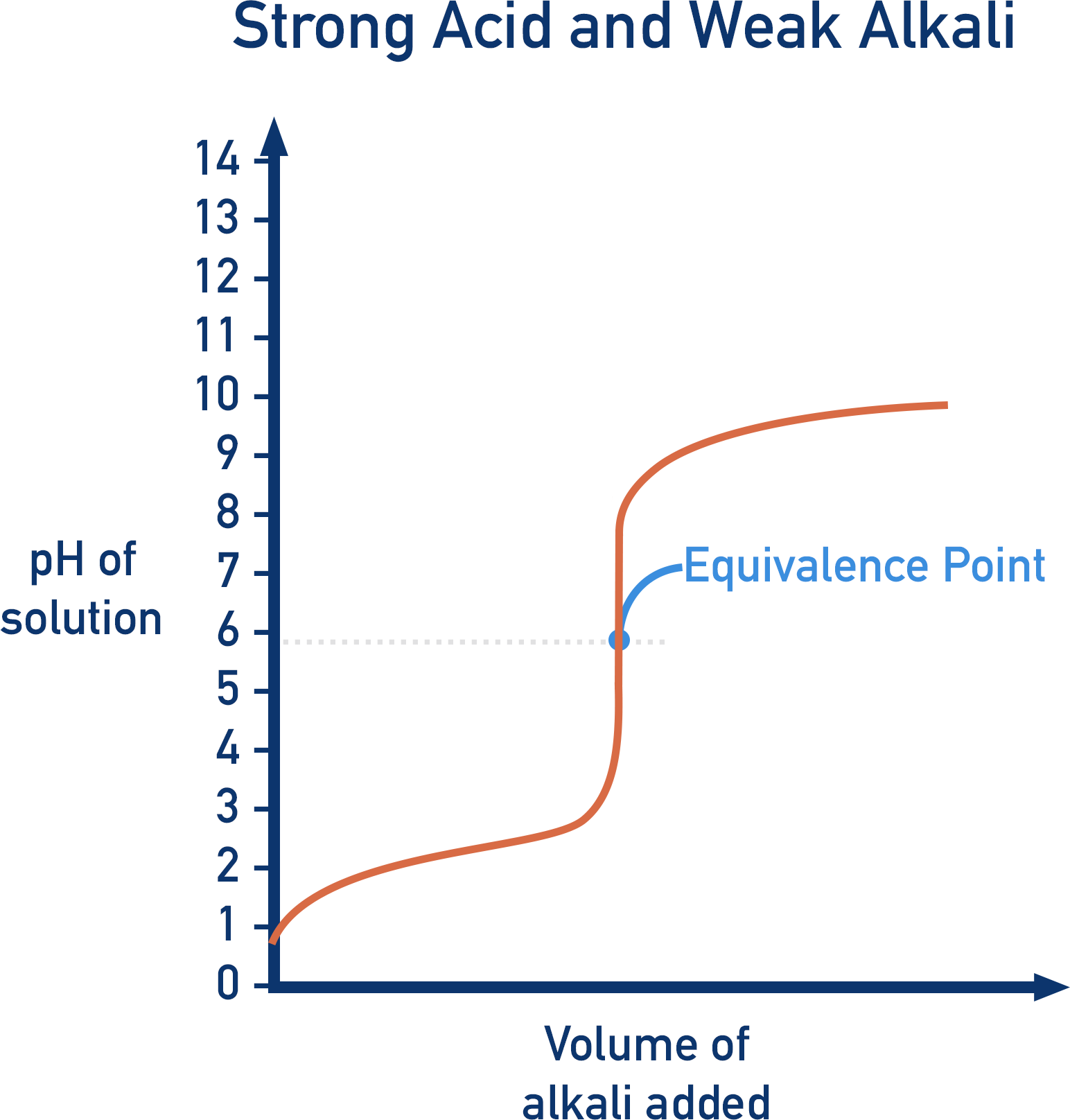
pH starts off low (strong acid to start with)

Example: HCl + NH3

• The equivalence point, the pH is lower than 7 and it is slightly acidic (the weak base does not completely balance out the strong acid)

• NH3 + H2O ⇌ NH4+ + OH- (does not go to completion, some of the acid remains as NH3 and some of it forms NH4+)

  • NH3 is a weak base and it’s conjugate acid is NH4+

  • ***The weaker the base, the stronger it’s conjugate acid is (same thing vice-versa)

  • The NH4+ contributes to the equivalence point being a little bit acidic.

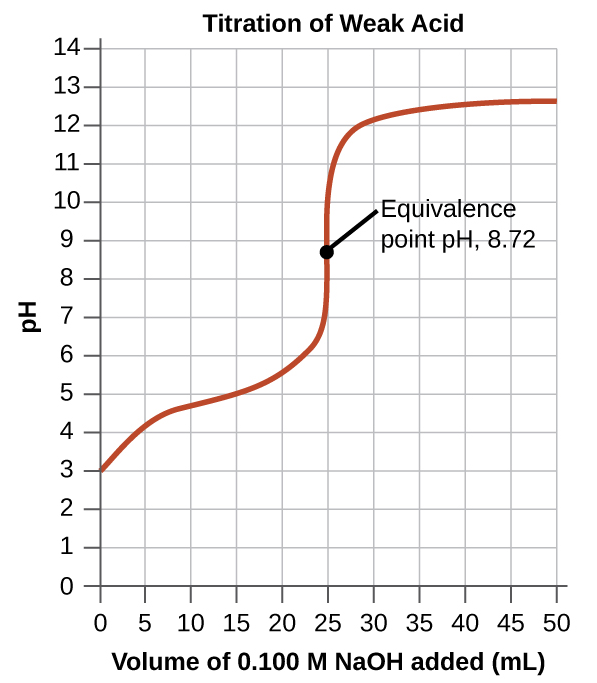
Starting pH is not as low and the equivalence point is at a pH above 7 (slightly basic)

• Ex: HC2H3O2 ⇌ C2H3O2- + H+ (partially dissociates)

  • C2H3O2- more of a strong acid (because HC2H3O2 is a weak base)

• Buffer Region (from x = 0, x = 20 around)

  • There will be a conjugate acid-base pair

  • Ex: NaOH will steal the H+ ions from the C2H3O2- + H+

  • This creates a bunch of C2H3O2- and HC2H3O2

  • Middle of the buffer region: pH = pKa

Henderson Hasselbach Equation - pH = pKa + log ([A-]/[HA]) —> this means that the concentratio of the conjugate base and conjugate acids are the same

1) Example: 28.9 mL of H2SO4 was completely titrated with 38.4 mL of a 0.25M NaOH solution. What is the concentration of H2SO4?

• H2SO4 + 2NaOH —> Na2SO4 + 2H2O

  • Strong Acid + strong base will completely neutralize each other and create a salt and a base

  • 38.4 mL x 1L/1000 mL x 0.25 mol NaOH/1 L x 1 mol H2SO4/2 mole NaOH x 28.9 mL/1000 mL = 0.1661 M H2SO4

• M1V1 (H2SO4) = M2V2 (NaOH)

  • # of protons in H2SO4 and # of OH- in NaOH

  • 2 protons in H2SO4 and 1 OH- in NaOH

  • Turns into 2M1V1 = M2V2 —> 2*M1 × 28.9 mL = 1 (0.25 M) (38.4 mL) **Make sure of units

    • M1 = 0.1661 M H2SO4

Kp = Kc (RT)^Δn (n = moles of gaseous products - reactants in a chemical reaction)

Finding the species at each major point

Le Chateliers Principle:

Common questions:

A saturated solution of which silver salt has the highest concentration of Ag+?

(A) AgCl, Ksp = 1.8 × 10-10

AgCl⇌Ag+ + Cl-

Ksp = [Ag+][Cl-] = x²

1.8 × 10^-10 = x² (solve for x)

(B) Ag2CrO4, Ksp = 1.1 × 10-12

(C) AgBr, Ksp = 5.0 × 10-13

(D) Ag2SO3, Ksp = 1.5 × 10-14

Greatest x value = highest concentration

Which one will precipitate? Find Qsp and compare it to Ksp, if Qsp>Ksp, then it will precipitate

Electrochemistry:

Formulas:

• Nernst Equation: E_cell = E - (RT/nF)lnQ

  • R = 8.314 J K-1 mol-1

  • T = Temp in Kelvins

  • n = number of electrons transferred int he balanced redoc reaction

  • F = Faraday’s constant 96485 C mol-1

  • Q = Reaction quotient

Oxidation and Reduction Reactions:

Balancing Redox reactions:

• Under Acidic conditions:

• Under Basic conditions:

Good overview of electrochemistry:

HW 18 Q 33: What is the standard reduction potential for the reduction of V3+(aq) to V(s)? V3+(aq) + 3 e– → V(s) Eº = ???

V2+ (aq) + 2e- --> V(s). V = -1.13

V3+ (aq) + e- --> V 2+ (aq). V = -0.26

G = -nFe

Atomic structure/periodicity/random

Nuclear stability

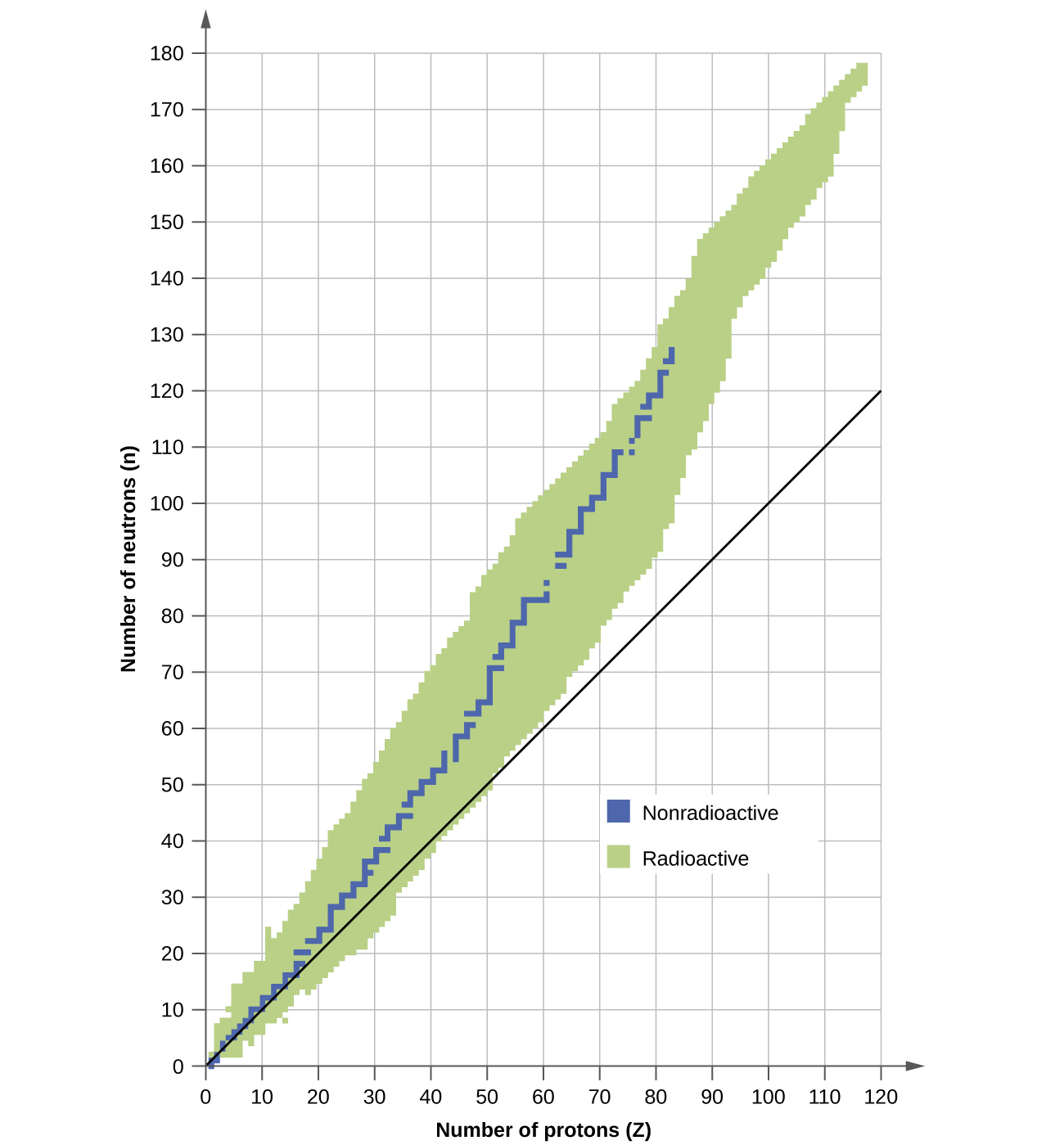
• Ration of n to p is 1 —> Stable

• For C-14

  • P = 6 and n = 8

  • n/p = 1.25 —> this is too high and there is too many neutrons so you must get rid of neutrons by beta emission

Radial Wavefunctions/Radial Nodes

• Total nodes: n-1

  • Nodes: Low probability that electrons will go to a certain region (on a graph, they are x-intercepts

• Radial nodes: n-1-L

• Agular nodes: L (Quantum number

Quantum numbers

Types of radioactive decay:

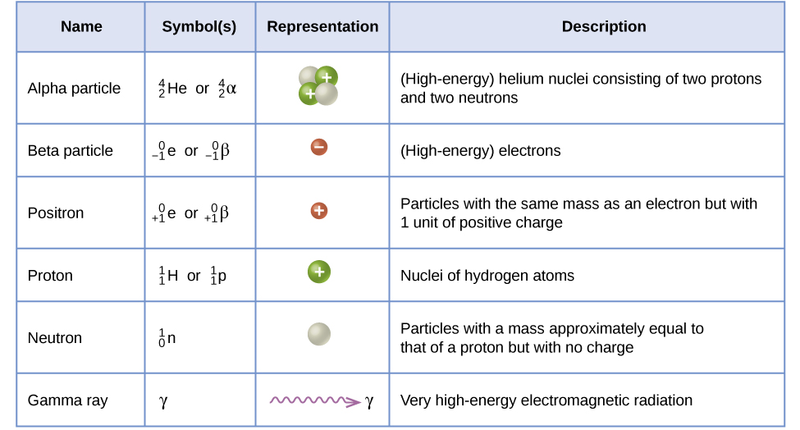
Important terminology

• Emission: Add the particle to the products

• Capture: Add the particle to the reactants

  • Ex: Electron capture

Complex Ions: A metal (Transition) ion surrounded by ligands (Covalently bonds)

• Ligands: Lewis base containing at least one lone pair like NH3, CN-, etc

  • Will donate electrons

• Coordination number: The number of coordinate covalent bonds (usually 2x the charge of the metal ion)

  • Most common number is 6

• Unidentate/monodentate

• Bidentate

• Polydentate

• Naming: Cation + Anion

• Common geometries: Octahedral, Tetrahedral, Square Planar (coordination # = 4)

  • Most complexes with coordination # = 4 are tetrahedral for the 3d metals

  • With Heavier metals (Pd and Pt) and a coordination # = 4 are square planar

• Isomers

• 

• Optical Isomers: optically active substance that can rotate the plane of polarize light

  • Enantiomers (Chiral molecules)

    • Non-superimposable mirror images

    • Rotate plane-polarize light in opposite directions

• Racemic mixture

  • An equal mixture of enantiomers

  • Does not rotate the plane of the polarize light at all

• Bidental - very easy to form enantiomers

Organic Chemistry

Complex Ion: Transition metal bonded with ligands

Chiral centers: R vs. S (Counterclockwise is S and Clockwise is R)

• If both chiral centers have opposite types —> enantiomers

• Diastereomers —> chiral centers are different but not exactly opposite from each other

• Ex:

  

1) Carbohydrate

• Degree of saturation

2) Lipid

3) Nucleic Acid

4) Protein

Electrophilic aromatic substitution reactions

Rules of aromatic compounds

Hukel’s rule: The number of pi electrons must be 4n+2 (n can be any integer)

Chiral Carbons

Carbon bonds to 4 unidentical groups

• Symmetrical structures are not chiral

Types of Isomers

1) Conformational isomers

2) Constitutional isomers

3) Stereoisomers

4) Geometric Isomers

Organic Chemistry reactions

• Alkyl Halides react because of their structure - has a high electronegativity

• Nucleophilic Substitutions

• Elimination reaction

Popular Organic Reactions:

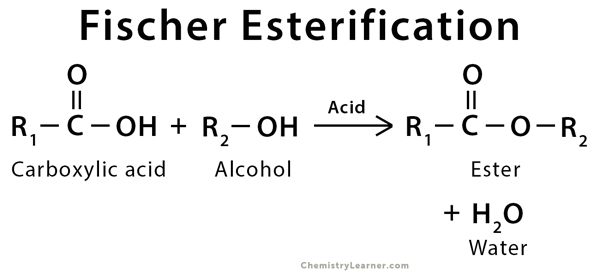
Esterification: Acid + alcohol (Use acid as a catalyst)

Sequential reactions

• One thing reacts and forms a compound. That compound reacts and forms another compound

Periodic Trends

• Reactivity

Questions:

Test 8, Q 19: A container with 100.0 g of ice at 0 °C is placed in a humid room whose temperature is 40 °C. The ice melts as water vapor condenses into the container. Assuming that all the heat transferred to the container comes from condensation, how much water will have condensed in the container once all the ice is melted and has reached 40 °C? The heat of fusion of ice is 334 J g-1 and the heat of vaporization of water is 2260 J g-1 .

Energy to melt: 100.0 g * 334 J g-1 = 33400 J

Energy to increase temp: 100.0 G 4.184 * (40 - 0) = 16736 J

Total energy: 16736 + 33400 = 50136 J

50136 J = m * 2260 —> m = 22.2 g of water vaporized

Test 8, Q 23: Two reactions have similar ∆H°rxn values (∆H°rxn (1) ≈ ∆H°rxn (2)), but reaction (1) has a much smaller standard entropy change than reaction (2) (∆S°rxn (1) << ∆S°rxn (2)). At 298 K, which statements about these two reactions must be correct?

I. Reaction (1) must have a larger equilibrium constant (Keq (1) > Keq (2)).

II. Reaction (1) must have a larger Arrhenius prefactor (A(1) > A(2)).

(A) I only (B) II only (C) Both I and II (D) Neither I nor II

Test 8, Q 35: What is the pH of a 0.10 M solution of NH4F? The Ka of NH4 + is 5.6 × 10-10 and the Ka of HF is 6.8 × 10-4 .

(A) 2.08 (B) 5.12 (C) 6.21 (D) 8.08

pKa1 = -log(5.6 × 10^10) = 9.25

pKa2 = -log(6.8 × 10^-4) = 3.17

0.5(9.25 + 3.17) = 6.21

• 0.1 M NaHCO3 in a solution and the problem gives Ka1 and Ka2

  • pH = ½ (pKa1 + pKa2)

  • pKa1 = -log (Ka1)

  • Amphoteric (can act as both a lewis base and acid) : Sodium won’t affect the pH

    • HCO3- + H+ —> H2CO3

    • HCO3- —> H+ + CO3 2-

Test 8, Q 42: The standard reduction potential of oxygen under acidic conditions at 298 K is +1.23 V. What is the standard reduction potential for the four-electron reduction of O2(g) under basic conditions?

(A) 1.23 V (B) 1.02 V (C) 0.83 V (D) 0.40 V

O2 + H+ + 4e- —> 2H2O (rxn1)

E = 1.23 (ΔG1)

Under Basic conditions:

• O2 + 2H2O + 4e- —> 4OH- (rxn2)

• E = x (ΔG2)

H2O —> H+ + OH- (rxn 2)

Kw - 10^-4 (ΔG3)

Must find (ΔG2)

Conversions:

1m = 10³mm = 10^6mm = 10^9 mm = 10^12 pm

** Super good youtube channel to watch: The Organic Chemistry Tutor