Chapter 3: Two Dimensional Kinematics
Fundamentals of Two-Dimensional Kinematics
Introduction
Kinematics is the study of motion without considering the forces causing it.
Two-dimensional kinematics deals with motion in a plane, considering both horizontal and vertical components.
3.1 Displacement, Velocity, and Acceleration
Initial position = r_{0}
Final position = r
Displacement = \Delta r=r-r_{0}
Average velocity is displacement divided by the elapsed time.
\overrightarrow{\overline{v}}=\dfrac{\Delta r}{\Delta t}
Instantaneous velocity is how fast a car moves and the direction of motion at each instant of time.
\overrightarrow{\overline{v}}=\lim _{\Delta t\rightarrow 0}\dfrac{\Delta r}{\Delta t}
Definition of average acceleration
\overrightarrow{\overline{a}}=\dfrac{\Delta v}{\Delta t}
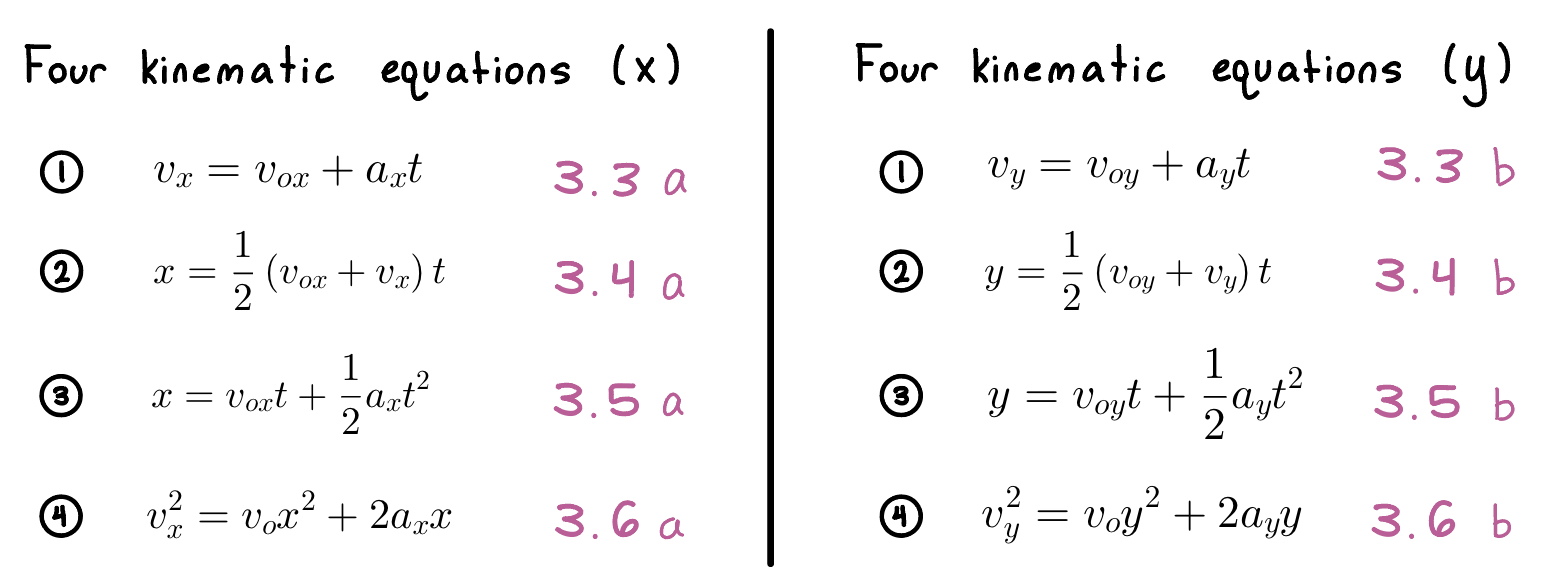
3.2 Equations of Kinematics in Two Dimensions
Kinematic equations are separated into x and y components:
If velocity and acceleration go in the same directions, velocity increases
Different directions means velocity is decreasing
When solving a problem for kinematics in two dimensions, list the given variables.
Ensure you include proper signs.
Variable table:
x-component
y-component
Units
Displacement
x
y
m
Initial Velocity
v_{0x}
v_{0y}
m/s
Final Velocity
v_{x}
v_{y}
m/s
Acceleration
a_{x}
a_{y}
m/s²
Time (same for both)
t
t
s
Reasoning Strategy: Applying the Equations of Kinematics in Two Dimensions
1.) Make a drawing
2.) Decide which directions are positive and negative for both the x axis and the y axis.
3.) Write down the values that are given for any of the five kinematic variables associated with each direction.
4.) In an organized way, write down the values (with appropriate + and - signs) that are given for any of the five kinematic variables associated with the x direction and the y direction. Be on the alert for implied data, such as the phrase “starts from rest”, which means that the values of the initial velocity components are zero: vox/y = 0 m/s. The data summary boxes used in the examples are a good way of keeping track of this information. In addition, identify the variables that you are being asked to determine.
5.) Before attempting to solve a problem, verify that the given information contains values for at least three of the kinematic variables. Do this for the X and the Y direction of the motion. Once three known variables are identified, use the correct equation.
3.3: Projectile Motion
Under the influence of gravity alone, an object near the surface of earth will acceleration downwards at 9.80 m/s2
ay = -9.80 m/s2
ax = 0
vx = v0x = constant
Projectile Motion
 Full Projectile
Full Projectile

Projectile Motion (Extra Notes)
Projectile motion refers to the motion of an object that is launched into the air and moves along a curved path under the influence of gravity.
It occurs when an object is given an initial velocity and then moves freely under the force of gravity.
The path followed by the object is called a parabola.
The motion can be divided into two independent components: horizontal and vertical motion.
The horizontal motion is constant and unaffected by gravity, while the vertical motion is influenced by gravity.
The object experiences a constant horizontal velocity throughout its motion.
The vertical motion is influenced by the acceleration due to gravity, which causes the object to accelerate downward.
The time of flight is the total time taken by the object to complete its trajectory and is determined by the initial velocity and the angle of projection.
The maximum height reached by the object is determined by the initial velocity and the angle of projection.
The range of the projectile is the horizontal distance covered by the object and is determined by the initial velocity and the angle of projection.
The range is maximum when the angle of projection is 45 degrees.
The velocity of the object at any point in its trajectory can be determined by resolving the initial velocity into its horizontal and vertical components.
The horizontal velocity remains constant, while the vertical velocity changes due to the acceleration due to gravity.
The time taken to reach the maximum height is equal to the time taken to return to the same vertical position.
Projectile motion is commonly observed in sports such as basketball, baseball, and javelin throwing.
3.4: Relative Velocity
Relative velocity is the velocity of an object or observer with respect to another object or observer.
Equation for relative velocity: \overrightarrow{v}_{AC}=\overrightarrow{v}_{AB}+\overrightarrow{v}_{BC}
It can be calculated by subtracting or adding the velocities of the objects involved, depending on their direction of motion.
This concept is important in physics, engineering, and navigation, as it helps understand the motion of objects in relation to each other and predict their interactions.
It is also used in solving problems related to collisions, projectile motion, and relative motion of objects in different frames of reference.
However, it's important to note that relative velocity depends on the choice of reference frame and different observers may have different relative velocities between the same objects.
Relative velocity is closely related to the concept of relative motion, which involves studying the motion of objects in relation to each other.
Conclusion
Two-dimensional kinematics involves analyzing motion in a plane, considering both horizontal and vertical components.
Displacement, velocity, and acceleration are vector quantities with magnitude and direction.
Projectile motion follows a parabolic path