Game Theory and Strategic Thinking - chapter 9
9.1. Games and Strategic Behaviour
- Game: a situation involving at least two people that requires those involved to think strategically
- Game theory: the study of how people behave strategically under different circumstances
- behaving strategically: acting to achieve a goal by anticipating the interplay between your own and others’ decisions
- Games have rules, which define the actions players are allowed to take
- Strategies: are plans of action that players can follow to achieve a desired goal
- Payoffs: the rewards that come from taking particular actions
9.2. One-Time Games and the Prisoners’ Dilemma
- Prisoners’ dilemma: a game of strategy in which two people make rational choices that lead to a less-than-ideal result for both
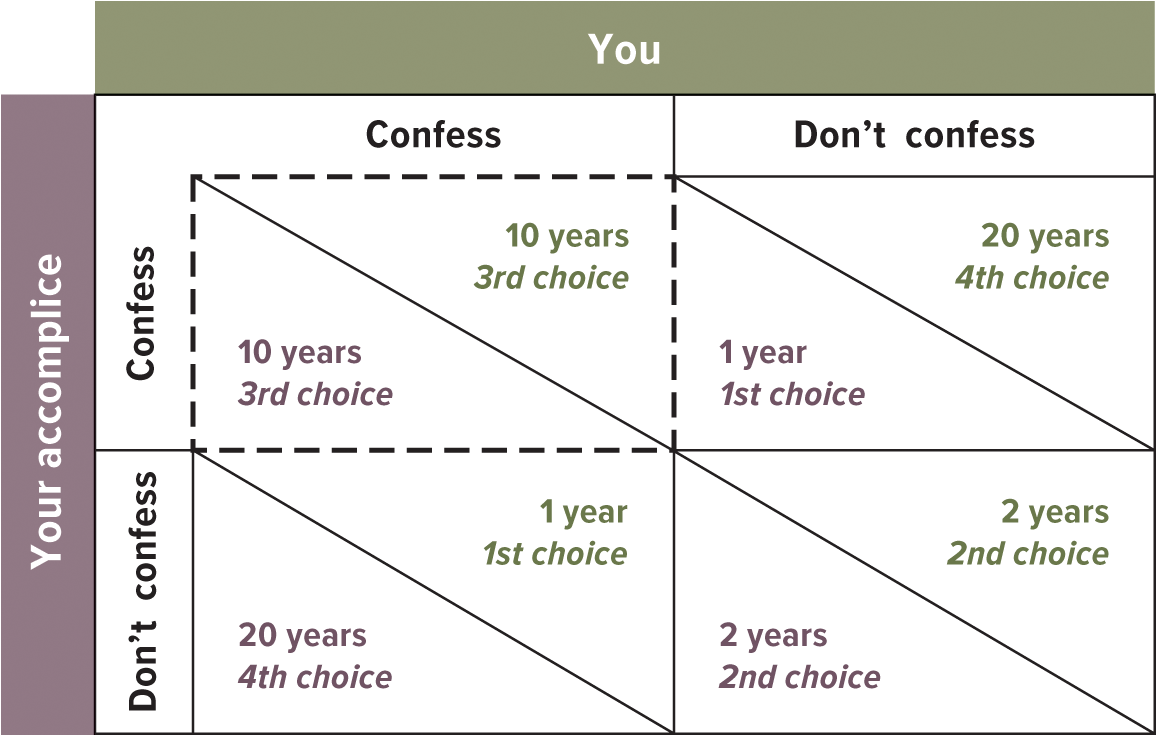
- Each player has a choice to cooperate or not to cooperate
- Each prefers mutual cooperation to mutual non-cooperation
- But non-cooperation is the best choice because its payoff is higher no matter what strategy other players choose
- In this game, pursuing you own self-interest leaves everyone worse off
9.3. Finding the Dominant Strategy
- Dominant strategy: When a strategy is the best one to follow no matter what strategy other players choose
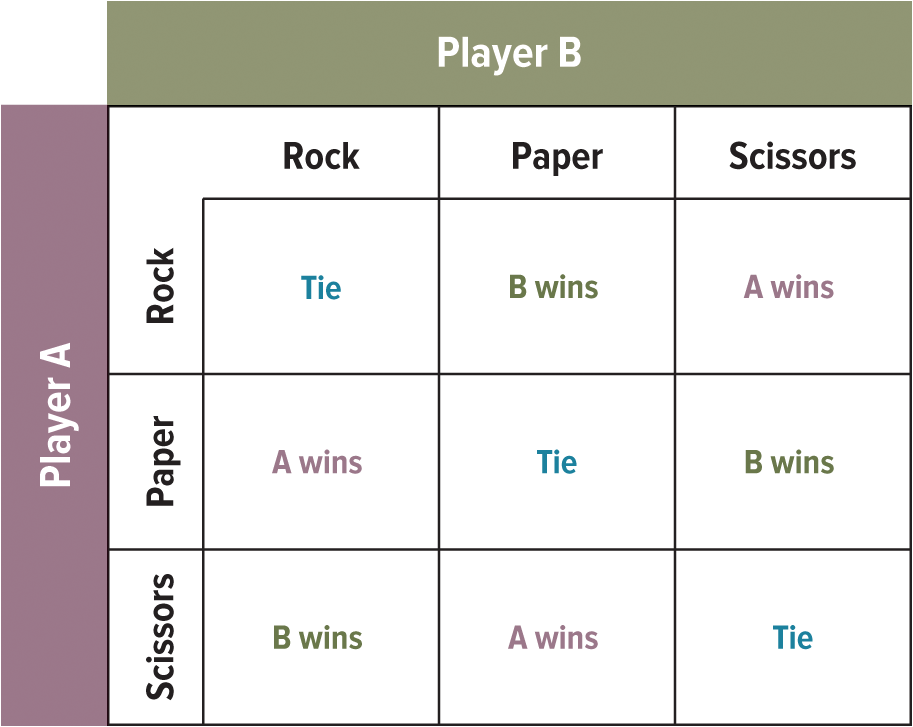
- Not all games feature a dominant strategy
- In some games there is no single strategy that will work best for you
9.4. Reaching Equilibrium
- When all players choose the best strategy they can, given the choices of all other players, those players have reached a Nash equilibrium
- Players who have reached a Nash equilibrium have no reason to regret their decision
- This doesn’t necessarily mean that an equilibrium outcome to a game is a good one
- We can see both negative-negative and positive-positive outcomes in equilibrium
- The only condition is that there’s no incentive to switch from one decision to another
9.5. Avoiding Competition Through Commitment
- In the prisoners’ dilemma, the players of the game would be better off if they could cooperate and make a deal beforehand not to confess
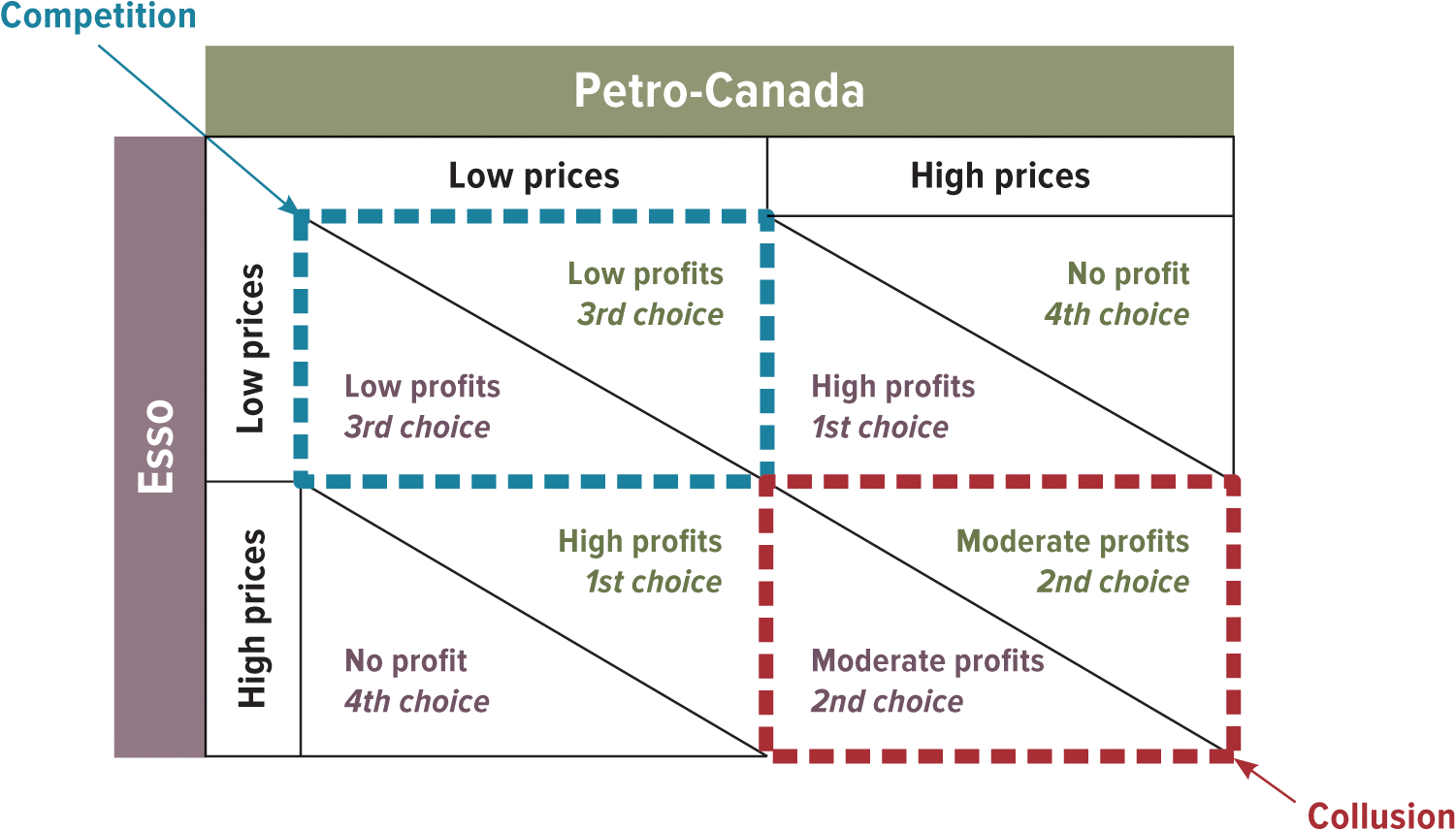
- However, even if you know you accomplices will not confess, you are still better off confessing
- To solve the problem, players may sometimes reach a mutually beneficial equilibrium by pursuing a commitment strategy
- Where they agree to submit to a penalty if they defect from the equilibrium
9.6. Repeated Play in the Prisoners’ Dilemma
- In a repeated game, players can penalize each other for defecting in one round by punishing each other in the next round
- As a result, the players can sometimes achieve a mutually beneficial equilibrium, even when they couldn’t do so in a single game
- A common strategy in repeated games is tit-for-tat, in which a player takes the same action as their opponent in the previous round
- Anyone who is playing against a person with a tit-for-tat strategy has a strong incentive to cooperate because defecting would push them into a less profitable equilibrium in every future round of the game
9.7. Think Forward, Work Backward
- Backward induction is the process of analyzing a problem in reverse
- Starting with the last choice, then the second to last choice, etc
- This problem-solving tool can be used to choose between options with different consequences down the road
- You first choose the goal you are trying to reach and then determine the steps needed to reach it
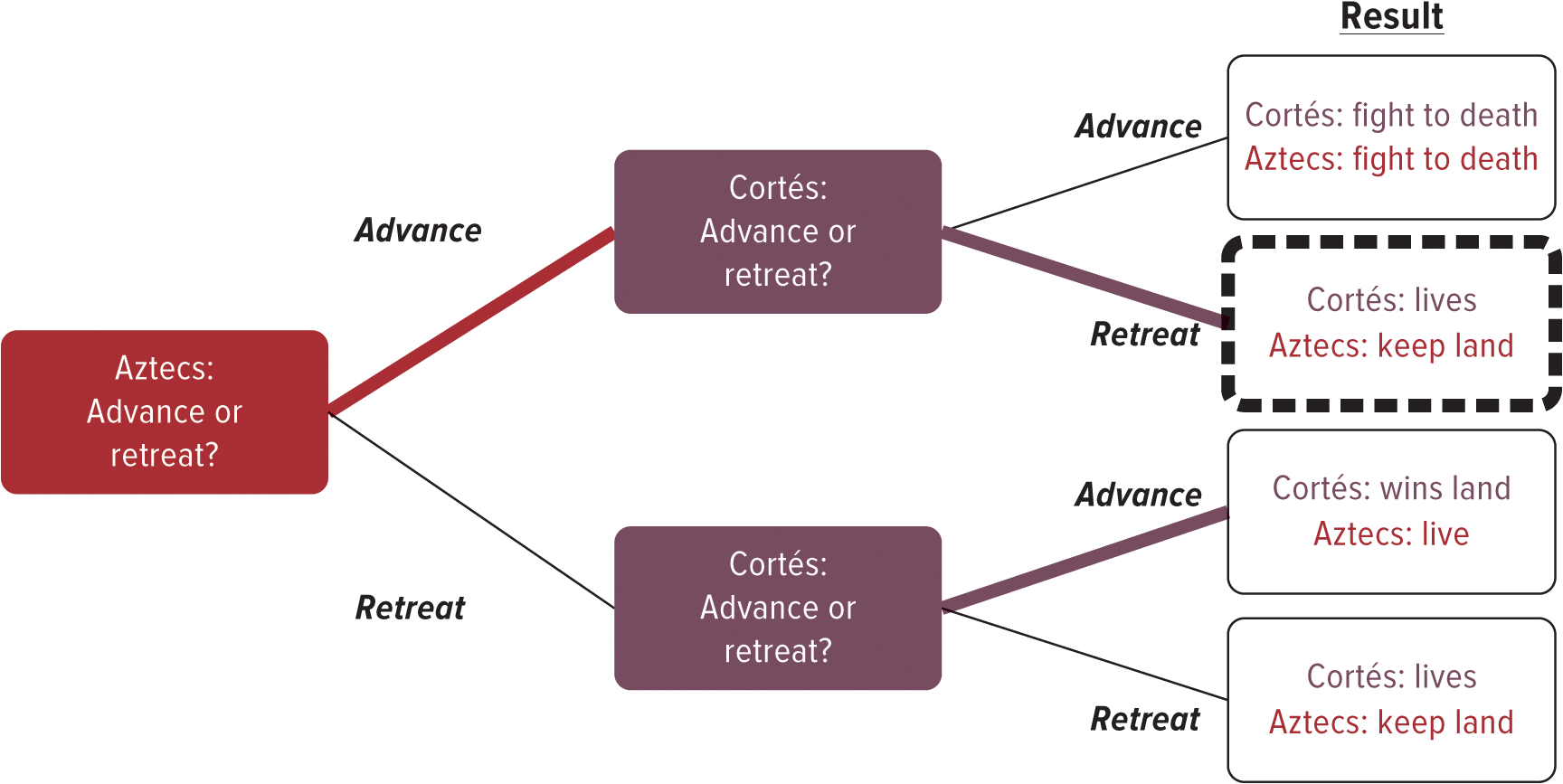
9.8. Deterring Market Entry: A Sequential Game
- In many situations, one person or company must make a decision before the other one
- These situations can be represented as games in which players move sequentially rather than simultaneously
- Because the payoff each achieves still depends on the other’s decision, the player who moves first must anticipate the decision the next player will make in response
- Decisions can be diagrammed as the nodes in a decision tree, which branch off into the choices or payoffs that follow from each option
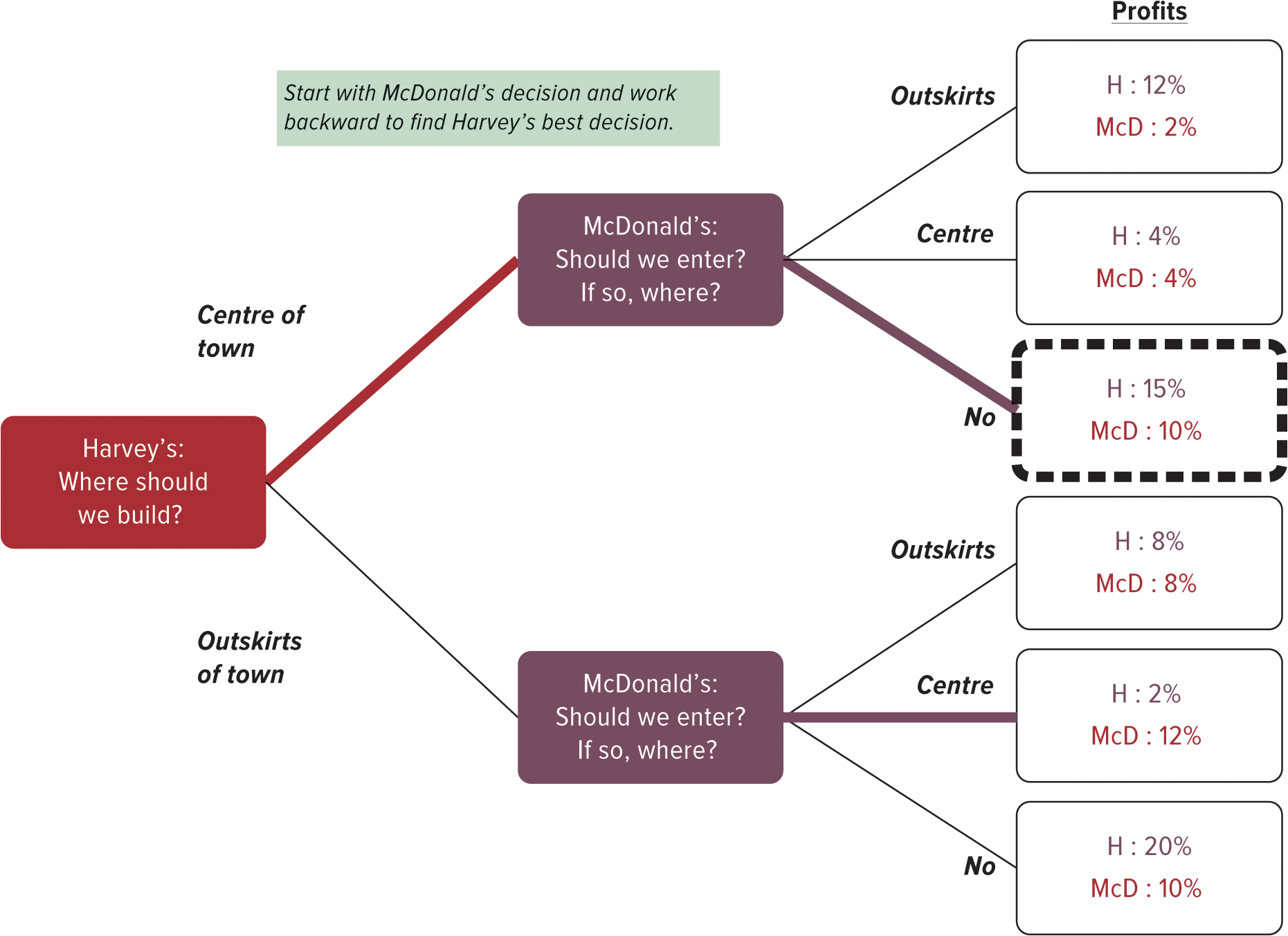
- Backward induction can be used to analyze decision tree and determine the best course of action at each stage of the game
9.9. First-mover Advantage in Sequential Games
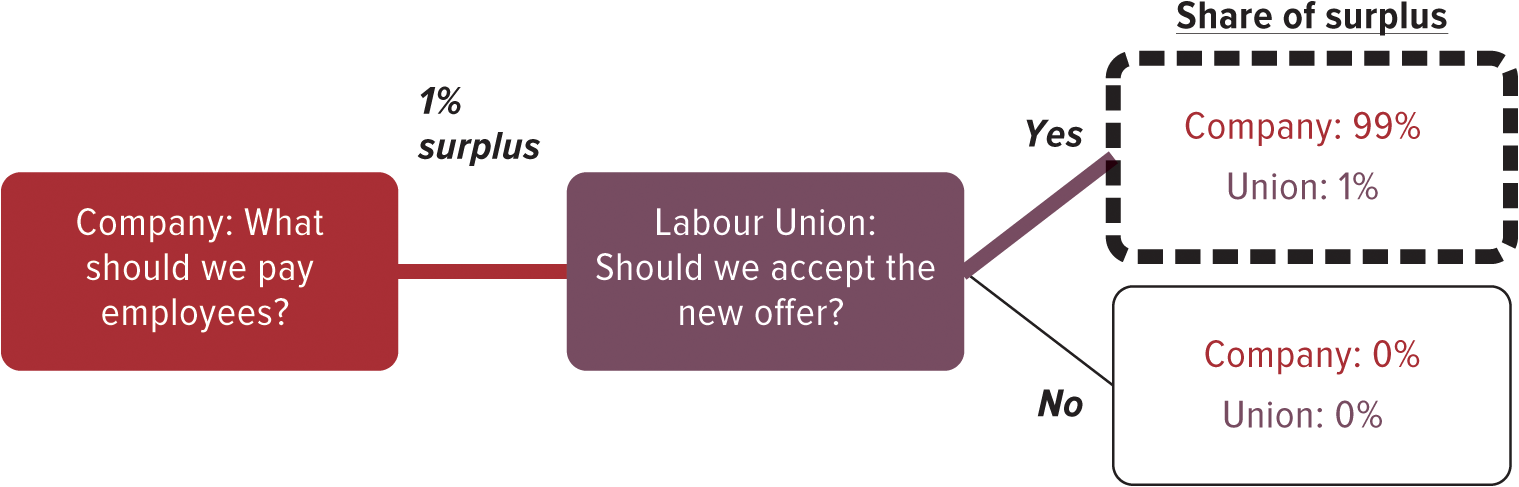
- In a game with first-mover advantage, the player who moves first gets a higher payoff than those who follow
- Example: a one-round bargaining game in which the person who makes the first offer gets virtually everything
- The ability to bargain over multiple rounds of offers and counter-offers dilutes the first-mover advantage
9.10. Repeated Sequential Games
- The ability to make counter-offers transforms bargaining from a game in which first-mover advantage trumps everything into a game of patience
- Bargaining takes time
- In every round of bargaining that takes place before the players reach agreement, the value of the payoff they are splitting goes down
- The more patient player who places more value on money in the future relative to money in the present, has an advantage
- The surplus will be divided in proportion to the patience of each player
9.11. Commitment in Sequential Games
In a sequential game, limiting your own choices can change your opponent’s behaviour
Example: following a commitment strategy turns a non-credible threat into a credible one, changing the payoffs associated with an opponent’s options