Mechanical Properties of Fluid
1. Pressure
Pressure (P):
Where:
F= Force, A = AreaPressure due to a fluid column:
P=hρg
Where:
h = height of the fluid,
ρ = density of the fluid,
g = acceleration due to gravity
2. Pascal's Law
Statement:
"Pressure applied to an enclosed fluid is transmitted undiminished to every portion of the fluid and the walls of the container."Applications:
Hydraulic lift:
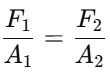
Where
F1,F2 are forces, and
A1,A2 are areas.
3. Viscosity
Newton's Law of Viscosity:
η=(F*L)/(A*V)
Where:
η = coefficient of viscosity,
A = area,
F = force required to maintain the flow (N)V/L = velocity gradient (rate of change of velocity with distance)
Stokes’ Law:
F=6πηrv
Where:
r = radius of the sphere,
v = velocity of the objectTerminal Velocity:
vt=2r2(ρs−ρf)g9ηv_t = \frac{2 r^2 (\rho_s - \rho_f) g}{9 \eta}vt=9η2r2(ρs−ρf)g
Where
ρs\rho_sρs = density of the sphere,
ρf\rho_fρf = density of the fluid
4. Streamline and Turbulent Flow
Equation of Continuity (Conservation of Mass):
A1v1=A2v2
Where
A = cross-sectional area,
v= velocity
5. Bernoulli’s Theorem
Energy Conservation for Fluids:
P+(1/2)ρv^2+ρgh=constant
Where:
P = pressure energy,
(1/2)ρv^2 = kinetic energy per unit volume,
ρgh = potential energy per unit volume
6. Surface Tension
Surface Energy:
Surface Energy=TΔA
Where T = surface tension,
ΔA = change in surface area
Excess Pressure:
Inside a liquid drop: ΔP=2T/r
Inside a bubble: ΔP=4T/r
Capillary Rise:
h=2Tcosθ/ρgrh
Where:
θ = angle of contact,
r = radius of the capillary
Simplified Notes:
Viscosity:
It’s the measure of a fluid's resistance to flow. Honey has higher viscosity than water.Bernoulli's Principle:
Faster-moving fluids exert lower pressure (used in airplanes and chimneys).Surface Tension:
It’s the tendency of liquid surfaces to shrink into the minimum surface area (seen in soap bubbles and water droplets).Capillarity:
Liquids rise or fall in a capillary tube due to adhesion and cohesion.Streamline vs. Turbulent Flow:
Streamline: Smooth and regular flow of fluid.
Turbulent: Chaotic and irregular flow.
 Knowt
Knowt
