Newton's First Law of Motion: Inertia

2.1

Aristotle on Motion:

Divided motion into two categories: * Natural motion * Violent motion
Natural motion occurs due to the nature of the object and depends on the elements it contains (fire, air, water, earth). * A lump of clay is of the earth. When it isn’t supported, it falls to the ground. * A puff of smoke is made of air and will rise to the air. * A feather is a mixture of earth and air and will fall to the ground when unsupported, but not as fast as the lump of clay.
Heavier objects fall faster, lighter objects fall slower, i.e., objects fall at speeds proportional to their weight.
The heavier the object, the faster it falls.
Natural motion is either straight up or straight down for objects on earth, and circular for celestial bodies.
Circular motion has no start or end; it keeps repeating.
He believed that different rules thus applied to celestial bodies. He stated that they were perfect spheres made of perfect, unchanging substances. He called this quintessence.
Violent motion is a result of pushing or pulling forces.
It is an imposed motion. It is externally caused. Objects do not move by themselves. * Someone pushing a cart. * Someone lifting a heavy weight. * Wind motion imposed on ships.
Difficulties in the concept of violent motion: forces causing the pushes and pulls weren’t always obvious. * Arrow moving due to a bowstring at first, but seeming to move on its own after that.

[Aristotle’s reasoning behind the arrow continuing to move: Parting of air by the moving arrow resulted in a squeezing effect at the end of the air, causing the air to rush back and prevent a vacuum from forming. Arrow then gets propelled through the air.]

\ Quick Summary: Motions of objects are due to either:

1. the nature of the object (comes from within)

external forces acting on the object (pushing/pulling)

An object in its proper place doesn’t move unless subjected to an external force.
Except for celestial objects, the normal state for an object is a state of rest.

Copernicus and the Moving Earth:

For his theory of the moving Earth, he assumed that Earth and other planets circled the Sun.
Worked privately for years because: * he was afraid of being persecuted, because his theory was entirely different from public opinion. * he wasn’t entirely sure of it himself. (The concept of a moving Earth didn’t make sense with the then existing theories on motion.)
His exposition was called De Revolutionibus. He sent it to the printer in his final years of life.
His theory and Aristotle’s views on motion challenged the beliefs of the Church. They threatened their authority and faith. Eventually, however, the Church accepted them.

2.2

Galileo’s Experiments:

He was a scientist of the early 17th century.

He proved Copernicus’ notion.

He did this by discrediting Aristotle’s views.

Leaning Tower:

Galileo dropped objects of different weights from the top of the Leaning Tower of Pisa and compared their falls.
When the objects were released together, they hit the ground at the same time. Their weight had nothing to do with the speed of their fall. This disproved Aristotle’s theory.
There was the minimal effect of air resistance.
Despite proving this publicly, some people still stuck to Aristotle’s word.

Inclined Planes:

Galileo was more concerned with how things moved than why they moved.
Aristotle based his theories on what he observed in nature around him, and thus came to the conclusion that motion always required a medium to resist it, like air or water. He believed that vacuums couldn’t exist because there was no medium.
Galileo rejected this principle. He tested his own hypothesis by judging the motions of objects on plane surfaces tilted at different angles.
Balls rolling downwards went faster. Balls rolling upwards went slower. Balls rolling on a horizontal plane stayed the same speed.

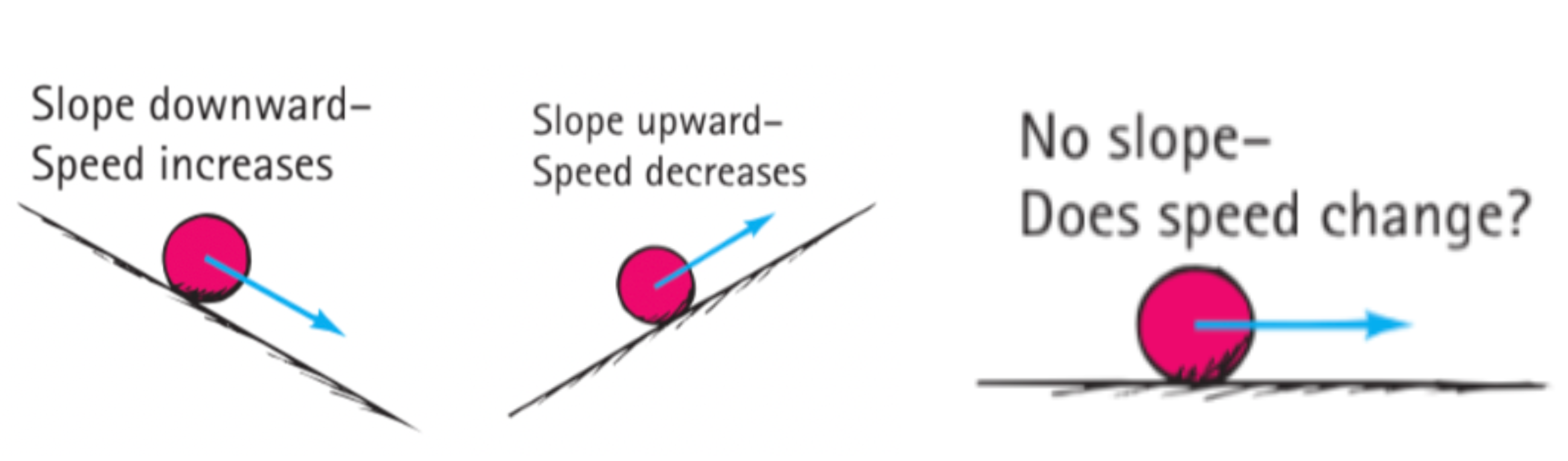
He stated that the balls finally came to rest because of friction, not because of their internal nature.
He backed this theory up by observing motion on smoother surfaces. The smoother the surface, the lesser the friction, the longer the object moved at the same speed.
Galileo thus realized that an object moving on a horizontal frictionless surface would keep moving at that speed infinitely.
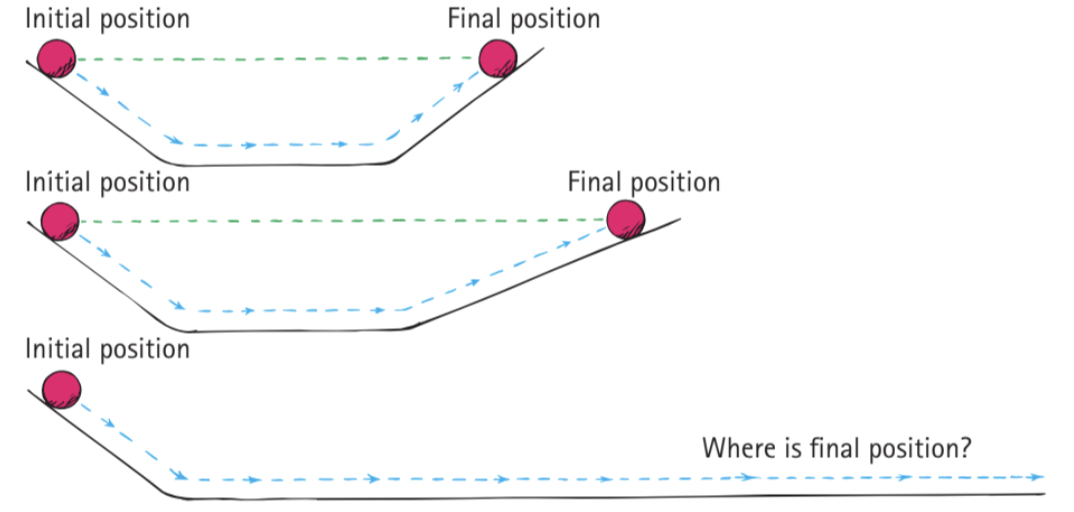
Another experiment Galileo used to prove his theory: * Placed two inclined planes facing each other. * If a ball was released from rest from the top of a downward sloping plane, it would rise to almost the same height again on the opposite upward sloping plane. * He stated that the only reason the ball didn’t rise to the exact same height was because of friction. * The smoother he made the planes, the higher the ball rose. * When he reduced the angle of the upward sloping plane, the ball had to travel a longer distance to reach the same height. * The steeper the slope, the more rapidly the ball loses its speed. * When there is no slope and no resistive force, the speed of the ball doesn’t change at all.
The property of an object to resist changes in motion is called inertia**.**
Aristotle couldn’t pin down the concept of inertia because he never imagined motion without friction.

Quick Summary:

→ Aristotle said moving objects are propelled by a steady force.

→ Galileo said moving objects are propelled by the absence of a force acting on them.

→ Inertia: The tendency of objects to resist changes in motion.

2.3

Newton’s First Law of Motion:

It’s called the Law of Inertia.
Statement:

Every object continues to remain in a state of rest or of uniform speed in a straight line, until it is acted upon by a nonzero net force.

keyword: continue.
Object continues doing what it’s doing until a force acts on it.
Eg: * state of rest: whipping a tablecloth out from under the dishes on the tabletop, without actually disturbing the dishes. * state of motion: space probes moving constantly in outer space.

2.4

Net Force and Vectors:

Changes in motion happen due to forces or combinations of forces.
Force → a push or pull.
Forces can be gravitational, electrical, magnetic, or muscular effort.
Net force → the force that’s a result of multiple forces acting on the same object. * e.g: two people pulling an object in the same direction with the same amount of force each doubles the force acting on the object. * if the two people pull with the same amount of force but in opposite directions, the sum of the forces (net force) becomes equal to zero.
Vector → an arrow drawn to represent a force, the length and direction of these arrows are drawn to scale.
Vector quantity → a quantity that’s defined by both magnitude and direction. * e.g: force, velocity, acceleration.
Scalar quantity → a quantity defined only by its magnitude. * e.g: mass, volume, speed
When two vectors are parallel to each other: * they’re added if they act in the same direction. * they’re subtracted if they act in opposite directions.
Resultant → Sum of two or more vectors.
The Parallelogram Rule is used to find the resultant of two vectors that aren’t exactly in the same or opposite direction.

(How to implement it: ) * construct a parallelogram with the vectors as adjacent sides. * the diagonal of the parallelogram is the resultant.

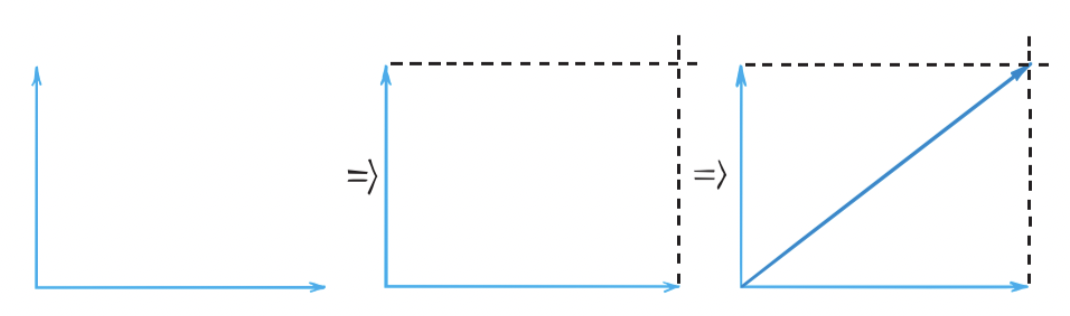
The parallelogram turns into a square for vectors equal in magnitude and perpendicular to each other. For any square, the length of the diagonal is root 2. So, the resultant is root 2 times one of the vectors.

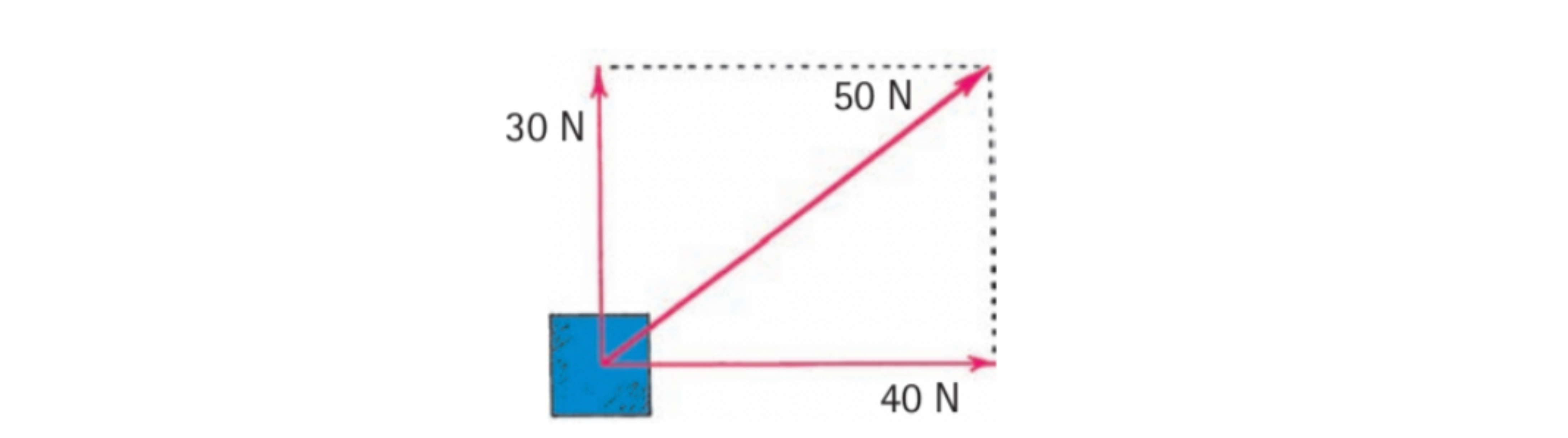
Force Vectors:

The figure shows force vectors acting on a box:

Forces: 30N, 40N, 50N

The next figure shows a doll hanging from a clothesline:

This figure shows three forces acting on the doll.

1. Her weight. 2. A tension in the left hand side of the rope. 3. A tension in the right hand side of the rope.

Due to the different angles, the rope tensions are different on either side.
The parallelogram rule shows us that the tension is greater in the right hand side.
Both the rope tensions combine to balance the weight of the doll.

2.5

The Equilibrium Rule:

If you tie a string around a 2-pound bag of flour and hang it on a weighing scale, a spring in the scale stretches until the scale reads 2 pounds.
Tension → the stretching force causing the string to stretch.
A scale in the science lab would read 9 newtons.
Pounds and newtons are units of weight, which are units of force.
2 pounds is equivalent to 9 newtons.
There are two forces acting on the bag of flour:

1. tension force acting upwards 2. weight acting downwards

These two forces are equal and opposite; they add up to zero. So, the bag stays in a state of rest. No net force acts on the bag.
The bag is in mechanical equilibrium.
ΣF = 0 → Equilibrium equation * Symbol stands for “vector sum of”, F stands for “forces”.
For a suspended object, if the sum of the forces acting upwards and the forces acting downwards is zero, it stays at rest.

2.6

Support Force:

A book lying at rest on a table is in equilibrium. Forces acting on the book:

1. gravity, exerted downwards, due to weight of the book. 2. support force, exerted upwards, to counter the force of gravity.

The support force is sometimes called the normal force.
It keeps the book in a state of equilibrium so it doesn’t move.
It’s equal to the weight of the book.
The book pressing down on the table is similar to pressing down on a spring. The table exerts a force back onto the book to keep it in place, just like how a spring pushes back onto your hand.

\ When you are in equilibrium, your weight is equal to the force of gravity acting on you.

2.7

Equilibrium of Moving Things:

Rest is one form of equilibrium.
An object moving at a constant speed in a straight line is another form of equilibrium.
Equilibrium is a state of no change. * Static Equilibrium → when the object is at rest. * Dynamic Equilibrium → when the object is moving at a constant speed in a straight line path.
By Newton’s first law, an object under the influence of only one force can’t be in equilibrium, because the net force can’t be zero.
To test whether something is in equilibrium or not, check for changes in its state of motion. * A crate being pushed across a factory floor is in dynamic equilibrium. (which means that more than one force acts on it.) * The force of friction acting between the crate and the floor is equal and opposite to the pushing force acting on the crate.
For any object in static or dynamic equilibrium, the sum of forces acting on it is always zero.

2.8

The Moving Earth:

Copernicus put forth the concept of a moving earth in the 16th century, but it sparked a lot of argument, as:

1. Inertia wasn’t understood then. 2. The amount of force required to keep the Earth moving was unfathomably large. 3. For a bird dropping vertically downwards from a tree to catch a worm, it wouldn’t have been able to catch it if the Earth moved.

The Earth would have to move at a speed of 107,000 km/h to circle the Sun in a year. That’s 30 km/s. Even if the bird managed to get to the worm in one second, the worm would’ve been moved 30 km away.
Without inertia, the concept of a moving Earth would never have been understood. * The Earth moves at 30 km/s. So does the tree, the bird, and the worm. * When things are in motion, they stay that way until unbalanced forces act on them.
If you stood next to a wall and jumped (so your feet aren’t in contact with the floor anymore), the wall wouldn’t slam into you.
It was hard to understand concepts like these 400 years ago, especially when people didn’t have fast moving vehicles to prove it to them. * If you flipped a coin vertically in a high speed vehicle, you’d be able to catch it. This is due to inertia.
Aristotle didn’t recognize the idea of inertia because, to him, all moving objects didn’t follow the same rules. * He saw vertical movement as natural, horizontal movement as unnatural and requiring a sustaining force.
Galileo and Newton acknowledged that all moving objects followed the same rules.

Moving objects continue to move until there’s an opposing force (like friction) stopping them.