AP chem unit 3 part 1: Properties of Substances and Mixtures
3.1: Intermolecular Forces
Intermolecular forces(IMF): the attractions between atoms, ions, or molecules
Intramolecular forces: chemical bonds holding the atoms in a molecule together

london dispersion forces | Dipole Dipole | Hydrogen bonding | Metallic | Ionic |
-all substances -only force present in nonpolar molecules/single atoms | -polar molecules -asymmetrical molecules with only non metals | -a strong type of dipole dipole -polar molecules with H directly bonded to F, O, N | -only metal atoms | -metals w/ non metals |
Ne, H2, CO2 | HCL, CH2O, CH3OH | HF, H2O, CH3OH | Au, Al, Zncu | NaCl, KBr, MgO |
London dispersion forces(LDFs):
occurs in all substances
as electrons move around a temporary dipole is formed
area of excess negative charge w/ lots of electrons & area of positive charge
negative and positive charges attract nearby molecules/atoms
forms an induced dipole
usually temporary
weak
polarizability: ability of an atom to form a temporary or induced dipole
stronger when atom/molecule has more electrons & when atom/molecule is bigger
halogens

Dipole Dipole:
occurs between molecules that have a permanent dipole from it being polar
polar when electron distribution is asymmetrical
similar to LDFs, but dipoles are permanent and forces are stronger
stronger when substance is more polar
Hydrogen bonding:
occurs in molecules that contain H-N, H-F, H-O bonds
difference in electronegativity is large
hydrogen is partially positive and F,O, or N is partially negative
Ion dipole:
occurs between an ion and a neutral dipole
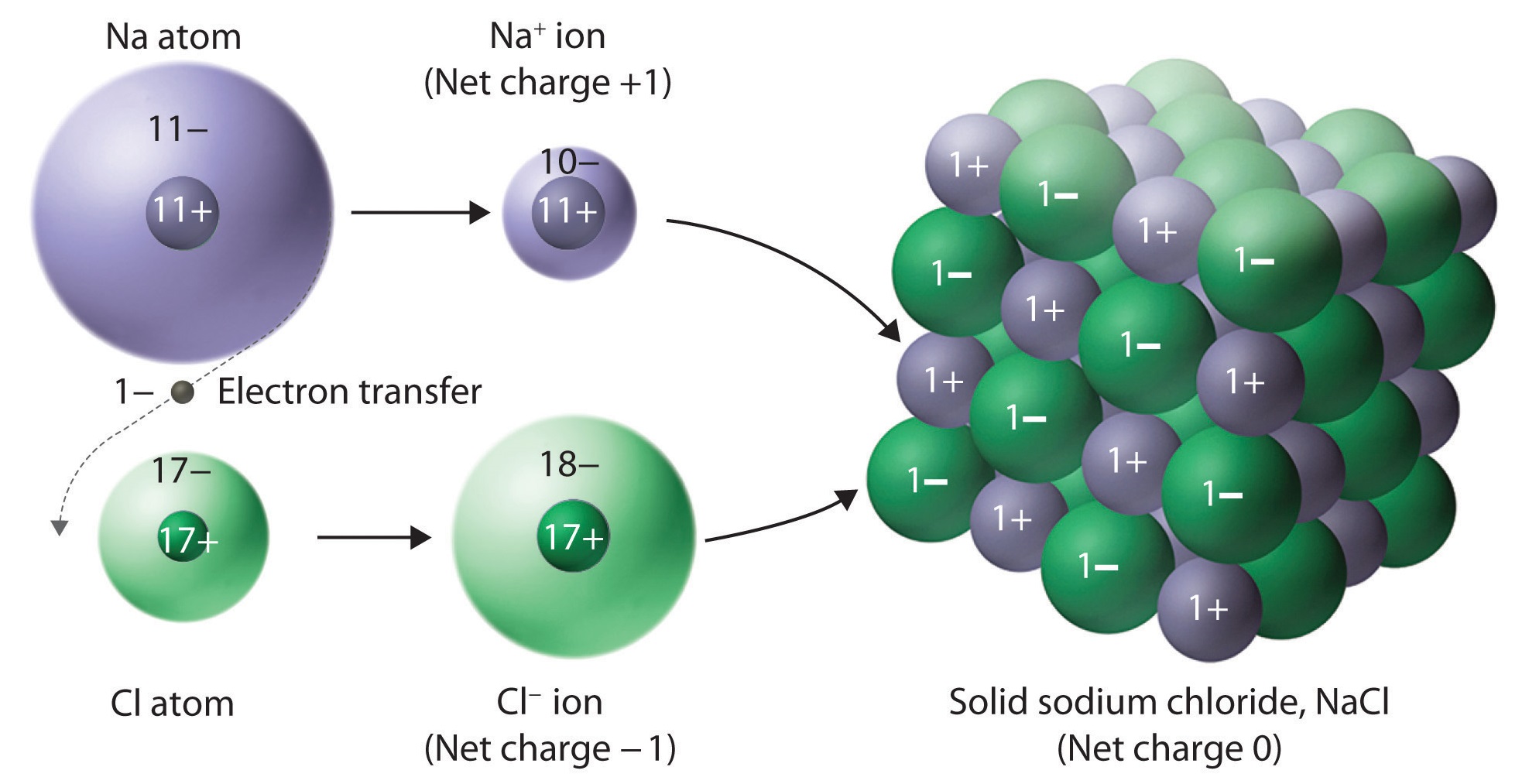
Ionic bonding:
occur between metal and nonmetal atoms
lose/gain electrons to form ions
stronger when charges are larger and ions are smaller
due to coulomb’s law
Metallic bonding:
occurs between metal atoms
attractions due to metallic cations being attracted to a delocalized sea of valence electrons
stronger with smaller cations and more valence electrons
properties as a result of IMF | effect of increasing IMF |
boiling/melting/freezing point | increase |
enthalpy of fusion/vaporization | increase |
vapor pressure | decrease |
viscosity | increase |
surface tension | increase |
solubility | decrease |
lattice energy | increase |
3.2: properties of solids
4 basic types of solids
ionic solids
covalent network solids
molecular solids
metallic solids
classified by what type of component occupies lattice points
Ionic solids:
have ions at lattice points
strong interactions between ions
low vapor pressures
high melting/boiling points
electrostatic attraction: attraction between positive and negative ions
smaller ions & ions with higher charges will have stronger attractions
results in higher lattice energy values
brittle
conduct electricity when ions are mobile
when solid is melted or dissolved
typically between metal cation and non metal anion
Covalent Network Solids:
atoms at lattice points with strong covalent bonds
only formed from non metals
high melting points
due to having to break covalent bonds
rigid and brittle
Molecular solids:
composed of distinct, individual units of covalently bonded molecules attracted through weak IMFs
have molecules at lattice points
molecules at lattice points composed of non polars
low melting point
due to weak IMFs
do not conduct electricity
valence electrons rightly held within covalent bonds
Metallic solids:
consist of metallic crystals w/ spherical metal atoms packed together
closed packed lattice of ions surrounded by sea of moving electrons
movement of electrons allow good conductivity
malleable & ductile
Metal alloys: mixture of metals
keep a sea of electrons so they can conduct
Substitutional alloy
atoms of similar sizes
density falls between 2 metals
Interstitial alloy
smaller atoms fill space between larger atoms
more rigid
strengths:
molecular solids<ionic solids~=metallic solids<covalent solids
3.3: solids, liquids & gases
particulate diagrams can be used to show the different properties between solids, liquids, and gases
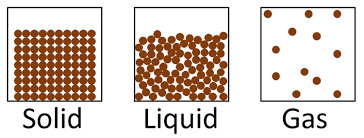
solids:
particles don’t have enough energy to move freely
vibrating
little space between particles
IMFs hold particles in place
rigid, fixed shape & volume
cannot be compressed
location can be crystalline or amorphous
crystalline solids have repeating 3D structure
amorphous have disordered particles
liquids:
particles close together
move around a tiny bit
cannot be compressed
temp. range determined by IMF
gases:
gained enough energy to overcome the IMFs holding particles
particles far apart and moving quickly
easily compressed
takes shape of container
no regular arrangement of particles
3.4: ideal gas law
law | relationship | formula |
boyle’s law | as gas pressure increases, the gas volume increases | P1V1=P2V2 |
charles’s law | as the temperature decreases, the volume decreases | V1/N1=V2/N2 |
avogadro’s law | as the # of moles of gas increase, the volume increases | V1/N1=V2/N2 |
ideal gas law: PV = nRT
P = pressure in atm
V = volume in L
n = moles of gas
R = universal gas constant(0.08206 L atm/mol K)
T = temperature in kelvin(celsius + 273)
molar mass(g/mol) = (density(g/L)*R*temp(kelvin))/pressure
dalton’s law of partial pressure: the sum of all the partial pressures of each gas in a mixture of gases is equal to the total pressure
when gases collected “over water”
mole fraction: XA = moles A/total moles
partial pressure A = XA * total pressure
3.5: kinetic molecular theory
kinetic molecular theory(KMT): theoretical model that describes the nature of ideal gases
the volume of gas particles can be ignored because they are so small
gas particles are in constant, random motions
particles are assumed to have no attractive/repulsive forces between them(IMFs can be ignored)
average kinetic energy of a sample of a gas is proportional to the kelvin temp. of the gas(KE = 1/2mv²)
maxwell boltzmann distribution: shows the distribution of the kinetic energies of particles at a given temp.
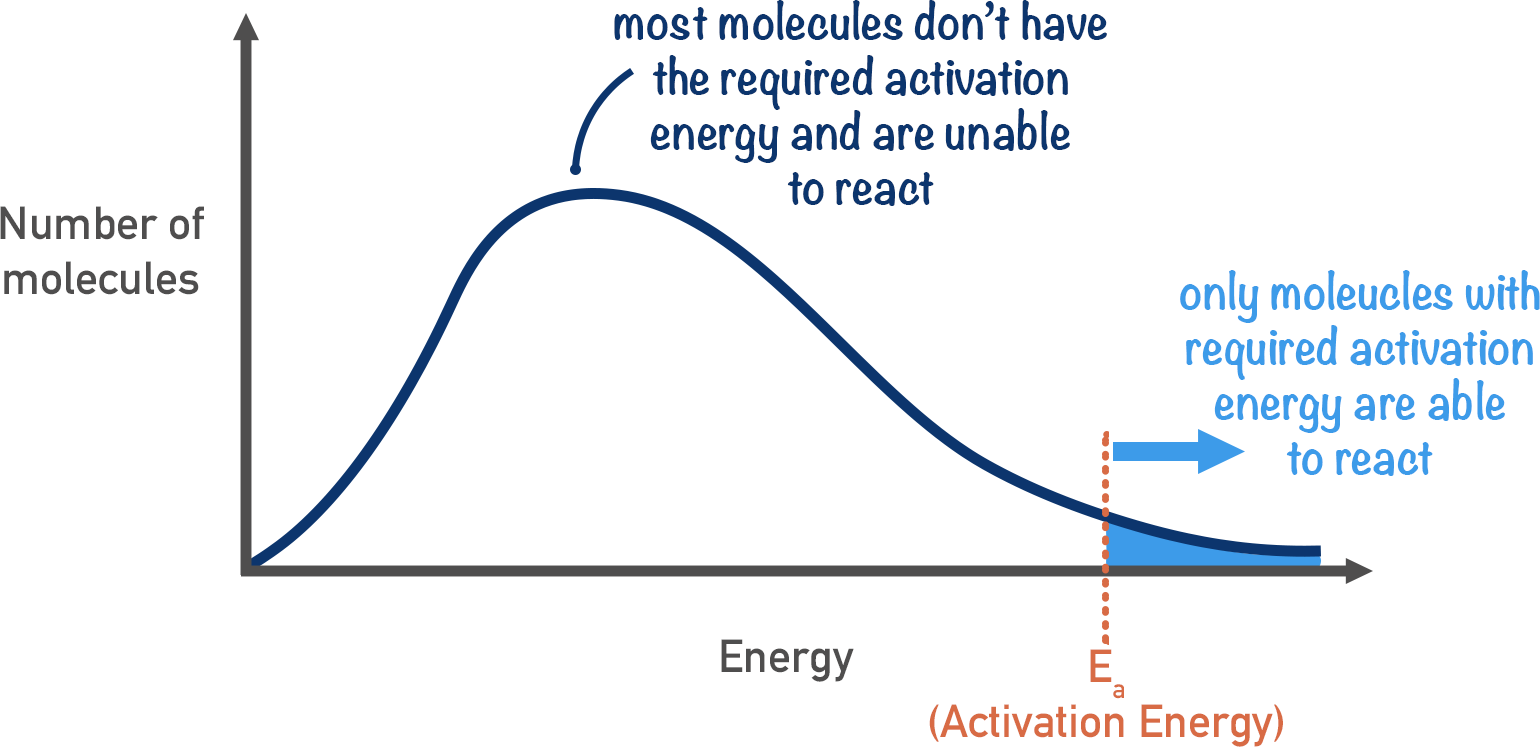
graham’s law: rate 1/ rate 2 = sqrt(molar mass 1/ molar mass 2)
3.6: deviations from ideal gas law
gases behave ideally under ordinary conditions-high temp. & low pressure
KMT assumes volumes of gas molecules are insignificant
under high temp. they are moving quickly & under low pressure they are distant from each other
not all real gases behave ideally at high pressures & low temps
when pressure is increased the particles are closer together
volume of gas molecules are significant
as temp. decreases the particles move slower
IMFs become significant
low temp
molecules move slower & have less energetic collisions
“clump” together more
collide with container less
decreases pressure
non zero molecular volume makes the actual volume greater than predicted
intermolecular attractions make the pressure less than predicted