PY 131 Chapter 20: Sound
Sound
In both fluids and solids (collectively known as bodies) there are internal forces acting between the 'elements' of the body. These internal forces are known as stress.
- Stress is the average force per unit area that an element of a body exerts on an adjacent element across an imaginary surface that separates them.
In general, the stress across the surface between two elements may be in any direction relative to the normal of the surface.
- Stress is divided into normal stress and shear stress.
In a fluid, the normal stress is the pressure.
Sound is a wave of stress in a body that acts as the medium.
In fluids sound only has a longitudinal form; in solids, it can be both longitudinal and transverse.
In a fluid, the elements move (are displaced) in the direction of the sound wave.
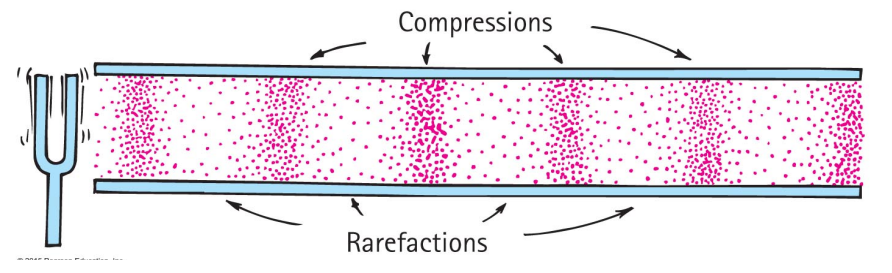
Where the fluid elements are squeezed together we find high pressure (compressions), where they have spread apart we find low pressure (rarefactions).
Musical Notes
- A musical note is a sound wave with a specific frequency.
- An octave corresponds to a doubling of frequency
- The ratio of frequencies of adjacent notes is constant, ^12√2
- The range of human hearing is ~20 Hz to ~20,000 Hz.
- Other animals can hear sounds with frequencies well below (elephants, whales) and well above (dogs, bats) this range.
- Sound waves with frequencies below 20 Hz are called subsonic, and sound waves with frequencies above 20,000 Hz are called ultrasonic.
- In general, the speed of sound in a solid is higher than in a liquid which is higher than in a gas.
- At a given P and T, less dense gases have higher sound speeds.
- The speed of sound in gas increases with P and T.
- If an object travels faster than the speed of sound in a medium its speed is described as supersonic.
EXAMPLE 1:
How do the frequency and wavelength of a sound wave change when it passes from air into water?
- ==Increase in speed==
- ==Frequency stays the same==
- ==Wavelength increases==
EXAMPLE 2:
You see a flash of lightning then 2 seconds later hear the thunder. How far away did the lightning strike the ground? Note the speed of light is so high (3 x 10^8 m/s, almost a million times faster than sound) that the time for light to travel to us is negligible compared to the time for the sound.
- d= V_sound x time
- 343 m/s x 2 = 686 m or ==1/2 mile==
Beats
Interference can also occur between waves with different frequencies fA and f B. Consider two waves with different frequencies fA and fB.

The net sound wave is the sum of the two waves

The frequency difference |fA – fB| is called the beat frequency.
EXAMPLE 1:
A tuning fork produces a steady 400 Hz tone. When this tuning fork is struck and held near a vibrating guitar string, twenty beats are counted in five seconds. What are the possible frequencies produced by the guitar string?
- f_beat = 20/5 = 4 Hz.
- fbeat = |fTF - f_G)|
- fbeat = |fTF - f_G)| > 4 = |400 - x|
- 4 - 400 = x > |-396| = x OR 4 + 400 = x > |404| = x
- ==F_G = 396 OR 404==
Musical Instruments
When a musical instrument is played the instrument vibrates.
To produce a note the vibrations must be dominated by a single frequency.
The instrument – or some part of it - is designed to support standing waves.
The vibrations of the instrument cause the fluid elements of the nearby air to be displaced changing the local pressure.
The pressure disturbances then travel through the air to a detector such as your ear.
The frequencies of the sound in the air are the same as the frequencies of the vibration of the instrument.
The wavelengths of the sound in air are not, in general, the same as the wavelengths of the standing waves on the instrument.
- The speed of waves on the instrument can be different from the speed of sound in air.
Usually, several standing waves are produced when the instrument is played – the fundamental usually dominates but the overtones also appear.
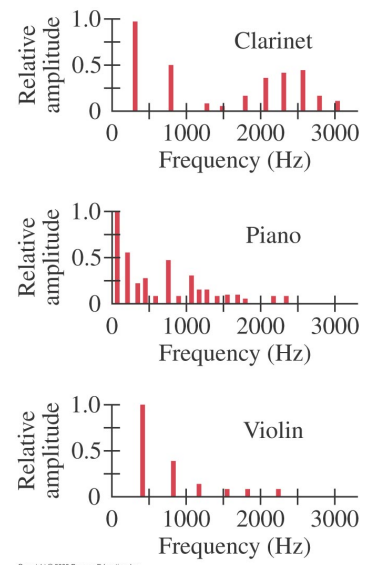
Stringed Instruments
- We have already considered the standing waves on taut strings. The ends of the string are fixed and correspond to nodes.
- The fundamental has a wavelength twice the length of the string. The overtones have wavelengths which are the integer fractions of the fundamental.
- λ1=2 L
- λn =λ1/n
- The frequency of the fundamental and the overtones are:
- f1= v / λ1
- fn =n (f1)
- The speed v is the speed of waves on the string and depends upon the tension FT in the string.
- When you tune a stringed instrument you change the tension FT
- This changes the speed v of waves on the string.
- The change in v changes the frequencies of the harmonics.
- Tuning the instrument does not change the wavelengths of the standing waves.
- When you play a stringed instrument such as a guitar or a violin you change the length of the string.
- This changes the wavelengths of the standing waves which changes the frequency of the waves.
- The speed of the waves upon the string remains the same.
Wind Instruments
- The notes from wind instruments are from standing waves of sound – air pressure/displacement - within a column of air (tube).
- The sound waves in the instrument are generated by blowing into the instrument and (usually) causing some object (reed or lips) to vibrate.
- The column of air is 3D: in order to restrict the number of standing waves produced when the instrument is played the pipe is usually long and narrow.
- There is one big difference between string and wind instruments:
- the ends of the column of air can be nodes or antinodes depending upon whether they are open or closed.
Open Tubes
For open tubes (as in a flute) the ends of the tube are nodes of pressure and antinodes of air displacement.
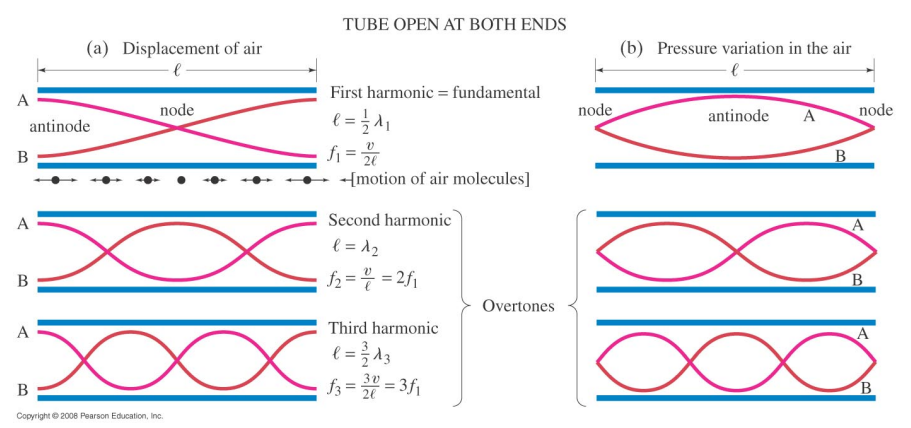
The relationship between the fundamental and the overtones is the same as for a string.
Closed Tubes
For closed tubes, the open end of the tube is a node of pressure (antinodes of displacement), and the closed end is an antinode of pressure (node of displacement).
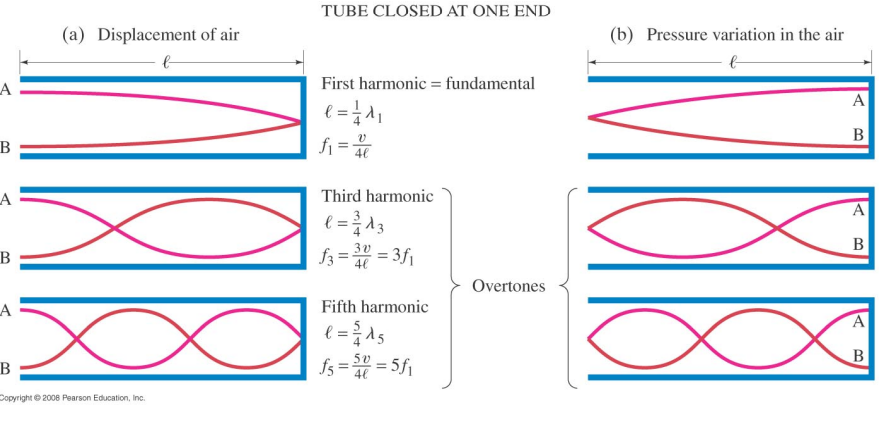
For a closed tube only the odd harmonics appear.
Organs of all types have lots of tubes (pipes) with both open and closed ends. For a flute, clarinet, trumpet, etc. the length of the air column is changed by either covering/uncovering holes or with valves.