Equilibria and acids

When ethanoic acid is dissolved in water it forms an equilibrium

• CH₃COOH(aq) ⇌ CH₃COO^-(aq) + H⁺(aq)

This can be expressed for any acid, as follows:-

• HA(aq) ⇌ H⁺(aq) + A^-(aq)

For the example of ethanoic acid

HA = CH₃COOH

A^- = CH₃COO^-

K_a

• HA(aq) ⇌ H⁺(aq) + A^-(aq)

As with any equilibrium we can derive an equilibrium constant, but instead of calling it K_c, we call it K_a

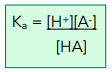
• [H⁺], [A^-], and [HA] are when at equilibrium

K_a is the acid association constant

• The larger the value of K_a, the higher the strength of the acid

• Always has units of moldm^-3

Just as we use pH to show the acidity, pH = -log[H⁺]

The huge range of K_a values mean we often use pK_a to show the strength of an acid

pK_a = -log(K_a)

Calculating the pH of weak acids

• HCOOH(aq) ⇌ HCOO^-(aq) + H⁺(aq)

Given the K_a of methanoic acid what is the pH of a 0.0500M solution?

K_a = 1.60 × 10^-4M

Firstly, assume that the [A^-] = [H⁺]

• K_a = [H⁺]² / [HA]

Secondly, since only a small proportion of the acid has dissociated we can say that [HA]_eqm = initial concentration

Rearrange the equation and substitute the values in

• 0.05 x (1.6 × 10^-4) = [H⁺] ²

Take the square root to find the pH