MOTION AND FORCES EDEXCEL
A force is either a push or a pull that one object exerts on another object.
It can produce, slow down, speed up, stop motion, or change its direction.
0. Previous knowledge
Vectors and scalars
A vector has magnitude and direction.
A scalar has just magnitude.
E.g., speed is a scalar, and velocity is a vector.
Vector diagrams
In vector diagrams, vector quantities such as forces are represented by an arrow.
The length of the arrow is proportional to the size of the force.
The direction of the arrow shows the direction of the force.
Steps to draw a vector:
1. Choose an appropriate scale
2. Measure the required angle using a protractor from the baseline/second force.
3. Draw an arrow of length proportional to the force using the scale taken.
Adding vectors
Parallel vectors
If they are in the same direction, they will be added.
If they are in opposite directions, the resultant will be in the direction of the greater force and its size will be the difference of the two vectors.
If they are equal and opposite, there will be no resultant.
Non-Parallel vectors
Tip-to-tail method: redraw one of the vectors, placing the tail of the vector at the tip of the second vector. Join the two corners to get the resultant.
Parallelogram method: complete the parallelogram, the diagonal of the parallelogram will be the resultant.
1. Movement and position
Speed
Speed is the distance travelled per unit of time.
It is a scalar.
e.g., 5 m/s.
It is always positive.
Speed \: (v) = \frac{distance \: travelled \: (d)}{time \: taken \: (t)}
Since distance is measured in meters, and time is measured in seconds, speed's unit is metres per second (m/s) or kilometres per hour (km/h).
Distance-time graphs
Distance-time graphs provide a record of how far an object has moved as time has passed.
Distance travelled is plotted on the vertical axis, and time is plotted on the horizontal axis.
The line's slope, or gradient, tells us the object's speed.
The steeper the line, the greater the speed.
Acceleration is when an object speeds up, as shown in diagram C.
Other distance-time graphs are also shown in the picture below:

Velocity
Unit: m/s (metres per second) or km/h (kilometres per hour).
Velocity is speed in a given direction, so it is a vector.
e.g., 5 m/s east.
We not only know how fast something is going but also where it's going.
It can be negative or positive.
Velocity \\: (V) = \frac{displacement \\: (d)}{time \\: taken \\: (t)}
Displacement means distance travelled in a particular direction from a specified point.
Average speed and average velocity
Speed or velocity refers to a specific time/point of a journey.
When calculating average speed/velocity, we calculate the speed/velocity of the entire journey.
This journey may include the resting time in between and any acceleration or deceleration in the journey.
Average \: speed \: (v) = \frac{distance \: moved \: (d)}{total \: time \: taken \: (t)}
Average \: velocity \: (v) = \frac{total \: displacement \: (d)}{total \: time \: taken \: (t)}
Acceleration
Acceleration = (final velocity (v) - initial velocity (u) ) / time taken (t)
It is a vector.
Acceleration is the rate of increase in velocity with time.
It is measured in m/s^2 (meters per second squared).
Deceleration means slowing down.
A negative acceleration simply means deceleration.
{(Final \: speed \: (vu))}² = {(initial \: speed \ (vi))}² + 2 * acceleration (a) * distance \: moved \: (d)
Velocity-time graphs
The gradient in the picture below is straight, which tells us that the velocity is increasing steadily.
This means that the acceleration is uniform.
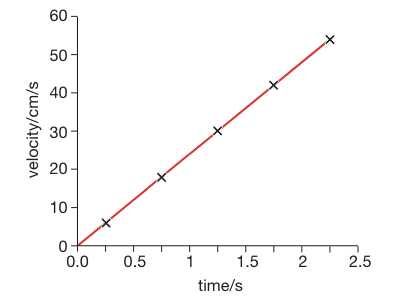
Average \: velocity \: (v) = \frac{initial \: velocity \: (vi) + final \: velocity \: (vf)}{2}
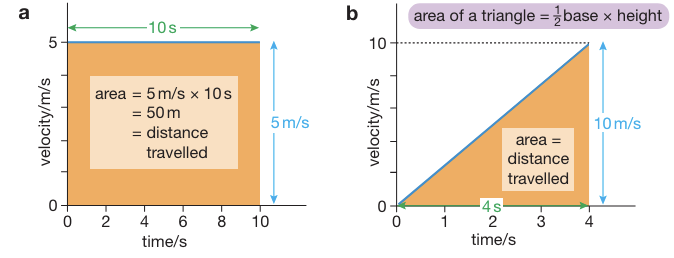
The area under a velocity-time graph is equal to the distance travelled by the object in a particular time interval.
Circular motion
When an object is travelling in a circle at constant speed, the velocity is always changing as the direction is constantly changing.
The resultant force of a circular motion is towards the center of the circle.
2. Forces and Shape
The unit used to measure force is the Newton (n).
A force of one newton will make a mass of one kilogram accelerate at one metre per second squared.
A force is a vector quantity.
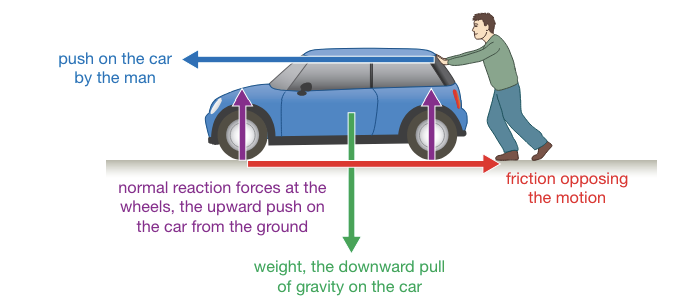
Common forces
Weight is the pull of the gravitational force on an object.
The normal force is a reaction force, the upward push on an object from the ground.
Friction is a force that opposes motion.
Balanced and unbalanced forces
The resultant force is the sum of all individual forces acting upon an object.
When the forces acting on something are balanced, the object does not change the way it is moving.
Unbalanced forces acting on an object cause it to change the way it is moving.
Inertial mass is a measure of how difficult it is to change the velocity of an object.
Inertial \: mass \: (m) = \frac{force \: (f)}{acceleration \: (a)}
Friction
Friction is the force that opposes motion between two surfaces in contact.
It causes objects moving to slow down and stop.
The kinetic energy of the moving object is transferred to heat as work is done by the friction force.
Reducing friction means that machines work more efficiently
Increasing friction helps tyres grip the road better.
Changing shapes
Sometimes, a force can make an object change shape, either permanently or temporarily.
Springs and wires
Springs are coiled lengths of certain types of metal, which can be stretched or compressed by applying a force to them.
They obey Hooke’s law, which states that the extension is directly proportional to the force causing it.
Hooke’s law only applies until the limit of proportionality, after which the object does not obey the law, and the extension is imbalanced.
A straight line on a graph of extension shows the object is obeying Hooke’s law.
If a spring is stretched beyond its elastic limit, it will not return to its starting length.

The extension doubles if two springs are connected to each other directly.
The extension halves a load attached to two springs in parallel as the load is shared.
Force \: (f) = mass\:(m)*acceleration\:(a)
Elastic bands
Elastic bands are another example of changing objects.
They do not follow Hooke’s law.

3. Forces and movement
Force, mass, and acceleration
The acceleration of an object depends on the size of the unbalanced force and the mass of the object.
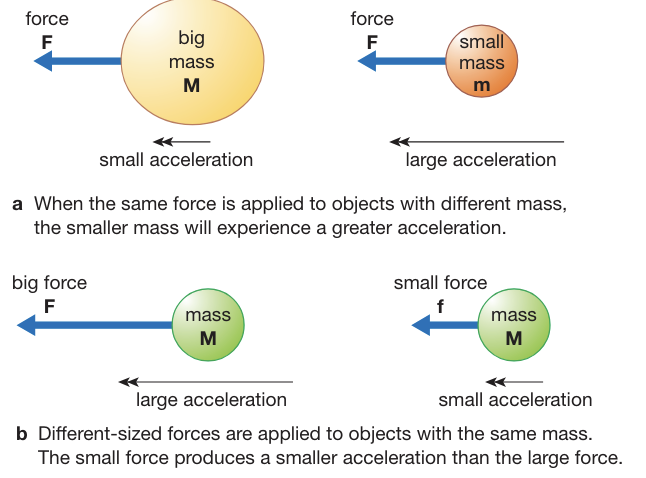
Acceleration is inversely proportional to mass.
Force \: (f) = mass\:(m)*acceleration\:(a)
Human Reaction Time
Human reaction time is the time taken between a stimulus being presented and the initiation of a response by an individual.
The average human reaction time is 0.25 seconds.
Factors Affecting Reaction Time:
Age and health of the individual.
State of alertness and concentration.
Influence of drugs or alcohol.
Nature of the stimulus (visual, auditory, etc.).
Stopping Distance
Stopping distance is the total distance covered by a vehicle from the moment the driver perceives a hazard until the vehicle comes to a complete stop.
Components of stopping distance:
Thinking Distance: The distance traveled by the vehicle during the driver's reaction time.
Braking Distance: The distance traveled by the vehicle while the brakes are applied and the vehicle decelerates to a stop.
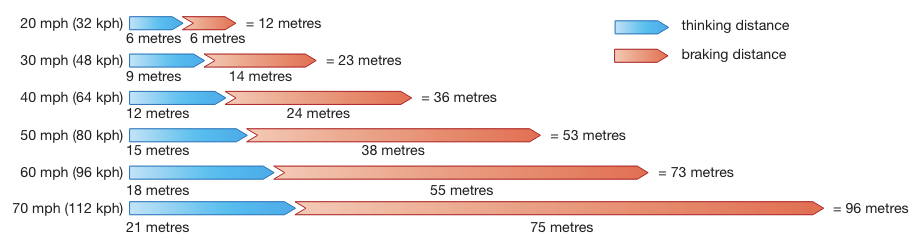
Factors affecting stopping distance:
Speed of the vehicle.
Road surface conditions (dry, wet, icy).
Condition of tires and brakes.
Reaction time of the driver.
Weight
The weight of an object is the force that acts on it because of gravity.
The gravitational field strength is the force that acts on each kilogram of mass.
Weight \: (w) = mass\:(m)*gravity\:(g)
Gravity on Earth is approximately 9.81N.
Air resistance and terminal velocity
Air resistance, or drag, is a frictional force that opposes motion in air. It slows down anything travelling through the air and may also cause the object to heat up.
The drag coefficient is a measure of how easily an object moves through the air.
Terminal velocity is when the drag force has increased to the point where it exactly balances the weight force.
Since there is now no unbalanced force on the object its acceleration is also zero.
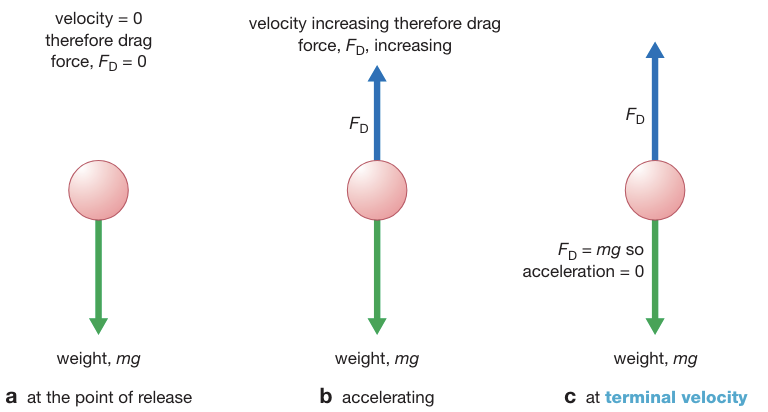
Work
The work done to stop an object is equal to the initial kinetic energy of the object.
KE=Fd=\frac{1}{2} mu²
4. Momentum
Momentum is a measure of how difficult it is to stop something that is moving.
Momentum \: (p) = mass\:(m)*velocity\:(v)
It is a vector quantity, measured in kilogram metres per second (kg m/s).
Momentum and acceleration
The rate of change of momentum of an object is proportional to the force applied to the object.
Force \: (F) = \frac{final \: momentum \: (mf) \: - \: initial \: momentum \: (mi)}{time \: taken \: (t)}
Momentum and collisions
The total momentum of objects that collide remains the same.
Newton’s Laws of Motion
Newton's first law
An object at rest remains at rest, and an object in motion remains in motion at constant speed and in a straight line unless acted on by an unbalanced force.
Newton's second law
When a resultant force acts on an object of a constant mass, the object will accelerate in the direction of the resultant force.
The product of the mass and acceleration of the object gives the resultant force.
Force \: (f) = mass\:(m)*acceleration\:(a)
Newton's third law
Every action has an equal and opposite reaction.
F₁=F₂
mass_1 * acceleration_1 = mass_2 * acceleration_2
⠀
5. The turning effect of forces
The moment of a force
A moment is the turning effect of a force.
They occur when a force causes an object to rotate about a pivot or hinge.
The size of the moment is determined by the size of the force and the perpendicular distance from the pivot.
Moment \: (M) = force \: (f) * perpendicular\:distance\:from\:pivot\:(d)
The unit of moments is Newton Metres (Nm).
Opening or closing a door is an example of a moment.
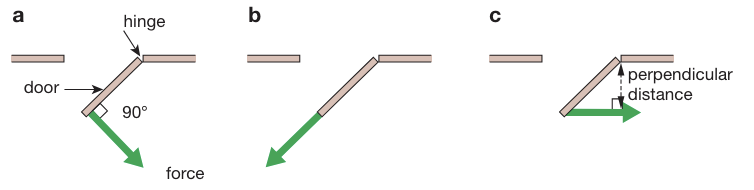
Principle of Moments
The Principle of Moments states that for an object to be balanced, the clockwise moment must be equal to the anticlockwise moment.
F_1 * D_1 = F_2 * D_2
Centre of Mass and Stability
The centre of mass of an object is the point at which the weight of the object acts.
For symmetrical objects, the centre of mass is at the point of intersection of its symmetries.
When an object is suspended, its centre of mass will come below it.
To find the centre of mass of a lamina, it is suspended from a point and a plumb line is hung next to it.
A line is drawn using a pencil along the plumb line.
This is repeated from multiple points, the point of intersection of the lines is the centre of mass.
An object is stable when its centre of mass is above its base.
If the centre of mass is not directly above its base, the object will topple over.
The lower the centre of mass of an object, the greater the stability.
The greater the base area of an object, the greater the stability.
 Knowt
Knowt
