PHYSICS I
Introduction to Physics
Physics deals with the interaction of matter, force, and energy.
Classical Physics (Pre-1900)
Mechanics - area of physics concerned with the relationships between force, matter, and motion among physical objects
Heat and Thermodynamics - it studies the relations between heat, work, temperature, and energy.
Optics - it studies how light moves through certain objects.
Electricity and Magnetism - it studies the relationship between electric currents and magnets
Wave Motion and Sounds - it studies how energy moves through air or water in the form of vibrations and sound
Modern Physics (Post-1900)
Nuclear Physics - it studies the interactions of atomic nuclei and it’s constituents
General Relativity - It describes how gravity is a geometric product of space and time
Special Relativity - explains how speed, mass, time, and space are all interrelated.
Quantum Mechanics - Quantum mechanics is the field of physics that explains how extremely small objects simultaneously have the characteristics of both particles
Particle Physics - is the study of fundamental particles and forces that constitute matter and radiation. The field also studies combinations of elementary particles up to the scale of protons and neutrons.
Measurement is the process of comparing something with standard.
There are two systems of measurement
Metric System (MKS and CGS)
English or Imperial System (FPS)
Physical Quantities
Fundamental units/quantities - units independent of one another.
Ex. Mass, amount of matter in an object.
Length, how long an object is
Derived Units/quantities - combination of fundamental units
Ex. Weight changes based on factors like density, gravity, and mass
Density changes based on an objects mass and volume, the more mass in a small space, the more dense. Or the less mass in a large space, the less dense it is.
Scientific Notation
is a conveniently and widely used method of expressing large and small numbers.
Ex. 5,000,000,000 = 5.00 × 109
0.000000005 = 5.00 × 10-9
Unit Conversion
a. 55 km to m
55 km x (1000 m / 1 km) = 55, 000 m
b. 12g to kg
12 kg x (1 kg / 1000 g) = 0.012 kg
c. 85 m/s to kph
85 m/s x (1 km / 1000 m) x (3600 s / 1 hr) = 306 kph
Uncertainty and Errors
Measurements always have some degree of uncertainty because of unavoidable errors.
Error is the deviation of a measured value from the expected or true value.
Uncertainty is a way of expressing this error.
Measured Value = True Value ± Uncertainty
Precision - closeness of a measurement to another measurement.
Accuracy - closeness of a measurement to the expected value of a physical quantity
Random Error - Results from unpredictable or inevitable changes during data measurement.
Example: When weighing a substance, the scale may show slightly different readings each time due to minor vibrations, air currents, or electrical interference, even if the substance's mass hasn't changed.
Systematic Errors - usually come from the measurement or in the design of the experiment itself.
Example: When measuring the length of a table multiple times with a ruler that is incorrectly marked, each measurement yields a consistent discrepancy. For instance, if the ruler is off by 1 cm, every measurement will be 1 cm shorter than the actual length, leading to biased results.
Percent Error = (| x - xr | / xr) x 100
xr = true or accepted value
x = measured value
Percent Difference = (|x1 - x2| / x1 + x2 / 2) × 100
Variance - measures the squared deviation of each number in the set from the mean.
Mean (μ) = (Σx) / N
Σx = sum of all values
N = number of values.
Variance (σ²) = Σ (xi - μ)² / N
σ² = variance
xi = each value
μ = mean
N = number of values
Standard Deviation (σ) = √(Σ(xi - μ)² / N)
Uncertainty indicates the range of values within which the measurement is asserted to lie with some level of confidence
Absolute Uncertainty = ± (Maximum Value - Minimum Value) / 2
Ex. Resistance of a wire 25.00 Ohm ± 0.05 Ohm
Range of Resistance 24.95 - 25.05 Ohm
25.05 - 24.95 / 2
= 0.05
Relative Uncertainty = (Absolute Uncertainty / Measured Value) × 100%
Ex. 0.05 Ohm / 25.00 Ohm x 100 = 0.2%
A zero variance means that all measurements are identical
Less variance means more precision
Standard Deviation is how spread out measurements from the average
Least Count refers to the smallest value that can be read from any measuring device.
Ex. Rulers in metric usually have 0.1 cm as the Least Count
Rule 1: If data are to be added, add their absolute uncertainties.
Ex. Perimeter = sum of all the sides = 2(L +W)
= 2(6.5m ± 0.1m + 3.4m ± 0.2m)
= 2(9.9m ± 0.3m)
= 19.8m
Rule 2: If data are to be multiplied or divided, add their relative uncertainties.
Ex. The area of a rectangle = L x W
Relative uncertainty for length = 1.54%
Relative uncertainty for width = 5.88%
Sum = 7.42%
= ((7.42%)(22.1m2) / 100%) = 1.64m2 - absolute uncertainty.
Vector Addition
Graphical - associated with illustrations, drawings, and pictures
Analytical - associated with computation and analysis
Scalar Quantity - Magnitude
From the Latin word Scala - “steps or ladders”
Vector Quantity - Magnitude + Direction
From the Latin word Veherre - “To Carry”
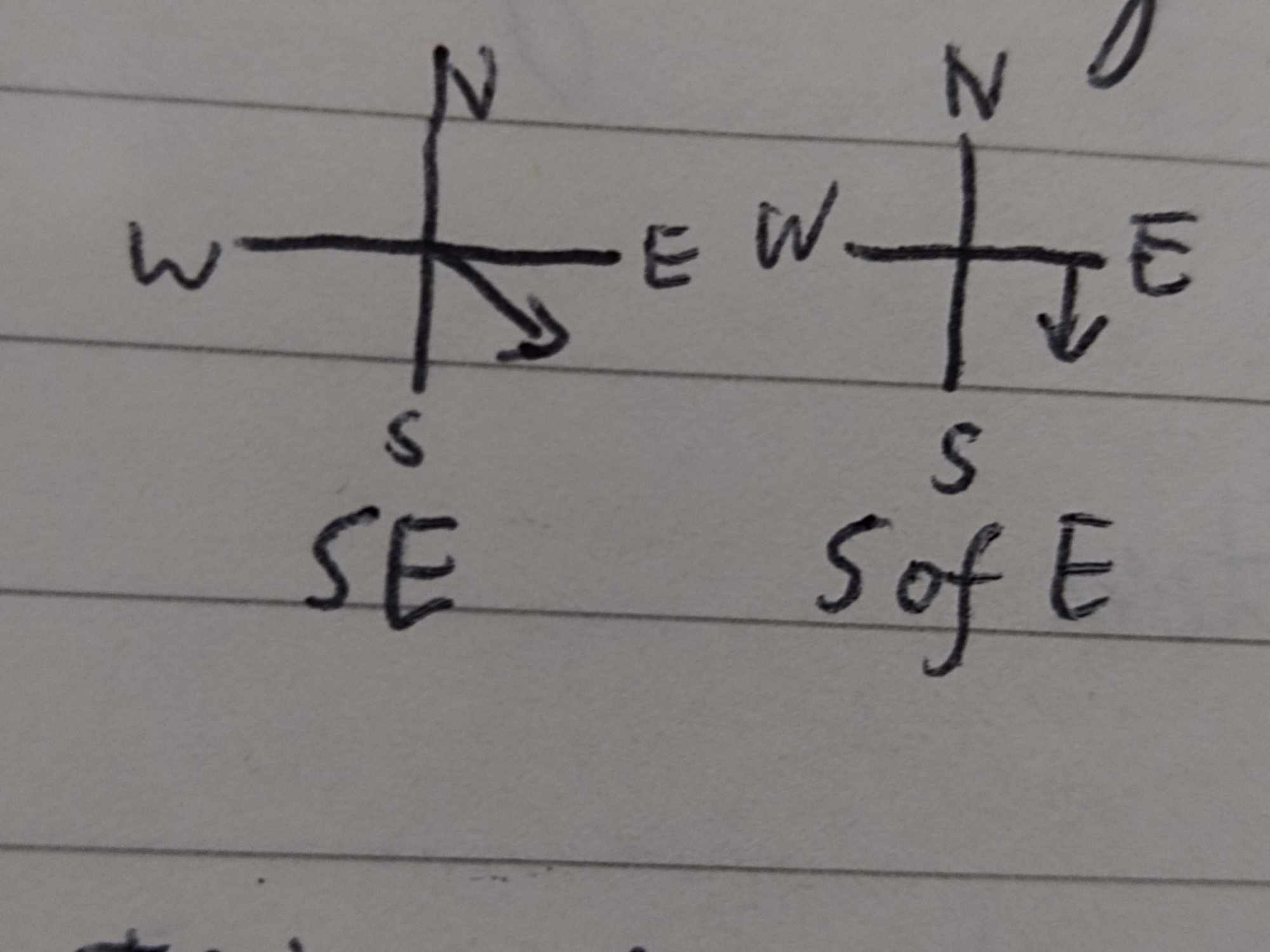
Things to consider when adding vectors:
Magnitude and direction
The term used to describe the sum of two or more vectors is called Resultant Vector or Vr
Vector addition has two properties:
Commutative - The order of adding vectors maybe changed without affecting the resultant. This property can be represented as A + B = B + B
Associative - when the addition of three or more numbers, the total/sum will be the same, even when the grouping of addends are changed. This property can be represented as; A+(B+C) = (A+B)+CT
The Graphical Methods:
Parallelogram Method - This method is useful for getting the resultant of two vectors, but cannot be applied beyond two vectors.
STEPS:
Using a suitable scale, draw the arrows representing the vectors from a common point.
Construct a parallelogram using the Two vectors.
Draw diagonal, this represents the resultant.
Measure the length of the diagonal, from the scale used.
Determine the direction using a protractor.
Head-to-Tail Method - This method is quite simple and useful, as it can be used for 3 or more vectors.
The Analytical Methods:
Component Method
Sine-Cosine Method
Component Method Example 1:
d1 = 10m, E
d2 = 15m, 30o N of E
d1x = 10m
d1y = 0m
d2x = (cos(30o))(15) = 13m
d2y = (sin(30o))(15) = 7.5m
Σx = 10m + 13m = 23m
Σy = 0 + 7.5m = 7.5m
dR = √((Σx)2 + (Σy)2)
dR = √((23m)2 + (7.5m)2)
dR = 24.18m
θ = tan-1[7.5m / 23m]
θ = 18.06o N of E
dR = 24.18m 18.07o N of E
Component Method Example 2:
d1 = 5 m, S
d2 = 12 m, E
d3 = 4 m, SW
d4 = 6 m, W
d1x = 0
d1y = -5 m
d2x = 12 m
d2y = 0
d3x = (cos 45o)(4 m)
d3y = (sin 45o)(4 m)
d4x = -6m
d4y = 0
Σx = 3.17m
Σy = -7.83m
dR = √((3.17m)2 + (-7.83m)2)
= 8.45m
θ = tan-1[7.83m / 3.17m]
= 67.96o S of E
dR = 8.45m, 67.96o S of E
Kinematic Equation of Motion
Motion - change in position in respect to the observer
Position - refers to the location of an object with respect to a reference point or origin.
Study of motion is divided into two:
Kinematics - describes motion in terms of displacement, velocity, and acceleration.
Dynamics - the study of force in relation to motion
Distance - refers to total length of path by any object in moving from it’s initial position
Displacement - is defined as the change in position of an object in relation to it’s starting position.
Ex. Andee jogged 12 times around a circular track of radius 5m. Find the total distance & displacement
c = 2πr
c = 2π(5) = 31.4 m
distance = 31.4 m × 12 = 377
displacement = 0
Speed = rate of change of distance
s = d / t
Velocity = rate of change of displacement
v = s / t
Average Speed - total distance traveled by the total time elapsed
savg = dt / tT
Instantaneous Speed - The recorded speed at a particular moment in time.
Average Velocity - displacement divided by the total time elapsed.
vavg = Δx / Δt = x - x0 / t - t0
Δx = change in position
Δt = change in time.
Instantaneous Velocity - Velocity at a specific point in time.
vins = lim(Δt → 0) (Δx/Δt)
Accelerations exists when there is a change in velocity.
a = v - u / t
u = initial velocity
v = find velocity
t = time
Instant Acceleration
ains = lim(Δt → 0) (Δv/Δt)
Example 1:
There is a 360 m long path, V starts walking at a rate of 3 m/s, A starts walking 10 s after at a rate of 4 m/s. How long will it take for A to overtake V?
VV = 3 m/s
VA = 4 m/s
d - 360 m
10 s
dv = da
3(t + 10) = 4t
3t + 30 = 4t
30 = 4t - 3t
t = 30 s
How far is V, when overtaken by A?
dA = 4t = 4(30) = 120
360 - 120 = 240 m
Example 2:
s(t) = -2t3 + 13t2, where s in meters and t in seconds.
a. find the average velocity from t = 4 s to t = 6 s.
s(4) = 80 m
s(6) = 36 m
vavg = 36 m/s - 80 m/s / 6 s - 4 s
vavg = 22 m/s
b. Find the instantaneous velocity at t = 4
vins = -6t2 + 26t
= -6(4)2 + 26(4)
= -6(16) + 104
= -96 + 104
vins = 8 m/s
Example 3:
Lean and MIckey are 150 m apart. They start walking at 3 m/s and 5 m/s, respectively.
Lean represents x / 3, Mickey represents 150 - x / 5
a. How long will it takes for them to meet?
ti = tm = x / 3 = 56.25 m / 3 m/s = 18.75 s
b. Find the corresponding distance traveled by each?
ti = tm
x / 3 = 150 - x / 5
5x = 3(150 - x)
5x = 450 - 3x
x = 450 / 8
x = 56.25 m
Example 4:
A particle moves along the x-axis so that it’s position at any time t greater than or equal to 0 is given by the function x(t) = t3 - 12t + 1, where x is measured in terms of feet, and t in seconds.
a. Find the displacement during t = 4
x(4) = 43 - 12(4) + 1
x(4) = 17 ft
b. Find the average velocity of the particle for 4 less than or equal to t less than or equal to 6.
t(4) = 17 ft
t(6) = 145 ft
vavg = 145 ft - 17 ft / 6 ft - 4 ft
vavg = 64 fps
c. Find Instantaneous Velocity at 3 s
d’(t) = 3t2 - 12
x(3) = 3(3)2 - 12
= 27 - 12
= 15 fps
Example 5:
The position of an object as a function of time t is given by x(t) = 3.5t3 - 2t + 5, where x is measured in meters and t in seconds.
a. Give the velocity and acceleration as a function of time.
Vins = 10.5(2)2 - 2
= 40 m/s
ains = 21(2)
= 42 m/s
Example 6:
The position, in meters, of a body at time t seconds is x(t) = t3 - 6t2 + 9t. Find the body’s acceleration each time the velocity is zero.
d(x) / t = 3t2 - 12t + 9 —> 3t2 / 3 - 12t / 3 + 9 /3 —> t2 - 4t + 3 = 0
(t - 1)(t - 3) = 0
t = 1, t = 3
d(x) / t = 6t - 12
t = 1 —> 6(1) - 12 = - 6 m/s2
t = 3 —> 6(3) - 12 = 6 m/s2
Free Fall
When the only force acting on an object is gravity, the object is said to be in free fall thus, acceleration is constant.
In a vacuum, all objects in free fall accelerate at the same rate, regardless of mass.
Newton’s Law of Gravitation
Characteristics of Free Fall
Free-falling objects do not encounter air resistance.
All free-falling (on Earth) accelerate downwards at a rate of 9.8 m/s2
Forward and Downward Motion
At the object’s maximum height, upward velocity and downward velocity are both equal to zero.
Terminal Velocity
When an object’s weight due to gravity is balanced with air resistance, it stops it’s acceleration and starts moving at a constant speed.
Equations for Uniformly Accelerated Motion
VF = Vi + gt
d = Vi t+ (1/2)gt2
VF2 = Vi2 + 2gd
VF = Final Velocity
Vi = Initial Velocity
g = acceleration due to gravity
d = displacement
t = time elapsed
Example 1:
Ralph dropped a stone from his bedroom window.
a. What is it’s velocity after 2 seconds
Vi = 0 Vf = ?
(0)+ (9.8m/s)(2 s)t = 2 s Vf = 19.6 m/s2
g = 9.8 m/s2
b. How far from the window has it fallen after 2 seconds?
d =
(0)(2 s)+ (1/2)(9.8 m/s2)(2 s)2d = 19.6 m from the window.
Example 2:
Paul threw a ball vertically upward from the ground. The ball returned to the ground in 4 s.
a. How high did the ball rise?
d =
(0)(2 s)+ 1/2(9.8 m/s2)(2 s)2
b. With what velocity was it thrown?
0 = Vi + (-9.8 m/s2)(2 s)
0 = Vi - 19.6 m/s2
Vi = 19.6 m/s2
Example 3:
If a body falls freely from rest, how far does it move during the twelfth second?
d =
(0)(12)+ (1/2)(9.8 m/s2)(12 s)2d = 705.6 m
Example 4:
A person throws an object into the air with an initial velocity 4.9 m/s off an 52 m tall building.
a. max. height relative to the ground.
Vf = Vi2 + 2gd
0 = (4.9 m/s2) + 2(-9.8 m/s2)d
0 = 24.01 m/s2 - (19.6 m/s2)d
(19.6 m/s2)d /19.6 m/s2= 24.01 m/s2 / 19.6 m/s2d = 1.225 m
dT = 52 m + 1.225 m
dT = 53.225 m
b. It’s time of flight or the total time in the air.
Vf = Vi + gt
0 = 4.9 m/s + (-9.8 m/s2)t
(9.8 m/s2)t /9.8 m/s2= 4.9 m/s / 9.8 m/s2t = 0.5 s
d =
Vit+ (1/2)gt253.225 = 1/2(9.8 m/s2)t2
√((53.225 m)/4.9 m/s2) =
(4.9 m/s2)√t2 /4.9 m/s2t2 = 3.3 s
tT = 3.3 s + 0.5 s =3.8 s
c. Velocity before it reaches the ground.
VF = 0 + (9.8 m/s2)(3.3 s)
VF = 32.34 m/s
Vt = 42.14 m/s
Projectile Motion
Projectile - any object upon which the only force acting is gravity.
A horizontally launched will reach the ground at the same as an object as an object dropped vertically from rest. The initial horizontal velocity is irrelevant.
All projectiles follow a parabolic path called a trajectory.
Horizontal Distance (dx) = Vxt
Vx = Horizontal Velocity
t = time
Vertical Distance (dy) = (1/2)gt2
g = acceleration due to gravity
t = time
Horizontal Velocity (vx) = constant
Vertical Velocity (vy) = gt
g = acceleration due to gravity
t = time
Time (t) = √(2dy / g)
dy = Vertical Distance
g = acceleration due to gravity
Actual Velocity (v) = √((vx)2 + (vy)2)
vx = Horizontal Velocity
vy = Vertical Velocity
Projectile Motion with an Angle
Initial Velocity X (vxi) = vi cos θ
vi = Initial Velocity
θ = Angle
Initial Velocity Y (vyi) = vi sin θ
vi = Initial Velocity
θ = Angle
Actual Final Velocity (v) = √((vxf)2 + (vyf)2)
vxf = Final Horizontal Velocity
vyf = Final Vertical Velocity
Distance X (dx) = vxit
vxi = Initial Horizontal Velocity
t = time
Maximum Height (hmax) = (2visin θ)2 / 2g
vi = Initial Velocity
θ = Angle
g = gravity
Time (t) = 2(vi sin θ) / g
vi = Initial Velocity
θ = Angle
g = gravity