Chapter 1-Conics

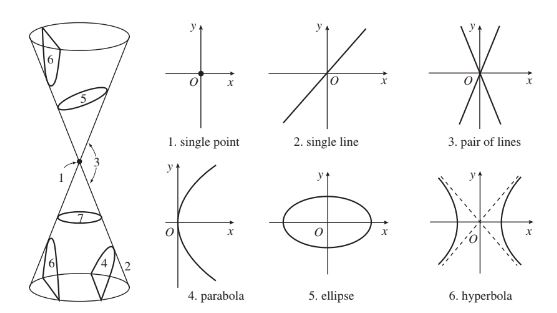
# 1.1 **Conic section**- Shapes that are obtained by taking different plane slices through a __double cone__ __Menaechmus__ (Greek mathematician) discovered conic sections around __350 BC__  * Slice 7 is considered an ellipse * **Ellipse-** An area where the sum of the distances from 2 fixed points on the plane remain constant * The __ellipse__ and the __hyperbola__ both have a **centre** * **Non-degenerate conics**- conics that are __parabolas, ellipses, or hyperbolas__ * **Degenerate**- A single point, line, and pair of lines ## 1.1.2 Circles * **Circle**- a __type of conic,__ a set of points that lie at a fixed distance (radius), from a fixed point (centre) * Use the techniques of coordinate geometry to find the __equation of a circle__ with given centre and radius. * **Theorem 1**: The equation of a circle with centre (a,b) and radius r is (x-a)^2 + (y-b)^2 = r^2 * Why? * This is a variation of the distance formula (d= (x1-x2)^2 + (y1-y2)^2) ### Example Problems Write the equation for the given centre and radius (a) centre the origin, radius 1 (1)^2 - (x-0)^2 + (y-0)^2 1= x^2 + y^2 **x^2 + y^2 -1 = 0** (b) centre (3, 4), radius 3 (3)^2 = (x-3)^2 + (y-4)^2 9= (x-3)(x-3)+ (y-4)(y-4) 9 = x^2 + y^2 -6x -8y + 25 **x^2 + y^2 -6x -8y +16=0** Determine the condition on the numbers f, g, and h in the equation x^2 + y^2 + fx + gy + h = 0 for the equation to pass through the __origin__ ### **Explanation:** * This is the __factored/ distributed form__ of our original equation * We have to find the state of the equation when **a and b equal zero**…. (x-0)^2 and (y-0)^2 * We can factor our this form to see the conditions * This would mean that **f,g, and h all must equal 0** (c) Consider the set of points (x,y) that satisfy the equation: x^2 + y^2 -4x +6y +9 = 0 1. Our goal is to complete the square (note that the coefficients on x^2 and y^2 are both 1) 2. Re-write equations containing just the x-terms, and just the y-terms x^2 − 4x = (x − 2)^2− 4 y^2 + 6y = (y + 3)^2 − 9 3. These terms can be substituted into our original equation (x-2)^2 -4 + (y+3)^2 -9 +9 = 0 (x-2)^2 + (y+3)^2 = 4 4. This means that the circle has a radius of 2 and a centre of (2, -3) **Theorem 2**: You can __complete the square__ in the equation x^2 + y^2 + fx + gy + h = 0 to put it in the form (x+1/2f)^2 + (y+1/2g)^2 = 1/4 f^2 +1/4g^2 -h This equation represents a circle with the centre (-1/2f, -1/2g) and a radius sqrt(1/4f^2 + 1/4g^2 -h) This only occurs when the 1/4f^2 + 1/4g^2 -h is greater than zero, if this is less than zero, we can prove that the figure is __not a circle__ **Why?** The equation is equal to r^2 (radius squared), when any number is squared, it has to equal a positive number. Also, when you go to take the square root of this side of the equation, it is impossible to get a rational answer when square-rooting a negative number. (d) Determine the centre and radius of the equation 3x^2 + 3y^2 − 12x − 48y = 0.  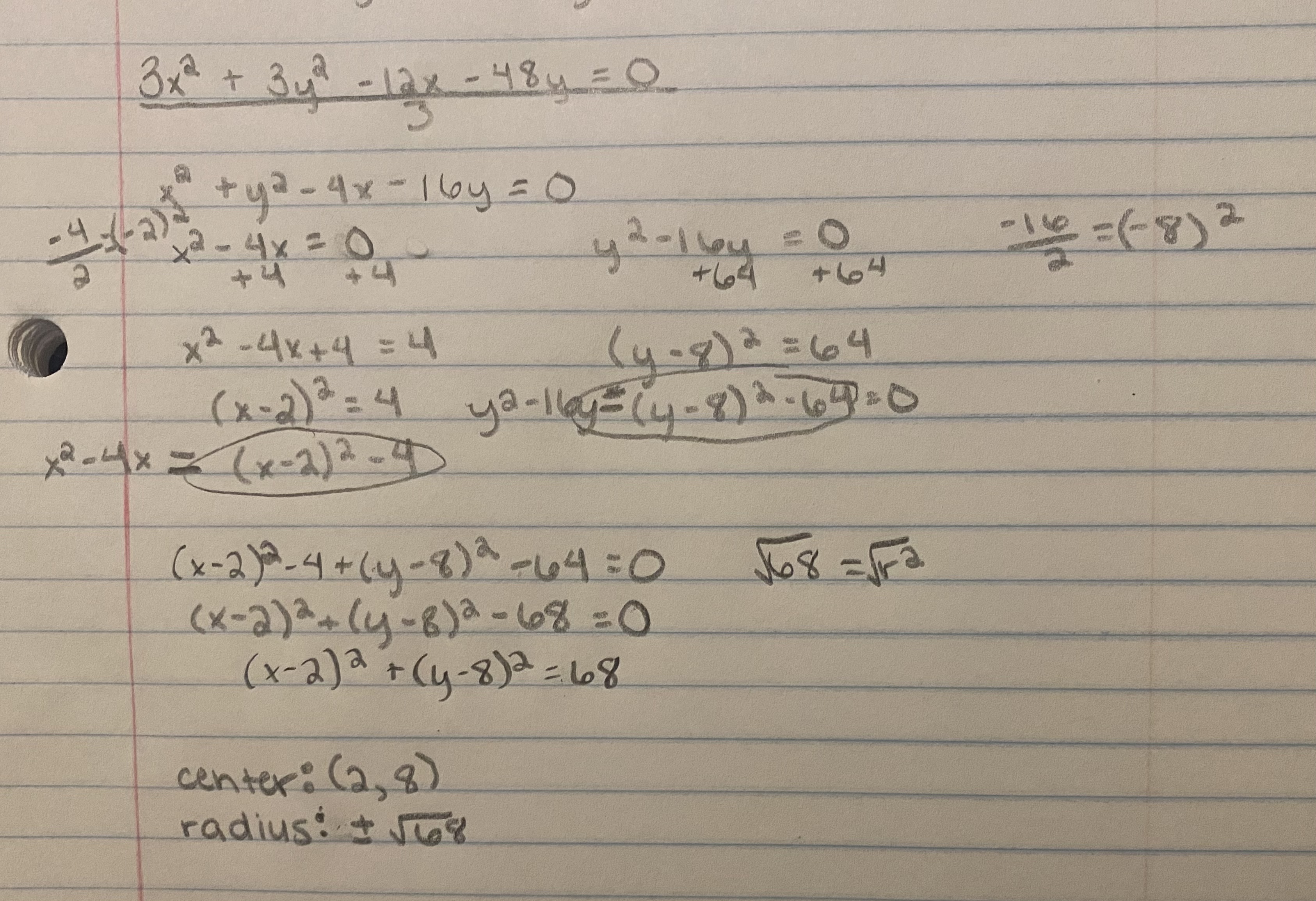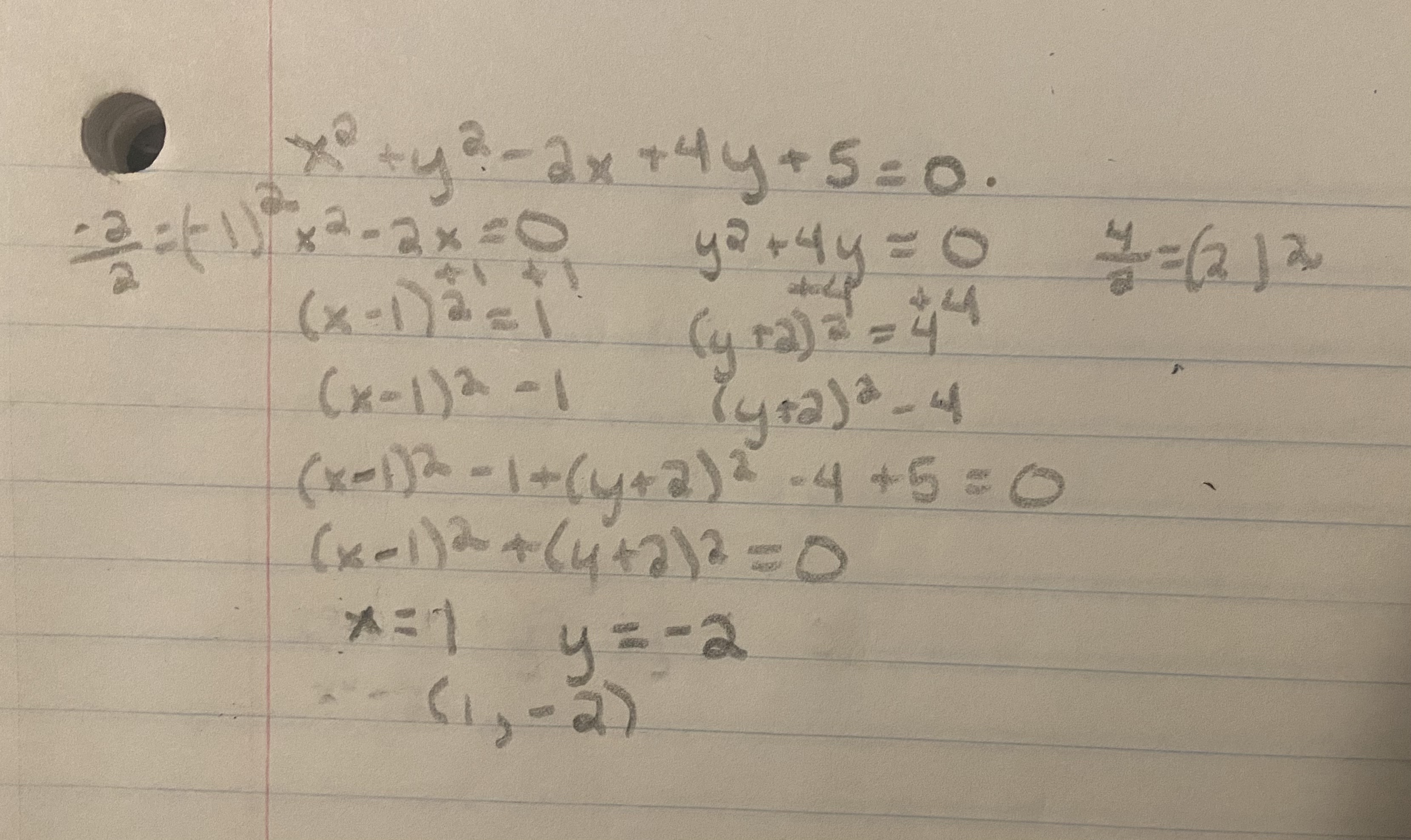(e) Determine the set of points that satisfies the following equation x^2 + y^2 − 2x + 4y + 5 = 0;  ### Orthogonal Circles Two intersecting circles that meet at __right angles__ **Theorem 3**: __Orthogonality__ test Two intersecting circles with equations x^2 + y^2 + f1x + g1 y + h1 = 0 and x^2 + y^2 + f2x + g2 y + h2 = 0, are only orthogonal if f1 f2 + g1g2 = 2(h1 + h2). Why? This can be tested with the __pythagorem theorem__, where P is a point of intersection, and A and B are points inside of each of the circles. This means that AP^2 + BP^2 = AB^2. 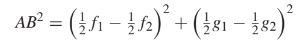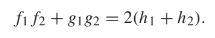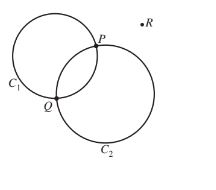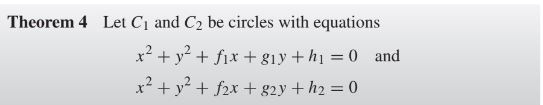Using our previous formulas, we know that  This can be solved to result in the original equation  ### Circles Through Two Points  * In this image, the circle intersects at distinct points P and Q  This equation represents a circle when k __does not__ equal -1, and this means that P and Q will satisfy the equation \   ### Explanation: These circles have distinct points of intersection, and the line containing these points can be expressed using the second equation, only when __k=-1.__ When k is any other number, this is the equation of __one of the circles__ \ ## 1.1.3 Focus-Directrix Definition of the Non-Degenerate Conics * The three __non-degenerate conics__ are the parabola, ellipse, and hyperbola * The distance of a set of points from a fixed point (**focus**) follow a constant multiple (**eccentricity)** * This distance is also followed from a line (**directrix**) * **Eccentricity**- It is an ellipse if e (eccentricity) is between 0 and 1, a parabola of e equals 1, and a hyperbola is e is greater than 1 ### Parabola (e=1) * < 0, e > 1. * Foci **(±ae, 0)** and Directrices **x = ±a/e** * Parametric equations: x = a sec t, y = b tan t (t ∈ (−π/2, π/2) ∪ (π/2, 3π/2)). * **Asymptotes-** the lines of the parabola that the branches grow closer to. They are notated as y = ±(b/a)x ### Rectangular Hyperbola * ^^e= sqrt (2)^^ * This means that e^2=2 and a=b * The __asymptotes__ have the equation **y = ±x,** so they are at __right angles__ * A hyperbola whose asymptotes are at right angles is called a __rectangular hyperbola__ * You can use the asymptotes as new __x and y axes,__ and the equation can then be written as xy = c^2 * The origin will always be at the __center__ in this form Parametric equations: x = ct, y = c/t where t = 0. 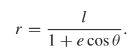### Polar Equation of a Conic * You can describe the equation of a non-degenerate conic in terms of **polar coordinates** * Polar coordinates: r and θ  This formula comes from the equation **OP = e · PM** where the distance from the origin to a point is equal to the eccentricity multiplied by the distance between that same point and the directrix. ## 1.1.4 Focal Distance Properties **Theorem 5:** The sum of the two focal distances from a point always equal double the major axis. Why? The distance from a point to __either foci__ is equal to the __eccentricity times the distance from said point__ __to the directrix.__ This distance is equal to the distance from the second foci to to second directrix **(d’ and f’).** When adding these two distances, they will always equal **2a** (double the major axis) **Theorem 6:** Differences of __Focal Distances__ of Hyperbola * If a point (P) is closer to **F**, then **PF’ - PF = 2a** * If P is closer to **F’** then **PF’ - PF = -2a** * |PF − PF| is __constant__ for every point on the parabola ## 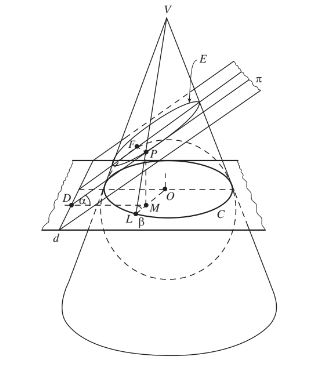1.1.5 Dandelin Spheres A slant plane (pi) that cuts one portion of a right, circular cone is an __ellipse__  # 1.2 Properties of Conics ## 1.2.1 Tangents Goals: Find the slope of the line that is __tangent__ to the curve at the __parameter__, so that you can determine the __equation of the tangent__ **Theorem 1:** The slope of a tangent to a curve is **y’(t)/ x’(t)** **Theorem 2:** Equations of tangents in __standard form__  **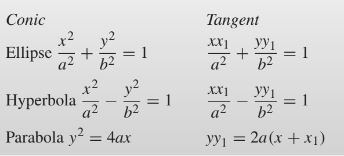Polar** of (a,b) with respect to the unit circle * If (a,b) is some point on a tangent, then it can be determined that **ax1 + by1 = 1** because of the equation **xx1 + yy1 = 1**. This equation can be re-written with points x2 and y2, which deduces the fact that points ( x1, y1) and (x2, y2) are on the __same line,__ and this line is called the __polar of (a,b) with respect to the unit circle__ **Theorem 3:** When a point (a,b) lies __outside__ of the circle, and the tangents from point (a,b) touch the circle at P1 and P2, then the equation of the line is **ax + by = 1** * **Normal of a curve**- the line through a point that is __perpindicular to the tangent__ of a curve at said point * To find the equation of a normal of the curve, you find its __slope__ and the __coordinates__ of the point on the parabola where they intersect ## 1.2.2 Reflections **Reflection law**- The angle that __incoming light__ makes with the __tangent__ to a surface is the same as the angle that the __reflected light__ makes with the tangent. * Applies to all mirrors, whether __plane__ or __curved__ * Mirrors are often designed to have a __conic curve__ **Reflection property of the Ellipse** - light which comes from one focus of an __elliptical mirror__ is reflected at the ellipse to pass through the __second focus.__ **Reflection property of the hyperbola-** light coming from one focus of a hyperbolic mirror is reflected at the __hyperbola__ and makes the light appear to have come from the other focus (**Internal Reflection property**). Light going towards one focus is reflected towards the __other focus__ **(External Reflection Property**) **Reflection Property of the Parabola**- incoming light __parallel__ to the axis is reflected at the __parabola__ to pass through the __focus__. 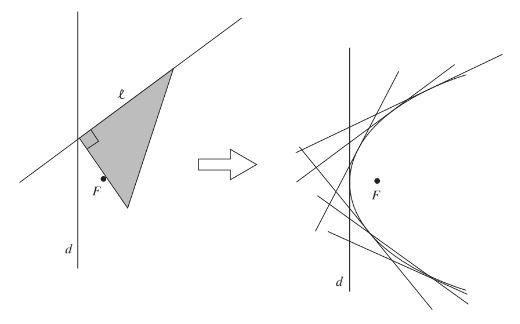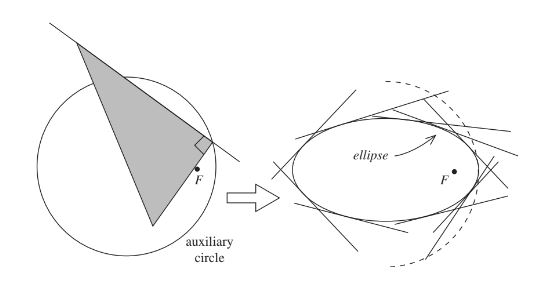Light coming from the focus of a parabola is reflected to give a beam of light parallel to the axis of the parabola ## 1.2.3 * __Non-degenerate conics__ are the __envelopes__ of a family of lines that are tangent to the conics * The conic being constructed is the __curve in the plane__ that has each of the lines in the family as a tangent * **Auxiliary circle**- A circle whose diameter is its major axis * The tangent to a parabola at its vertex is the auxiliary circle of the parabola **Theorem 4:** A perpindicular from a focus of a non-degenerate conic to a tangent meets the tangent on the auxiliary circle of the conic * When given a parabola and its axis, this theorem is used to identify the focus ### Envelope Drawings **Parabola:**  **Ellipse:**  **Hyperbola:**  \ # 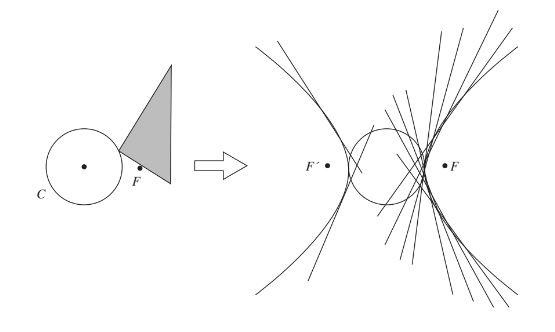1.3 Recognizing Conics **Standard form of conics**- the centre is at the __origin__, and the axes are parallel to the __x and y axis__ * Equations of all __non-degenerate conics__ follow this equation in __standard form:__ * Ax^2 + Bxy + Cy^2 + Fx + Gy + H = 0, * Any non-degenerate conic can be determined from a conic in standard form using a __rotation__ **(x, y) → (x cos θ − y sin θ, x sin θ + y cos θ)** followed by a __translation__ **(x,y) → (x-a, y-b)** * Both tranformations are __linear__, so the equation of the conic at each stage is just a __second degree__ of the original equation ### Matrices Matrix form of the equation: **x^T Ax + J^T x + H = 0.** * Look back at the old equation: Ax^2 + Bxy + Cy^2 + Fx + Gy + H = 0 * The values from this form are used to plug into the __matrix form__ *  \ **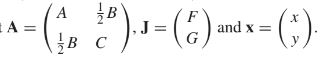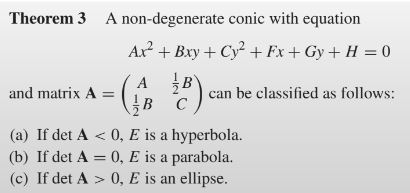Theorem 2:** A 2x2 matrix P represents a __rotation__ of R^2 when P is __orthogonal__ and the det of P = 1 Classify a conic (E) with the equation Ax^2 + Bxy + Cy^2 + Fx + Gy + H = 0 1. Write the equation in __matrix__ form (**x^T Ax + J^T x + H = 0**) 2. Determine the __orthogonal matrix__ P, with a determinant of **1** that __diagonalizes__ A 3. Make the change of coordinate system **x=Px’**. This changes the equation to **λ1x’^2 + λ2 y’^2 + f x’ + gy’ + h = 0** 1. λ1 and λ2 are the __eigenvalues__ of A 4. Complete the square to rewrite the equation of E into **(x’’, y’’)** as the equation of a conic in standard form 5. Use the equation x’ = P^Tx to determine the __centre__ and __axes__ of E in the terms of the __original__ coordinate system.  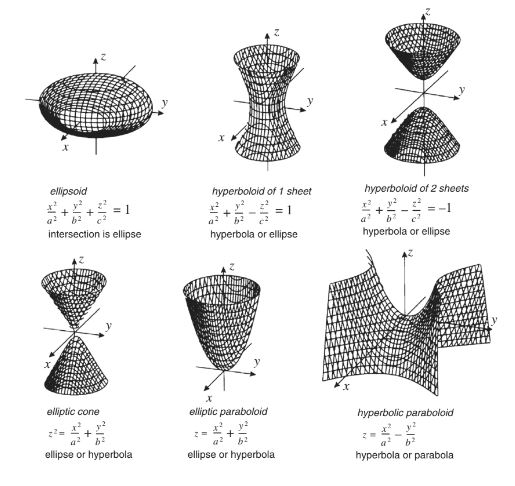# 1.4 Quadric Surfaces * **Quadric surfaces**- (quadrics) surfaces in R^3 that are the __natural analogues__ of the conics. * Ax^2 + By^2 + Cz^2 + Fxy + Gyz + Hxz + Jx + Ky + Lz + M = 0 * **Degenerate quadrics**- Empty set, single point, single line, single plane, pair of planes, and a cylinder * **Cylinder**- any surface that consists of an ellipse, parabola, or hyperbola in some plane  \ ## 1.4.2 * Use the __orthogonal diagonalization__ of 3x3 matrices to classify non-degenerate quadrics, similarly to how 2x2 matrices were used to __classify conics__ **Idetifying matrices:** In the equation Ax^2 + By^2 + Cz^2 + Fxy + Gyz + Hxz + Jx + Ky + Lz + M = 0,  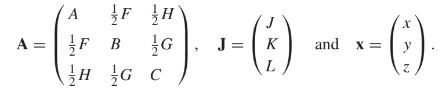If a 3x3 matrix (P) represents a __rotation__ of R^3 about the origin when __P is orthogonal__ and **det P = 1** Classify quadric E with the equation Ax^2 + By^2 + Cz^2 + Fxy + Gyz + Hxz + Jx + Ky + Lz + M = 0, 1. Write the equation in matrix form (**x^T Ax + J^T x + M = 0**) 2. Determine the __orthogonal matrix P, with a determinant of 1 that diagonalizes A__ 3. Make the change of coordinate system **x=Px’.** This changes the equation to λ1x’^2 + λ2 y’^2 + λ3 z’^2 + jx’ + ky’ + lz” +m = 0 1. λ1, λ2, and λ3 are the __eigenvalues__ of A 4. Complete the square to rewrite the equation of E into **(x’’, y’’, z’’)** as the equation of a __quadric__ in standard form 5. Use the equation x’ = P^Tx to determine the centre and axes of E in the terms of the __original__ coordinate system. ## 1.4.3 Rulings of Quadric Surfaces **Ruled Surface in R^3**- a surface that can be made up from a family of __straight lines__ ### Hyperboloid of One Sheet * **x^2 + y^2 - z^2 = 1** * The surface meets each horizontal plane in a circle whose __centre lies on the z-axis__ * The surface meets each plane containing the z-axis in a __rectangular hyperbola__ * **Family of Generators**- (generating lines) straight lines that __construct__ the hyperboloid * **Ruled surface**- __surface__ created by the generating lines * There is a __second family__ of lines that are also generators of said surface, these lines are __rotated__ around the z-axis * The lines that share a family never meet, but the lines of opposite families will always meet **Theorem 2:** A hyperboloid of one sheet contains __two families__ of generating lines, **The members of each family are disjoint, and each member of either family intersects each member of the other,** with exactly __one exception__ ### Hyperbolic Paraboloid * Theorem 2 is also valid for this figure \ \