Sept. 3 2024

• Euclidean Geometry

  • Named after mathematician (Euclid)

  • 300 BC

  • Book(s): The Elements

    • Organized logical system

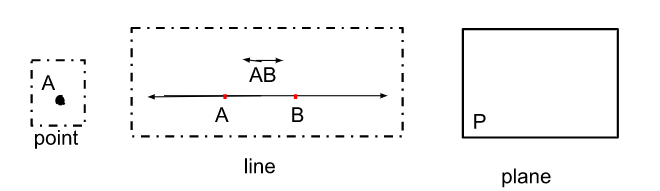
• Undefined Terms (building blocks of Geometry)

  • point - represented by a dot

    • indicated a place/position

    • no length, width, or thickness

    • named by a capital letter

  • line

    • set of points that extend endlessly in both directions

    • named by using two points

  • plane

    • set of points that forms a completely flat surface expanding indefinitely in all directions

    • 

Sept. 4, 2024

• Definitions involving segments

  • line segment/segment

    • set of points consisting of 2 points on a line, named using end points, and includes all points on the line between the end points

    • To name a segment, write the names of two points (ex. AB) two points with a line on top.

    • To write the measure, write the names of two points (ex. AB)

  • congruent segments

    • segments that have the same measure

    • ≅ is the symbol for congruence (always the same)

    • Since AB = CD, segment AB ≅ segment CD

  • collinear points

    • a set of points all of which lie on the same line.

    • if A, B, and C are collinear, B is between A and C, and AB + BC = AC

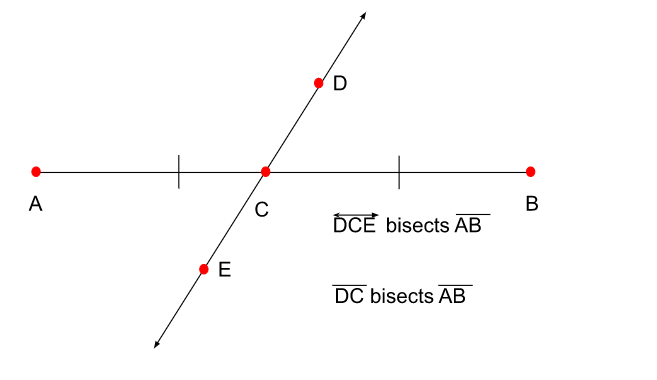
  • midpoint of a segment

    • the point of the segment that divides the segement into two congruent segments

    • 

  • bisector of a segment (bisector)

    • any line or subject of a line that intersects the segment at its midpoint

    • 

• Definitions involving angles

  • ray

    • endpoint gets named first

    • arrow on top of the name points right

  • opposite rays

    • 2 rays of the same line w/a common endpoint and no other point in common

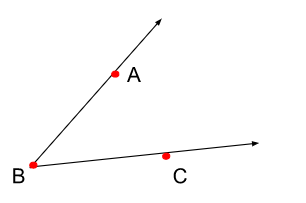
  • angle

    • the union of two rays w/same endpoint

    • 

  • straight angle

    • union of opposite rays

    • looks like a line

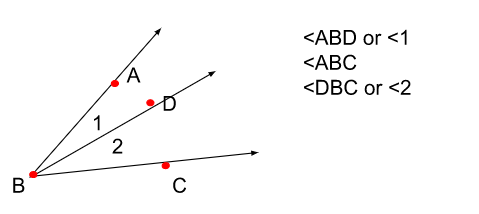
  • congruent angles

    • angles that have the same measure

      • <ABD ≅ <CBD means m<ABD = m<CBD

      • (lines in the angles show congruence)

        

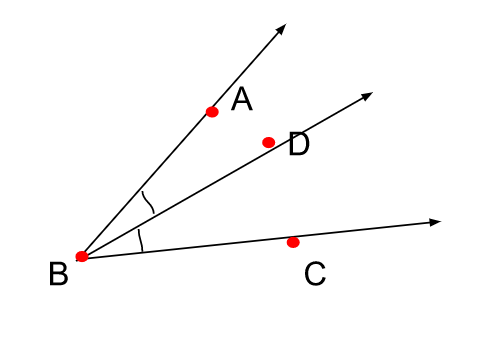
  • bisector of an angle

    • a ray who’s endpoint is the vertex of the angle and which divides the angle into two congruent angles

• Properties & Postulates

• postulate - statement accepted without proof

  • Reflexive Property of Equality

    • A quantity is equal to itself (measure something multiple times and get the same answer)

      • m<A = m<A BC = BC

  • Symmetric Property of Equality

    • If a = b, then b = a

    • If m<C = m<E, then m<E = m<C

  • Transitive Property of Equality

    • if a = b & b = c, a = c

      • All three also hold with congruence

  • Partition Postulate

    • A whole equals the sum of all its parts

  • Substitution Postulate

    • A quantity may be substituted for its equal in any expression

• Arithmetic Postulates

  • Addition postulate

    • If a = b & c = d, then a + c = b + d

      • 7 = 7 & 5 = 5, then 7 + 5 = 7 + 5

  • Subtraction postulate

    • If a = b & c = d, then a - c = b - d

  • Multiplication postulate

    • If a = b & c = d, ac = bd

  • Division postulate

    • If a = b & c = d, a/c = b/d