Gases fill any container, easily compressed, and mixes completely with any gas.

A device to measure atmospheric pressure, the barometer, was invented in 1643. It works by filling a tube with mercury, and inverting it on a dish of mercury. The mercury stops flowing out of the tube when the pressure of the column of the mercury is equal to the pressure of the air. This makes the mercury look like its standing on the surface of mercury.

The conversion 1 atm= 760 mmHg, or torr, comes from the barometer experiment because at sea level the height of the column of mercury averages 760 mm. This measure changes by weather conditions like when there is a storm that decreases the atmospheric pressure or with altitude.

For example, when Torricelli’s experiment is done in Breckenridge, Colorado (elevation 9600 feet), the atmosphere supports a column of mercury only about 520mm high because the air is “thinner.” This means that there is less air pushing down at earth’s surface at Breckenridge than at sea level because the air molecules get denser, so the pressure decreases.

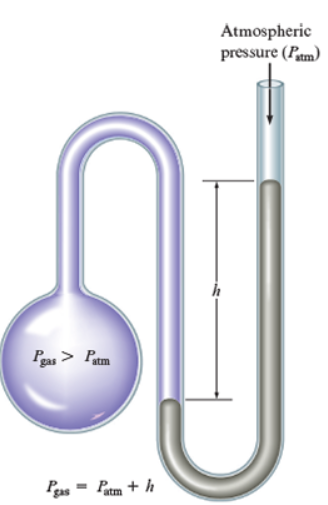
Another instrument used to find pressure is with the manometer. In order to read the pressure on a manometer, you must look at the height or difference in the mercury levels.

Boyle’s Law is P₁V₁=P₂V₂. The graph of Boyle’s Law gives a hyperbola because as the pressure decreases from the y-axis, the volume increases in the x-axis.

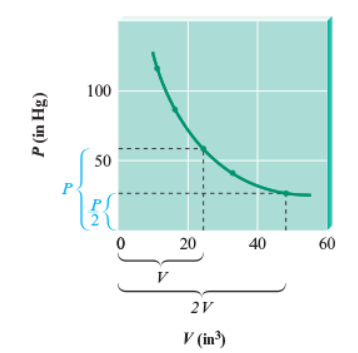
However, Boyle’s law only holds at very low pressure. When higher pressures are measured, the changes in PV become more significant. However, there are gases that strictly obey Boyle’s Law which are called an ideal gas.

When checking your answers for errors when using Boyle’s Law, make sure the answer makes sense. If the pressure was increased, the new volume has to decrease. If the pressure was decreased, the new volume has to increase.

Charles’s Law graph shows that the volume of a gas is directly proportional to the temperature, extrapolates to zero at 0 K, and creates a linear graph with temperature directly affecting the volume. (Temp x-axis, y-axis is volume)

In Charles’s law, when temperature increases at constant pressure, the volume should increase. This is because the graph is linear.

The equation is V₁/T₁=V₂/T₂

Kelvin is used instead of Celsius because there can’t be a negative volume. This is called absolute zero, 0 K, and it can never be reached.

Avogadro’s Law is represented by V₁/N₁=V₂/N₂. This equation states that a gas at constant temperature and pressure, the volume is directly proportional to the number of moles of gas.

All of these laws (Avogadro’s, Charle’s, and Boyle’s) show how volume of a gas depends on pressure, temperature, and number of moles of gas present.

When the laws are combined, it becomes the ideal gas law: PV = nRT. The ideal gas law finds the condition of the gas at a given time.

The R in the ideal gas law equation is a constant with a value of 0.0821 L*atm/K*mol.

The ideal gas law is an empirical equation or an equation that finds the hypothetical value. It finds the real gases’ behavior when it approaches low pressures and high temperatures, the conditions of an ideal gas.

The ideal gas law equation works best when the pressures are below 1 atm because it provides the result with minimal errors.

The ideal gas law works well when dealing with the initial and final states. But, during a change in state, we must place the variables that change on left side of the equal sign and the constants on the right. For example, if only the pressure and volume was changing, but the moles and the temperature remains constant, the pressure and volume (PV) will stay on the left while the constants (nRT) stays on the right.

The molar volume of an ideal gas (0 degrees Celcius and 1 atm) is 22.42 L. Therefore, the conversion 1 mol/22.42 L will work with many ideal gases.

0 degrees Celcius and 1 atm are used as the ideal gas conditions because they are STP, or standard temperature and pressure.

One can use the molar volume for gas stoichiometry. This process can be listed as followed:
1. Balance the equation
2. Find the mols of the reagents that need to be included in the stoichiometry problem. Find the limiting reactant if there are two reactants.
3. Use the mol ratio.
4. Use the molar volume to convert the mols to volume.

It is important to note that molar volume can only be used when the conditions of the problem are at STP. If the problem is not at STP, then the ideal gas law must be used to compute the volume.

One can use ideal gas law to find the calculation of the molar mass and its density. The process to derive it from the ideal gas law is this:
1. n, the variable that represents te number of moles in a gas is expressed by:
- Grams of gas/molar mass = mass/molar mass = m/molar mass.
2. When substituting the calculation of the molar mass of the gas, you can find the measured density.
- P = nRT/V = (m/molar mass)RT/V = m(RT)/V(molar mass).
- m/V is equal to the density, or d.
3. Knowing m/V equals to d, it can be rewritten as
- P=dRT/molar mass
- molar mass = dRT/P

In summary, n = m/molar mass and d=m/V. These equations can be used to substitute the ideal gas law components to find the molar mass of a gas.

Dalton’s law of partial pressures is expressed as P_TOTAL = P₁+P₂+…

The mole fraction is defined by the textbook as the ratio of the number of moles of a given component in a mixture to the total number of moles in the mixture. This is essentially saying the partial pressure over the total pressure results in a percentage or fraction.

When gas is collected over water, a vapor pressure of water occurs. This is because the water molecules escape the surface of the liquid and collect in the space above the liquid. They also return back to the liquid. When the rate of escaping the liquid equals the rate of return, the number of water molecules stay constant = vapor pressure of water. (kind of like the barometer experiment with the mercury staying still)

Due to water vapor, when solving stoichiometry problems, the water vapor pressure must be added to the total pressure.

Kinetic Molecular Theory (KMT) explains the properties of IDEAL gas. There are four postulates of the KMT.
1. The volume of the individual particles can be assumed to be negligible because they are so small.
2. The gas particle are in constant motion, and the collisions of the walls and particles are what causes the gas to exert pressure. These collisions are elastic, meaning no kinetic energy is lost.
3. The gas particles are assumed to neither attract nor repel each other.
4. The average kinetic energy is directly proportional to the temperature.

In real life, molecules in a real gas have finite volumes and do exert forces on each other. This shows that the KMT postulates explain ideal gas behavior, not real gas behavior.

KMT based on Boyle’s Law: It makes sense because a decrease in volume (container) would increase the gas particles to hit each other and the walls thus increasing pressure.

KMT based on pressure and temperature: it makes sense because when the temperature increases, the speed of the particles increases. This makes the particles hit the wall and each other with greater force and frequency, leading to increase of pressure.

KMT based on Charles’s Law: Charles’s Law predicts that in constant pressure, the volume and temperature both must increase. This is true because temperature increases pressure, so in order to have a constant pressure, the volume must increase as well to compensate.

KMT based on Avogadro’s Law: this makes sense because more gas particles at the same temperature and volume will cause the pressure to increase.

Mole fraction: mol of substance/total moles * the total pressure = the partial pressure.

The root mean square velocity equation is

Because this equation represents the average velocity/speed, it needs to be measured in energy, or Joules. A joule is a kilogram meter squared per second squared (kg*m²/s²)

This means that in the root mean square velocity equation, the M is in kilograms, R is now 8.3145 not 0.0821, and the units is m/s

In a real gas, there are many collisions within a container. But, when a gas is released into a bigger space, like a room, it takes time for the gas to spread. This delays collisions and the mixing process, and if you wanted the gas to meet in the middle, the slower gas would have to go first as a “headstart”

While the root mean square velocity answer “U_rms” gives the average meters per second of a gas, not all molecules have this velocity. When a gas molecules collide, they exchange kinetic energy meaning the one hit moves faster than the one who hits. This distribution is shown by the Maxwell-Boltzmann curve.

In the Boltzmann curve, the temperature and molecular velocity have a relationship. The peak of a curve reflects the most probable velocity that can be found in the various particles.

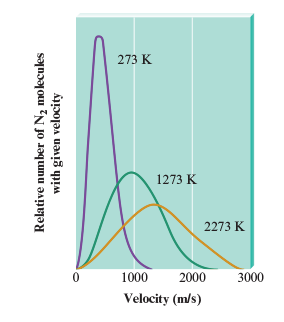
However, it is important to note that average kinetic energy increases with temperature, and not average velocity increases with temperature. (AKA NO LINEAR RELATIONSHIP). There are places in the average velocity-temperature graph (Boltzmann) where some molecules have lower velocities compared to others at different temperatures.

Effusion is when the gas passes through a tiny hole into an empty chamber. The rate of effusion measures the speed of which the gas transfer into said chamber.

The effusion rate for a gas depends directly on the average velocity of its particles, which can be calculated using the root mean square velocity equation. The faster the gas particles, the more likely it will pass through the hole.

Graham’s Law states that the ratio of the rate of effusion for gases 1 and 2 is inversely proportional to the square roots of the masses of the gas particles when it has constant temperature and pressure.

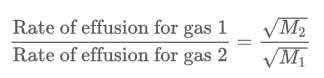
The faster, lighter gas is gas 1 and the slower, heavier gas is gas 2. You can check your work to see if you get a positive rate. If it is negative, you may have put a molar mass in the incorrect spot in the formula.

Diffusion is when gases are mixed. The rate of diffusion is the rate of the gases mixing. Graham’s Law can not be used with diffusion because so many collisions occur when gases mix, like with O2 and N2 (air), that the theoretical value is inconsistent.

Ideal gases are not real. Think of them as asymptotes; gas conditions of low pressures and/or high temp that real gases approach. A real gas can only exhibit behavior close to ideal behavior at low pressures and high temperatures.

The two postulates that are incorrect in the KMT postulates is that it was theorized gases are negilibile, or no volume, meaning it has the same volume as the container. However, real gas consists of atoms/molecules have finite volumes.

There is also intermolecular forces between gas molecules. Because of these attraction, it causes the particles to hit the wall less often as some of its time is taken to being attracted to other molecules. This leads to a lower pressure than expected in the absence of interactions.

In the van der Waals equation, the (V-nb) corrects the volume to become finite, and the Pobs +a(n/V)² corrects the attraction.

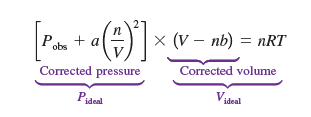
the van der Waals makes sense because the finite volume is less important when it is in a large container, which means low pressure, but if it is at a small container, the finite volume needs to be accounted for as there is high pressure. Similarly, if there is high temperature the particles don’t attract as quickly.

A low value for a means a weak intermolecular force.