COMPOUND INTEREST

compound interest (def). interest where the money earnt in deposited at a certain frequency and is used to calculate the next interest payment - e.g. superannuation or investments

FV = PV x (1 + r/100k)^kn

FV = Future Value - how much money in total after the investment period has ended

PV = Present Value - how much money has been invested to begin with

k = how many times a year the interest is paid

• e.g. yearly, k = 1

• monthly, k = 12

• weekly, k = 52

• quarterly, k = 4

• half yearly, k = 2

n = number of years the investment period goes for

r = the interest rate

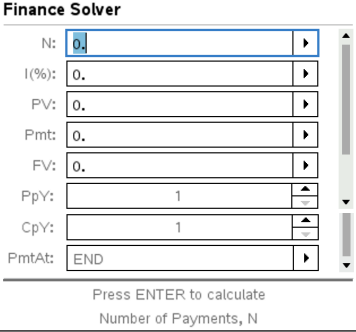TEXAS INSTRUMENTS TI-NSPIRE CX 2 - finance solver (used to solve compound interest)

N = amount of times that the interest is paid through the

I - Interest Rate

PV - Present Value - how much money has been deposited at the beginning of the investment, should be entered as a negative value

Pmt - the amount of any extra payments, not usually applicable within compound interest

FV - Future Value - will come out as the opposite value (positive or negative) to the PV

PpY - Payments per Year - how many times each that the interest is paid into the account

CpY - Compounds per Year - how many times each year that the interest calculated

• PpY = CpY

PmtAt - when the payments are paid - for compound interest it will almost always be at the end unless the question specifies

OXFORD IB TEXTBOOK : Exercises 10D and 10E

DEPRECIATION
depreciation (def). the way that the value of something reduces overtime, measured with a rate of depreciation

• in calculator, the rate of depreciation needs to be set as a negative value

• in answers, the rate of depreciation needs to be given as a positive value

OXFORD IB TEXTBOOK : Exercises 10D and 10E

INFLATION

inflation (def). how the value of money changes to be worth less over the years, or a decrease in the currencies buying power (how much can it buy)

• one dollar now will buy more that a dollar 50 years in the future but less than a dollar 50 years in the past

• calculated yearly

• REMEMBER - make sure that the interest rate is annual - if not, multiply the monthly interest usually given by 12

real value (def). how much an amount of money in the future will actually be worth, accounting for inflation

real value = future value/inflation factor

inflation factor = (100% +inflation rate%)^years

EXERCISE

ANNUITIES

annuity (def). a fixed sum that is paid or taken out of an account at regular intervals over a certain period

• e.g. a savings fund

• in most questions, the PV or FV will be zero

• + payments are ones going to you/out of the bank

• - payments are ones going to the bank or other recipients

Pmt = the amount that is being paid

PpY = the amount of times per year the amount is being paid

OXFORD IB TEXTBOOK : Exercise 10F

AMORTIZATION

amortization (def). paying off a loan or debt, translates to “kill off”

• FV will most often be zero - the loan has been paid off

• interest is per year

OXFORD IB TEXTBOOK : Exercise 10G

HOW TO CALCULATE TOTAL INTEREST PAID OVER A PERIOD OF TIME

EXAMPLE

given information

PV = $170000

N = 30 × 12 = 360

I = 3.8%

CpY = 12

PpY = 12

part a

*use the finance solver*

Pmt = ?

(using finance solver) : Pmt = 792.1274…

A(a). will pay $792.13 each month

part b

i.

Pmt = $792.12… (use unrounded value through working)

N = 10 × 12

N = 120

FV = ?

(using finance solver) : FV = $133020.301…

A(bi). will still owe $133020.30 after 10 years

ii.

FV = $133030.301…

total payments over 10 years (TPmt) = ?

TPmt = Pmt x (PpY x yrs)

TPmt = 792.12… x (12 × 10)

TPmt = 792.12… x 120

TPmt = $95055.299…

total capital paid (TCP) (def. how much of the original value has been paid off) = ?

TCP = PV - FV

TCP = 170 000 - 133030.301…

TCP = $36979.698…

total interest paid (TI) = ?

TI = TPmt - TCP

TI = 95055.299… - 36979.698…

TI = $58075.6013…

A(bii). $58075.60 of interest paid over the 10 years