INTRODUCTION TO PHYSICS (6)

• Three main types of forces will be explained in detail here: frictional force, drag force, and centripetal force.

FRICTIONAL FORCE

• If frictional forces were not counteracted in our daily lives, they would literally stop everything from moving. However, if friction was totally absent, it would lead to everything dissembling.

• One type of frictional force is the static frictional force which resists force that is applied to an object, and the object remains at rest until the force of static friction is overcome.

• For example, a block kept on a table is exactly balanced by the gravitational force and the normal contact force acting downwards and upwards respectively.

• If a small force F is applied to it, the box does not move. This is because the static frictional force balances it.

• When the force applied becomes larger than the static friction, that is when the box starts to move and accelerates.

• To maintain a constant speed once the box has started moving, the force applied F is gradually reduced to match the frictional force.

• It is to be noted that when force is applied to the box, the static frictional force also keeps increasing until a certain magnitude. It is only after that that the box starts moving.

• This brings us to the next type of frictional force: the kinetic frictional force.

• Kinetic frictional force is the force that opposes motion.

• Usually the magnitude of the kinetic frictional force is less than the magnitude of the static frictional force. This is why the force applied has to be reduced to maintain a constant speed once the object starts moving.

• On an atomic level, frictional force is basically the vector sum of many forces acting between the surface atoms of one body and those of another body.

• When two ordinary surfaces are placed together, the area in contact on the microscopic level is much less than is generally thought since only the high points of each surface touch each other.

• Many surfaces still cold-weld together (joining surfaces to one another without the use of heat, by forcing them together so hard that adhesion occurs.)

• When the force is applied relative to them, static friction is produced.

• If the applied force is large enough to pull one surface across the other, there is first a tearing of welds (at breakaway) and then a continuous re-forming and tearing of welds as movement occurs and chance contacts are made.

• The kinetic frictional force that opposes the motion is the vector sum of the forces at those many chance contacts.

• If the two surfaces are pressed together harder, many more points cold-weld.

• Usually the surfaces sick and slip together which produces a squealing sound, e.g. when tyres skid on the road.

PROPERTIES OF FRICTION

• It is observed that when two dry and unlubricated surfaces are caused to move against each other, the friction produced has three qualities:

• If the body remains stationary, the static frictional force Fs is equal to the applied force F. They are equal in magnitude and opposite in direction so they balance each other out.

• The maximum value of static friction is given by multiplying the coefficient of static friction by the magnitude of the normal force on the body from the surface. If the applied force F exceeds this value, the body begins to slide along the surface.

• 

• If the body begins to slide along the surface, the magnitude of the frictional force reduces to a value of Fk which is the product of the coefficient of kinetic friction and the magnitude of the normal force on the body from the surface.

DRAG FORCE AND TERMINAL FORCE

• A fluid is anything that can flow, which is usually liquid or gas.

• When a body moves through a fluid or a fluid moves past a body, there is a relative velocity between the body and the fluid.

• Whenever there is relative velocity, the body experiences a drag force that opposes the relative motion and points in the direction in which the fluid flows relative to the body.

• In cases when the body is fast enough that the air becomes turbulent, the magnitude of the drag force is related to the relative speed v by an experimentally determined drag coefficient C, by the equation:

where r is the air density (mass per volume) and A is the effective cross-sectional area of the body.

• If the value of v varies significantly, the value of C may also vary, but generally, it is thought to be a constant.

• Drag force has to be reduced in order to increase speed, which is usually done by the streamlined position.

FALLING

• When a blunt body falls, the drag force is directed upward.

• The drag force gradually increases from 0 as the speed of the body increases.

• The drag force opposes the downward gravitational force. It can be related to acceleration by the equation:

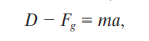
• If the body falls long enough, the drag force eventually balances the gravitational force and the speed becomes constant. This is called the terminal speed.

• At terminal velocity, the acceleration is zero. Therefore replacing a with 0 and rearranging the previous equation gives:

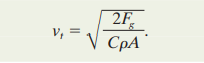
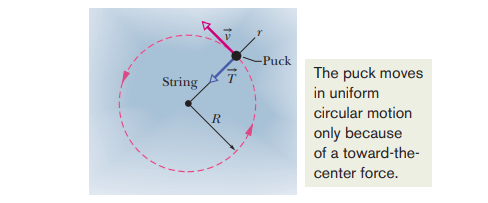
UNIFORM CIRCULAR MOTION

• When a body moves in a circle (or a circular arc) at constant speed v, it is said to be in a uniform circular motion.

• It also has a centripetal acceleration (directed toward the center of the circle) of constant magnitude which is given by:

where R is the radius of the circle.

• Examples of circular motion include a car turning a corner, the electrons orbiting the nucleus, and the earth orbiting the sun.

• For any situation, the centripetal force accelerates a body by changing the direction of the body’s velocity without changing the body’s speed.

• The magnitude of the net centripetal force can be written as:

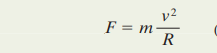since the speed v is constant, the magnitude of acceleration and force are also constant.

• However, the direction varies continuously so it is always pointing towards the centre of the circle. Therefore, the force and acceleration vectors are sometimes drawn along a radial axis r that moves with the body and always extends from the center of the circle to the body, even though the positive direction of the acceleration is radially outward.