Area under curves

Areas

• You need to be able to estimate the area under a curve
• You can do this by drawing trapeziums in equal intervals undernearth the graph

Examiners report

• The area under a velocity-time graphs tells you the distance travelled

• Divide the area into four equal intervals and draw trapeziums

• If you label your trapeziums it will make your working clearer

• The formula for the area of a trapezium is

  • Area = 1/2(a+b)h
  • It’s easiest to think of the width of the trapezium as h and a and b as the height of the endpoints

  

• Look at the shape of the curve

  • Underestimate and Overestimate

  

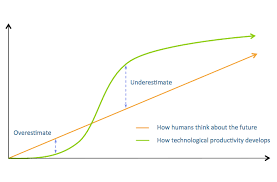
Understanding the meaning of the area

• Showed how the units can be used to identify the meaning of the gradient: by dividing the vertical axis units by the horizontal axis units.
• The meaning of the area under a graph can be found by multiplying the units.
  • For example,
  • m/s x s = metres/second x seconds/1 = metres

So the area represents distance in metres.