Chemistry 12HL Reactivity 1-3

Reactivity 1

1.1: Measuring enthalpy changes

Heat transfers

• energy is a measure of the ability to do work

  • to move an object against an opposing force

  • can be transferred through heat, light, sound, electricity, etc.

• heat - form of energy transfer that occurs as a result of a temperature difference

  • when heat is transferred to a system, the average KE of molecules and the temperature are increased → KE is more dispersed among the particles

System and Surroundings

• system → area of interest

  • open system → energy/matter can be exchanged with surroundings

  • closed system → energy can be exchanged but matter cannot

  • isolated system → energy and matter cannot be exchanged with surroundings

• surroundings → everything else in the universe

Enthalpy of a system

• enthalpy → chemical potential energy of a system

  • system acts as a reservoir of chemical potential energy/enthalpy

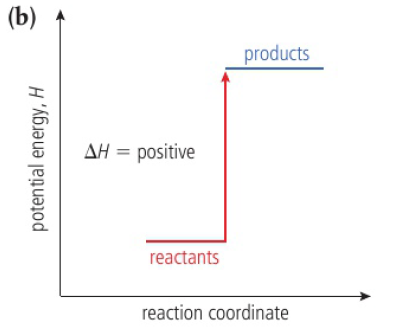
• when heat is added to a system from its surroundings, its enthalpy increases (+ΔH)

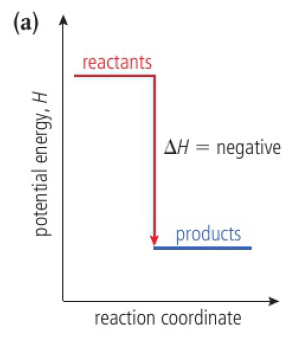
• when heat is given out by a system, its enthalpy decreases (-ΔH)

  • ΔH = change in enthalpy

• endothermic:

  

• exothermic:

  

Direction of a reaction

• there is a natural direction for change

  • to lower stored/potential energy (exothermic)

  • chemicals change in a way that reduces their chemical potential energy

• products of an exothermic reaction are more stable than the reactants

  • stability is a relative term

• most combustion reactions are exothermic

  • the energy needed to break the bonds is less than the energy produced as bonds form

• activation energy → minimum KE to react

  • some bonds must be broken before new bonds are formed

  • 

Measuring enthalpy changes

• standard enthalpy change (ΔH^Ꝋ)

  • pressure → 100kPA

  • concentration of 1mol/dm³ for all solutions

  • all substances in their standard states

  • usually 298K as temperature

• thermochemical equations

  • ex: CH₄(aq) + 2O₂(g) → CO₂(g) + 2H₂O(l) ΔH_reaction = -890kJ/mol

    • 1 mole of CH₄ reacts with 2 moles of O₂ to produce 1 mole of CO₂ and 2 moles of H₂O and releases 890kJ of heat energy

Calculating enthalpy changes

• absolute temperature (K) is a measure of the average KE of the particles

  • more/less particles with same heat energy added will result in a different temperature

• q=mcΔT

  • q: heat added (J), m: mass (g), c: specific heat capacity (J/gK), ΔT: temperature change (K)

• specific heat capacity → heat needed to increase the temperature of a unit mass of a substance by 1K

  • depends on the number of particles present

Combustion: enthalpy changes

• enthalpy change of combustion (ΔH_c) can be determined using this apparatus:

  • 

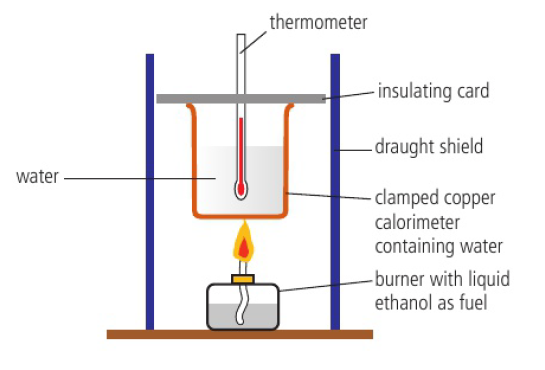
  • heat absorbed by the water can be calculated from the temperature change and mass

  • heat absorbed by calorimeter can also be calculated using c

• temperature of the water increases due to heat released from the combustion reaction

  • heat released as ethanol and oxygen turn into carbon dioxide and water

  • there is a decrease in enthalpy in this reaction

Reaction in the solution: enthalpy changes

• calculated by carrying out the reaction in an isolated system

  • heat released/absorbed by reaction can be measured from the temperature change in the water (solvent)

  • calorimeter made from an insulator to maximize heat transferred to water by reaction

• error → all heat produced in the reaction is absorbed by the water

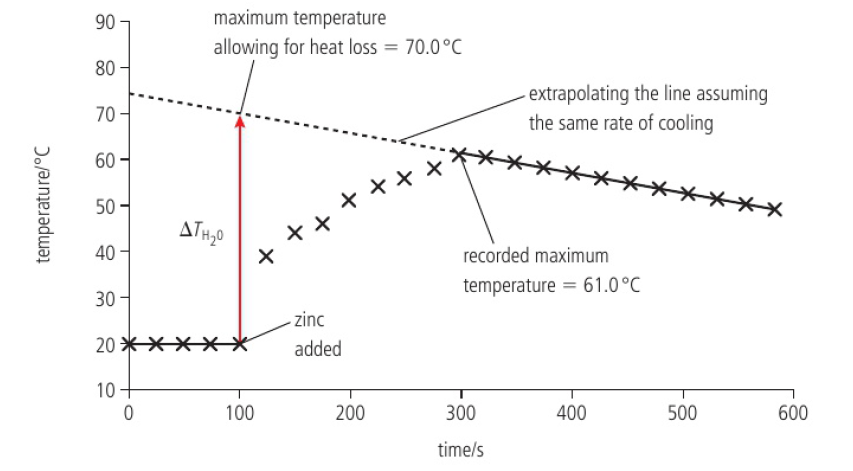
  • heat is lost from the system as soon as its temperature rises above the temperature of its surroundings

    • by extrapolating the cooling section to the time when the reaction started, it now creates some allowance for heat loss, so now we can assume:

      1. no heat loss from system

      2. all heat goes from reaction to water

      3. solution is dilute

      4. water has density of 1g/cm³

  • 

1.2: Energy Cycles in Reactions

• energy changes occur when bonds are broken or new bonds are formed

  • energy is required to separate particles and energy is released when particles come together

    • net enthalpy change is the difference between these two energy contributions

  • law of conservation of energy → energy cannot be created or destroyed, only transferred

Bond enthalpy

• bond enthalpy → energy needed to break one mole of bonds in gaseous molecules in standard conditions

  • ex: Cl₂(g) → 2Cl(g) ΔH=+242kJ/mol

• breaking bonds is an endothermic process (positive enthalpy change)

  • bond enthalpies differ, it may be harder to break depending on environment

    • to compare bond enthalpies which occur in different environments, average bond enthalpies will be used

• all bond enthalpies refer to reactions in the gaseous state

  • any enthalpy changes resulting from the formation/breaking of intermolecular forces are not included

• multiple bonds (involves more bonding electrons) generally have higher bond enthalpies and shorter bond lengths

• the more polar the bond, the stronger it will be

• making bonds is an exothermic process (negative enthalpy change)

  • the same amount of energy is absorbed when a bond is broken as is released when a bond is formed

Energy changes in reactions

• ex: complete combustion of methane

  • CH₄ + 2O₂ → CO₂ + 2H₂O

  • energy is taken in to break the C-H and O=O bonds in the reactants

  • energy is given out when the C=O and O-H bonds are formed in the products

  • reaction is exothermic overall as the bonds formed are stronger than those broken

    • opposite would be true for endothermic reactions

• ΔH = ΣE_{bonds broken} - ΣE_{bonds formed}

• some reactants need to be given an initial energy (activation energy) before they will react

  • some bonds in the reactants must break before new bonds can form

• rate of some reactions can be explained by the relative bond enthalpy of the bonds broken

Hess’s Law

• the enthalpy change for any chemical reaction is independent of the route, provided the same starting conditions and final conditions, and reactants and the products, are the same

  • ΔH₃=ΔH₁+ΔH₂

  • 

    • due to the conservation of energy

  • can be used to find enthalpy change of reactions that cannot be measured directly

Calculating enthalpy changes

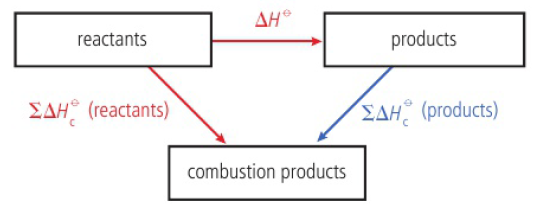
• standard enthalpy change of combustion (ΔH_c^Ꝋ)

  • enthalpy change that occurs when one mole of the substance burns completely under standard conditions

  • can be measured by calculating temperature change of water heated by the combustion

  • reactants - products

    • 

• standard enthalpy of formation (ΔH_f^Ꝋ)

  • enthalpy change that occurs when one mole of the substance is formed from its elements in their standard states

    • standard measurements taken at a specific temperature (usually 298K) and pressure of 1×10⁵ Pa

    • standard state of an element is its most stable form under these conditions

  • products - reactants

    • 

Lattice enthalpies

• first ionization energy (ΔH_i^Ꝋ) → energy needed to form the positive ion of a gaseous atom

  • endothermic process (pulling electron away from electrostatic force)

• first electron affinity (ΔH_e^Ꝋ) → enthalpy change when one mole of gaseous atoms attracts one mole of electrons

  • exothermic process (electron is attracted to positively charged nucleus)

• lattice enthalpy (ΔH_lat^Ꝋ) → formation of gaseous ions from one mole of a solid crystal breaking into gaseous ions

  • ex: NaCl(s) → Na⁺(g) + Cl^-(g) ΔH_lat^Ꝋ=+790kJ/mol

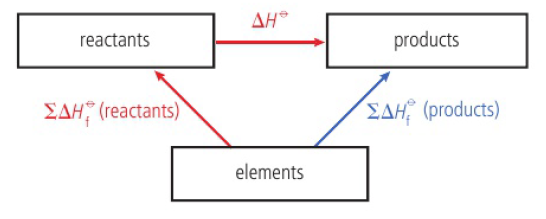
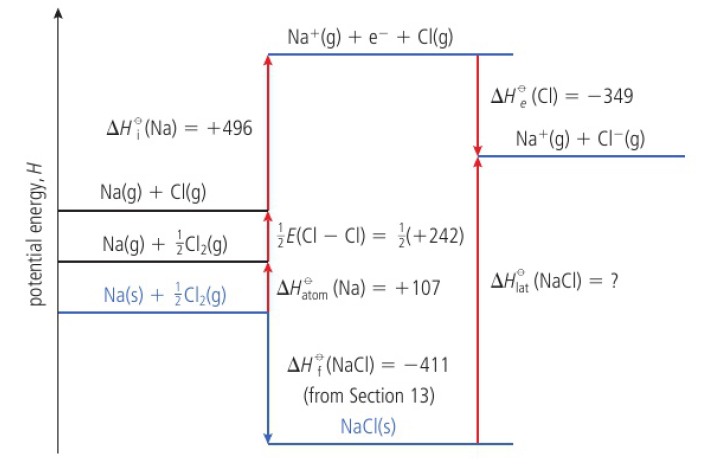
Born-Haber cycles

• formation of an ionic compound from its elements is supposed to take place in a number of steps, including the formation of the solid lattice from its constituent gaseous ions

  • from Hess’s Law, the enthalpy change for the overall formation of the solid must equal the sum of the enthalpy changes accompanying the individual steps

• ex: Na(s)+1/2 Cl2(g) → NaCl(s) ΔHf(NaCl)=-411kJ/mol

  1. Na(s)→Na(g) sodium is atomized ΔHatom(Na)=+107kJ/mol

  2. ½ Cl2(g)→Cl(g) 1/2E(Cl-Cl)=1/2(+242KJ/mol) E=bond enthalpy

  3. Na(g)→Na+(g)+e- ΔHi(Na)=+496kJ/mol

  4. Cl(g)+e-→Cl-(g) ΔHe(Cl)=-349kJ/mol

  5. Na+(g)+Cl-(g)→NaCl(s) -ΔHlat=+786kJ/mol → sum

• 

1.3: Energy from fuels

Combustion reactions

• many substances undergo combustion reactions when heated in oxygen

  • s-block metals form ionic oxides (basic)

  • p-block non-metals generally form covalent oxides (acidic)

• many hydrocarbons/alcohols are used as fuels as their combustion reactions release energy at a reasonable rate to be useful

  • high activation energy → do not spontaneously combust, safe transport and storage

  • kinetically stable

• complete combustion of organic compounds break the carbon chain → results in CO₂ + H₂O and a release in (a lot of) heat energy

  • complete combustion → products are fully oxidized

  • when oxygen supply is limited, incomplete combustion occurs

• incomplete combustion of organic compounds

  • if the air supply is limited/compound has high carbon content, incomplete combustion occurs

    • results in carbon monoxide / carbon (soot)

    • releases less heat than complete combustion

Fossil fuels

• an ideal fuel releases significant amounts of energy at a reasonable rate and produces minimal pollution

• fossil fuels are non-renewable → used at a rate faster than they are replaced

• liquid fuels have significantly greater energy densities than gases (per unit volume)

• fossil fuels were formed by the reduction of biological compounds

  • oxygen is lost from biological molecules which generally result in hydrocarbons

• coal → most abundant, 80-90% carbon (by mass)

• crude oil → mixture of straight-chain and branched-chain saturated alkanes, cycloalkanes, and aromatic compounds, used as fuel for transportation and electricity

• natural gas → primarily methane, cleanest fossil fuel → low carbon content

• coal

  • advantages

    • cheap, abundant

    • longest lifespan (compared to other fossil fuels)

    • can be converted into synthetic liquid fuels and gases

    • safer than nuclear power

    • ash produced can be used to make roads

  • disadvantages

    • contributes to global warming (CO₂ emissions)

    • contributes to acid rain (SO₂)

    • produces particulats (electrostatic preceptors can remove most of these)

    • difficult to transport

    • waste can lead to visual + chemical pollution

    • mining is dangerous

• petroleum

  • advantages

    • easily transported in pipelines/tankers

    • convenient fuel for use in cars → volatile, burns easily

    • high enthalpy density

    • sulfur impurities can be easily removed

  • disadvantages

    • limited lifespan and uneven world distribution

    • contributes to acid rain and global warming

    • transport can lead to pollution

    • carbon monoxide is produced through incomplete combustion (pollutant)

    • photochemical smog is produced

• natural gas

  • advantages

    • higher specific energy

    • clean and easily transported

    • does not contribute to acid rain

  • disadvantages

    • limited supplies

    • contributes to global warming

    • risk of explosion (leaks)

Combustion of alkanes

• increase in %carbon content down the homologous series suggests that incomplete combustion increases with length of the carbon chain

• mass of CO₂ produced per unit mass of fuel increases with %carbon content

• the higher %carbon content (and lower %hydrogen), the lower the specific energy

The greenhouse effect

• it is estimated that CO₂ contributes to about 50% of global warming

• greenhouse gases allow shortwave radiation from the Sun to pass through the atmosphere but absorb the longer wave infrared radiation that was re-radiated from Earth’s surface

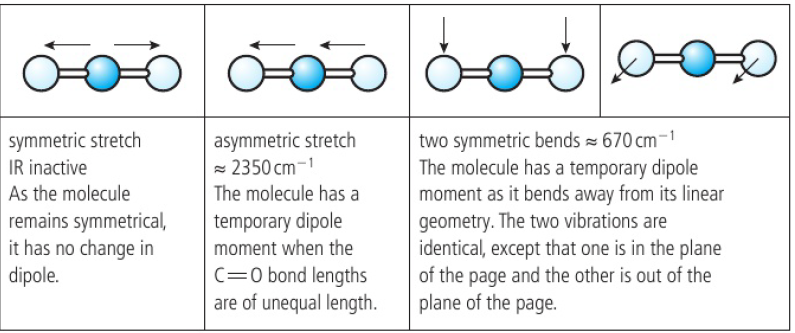
  • CO₂ is a GHG as its molecules increase their vibrational energy by absorbing IR radiation

    • 3 of the vibrational modes of CO₂ are IR active → dipole changes as it vibrates

• molecules then re-radiate the absorbed energy back to Earth’s surface → global warming

• 

• greenhouse effect increased as CO₂ levels increased, causing (due to change in temperature):

  • changes in agriculture (crop yields)

  • changes in biodistribution due to desertification and loss of cold-water fish habitats

  • rising sea levels caused by thermal expansion and melting of polar ice caps/glaciers

Biofuels

• photosynthesis converts light energy into chemical energy

  • chlorophyll (green pigment) absorbs solar energy which is used in this reaction:

    • 6CO₂(g) + 6H₂O(l) → C₆H₁₂O₆(s) + 6O₂(g)

    • carbon dioxide + water → (using solar energy) glucose + oxygen

  • biofuels → produced from the biological fixation of carbon over a short period of time

• ethanol is a liquid biofuel → used in internal combustion engines

  • made from biomass by fermenting plants high in starches and sugars

    • C₆H₁₂O₆ → 2C₂H₃OH + 2CO₂

  • process done at around 37C in absence of oxygen by yeast (provides enzyme)

  • advantages (when used in gasohol: 10% ethanol, 90% unleaded gasoline)

    • renewable, lower emissions of CO and nitrogen oxides, decreases dependance on oil)

  • disadvantages

    • ethanol absorbs water (it can form hydrogen bonds) so it seperates from the hydrocarbons

    • can cause corrosion

• methane → made form bacterial breakdown of plant mateiral in absence of oxygen

  • C₆H₁₂O₆ → 3CO₂ + 3CH₄

• advantages of biofuels

  • cheap + readily available

  • renewable (if crops/trees are replanted)

  • less polluting than fossil fuels

• disadvantages of biofuels

  • uses land → can be used for other purposes (ex: growing food)

  • high cost of harvesting and transportation

  • takes nutrients from soil / uses large amounts of fertilizers

  • lower specific energy than fossil fuels

Fuel cells

• hydrogen fuel cell

  • H₂(g) + ½ O₂(g) → H₂O(l)

  • this is a redox reaction (transfer of electrons from hydrogen to oxygen)

    • can produce an electric current if reactants are physically seperated

  • hydrogen fuel cell operates with either an acidic or alkaline electrolyte

    • in fuel cells, reactants are continuously supplied to different electrodes

• hydrogen-oxygen fuel cell → alkaline electrolyte (most commonly used)

  • 

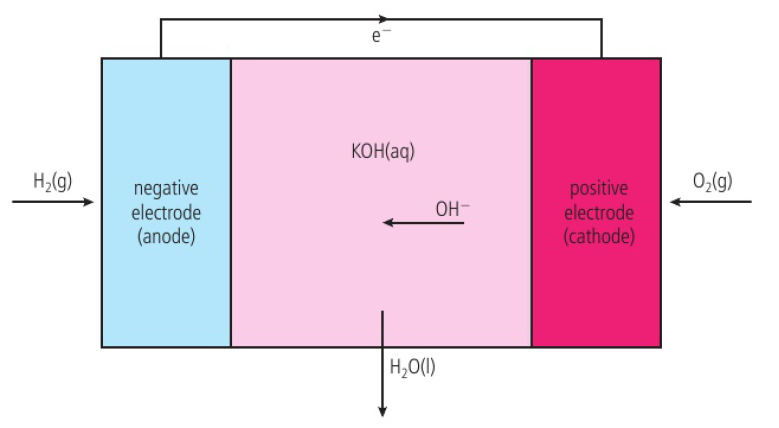
  • fuel cell will function as long as H₂ and O₂ are supplied

  • electrodes are often made of porous carbon with added transition metals (ex: nickel)

  • KOH (potassium hydroxide) provides the OH^- ions that are transferred across the cell

  • problem: hydrogen gas must be extracted from other sources so might not be renewable

• methanol fuel cell; DMFC → Direct Methanol Fuel Cell

  • methanol → stable liquid at normal environmental conditions, high energy density, easy to transport

  • DMFC → fuel is oxidized under acidic conditions on a catalytic surface to form CO₂

    • H⁺ ions formed are transported across a proton exchange membrane from anode to cathode where they react with oxygen to form water

    • electrons are transported through an external circuit from anode to cathode

    • water is consumed at the anode and produced at the cathode

• difference between fuel cells and primary voltaic cells:

  • fuel cells do not run out

    • fuel is supplied continuously to the cell as it is oxidized

1.4: Entropy and spontaneity

• second law of thermodynamics: matter and energy tend to disperse and become more dispersed

  • entropy (S) → degree of dispersal of matter and energy of a system

  • spontaneous change → dispersion occurs naturally without work

Entropy

• the natural tendency to change can be reversed if work is done

• entropy → measure of dispersal/distribution of matter/energy in a system

  • ordered states with small energy distribution → low entropy

    • ex: gas particles concentrated in a small volume

  • disordered states with high energy distribution → high entropy

    • ex: gas particles dispersed throughout

  • as time moves forward, matter and energy become more dispersed → increases total entropy of universe

Predicting entropy changes

• doubling number of particles also increases opportunity for matter/energy to be dispersed

  • doubling amount of a substance → entropy doubles

• solid state → most ordered state with least dispersal → low entropy

  • increasing entropy: solid→liquid, solid→gas, liquid→gas

  • decreasing entropy: liquid→solid, gas→solid, gas→liquid

• change due to number of particles (in gaseous state) is usually greater than any possible factor

Absolute entropy

• entropy of a substance under standard conditions → section 13

• all entropy values are positive

  • a perfectly ordered solid at absolute zero has an entropy of zero

Calculating entropy changes

• calculated using differences between total entropy of the products and total entropy of the reactants

  • ΔS = ΔS_products - ΔS_reactants

• calculations similar to enthalpy changes

  • entropy values are absolute values → always positive

Entropy changes of surroundings

• to consider the total entropy change of a reaction, the entropy change in surroundings must also be considered

  • in an exothermic reaction, heat is transferred to the surroundings → general dispersal of energy

• entropy of the surroundings increases as heat given out by reaction increases diispersal of surroundings

• change in entropy of surroundings = enthalp ychange in the system x -absolute temperature

  • ΔS_surroundings = -ΔH_system/T

  • exothermic reaction (-ΔH) increases entropy of surroundings

Calculating total entropy changes

• second law of thermodynamics says that for a spontanous change:

  • ΔS_total = ΔS_system + ΔS_surroundings > 0

  • ΔS_total = ΔS_system - ΔH_system/T > 0

• endothermic reactions occur if change in entropy of system can compensate for negative entropy change of surroundings produced as the heat is transferred from surroundings to the system

  • strongly endothermic reactions are possible because there is a very large increase in dispersal of matter and entropy of the system

• order may increase in local areas but only at the expense of greater disorder elsewhere

  • for chemical reactions, neither ΔH_system or ΔS_system can reliably be used to predict the feasability of a reaction

Gibbs energy

• criterion or feasability of a reaction is given by:

  • ΔS_total = ΔS_system - ΔH_system/T > 0

  • ΔG_system = ΔH_system - TΔS_system = -TΔS_total < 0

  • ΔG_system → Gibbs energy

    • must be negative for a spontaenous process

      • for spontaneity, reaction must have ΔG_system < 0

    • measure of quality of energy available

• measure of energy free to do work rather than leave as heat

  • spontaneous reactions have negative Gibbs energy because they can do useful work

• it is not essential for all heat to be transferred to surroundings to produce the necessary increase in the total entropy

  • enough energy must be transferred to surroundings to compensate for entropy decrease in the system, but the remaining energy is available to do work

    • this is the amount of energy that can be converted to electrical energy in a fuel cell

  • necessary energy transferred to surroundings = -TΔS_system

  • energy available to do work = -ΔH_system + TΔS_system = -ΔG_system

• ΔG = ΔH - TΔS

  • 

• ΔG is related to total energy change and this is just a reformulation of the 2nd law of thermodynamics

  • ΔG takes into account direct entropy change from transformation of chemicals in the system and indirect entropy change of surroundings resulting from the transfer of heat energy

• ΔH_system < TΔS_system (T is always positive)

  • at low temperatures (TΔS_system=0), this condition is met (exothermic) as ΔH_system<0

  • endothermic reactions (positive ΔS_system) can be spontaneous at higher temperatures

    • TΔS_system > ΔH_system

• temperature Tspontaneous at which an endothermic reaction becomes spontaneous can be determined from:

  • T_spontaneous * ΔS_system = ΔH_system

    • T_spontaneous = ΔH_system/ΔS_system

The effect of ΔH, ΔS, and T on spontaneity of the reaction

• 

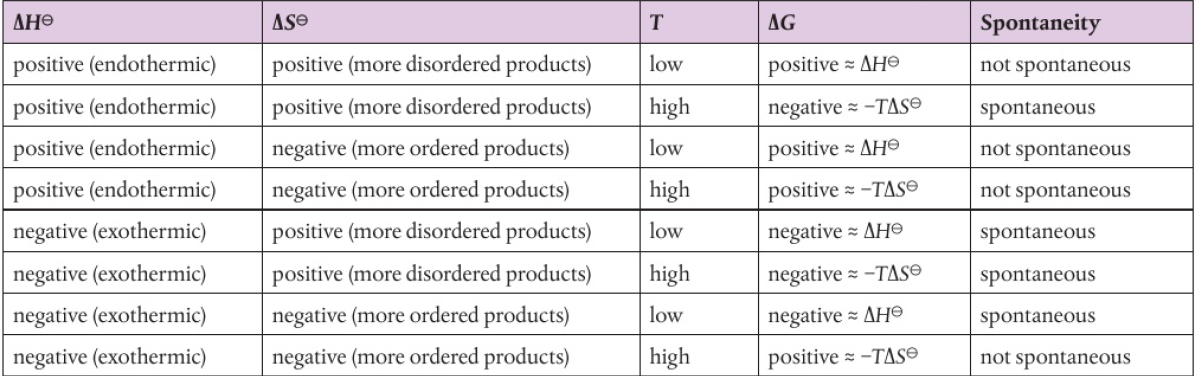
• ΔG_system = ΔH_system - TΔS_system

  • if ΔG<0, reaction is spontaneous so:

    • if ΔH_system > TΔS_system, reaction is spontaneous

    • so if T is high, most likely not spontaneous makes ΔH_system is high

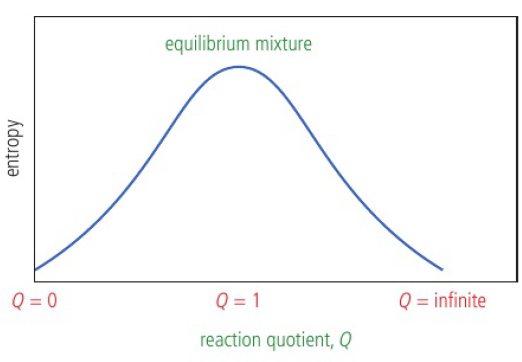
GIbbs energy and equilibrium

• only reactions where all reactants are formed into products have been considered

• equilibrium mixture when ΔG=0

  • spontaneous reactions only occur when ΔG<0, so when ΔG=0

  • a mixture of reactant and product has higher entropy than pure samples

    • total entropy reaches a maximum when reactant = product

  • reaction quotient (Q) → ratio of products to reactants

    • ex: Q=[products]/[reactants] so at beginning, Q=0 and at the end, Q=infinity

  • 

  • 

• equilibrium mixture when ΔG<0 (negative)

  • at beginning of reaction, total Gibbs energy of reactants > products so reaction proceeds in forward direction and Q increases (products increase, reactants decrease)

  • as reaction proceeds, Gibbs energy (system) decreases until equilibrium is reached (Q=K)

  • once equilibrium is reached, all possible changes are not likely to happen (ΔG increases)

  • position of equilibrium corresponds to a mixture with more products than reactants

  • 

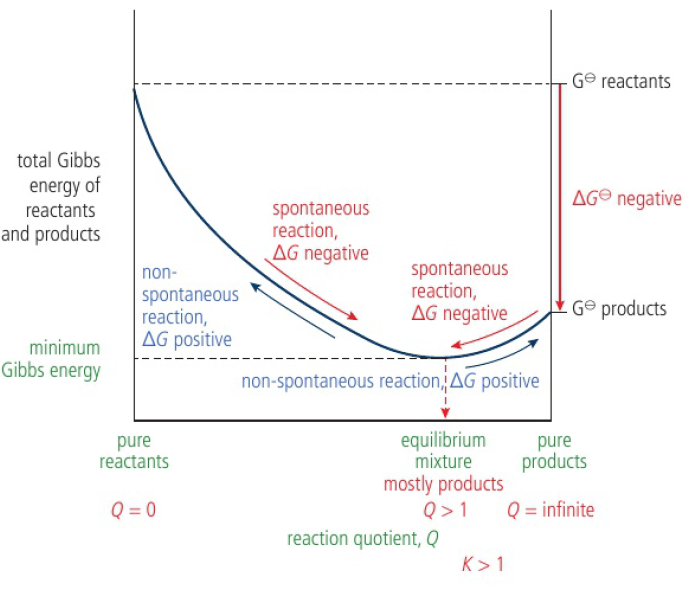
    • minimum Gibbs energy → equilibrium state, net reaction stops

    • relative amounts of reactants and products are at equilibrium

    • composition of equilibrium mixture is determined by the difference in Gibbs energy between reactants and products

    • K=[products_eqm]/[reactants_eqm] > 1 when ΔG<0

The equilibrium constant K

• relationship between K (equilibrium constant) and ΔG (change in Gibbs energy)

  • 

• so ΔG=-RT * lnK

• useful when K is difficult to measure directly

  • ex: reaction is too slow to reach equilibrium/amounts of components are too small to measure

• relationship between ΔG and extent of reaction:

  • 

2.1: How much? The amount of chemical change

Using chemical equations to find volumes of gaseous reactants and products

• Avogadro’s Law → equal amounts of all gases measured under the same conditions of temperature and pressure contain equal numbers of molecules

  • equal number of particles of all gases occupy equal volumes

  • V has a direct relationship with n

• volume occupied by one mole of any gas (molar volume, Vm) must be the same for all gases when measured under the same temperature and pressure

  • at STP, one mole of gas has a volume of 22.7dm³/mol

    • STP → OC (273K) and 100kPa

  • increase in temperature = increase in molar volume

  • increase in pressure = decrease in molar volume

• number of moles of gas (n) = volume/molar volume

Titration

• uses volumetric analysis to find unknown volumes or concentrations

• pipette used to measure known volume into a conical flask

• other solution put into a burette

  • point at which the two solutions have reacted completely → equivalence point

    • known when indicator changes color at the end point

• titre → volume needed to reach equivalence point

Back titration

• done in reverse by returning to the end point after it has passed

  • used when end point is hard to identify or when one of the reactants is impure

• known excess of one reactant is added to reaction mixture, and unreacted excess is then determined by titration against a standard solution

  • reacting amount is determined by subtracting the amount of unreacted reactant from its original amount used

Limiting reactant and theoretical yield

• limiting reactant → reactant that determines the quantity of product

  • always the one fully used up, other reactants are added in excess

• theoretical yield → maximum amount of product obtainable (assuming 100% of limiting reactants is used)

  • usually expressed in grams or moles

Percentage yield

• theoretical yield assumes that chemical reactions have no loss, waste, or impurities

  • experimental yield → actual yield with factors taken into account

• factors that may cause experimental yield to be lower than the theoretical yield:

  • side reactions occuring

  • decomposition of reactants and/or products

  • loss of product during purification

  • reversible chemical reactions preventing process completion

• factors that may cause experimental yield to be higher than the theoretical yield:

  • impurities in a product

  • when a product has not been fully dried

• factors that impact experimental yield in both directions (depending on type of reaction):

  • an incomplete reaction

• percentage yield = experimental yield/theoretical yield * 100

Atom economy

• Green Chemistry → sustainable design of chemical products and chemical processes

  • aims to minimize use of chemical substances that are hazardous to human health / the environment

• percentage yield does not give a quantity of waste produced

• atom economy is maximized by turning as much reactant atoms into products

• % atom economy = molar mass of desired product / molar mass of all reactants * 100

  • efficient processes have high atom economies → uses fewer resources and generates less waste

2.2: How fast? The rate of chemical change

Rate of reaction

• rate of reaction → rate of change in concentration

  • as the reaction proceeds, reactants are converted into products

    • concentration of reactants decrease and concentration of products increase

• rate of reaction (moldm³/s)= increase in product concentration / time taken = decrease in reactant concentration / time taken

  • if the line is a curve, use the gradient of the tangent

• rate of reaction is not constant, but is greatest at the start and decreases over time

Measuring rate of reaction

• change in volume of gas produced

  • used if one of the products is a gas

  • collecting the gas and measuring change in volume at regular time intervals

    • using a gas syringe or displacement of water in an inverted burette

    • displacement method can only be used if gas collected has low solubility in water

• change in mass

  • if one of the products is a gas, this can be done by setting the reaction on a scale

    • does not work if the gas is hydrogen → too light

• change in transmission of light: colorimetry/spectrophotometry

  • used if one of the reactants/products is colored (so gives characteristic absorption in the visible region)

    • sometimes indicator is added to make it a colored compound

  • colorimeter/spectrophotometer measures the intensity of light transmitted by reaction components

  • rate of product formation → change in absorbance

• change in concentration → titration

  • quenching → a substance is introduced that effectively stops the reaction, obtaining a “freeze frame” shot

    • done to avoid chemically changing the reaction mixture

    • samples are taken from the reaction mixture at regular time intervals and analyzed by titration

    • titration takes time, during which the reaction would proceed → quench

• change in concentration using conductivity

  • total electrical conductivity of a solution depends on the total concentration of its ions and charges

  • measured using a conductivity meter

• non-continuous methods of detecting change during a reaction: ‘clock reactions’

  • measure time it takes for the reaction to reach a certain chose point

    • uses time as the dependent variable

    • limitation: only gives average rate of reaction

Collision theory

• particles in a substance move randomly as a result of their kinetic energy

  • not all particles will have the same kinetic energy, but instead a range

    • therefore the measurement is an average

• increasing temperature = increasing average kinetic energy of particles

  • kinetic theory of matter (S1.1)

• Maxwell-Boltzman energy distribution curve

  • DIAGRAM HERE

  • the number of particles having a specific value of kinetic energy (or probability of that value occuring) against values of kinetic energy

  • area under the curve → total number of particles in sample

• nature of collisions between particles

  • when reactants are placed together, their kinetic energy cause them to collide

  • energy from collisions may cause bonds to break and new bonds to form

  • as a result, products ‘form’ and the reaction stops

• rate of reaction depends on the number of successful collisions which form products

  • successful collisions depend on:

    • energy of collision

    • geometry of collision

• energy of a collision

  • particles must have the required activation energy (E_a) necessary for overcoming repulsion between molecules, and often breaking bonds in reactants

  • when Ea is supplied, reactants achieve the transition state from which products can form

  • activation energy is thus an energy barrier for the reaction → different for all reactions

  • E_a → threshold value

    • if you pass, you may react

  • DIAGRAM HERE → activation energy

    • particles with E_k>=E_a will collide successfully

    • particles with E_k<E_a may still collide, but unsuccessfully

  • therefore, rate of reaction depends on proportion of particles that has E_k>E_a

  • DIAGRAM HERE → Maxwell curve activation energy

  • generally, reactions with high activation energy will proceed more slowly as fewer particles will have the required energy for a successful collision

• geometry of a collision

  • DIAGRAM HERE → different collisions

  • because collisions between particles are random, there are many likely orientations → only some are successful

• therefore, rate of reaction is determined by:

  • values of kinetic energy greater than activation energy

  • appropriate collision geometry

Factors that influence the rate of reaction

• temperature

  • increasing temperature increases average kinetic energy of particles

  • DIAGRAM HERE → Maxwell curve

  • area under both curves is the same → same number of particles

  • at higher temperature, more particles have higher kinetic energies so the peak of the curve shifts rightwards

  • as temperature increases, collision frequency increases due to higher kinetic energy → more collisions involving particles with necessary activation energy

  • therefore, more successful collisions (every +10K, reaction rate doubles)

• concentration

  • increasing concentration increases frequency of collisions between reactants → more successful collisions

  • as reactants are used up, the concentration decreases and the rate of reaction decreases

• pressure

  • increasing pressure “compresses” the gas, effectively increasing concentration

• surface area

  • increasing surface area allows for more contact and a higher probability of collisions

    • instead of one big chunk, divide it into smaller sections to increase total surface area

    • stirring can increase total surface area by ensuring individual particles are spread

• catalyst → a substance that increases rate of reaction without itself undergoing chemical change

  • most catalysts work by providing an alternative route for the reaction that has lower activation energy

  • DIAGRAM HERE → uncatalyzed reaction, catalyzed reaction

  • without increasing temperature, more particles will have E_k>E_a, so will be able to undergo successful collisions

  • catalysts equally reduce E_a for both forward and reverse reactions, so does not shift equilibrium or yield

  • DIAGRAM HERE → Maxwell curve

  • catalysts increase efficiency, and there are “best” catalysts for certain reactions → otherwise reactions move too slowly or are conducted at too high temperatures

Catalysts

• every biological reaction is controlled by a catalyst → enzyme

  • there is a specific enzyme for every particular biochemical reaction

• biotechnology → field that searches for possible applications of certain enzymes

• catalysts can replace stoichometric reagants → greatly enhances selectivity of processes

  • therefore, important aspect of Green Chemistry

• catalysts are effective in small quantities and can frequently be reused

  • therefore do not contribute to chemical waste → increases atom economy

Reaction mechanisms

• most reactions that occur at a measurable rate occur as a series of simple steps, each involving a small number of particles

  • this sequence of steps is known as the reaction mechanism

  • the individual steps (elementary steps) usually cannot be observed directly

    • therefore this is only a theory → cannot be proved (but there are clues)

• often the products of a single step in the mechanism are used in a subsequent step

  • exists only as reaction intermediates, not as final products

  • ex: NO₂(g) + CO(g) → NO(g) + CO₂(g)

    • mechanism follows these elementary steps:

      1. NO₂(g) + NO₂(g) → NO(g) + NO₃(g)

      2. NO₃(g) + CO(g) → NO₂(g) + CO₂(g)

      3. overall reaction: NO₂(g) + CO(g) → NO(g) + CO₂(g)

    • reactants and products cancel out → reaction intermediates

    • NO₂ in reactants in step 1 and products in step 2 cancel out

    • NO₃ in products in step 1 and reactants in step 2 cancel out

• molecularity → used in reference to an elementary step to indicate number of reactant species involved

  • unimolecular → elementary step that involves a single reactant particle

  • bimolecular → elementary step with two reactant particles

  • trimolecular reactions are rare → extremely low probability of >2 particles colliding at same time with sufficient energy and correct orientation

Rate-determining step

• the rate-determining step is the slowest step in the reaction mechanism

  • products of the reaction can only appear as fast as the products of this slowest elementary step

    • rate-determining step therefore determines overall rate of reaction

• DIAGRAM HERE → reaction coordinate, potential energy

  • two maxima represent the transition states

  • minimum represents the intermediate species

  • in this example, first maxima (first step) is higher, so more activation energy required → thus slowest step, so rate-determining

  • catalysts usually find an alternative for the slowest step to speed up the reaction (rate-determining step made faster or changes)

Rate equations

• rate equations are determined experimentally and depend on the mechanism of a reaction

  • consider the reaction: C₆₀O₃ → C₆₀O + O₂

  • we can follow the reaction by recording the change in absorbance of light of a certain wavelength

    • absorbance is directly proportional to concentration of C₆₀O₃

  • rate of reaction is equal to the rate of change in concentration of C₆₀O₃

    • rate=- [C₆₀O₃]/t (negative because concentration is decreasing)

    • rate can be calculated by finding gradient of line’s tangent at a specific point

  • rate slows down as concentration of C₆₀O₃ decreases

  • similarities in concentration VS time and rate VS time graphs suggests that the rate must be related ot concentration at each time

  • straight-line graph between absorbance and rate confirms that the rate of reaction is directly proportional to concentration of C₆₀O₃

    • reaction rate is directly proportional, so reaction rate = k[C₆₀O₃]

      • k is the rate constant

    • this equation is a rate equation → first order rate equation because the concentration of the only reactant is raised to the first power

• rate of all reactions can similarly be shown to depend on concentration of one or more of the reactants, and the particular relationship depends on the reaction

• generally, rate is proportional to products of concentrations of reactants, each raised to a power

  • A+B → products so rate=k[A]^m[B]ⁿ

    • m and n are known as the orders of the reaction with respect to A and B

    • overall reaction order is sum of individual orders (m+n)

    • orders can only be determined by experiment (empirically)

    • no connection between reaction equation (coefficients, moles) and rate equation

Rate equation and reaction mechanism

• as the rate of reaction depends on the rate-determining step, the rate equation for the overall reaction must depend on the rate equation for the rate-determining step

  • because the rate-determining step is an elementary step, its rate equation comes directly from its molecularity:

    • A → products: unimolecular, so rate=k[A]

    • 2A → products: bimolecular, so rate=k[A]²

    • A+B → products: bimolecular, so rate=k[A][B]

  • rate equation for rate-determining step, predictable from its reaction equation, leads to the rate equation for the overall reaction

    • when rate-determining step is not the first step, the intermediate cannot be used in the rate equation → instead, substitute

  • order of reaction with respect to each reactant is not linked to coefficients in overall equation, but is instead determined by their coefficients in the equation for the rate-determining step

Order of a reaction

• reaction that is zero-order with respect to a particular reactant → the reactant is required for reaction but does not affect rate as it is not present in the rate-determining step

  • if a reactant is present in the rate-equation, it partakes in the rate-determining step

• reaction order can be fractional or negative in more complex reactions

• concentration-time graphs do not give a clear distinction between first and second order

  • rate-concentration graphs clearly reveal the difference

• zero-order: rate=k[A]⁰=k

  • DIAGRAM HERE

  • concentration-time → straight line, constant rate

    • gradient of line = k

  • rate-concentration → horizontal line

• first-order: rate=k[A]

  • DIAGRAM HERE

  • concentration-time → rate decreases with concentration

  • rate-concentration → straight line passing through origin with gradient k

• second-order: rate=k[A]²

  • DIAGRAM HERE

  • concentration-time → curve, steeper at start than first-order graph but leveling off more quickly

  • rate-concentration → parabola (square function), gradient proportional to concentration and initially zero

• order of reaction can only be determined experimentally, thus these graphs are required to distinguish them

Determination of the overall order of a reaction

• methods for determining order of reaction depends on the reactants

  • two methods, but only initial rate method is covered

• initial rates method

  • carrying out a number of separate experiments with different starting concentrations of reactant A, and measuring the initial rate of each reaction

    • concentration of other reactants are held constant to see effect of A on reaction rate

  • changing concentration of A but no effect on rate → zero order with respect to A

  • changing concentration of A produces directly proportional changes in rate of reaction → first order with respect to A (doubling concentration of A doubles reaction rate)

  • changing concentration of A leads to the square of that change in the rate → second order with respect to A (doubling concentration of A leads to a four-fold increase in reaction rate)

• use of the integrated form of the rate equation

  • calculus is used to analyze the integral of rate equation

    • direct graphical analysis of functions of concentration against time

The rate constant, k

• units of k vary with order of reaction

  • zero order: rate=k, k=moldm³/s

  • first order: rate=k[A], k=rate/concentration=s^-1

  • second order: rate=k[A]², k=dm³/mols

  • third order: rate=k[A]³, k=dm⁶/mol²s

• k is temperature dependent → general measure of rate of a reaction at a particular temperature

  • temperature dependence of k depends on value of activation energy

    • high E_a → temperature rise causes significant increase in particles that can react

    • low E_a → same temperature rise will have proportionally smaller effect on reaction rate

  • temperature dependence of k is expressed in the Arrhenius equation

The Arrhenius equation

• Suante Arrhenius showed that the function of molecules with energy greater than the activation energy at temperature T is proportional to e^-E_a/RT (R is gas constant)

  • reaction rate and therefore rate constant are also proportional to this value

  • k=Ae^-E_a/RT

    • A → Arrhenius factor (frequency factor, pre-exponential factor)

    • A takes into account the frequency of successful collisions based on collision geometry

    • A is a constant for a reaction and has same units as k (so varies with order)

• Arrhenius plot → lnk=-E_a/RT + lnA

  • rule of thumb → 10K increase doubles reaction rate

2.3: How far? The extent of chemical change

Dynamic equilibrium

• reaction takes place at same rate as its reverse reaction, so no net change is observed

• physical systems (ex: bromine in a sealed container at room temperature)

  • bromine is a volatile liquid (boiling point close to room temperature)

  • significant amount of Br₂ molecules will have enough energy to leave the liquid state (evaporate)

    • container is sealed so bromine vapour cannot escape → concentration increases

    • some vapour molecules will collide with surface of liquid, lose energy, and become liquid

  • Br₂(l) ⇌ Br₂(g)

  • rate of condensation increases with concentration of vapour (more vapour particles)

    • eventually, rate of condensation will equal rate of evaporation

    • no net change → equilibrium (only occurs in a closed system)

  • DIAGRAM HERE → rate of condensation = rate of evaporation

• chemical systems (ex: dissociation between hydrogen iodide (HI) and its elements (H₂, I₂)

  • 2HI(g) ⇌ H₂(g) + I₂(g)

  • colourless gas ⇌ colourless gas + purple gas

  • there will be an increase in purple hue when the reaction starts (production of I₂)

  • at some point, the increase in colour will stop

    • rate of dissociation of HI is fastest at the start as the concentration of HI is the greatest, then falls as the reaction proceeds

    • reverse reaction had initially zero rate (no H₂ or I₂ present) then starts slowly and increases in rate as concentrations of H₂ and I₂ increase

  • eventually, the rate of the forward and reverse reactions will equal, so concentrations remain constant

    • equilibrium → dynamic because both reactions are still occuring

  • if the contents of the flask were analyzed at this point, HI, I₂, and H₂ would all be present with constant concentrations → equilibrium mixture

  • DIAGRAM HERE → equilibrium

    • if the experiment were reversed (starts with H₂ and I₂), eventually an equilibrium mixture will again be reached

• reactants ⇌ products

  • → forward, ← backward

• constant concentrations of products and reactants does not mean equal amounts

  • equilibrium position → proportion of reactant and product in equilibrium

    • predominantly products → lie to the right

    • predominantly reactants → lie to the left

Equilibrium Law

• consider the reaction: H2(g) + I2(g) ⇌ 2HI(g)

  • if we were to carry out a series of experiments on this reaction with different starting concentrations of H2, I2, and HI, we could wait until each reaction reached equilibrium and then measure the composition of each equilibrium mixture

  • there is a predictable relationship among the different compositions of these equilibrium mixtures

    • [HI]²/[H2][I2] → concentration at equilibrium ([HI] is squared because that is its coefficient in the equation)

    • K → constant value, equilibrium constant (fixed value at specified temperature)

    • every reaction has its particular value of K

• equilibrium constant expression for reaction: aA + bB ⇌ cC + dD

  • K = [C]_eqm^c[D]_eqm^d / [A]_eqm^a[B]_eqm^b

    • [A] → concentration, a → coefficient in reaction equation, products → numerator, reactants → denominator

  • high value of K → at equilibrium, proportionally more products than reactants

    • lies to the right, reaction almost to completion

  • K values tells differing extents of reactions

    • higher value = reaction has taken place more fully

  • K » 1: reaction almost goes to completion (right)

  • K « 1: reaction hardly proceeds (left)

Le Chatelier’s principle

• a system at equilibrium when subjected to a change will respond in such a way as to minimize the effect of the change

  • whatever done to a system at equilibrium, it will respond in the opposite way

  • after a while, a new equilibrium will be established with different composition

• changes in concentration

  • equilibrium mixture disrupted by increase in concentration of a reactant:

    • rate of forward reaction increases: forward =/ backward anymore

    • equilibrium will have shifted in favour of products (rightward)

    • value of K remains unchanged

  • same happens with decrease in concentration of product

    • rate of backward reaction decreases → new equilibrium position will be achieved (rightward)

  • often in industrial processes, product will be removed as it forms

    • ensures equilibrium is continuously pulled rightward → increasing yield of product

• changes in pressure

  • equilibria involving gases will be affected if there is a change in the number of molecules

    • there is a direct relationship between pressure exerted by gas and the number of gas particles

  • increase in pressure → system response: decrease pressure by favouring the side with less molecules

    • new equilibrium position, K remains unchanged (if temperature does not change)

  • ex: CO(g) + 2H₂(g) ⇌ CH₃OH(g)

    • increase in pressure shifts equilibrium rightward → in favour of smaller number of molecules

    • increase in pressure → increases yield of CH₃OH

  • if number of molecules are the same for both sides, pressure will not change equilibrium

• changes in temperature

  • K is temperature dependent → changing temperature affects K

  • ex: 2NO₂(g) ⇌ N₂O₄(g) ΔH=-57kJ/mol (forward reaction exothermic)

    • decrease in temperature → system produces heat → favours forward exothermic reaction

    • new equilibrium mixture (rightward) → K increases (higher yield at lower temperature)

    • increasing yield takes too long→ decreasing temperature lowers rates of reactions

• addition of a catalyst

  • catalyst speeds up rate of reaction by providing alternative reaction pathway with a transition state with a lower activation energy

    • increases number of particles that have sufficient energy to react (without increasing temperature)

  • because both forward and backward reactions pass through the same transition state, both rates will increase → no change in equilibrium position and K

    • will not increase equilibrium yield of a product

  • speeds up attainment of equilibrium state → products form more quickly

  • has no effect in equilibrium concentrations → not chemically changed

The reaction quotient, Q

• K is calculated using concentrations at equilibrium

  • Q → calculated using concentrations when not at equilibrium

  • as time passes and reaction proceeds, concentrations will change and eventually reach equilibrium

    • Q changes in direction of K → used to predict direction of reaction

    • if Q=K, reaction at equilibrium, no net reaction occurs

    • if Q<K, reaction proceeds rightward in favour of products

    • if Q>K, reaction proceeds leftward in favour of reactants

Quantifying the composition at equilibrium

• done by calculating equilibrium constant (K) or concentration of reactants/products

  • only homogeneous equilibria → all reactants/products in the same phase (gas or solution)

• equilibrium law can be used to solve for K and initial/final concentrations

Measuring the position of equilibrium

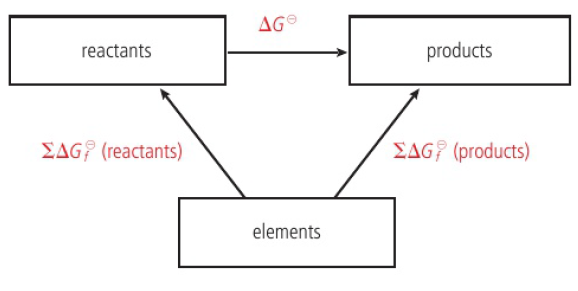
• Gibbs energy change can be used to measure the position of equilibrium

  • ΔG → measure of work available from a system calculated for a particular composition of reactants and products (ΔG=Gproducts-Greactants)

  • ΔG=negative → reaction proceeds in forward direction

  • ΔG=positive → reaction proceeds in backward direction

  • ΔG=0 → reaction is at equilibrium (Gproducts=Greactants)

• at the start of a reaction, total Gibbs energy of reactants is greater than products (lot of work is available) → ΔG=negative, reaction proceeds in forward direction

  • as reaction proceeds, total GIbbs energy of reactants decreases but of products increase

    • ΔG less negative, less work is available

  • system reaches equilibrium when total Gibbs energy of reactants and products are equal

    • no work can be extracted from system (ex: dead battery)

• total Gibbs energy decreases as reaction progresses as work is done by the system

  • occurs both when reaction starts with reactants and products

  • equilibrium state → net reaction stops → minimum value of Gibbs energy

  • DIAGRAM HERE → equilibrium

  • DIAGRAM HERE → equilibrium

• decrease in total Gibbs energy appears as work done or increase in entropy

  • system has highest possible value of entropy when Gibbs energy at minimum (at equilibrium)

• reaction with large and negative ΔG value → spontaneous, equilibrium with high products

• reaction with large and positive ΔG value → non-spontaneous, predominantly reactants

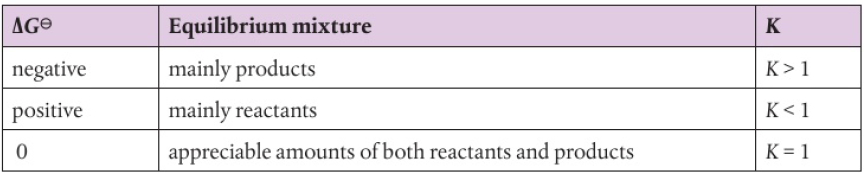
• ΔG=-RT*lnk

  • ΔG negative, lnK positive, K>1: equilibrium mainly products

  • ΔG positive, lnK negative, K<1: equilibrium mainly reactants

  • ΔG=0, lnK=0, K=1: appreciable amounts of both reactants and products

Rate of reaction and equilibrium

• ex: reaction that occurs in a single step

  • A + B ⇌ C + D

    • rate of forward reaction: k[A][B]

    • rate of backward reaction: k’[C][D]

    • K=[C][D]/[A][B] (equilibrium constant)

  • at equilibrium, rate of forward reaction = rate of backward reaction

    • k[A][B]=k’[C][D]

    • rearranging gives: K=k/k’

  • if k»k’, K is large → reaction proceeds to completion

  • if k«k’. K is small → reaction barely takes place

  • increasing concentration of reactants speeds up forward reaction (vice versa)

    • shifts equilibrium rightward

    • equilibrium constant stays the same regardless

  • adding a catalyst increases k and k’ by same factor → K stays the same

  • k=Ae^-E_a/RT → activation energies of forward and backward reactions are different

    • differently affected by temperature

  • for endothermic reactions (E_a(forward) > E_a(backward)), increasing temperature will have greater effect increasing k than k’, so K increases

3.1: Proton transfer reactions

• H⁺ is equivalent to a proton

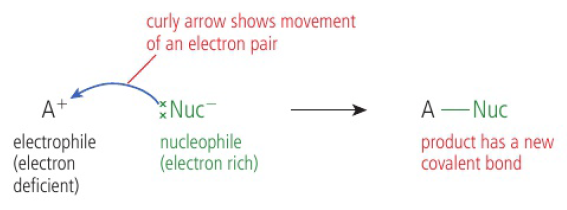
• proton is transferred when:

  • reactant loses H⁺ so loses positive charge

  • product gains H⁺ so gains positive charge

  • this type of reaction can only occur between certain species: reactants that can release H⁺ and products with a lone pair that can accomodate an additional H⁺

    • Bronsted-Lowry acid-base behavior

Bronsted-Lowry acids and bases

• acids donate H⁺, bases accept H⁺

  • Bronsted-Lowry acid is a proton (H⁺) donor

  • Bronsted-Lowry base is a proton (H⁺) acceptor

• act of donating cannot act in isolation, must always have an acceptor

  • an acid can only be a proton donor if a base is present to accept it

• ex: HA + B ⇌ A^- + BH⁺

  • HA acts as an acid, donating a proton to B → B acts like a base, accepting it

    • in reverse reaction, BH⁺ is the acid while A^- acts as a base

  • acid HA has reacted to form base A^- and base B reacted to form acid BH⁺

    • HA and A^-, B and BH⁺ → conjugate acid-base pairs

• conjugate acid-base pairs differ by one proton

  • acid always has one more proton than its conjugate base (H⁺)

    • ex: H₃O⁺ → H₂O, NH₃ → NH₂^-

• most polyatomic ions can form Bronsted-Lowry acids by accepting H⁺ (ex: OH^-, NO₃^-)

  • ammonium (NH₄⁺) cannot accept H⁺ to form an acid, instead loses H+

    • acts as a Bronsted-Lowry acid and forms its conjugate base

• Bronsted-Lowry bases are defined as any species which can accept a proton

  • a small group of Bronsted-Lowry bases (alkalis) are soluble bases which dissolve in water to release the hydroxide ion (OH^-)

Amphiprotic species

• some species can act as both Bronsted-Lowry acids and bases (ex: water)

  • these species are amphiprotic

  • depends on what the species is reacting with

• amphiprotic substances must possess both a lone pair of electrons (to accept H⁺) and hydrogen that can be released as H⁺ (to dissociate)

• moving left to right across a period: basic metal oxides through amphiprotic oxides to acidic oxides

  • ex: period 3

    • Na₂O, MgO → basic

    • Al₂O₃ → amphoteric

    • SiO₂, P₄O₁₀, SO₂, Cl₂O → acidic

The pH scale

• measure of acid strength based off its H+ concentration

  • pH = log10[H⁺] or [H⁺] = 10^-pH

    • ex: a solution with [H⁺] = 0.1mol/dm³ → 10^-1mol/dm³ → -(-1)pH=1pH

  • most common acids and bases will have a positive pH in the range 0-14

  • pH has no units and is inversely related to [H⁺]

  • DIAGRAM HERE

    • for each increase of 10 times in [H⁺]. pH will decrease by 1 unit

• pH is measured by estimating using universal indicator paper or solution

  • substance tested will give a characteristic colour → compared to indicator’s colour chart

• pH is measured using a pH meter that directly reads the H⁺ concentration using a special electrode

  • must be calibrated before use (pH is temperature-dependent)

Ionization of water

• majority of acid-base reactions involve ionization in aqueous solution

  • water does ionize, only very slightly at normal temperatures and pressures

  • H₂O(l) ⇌ H⁺_(aq) + OH^-_(aq)

    • K=[H⁺][OH^-]/[H₂O] → concentration of water is considered constant as so little of it ionizes

    • K_w=[H⁺][OH^-] → ionic product constant of water

    • at 298K, K_w=1×10^-14

  • H⁺ in aqueous solution always exists in H3O⁺_(aq)

  • in pure water, [H⁺]=[OH^-], so [H⁺]=√K_w

    • [H⁺]=1×10^-7, pH=7 → neutral

• [H⁺] x [OH^-] is constant, so they have an inverse relationship

  • when one increases, the other decreases

  • acidic: [H⁺]>[OH^-], pH<7

  • neutral: [H⁺]=[OH^-], pH=7

  • alkaline: [H⁺]<[OH^-], pH>7

• K_w is an equilibrium constant → temperature dependent

  • increasing temperature shifts equilibrium rightward → increases K_w

    • [H⁺] and [OH^-] increase → pH decreases

  • decreasing temperature shifts equilibrium leftward → decreases K_w

    • [H⁺] and [OH^-] decrease → pH increases

  • pH of water is 7.00 only at 298K

    • still neutral regardless of temperature because [H⁺]=[OH^-]

Strong and weak acids and bases

• Bronsted-Lowry acids and bases dissociate in solution

  • acids produce H⁺ ions and bases produce OH^- ions

  • aqueous solutions exist as equilibrium mixtures containing both undissociated form and its ions

• ex: HA_(aq) + H₂O_(l) ⇌ A^-_(aq) + H₃O⁺_(aq)

  • if this acid dissociates fully, its equilibrium is positioned at the right → exists virtually entirely of ions

    • this is said to be a strong acid

  • if the acid dissociates only partially, equilibrium lies to the left → undissociated form dominates

    • this is said to be a weak acid

• ex: HCl_(aq) + H₂O_(l) → H₃O⁺_(aq) + Cl^-_(aq)

  • strong acid + base → conjugate acid + conjugate base

  • strong acids are good proton donors

    • because their dissociation reaction goes almost to completion, their conjugate bases do not readily accept protons

    • in this example, the strong acid HCl reacts to form conjugate base Cl^- which shows no basic properties

  • weak acids are poor proton donors

    • conjugate bases readily accept protons

  • acid dissociation reactions favour the production of the weaker conjugate

• ex: NaOH_(aq) → Na⁺_(aq) + OH^-_(aq)

  • NaOH is a strong base because it ionizes fully

  • strong bases are good proton acceptors → react to form conjugates that show no acidic properties

    • base ionization reactions favour the production of the weaker conjugate

• strength of an acid or base is a measure of how readily it dissociates in aqueous solution

• TABLE HERE → common strong and weak acids and bases

• since strength of an acid depends on the ease with which it dissociates to release H⁺ ions, it thus depends on the strength of the bond that has to be broken to release H⁺

  • longer bond = weaker bond = stronger acid

    • thus acid strength of hydrogen halides increase down the group (HF<HCl<HBr<HI)

• distinguishing strong/weak acids/bases → strong acids/bases will contain a higher concentration of ions

  • comparisons only work when solutions of the same concenration are compared at the same temperature

• electrical conductivity

  • depends on concentration of mobile ions (solution)

  • strong acids/bases will show higher conductivity

    • tested using a conductivity meter, probe, or pH meter with conductivity setting

• rate of reaction

  • stronger acids would have an increased rate

• pH

  • the stroner the acid, the lower the pH value

    • measured using universal indicator or pH meter

Neutralization reactions

• reaction between an acid and base (H⁺ + OH^- → H₂O) is a neutralization reaction

  • during the reaction, an ionic compound (salt) forms → hydrogen in acid is replaced by a metal/positive ion

    • parent acid, parent base → relationship between an acid, a base, and their salt

• when acids react with reactive metals, a salt is also formed (metal ion replaces hydrogen)

  • no H⁺ transfer, hydrogen reduced as gas (H₂)

  • hydrogen ions are becoming electrically neutral by accepting electrons

  • metal is being ionized by electron loss

  • ex: Mg_(s) + 2HCl_(aq) → MgCl_2(aq) + H_2(g)

    • Mg_(s) → Mg²⁺_(aq) + 2e^- (electron loss → oxidation)

    • 2H⁺_(aq) + 2e^- → H_2(g) (electron gain → reduction)

    • redox reactions, cannot be described by Bronsted-Lowry acid-base theory

• acid + base → salt + water

  • metal oxides/hydroxides are bases which react with acids to produce a salt and water

    • ex: HCl_(aq) + NaOH_(aq) → NaCl_(aq) + H₂O_(l)

  • ammonia solution is also a soluble base which reacts with acids to form a salt and water

    • ex: HNO_3(aq) + NH₄OH_(aq) → NH₄NO_3(aq) + H₂O_(l)

  • when choosing a base in solution to make a specific salt, use solubility rules:

    • only soluble carbonates/hydrogencarbonates are: NH₄CO₃, Na₂CO₃, K₂CO₃, KHCO₃, Ca(HCO₃)₂

    • only soluble hydroxides are: NH₄OH, LiOH, NaOH, KOH

• acid + carbonate → salt + water + carbon dioxide

  • metal carbonates/hydrogencarbonates react with acids to produce a salt, water, and carbon dioxide

    • ex: 2HCl_(aq) + CaCO_3(aq) → CaCl_2(aq) + H₂O_(l) + CO_2(g)

    • 2H⁺_(aq) + CO₃^2-_(aq) → H₂O_(l) + CO_2(g)

    • H⁺_(aq) + HCO3^-_(aq) → H₂O_(l) + CO_2(g)

  • gas is given off, visibly produces bubbles → effervescence

• neutralization reactions are exothermic

  • net reaction is formation of H₂O from its ions (H⁺_(aq) + OH^-_(aq) → H₂O_(l))

    • other ions (spectator ions) do not change during reaction → can be cancelled out

  • for reactions between strong acids/bases, enthalpy of neutralization → H=-57kJ/mol

    • expressed per mole of H₂O formed → overall reaction is formation of water

pH curves

• following examples all use (for better comparison):

  • 0.10 mol/dm³ solutions for all acids and bases

  • initial volume of 50cm³ of acid in conical flask, base added with burette

  • monoprotic acids and bases → reacts in 1:1 ratio, equivalence is achieved at equal volumes for these equimolar solutions (i.e. when 50cm³ of base has been added to 50cm³ of acid)

• acid-base titration → controlled volumes of one reactant are added gradually from a burette to a fixed volume of the other reactant that has been measured using a pipette and placed in a conical flask

  • reaction between acid and base takes place in flask until the equivalence/stoichometric point is reached

    • until they exactly neutralize each other

    • pH meter or indicator is used to detect the exact point equivalence is reached

• when a base is added to an acid in a neutralization reaction, there is a change in pH

  • change is not linear (mostly due to logarithmic nature of pH) → shown on pH curve

  • in most titrations, a big jump in pH occurs at equivalence (point of inflection)

    • at equivalence, acid and base exactly neutralized each other → solution is only salt and water

• ex: HCl_(aq) + NaOH_(aq) → NaCl_(aq) + H₂O_(l) (strong acid + strong base)

  • full dissocation is assumed → both acid and base are strong

  • when base is added, volume also increases → changing concentration, changing pH

  • DIAGRAM HERE

    1. initial pH=1 → pH of strong acid

    2. pH changes only gradually until equivalence

    3. very sharp jump in pH at equivalence (3→11)

    4. after equivalence, flattens out at a high value → pH of strong base

    5. pH at equivalence (pH=7)

    • measured by continuously using a pH probe

• weak acid + strong base

  • DIAGRAM HERE

    1. initial pH fairly high (pH of weak acid)

    2. pH stays relatively constant until equivalence → buffer region

    3. jump in pH at equivalence (not as much of a jump as strong acid)

    4. after equivalence, curve flattens out at high pH (pH of strong base)

    5. pH at equivalence > 7

       • anion hydrolysis releases OH^-

  • point where 25/50cm³ of base is added → half-equivalence point (half of the acid has been neutralized)

    • mixture has equal quantities of a weak acid and its salt → buffer solution

      • buffer region → pH is relatively resistant to change

    • at this point, pH=pK_a because [acid]_eqm=[HA]_initial so [salt]_eqm=[A^-]_eqm

• strong acid + weak base

  • DIAGRAM HERE

    1. initial pH=1 → strong acid’s pH

    2. pH remains relatively constant through buffer region until equivalence

    3. jump in pH at equivalence (pH 3-7)

    4. curve flattens out after equivalence at fairly low pH (pH of weak base)

    5. pH at equivalence < 7

• weak acid + weak base

  • pH at equivalence is difficult to define

  • DIAGRAM HERE

    1. initial pH is fairly high (weak acid)

    2. addition of base causes pH to rise steadily

    3. change in pH at equivalence is much less sharp than other titrations

    4. after equivalence, curve flattens out at fairly low pH (weak base)

  • there is no defined pH at equivalence because there are several equilibria involved

The pOH scale

• pH scale simplifies [H⁺], pOH simplifies [OH^-]

  • OH^- ions are often found in low concentrations in solutions

  • pOH = -log₁₀[OH^-], pH = -log₁₀[H⁺]

• since [H⁺][OH^-] = K_w = 1×10^-14 at 298K:

  • pH + pOH = 14.00 at 298K

• pK_w = -log₁₀(K_w)

  • K_w = 10^-pK_w

  • pH + pOH = pK_w

Dissociation constants

• weak acids and bases do not fully dissociate → concentrations of ions in solutions cannot be deduced from initial concentrations, depends on extent of dissociation

  • dissociation of weak acids and bases are represented as equilibrium expressions

• ex: HA_(aq) + H₂O_(l) ⇌ H₃O⁺_(aq) + A^-_(aq) (weak acid HA dissociating in water)

  • K=[H₃O⁺][A^-] / [HA][H₂O] → concentration of water is constant

  • K_a=[H₃O⁺][A^-] / [HA] → acid dissociation constant

    • fixed value for a particular acid at a specified temperature

• the higher the value of K_a at a particular temperature, the greater the dissocation, so the stronger the acid

  • because K_a is an equilibrium constant, it is not dependent on the acid’s concentration or other ions

• ex: B_(aq) + H₂O_(l) ⇌ BH⁺_(aq) + OH^-_(aq) (weak base B dissociating in water)

  • K_b=[BH⁺][OH^-] / [B] → base dissociation constant

• the higher the value of Kb at a particular temperature, the greater the ionization, so the stronger the base

• calculation rules involving K_a and K_b:

  • given concentration of an acid/base is its initial concentration → before dissociation

  • pH/pOH values refers to concentration of H⁺/OH^- ions at equilibrium

  • concentration values for K_a/K_b must be the equilibrium values for reactants and products

  • when extent of dissociation / K_a and K_b value is very small, [acid]_initial=[acid]_equilibrium (same for base)

pK_a and pK_b

• pK_a = -log₁₀K_a, pK_b = -log₁₀K_b → similar to pH/pOH scale

  • pK_a and pK_b numbers are usually positive and have no units

  • relationship between K_a/pK_a and K_b/pK_b is inverse

    • stronger acid → K_a increases, pK_a decreases; stronger base → K_b increases, pK_b decreases

  • pK_a/pK_b must be quoted at a specified temperature

• K_a x K_b = K_w so pK_a + pK_b = 14.00 at 298K

  • the stronger the acid, the weaker its conjugate base will be

  • the higher the K_a, the lower the K_b for its conjugate

pH of salt solutions

• salt formed in neutralization reaction is an ionic compound → cation is conjugate acid of parent base, anion is conjugate base from parent acid (MOH + HA → M⁺A^- + H₂O)

  • pH of a salt solution depends on whether/to what extent their ions react with water and hydrolyze it

• strong acid + strong base

  • neither ion hydrolyses

  • neutral, pH=7

• weak acid + strong base

  • anion hydrolyses

  • basic, pH>7

• strong acid + weak base

  • cation hydrolyses

  • acidic, pH<7

• weak acid + weak base

  • both ions hydrolyse

  • depends on relative strengths, pH cannot generalize

Acid-base indicators

• indicators are weak acids/bases in which their undissociated and dissociated forms have different colours

  • only weak acids will be considered → HInd (generic acidic indicator)

    • HInd_(aq) ⇌ H⁺_(aq) + Ind^-_(aq) (different colours as HInd and Ind^-)

    • increasing [H⁺] → equilibrium shifts leftward in favour of HInd

• K_a=[H⁺][Ind^-] / [HInd]

  • at the point where the equilibrium is balanced ([Ind^-]=[HInd]), the indicator is exactly in th emiddle of its colour change: K_a=[H⁺][Ind^-] / [HInd] = [H⁺] so pK_a=pH

    • addition of a very small volume of acid/base will shift equilibrium → change colour

  • this point is the end point (pH=pK_a(indicator))

    • different indicators have different pK_a values so will have different end points

• an indicator will be effective in signalling the equivalence point when its end point coincides with the pH at equivalence

  • different indicators must be used for different titrations, depending on the pH at equivalence

  • choosing an appropriate indicator:

    1. determine what combination of strong/weak acids/bases are reacting together

    2. deduce pH of salt solution at equivalence

    3. consult data tables to choose an indicator with end point in range of equivalence point

Buffer solutions

• buffer → something that acts to reduce the impact of one thing on another (shock absorber)

  • buffer acts to reduce the pH impact of added acid/base on a chemical system

  • buffer solution is resistant to changes in pH on the addition of small amounts of acid/alkali

• acidic buffers (maintain pH<7)

  • made by mixing an aqueous solution of a weak acid with a solution of its salt of a strong alkali

  • ex: NaCH₃COO_(aq) → Na⁺_(aq) + CH₃COO^-_(aq) (soluble salt → fully dissociates)

    • mixture contains relatively high concentrations of CH₃COOH and CH₃COO^- (acid and its conjugate base)

      • resovoirs, ready to react with added OH^- and H⁺ in neutralization reactions

    • addition of H⁺ (acid): CH₃COO^-_(aq) + H⁺_(aq) ⇌ CH₃COOH_(aq)

    • addition of OH^- (base): CH₃COOH_(aq) + OH^-_(aq) ⇌ CH₃COO^-_(aq) + H₂O_(l)

• basic buffers (maintain pH>7)

  • made by mixing an aqueous solution of a weak base with its salt of a strong base

  • ex: NH_3(aq) and NH₄Cl_(aq) (weak base and salt of weak base with strong acid)

    • NH_3(aq) + H₂O_(l) ⇌ NH₄⁺_(aq) + OH^-_(aq) (equilibrium lies to the left (weak base))

    • NH₄Cl_(aq) → NH₄⁺_(aq) + Cl^-_(aq) (soluble salt → fully dissociates)

    • resovoirs → NH₄⁺ and NH₃ to be used in neutralization reactions

    • addition of acid (H⁺): NH_3(aq) + H⁺_(aq) ⇌ NH₄⁺_(aq)

    • addition of base (OH^-): NH₄⁺_(aq) + OH^-_(aq) ⇌ NH_3(aq) + H₂O_(l)

• buffer solutions are a mixture containing both an acid and base of a weak conjugate pair

  • buffer’s acid neutralizes added alkali, buffer’s base neutralizes added acid → pH change is resisted

  • has to be weak because strong acids/bases dissociate fully → do not exist in equilibrium, so cannot carry out reversible reactions to respond to both added H⁺ and OH^- ions

Buffer composition and pH

• pH of a buffer solution depends on the interactions among its components

• ex: generic weak acid HA and its salt MA

  • HA_(aq) ⇌ H⁺_(aq) + A^-_(aq), MA_(aq) → M⁺_(aq) + A^-_(aq)

    1. the dissociation of the weak acid is so small it is considered negligible

       • [HA]_initial = [HA]_equilibrium

    2. salt is considered to be fully dissociated into its ions

       • [MA]_initial = [A^-]_equilibrium

  • K_a=[H⁺][A^-] / [HA] so [H⁺] = K_a[HA] / [A^-]

    • [H⁺] = K_a[HA]_initial / [MA]_initial

  • [H⁺] = K_a[acid] / [salt]

• pH = pK_a + log₁₀[salt]/[acid]

• pOH = pK_b + log₁₀[salt]/[base]

  • pH of a buffer solution can be determined from:

    • pK_a/pK_b values of its component acid/base

    • ratio of initial concentrations of acid/base and salt used to prepare the buffer

  • when [acid] = [salt], pH=pK_a / pOH=pK_b

Factors that can influence buffers

• dilution

  • dilution does not change K_a/K_b or ratio of acid/base to salt concentration → does not change pH

  • buffering capacity → amount of acid/base absorbed without significant changes in pH

    • decreases when concentrations are decreased with dilution

• temperature

  • affects K_a and K_b so affects pH of the buffer

3.2: Electron transfer reactions

Redox reactions

• oxidation is the loss of electrons

• reduction is the gain of electrons

• oxidation is hydrogen loss and reduction is hydrogen gain

• oxidation is an increase in oxidation state and reduction is a decrease in oxidation state

  • oxidation state for molecules with more than one atom is an average value → can be a non-integer

• oxidizing agent → reactant that accepts electrons (brings about the oxidation of the other reactant)

• reducing agent → reactant that supplies electrons (brings about reduction and is oxidized)

Redox titrations

• similar to acid-base titrations → redox reaction between two reactants to find equivalence point and thus titre

• ex: Iodine-thiosulfate solution

  • several different reactions use an oxidizing agent to convert excess iodine ions into idoine:

    • 2I^-_(aq) + oxidizing agent → I_2(aq) + other products

    • liberated I2 then reacts with sodium thiosulfate (Na₂S₂O₃)

  • oxidation: 2S₂O₃^2-_(aq) → S₄O₆^2-_(aq) + 2e^-

  • reduction: I_2(aq) + 2e^- → 2I^-_(aq)

    • 2S₂O₃^2-_(aq) + I_2(aq) → 2I^-_(aq) + S₄O₆^2-_(aq)

  • starch can be used as an indicator → deep blue in presence of I₂

Strength of oxidizing/reducing agents

• strength depends on relative tendencies to lose/gain electrons

• halogens react by gaining electrons and becoming negative ions → act as oxidizing agents

  • tendency to gain electrons decreases down the group (F₂>I₂ in reactivity)

  • the more reactive it is, the stronger it is as an oxidizing agent

  • ex: Cl_2(aq) + 2KI_(aq) → 2KCl_(aq) + I_2(aq)

    • K⁺ does not change, it is a spectator ion → can be disregarded

    • Cl is more reactive so “wins” over K because it is a stronger oxidizing agent (displaces K)

• metals have a tendency to lose electrons and form positive ions → act as reducing agents

  • more reactive metals are stronger reducing agents

  • reactivity of group 1 metals increase down the group (K>Na as a reducing agent)

    • can be confirmed through displacement reactions

      • if the metal is displaced by another, it is a weaker reducing agent

Oxidation of metals by acids

• acids react with metals to form salts and release hydrogen

  • ex: 2HCl_(aq) + Zn_(s) → ZnCl_2(aq) + H_2(g)

  • removing spectator ions: 2H⁺_(aq) + Zn_(s) → Zn²⁺_(aq) + H_2(g)

    • zinc is oxidized (0→+2) → acts as a reducing agent

    • zinc has the strength to “force” the hydrogen to accept the electrons as it is a strong reducing agent

  • no reaction occurs when copper is added to a dilute acid

    • copper lacks the strength to displace hydrogen

• hydrogen is less reactive than zinc but more reactive than copper

  • this is why acids are so corrosive (except to low reactivity metals like Ag and Au)

Comparing voltaic and electrolytic cells

• voltaic and electrolytic cells are known as electrochemical cells

• voltaic cells convert chemical energy to electrical energy

  • a spontaneous chemical reaction drives electrons around a circuit

• electrolytic cells convert electrical energy to chemical energy

  • an electric current reverses the normal directions of chemical change → non-spontaneous reactions occur

• oxidation occurs at the anode and reduction at the cathode for both cells

  • anode is the negative terminal in a voltaic cell but the positive terminal is an electrolytic cell

Primary voltaic cells

• if the half-reactions in a redox reaction occur at spatially seperated electrodes, the electrons must pass through an external circuit

  • electric current is produced → voltaic/galvanic cell

    • chemicals are not renewed during the process → primary cell

  • battery normally consists of several cells connected together

• ex: Zn_(s) → Zn²⁺_(aq) + 2e^-, Cu²⁺_(aq) + 2e^- → Cu_(s)

  • half-cell made by putting a strip of metal into a solution of its ions

  • in the zinc half-cell, zinc atoms form ions by releasing electrons

    • makes surface of the metal negatively charged with respect to the solution

  • negative charge on electrode attracts zinc ions which can gain electrons in the reverse process (Zn_(s) ← Zn²⁺_(aq) + 2e^-)

  • at a particular charge seperation, the rates of both processes will equal and equilibrium will be reached

    • Zn_(s) ⇌ Zn²⁺_(aq) + 2e^-

  • position of equilibrium depends on reactivity of metal (the more reactive, the more formation of ions → greater negative charge, more electrons)

  • when the two half-cells are connected, electrons will have a tendency to flow spontaneously to the less negative half-cell

    • tendency of electrons to flow is quantified by measuring the potential difference between 2 electrodes

  • anode → where oxidation occurs (zinc), cathode → where reduction occurs (copper)

• cell diagram convention

  • phase boundary → single vertical line between a solid electrode and an aqueous solution in a half-cell

  • salt bridge → double vertical line

  • aqueous solutions of each electrode are placed next to the salt bridge

  • anode on left, cathode on right (electrons flow from left to right)

Half-cells and voltage

• any two half-cells can be connected to make a voltaic cell

  • direction of electron flow and voltage produced will be determined by the difference in reducing strength

    • most cases can be judged by the relative position of the metals in the activity series

  • larger voltage will be produced when there is a greater difference in electrode potentials

  • electrons flow from more to less reactive metal (ex: Zn→Cu, Zn→Ag)

    • anode (where electrons are produced) to cathode (where electrons are accepted)

Secondary (rechargeable) cells

• involves reversible redox reactions using electrical energy

• lithium-ion battery → benefits from lithium’s low density and high reactivity

  • can store a lot of electrical energy per unit mass

  • as lithium is reactive, steps must be taken to prevent it from forming an oxide layer (decreases contact with electrolyte)

    • lithium cathode placed in lattice of a metal oxide (MnO2), lithium anode mixed with graphite

  • non-aqueous polymer-based electrolyte is used

  • discharging:

    • lithium is oxidized at the negative electrode: Li_(polymer) → Li⁺_(polymer) + e^-

    • Li⁺ ion and MnO₂ are reduced at the positive electrode: Li⁺_(polymer) + MnO_2(s) + e^- → LiMnO_2(s)

    • the two half reactions are reversed when the battery is recharged

• fuel cells → combustion reactions are redox reactions, can be used to produce an electric current if reactants are physically seperated

• hydrogen fuel cell: H_2(g) + ½O_2(g) → H₂O_(l) ΔH_f = -285.8kJ/mol

  • Gibbs energy gives maximum energy available for electrical output

    • H_2(g) + ½O_2(g) → H₂O_(l) ΔG = -228.6kJ/mol

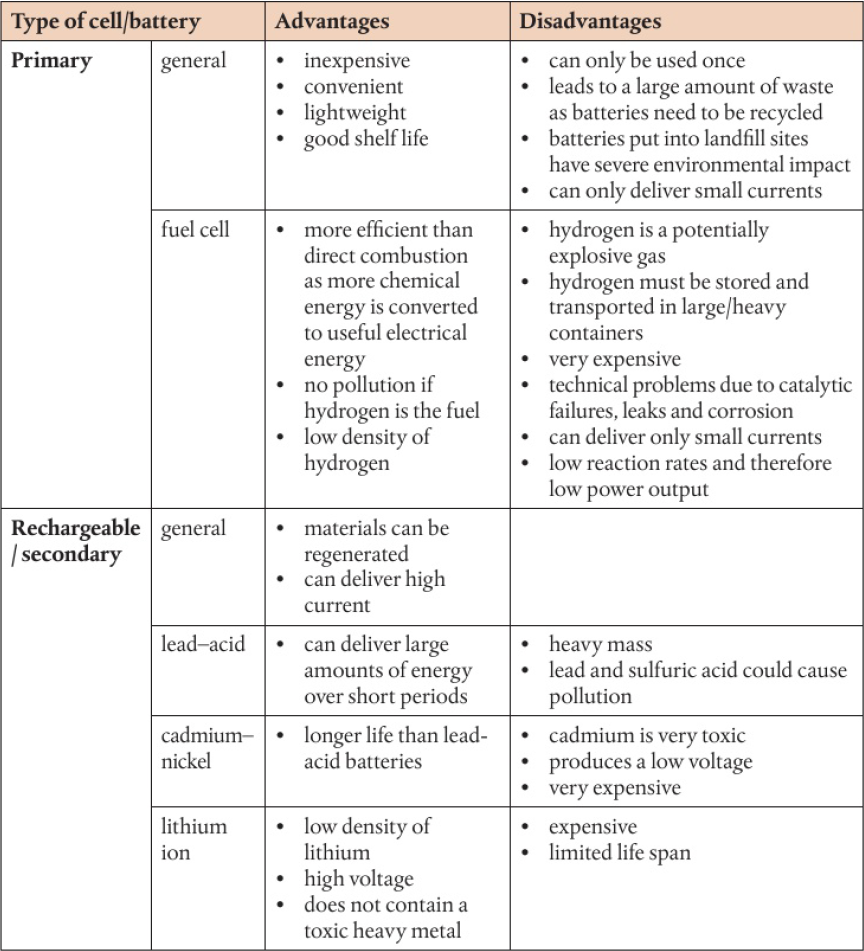
Advantages and disadvantages of fuel cells, primary cells, and secondary cells

Electrolytic cells

• voltaic cell takes the energy of a spontaneous redox reaction and harnesses it to generate electrical energy

  • electrolytic cell reverses this process using an external source of electrical energy to bring about a non-spontaneous redox reaction that would otherwise not take place

  • electrolysis → process where electricity is used to bring about chemical reactions which break down substances

• reactant must contain mobile ions which allow the currrent to pass through the electrodes

  • electrolyte → liquid, usually a molten ionic compound or an aqueous solution of an ionic compound

  • as an electric current passes through the electrolyte, redox reactions occur at the electrodes, removing the charges on the ions and forming products that are electrically neutral

    • ions are discharged during this process

• 

  • source of electric energy is a battery/DC power source

  • electrodes are placed in the electrolyte and connected to the power supply

    • electrodes are made from a conducting substance (metal/graphite)

    • electrodes are inert → do not take part in the redox reactions

    • electrodes must not touch each other → “shorts” the circuit, current would bypass the electrolytic cell

  • electric wires connect electrodes to power supply

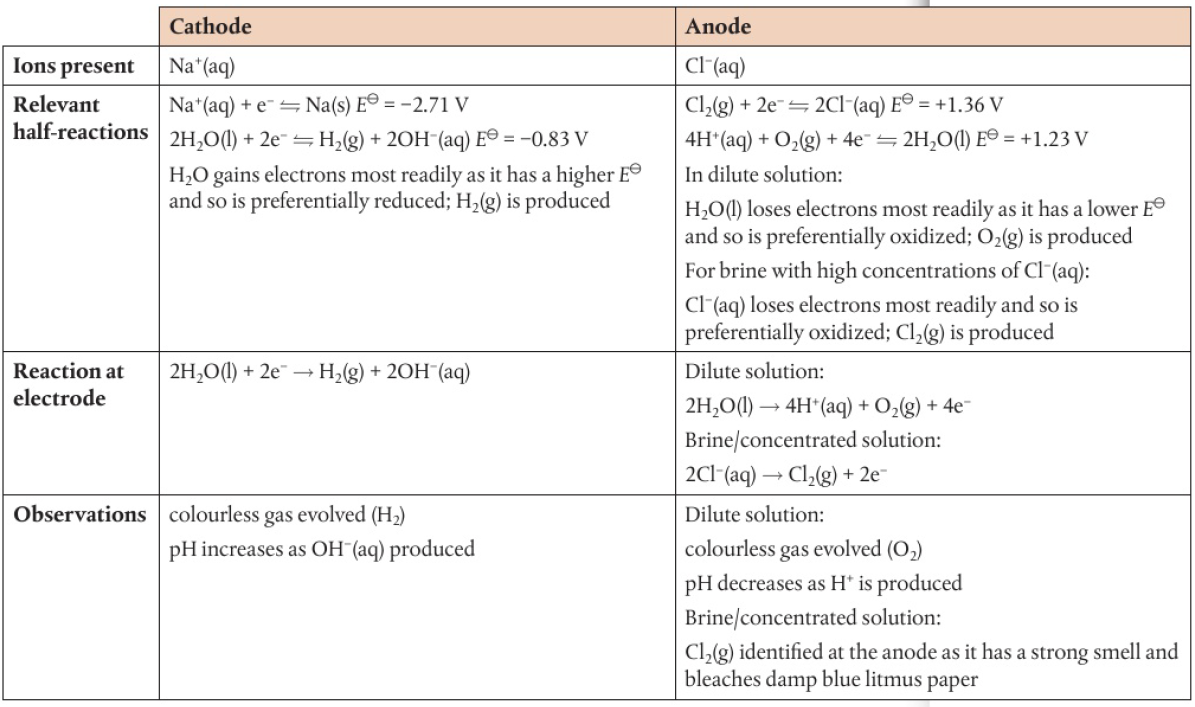
• ex: spontaneous reaction Na_(s) + ½Cl_2(g) → NaCl_(s)

  • electrons are transferred from sodium to chlorine

  • process can be reversed if solid sodium chloride is first converted into the molten state: NaCl_(s) → Na⁺_(l) + Cl^-_(l)

  • ions are now mobile, ions can act as an electrolyte in an electrochemical cell

  • battery pushes electrons towards the negative electrode (cathode) → accepted by Na⁺ ions as they are reduced in the electrolyte

    • Na⁺_(l) + e^- → Na_(l)

  • electrons are released at the positive terminal (anode) → chloride ions are oxidized

    • 2Cl^-_(l) → Cl_2(g) + 2e^-

  • chemical reactions occuring at each electrode remove ions from electrolyte

    • compound has been split into its constituent ions → electrolysis has occured

Oxidation of functional groups in organic compounds

• oxidation of alcohols

  • commonly the laboratory process uses acidified potassium dichromate (VI) → bright orange solution, reduced to green Cr³⁺_(aq) as alcohol is oxidized on heating

  • when writing equations for these reactions, it is convenient to show the oxidizing agent simply as “+[O]”

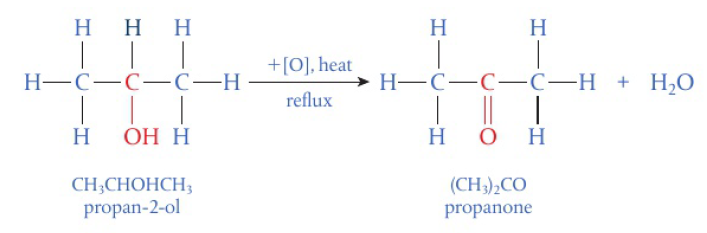
  • primary alcohols → 2 H atoms attached to the carbon with the hydroxyl group, can be oxidized in a two-step reaction

    • removal of the first H atom leads to the formation of the aldehyde

    • the second H atom is removed under prolonged reaction, carboxylic acid is formed

    • 

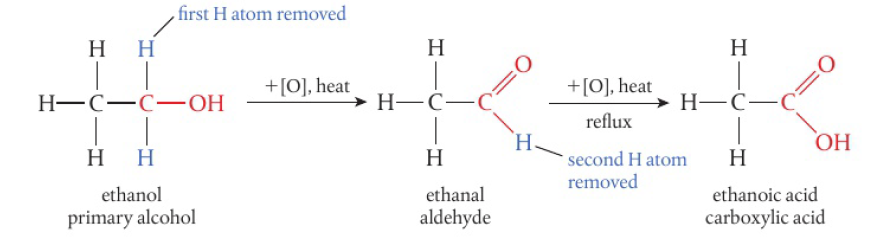
  • secondary alcohols → only 1 H attached to the carbon bonded to the hydroxyl group → one-step reaction

    • 

  • tertiary alcohols have no H atoms attached to the carbon bonded to the hydroxyl group → not readily oxidized without breaking the carbon chain

    • carbon skeleton of the molecule is broken in combustion

    • 

  • 

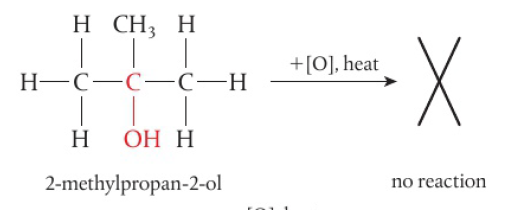
Reduction of functional groups in organic compounds

• reduction of carbonyl compounds

  • redox reactions are more conveniently thought of as gain and loss of oxygen/hydrogen instead of electrons

  • oxidation of secondary alcohols to ketones involved the removal of 2 H atoms

    • this process can be reversed: ketones can be reduced to secondary alcohols by adding two H atoms

  • carbonyl group is polar with a partial positive charge on the carbon atom → can be attacked by species with a lone pair of electrons

    • hydride ion (H^-) acts as a nucleophile on the electron-deficient carbonyl carbon

  • carboxylic acids can be reduced to aldehydes

    • reactions can be shown using [+H] like [+O] for oxidation

    • 

    • conditions: heat with LiAlH₄ in dry ether, reaction cannotbe stopped at the aldehyde → reacts too readily with LiAlH₄

• 

Reduction of unsaturated compounds

• alkenes and alkynes are unsaturated hydrocarbons

  • they can undergo addition reactions so form a range of different saturated products

  • alkenes generally react by electrophilic addition

• alkenes can be reduced to alkanes by reacting with hydrogen

  • C_nH_2n + H₂ → C_nH_2n+2

  • alkynes are similarly reduces to alkenes and alkanes through addition of hydrogen

The standard hydrogen electrode

• voltaic cell generates a potential difference → electromotive force (emf)

  • electrons tend to flow from more negative half-cell to more positive half-cell → potential generated is cell/electrode potential (E)

  • measured using a voltmeter: depends on difference in the tendencies of these two half-cells to undergo reduction

  • it is convenient to compare relative reducing power of different half-cells with the same fixed reference point

• standard hydrogen electrode (SHE) → baseline for measuring and comparing electrode potentials of other half-cells

  • 

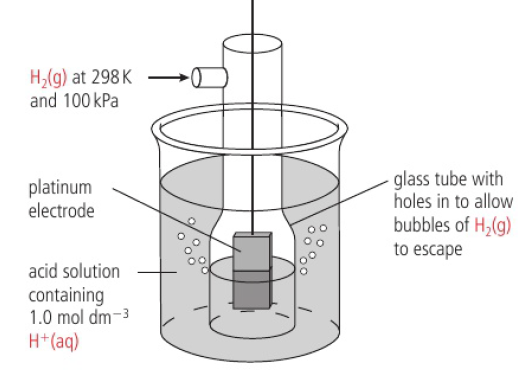
  • platinum is used as conducting metal → inert and will not ionize, catalyzes the reduction of H⁺_(aq) ions

    • surface of metal is coated with very finely divided platinum (platinum black, “platinized platinum”) which increases the large surface area → helps in adsorption of hydrogen gas

    • increases rate of reaction of both forward and backward reactions

  • concentration of H⁺_(aq) is 1mol/dm³ (pH=0), pressure of H_2(g) is 100kPa at 298K

  • as the electrode is immersed in the acidic solution, an equilibrium is set up between the adsorbed layer of H_2(g) and H⁺_(aq) ions

    • 2H⁺_(aq) + 2e^- ⇌ H_2(g)

    • H⁺_(aq) ions are reduced in the forward reactions, H_2(g) molecules are oxidized in the backward reaction

    • position of equilibrium depends on electrode potential of the other half-cell to which the SHE is connected to in a circuit

  • hydrogen half-cell is assigned an electrode potential of zero volts (0V)

Measuring standard electrode potentials

• standard conditions (standard half-cells):

  • all solutions must have a concentration of 1.0 mol/dm³

  • all gases must be at pressure 100kPa

  • all substances used must be pure

  • temperature is 298K

  • if the half-cell does not include a solid metal, platinum is used as the electrode

• when the SHE is connected to another standard half-cell by an external circuit, the emf generated is the standard electrode potential (E^Ꝋ)

• ex: Cu²⁺_(aq) / Cu_(s) half-cell: +0.34V

  • 

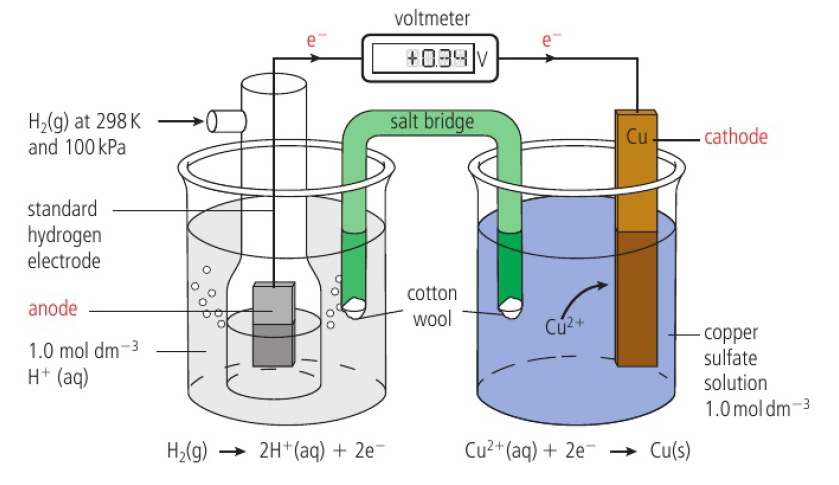
  • positive value for E shows that electrons tend to flow from hydrogen half-cell to copper half-cell → electrons are produced by oxidation of H_2(g) and used to reduce Cu²⁺_(aq) ions

  • hydrogen half-cell is the anode, copper half-cell is the cathode

  • positive cell potential → Cu²⁺ ions have a higher tendency to be reduced than H⁺ ions → low reactivity of copper

• standard electron potential is always given for the reduction reaction

  • standard electrode potential values can be known as standard reduction potentials

  • 

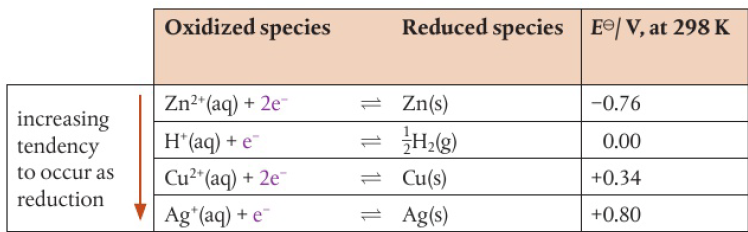
  • E^Ꝋ values do not depend on total number of electrons (is not scaled up/down with stoichometry of equation for a reaction)

  • the more positive the E^Ꝋ value for a half-cell, the more readily it is reduced

  • electrons always flow through the external circuit in a voltaic cell from the half-cell with the more negative standard electrode potential to the half-cell with the more positive standard electrode potential

• E^Ꝋ_cell = E^Ꝋ_{half-cell where reduction occurs} - E^Ꝋ_{half-cell where oxidation occurs}

  • E^Ꝋ values must be the reduction potentials as supplied in data tables

• the voltaic cell will always run in the direction that gives a positive value for the E^Ꝋ_cell

  • if E^Ꝋ_cell is positive, the reaction is spontaneous

  • if E^Ꝋ_cell is negative, the reaction is non-spontaneous and reverse reaction is spontaneous

• metals with the most negative E^Ꝋ values are the strongest reducing agents

• non-metals with the most positive E^Ꝋ values are the strongest oxidizing agents

  • 

Electrode potentials and Gibbs energy changes

• ΔG^Ꝋ = -nFE^Ꝋ_cell

  • n = number of moles of electrons transferred in the reaction

  • F = the charge carried by 1 mol of electrons (Faraday constant, 96500C/mol)

• reaction is spontaneous when:

  • E^Ꝋ_cell is positive

  • ΔG^Ꝋ is negative

• the more positive the value of E^Ꝋ_cell, the more energetically favourable the reaction

  • voltmeter can be considered an indirect measure of Gibbs energy change and electrode potential

• electrons always tend to flow towards the half-cell with the highest E^Ꝋ value

Electrolysis of aqueous solutions

• predicting the products at the electrodes in electrolysis of aqueous solutions is more difficult because the water present can also be oxidized/reduced

• ex: electrolysis of sodium chloride

  • cathode: sodium cannot be produced as it would react immediately with water to produce hydrogen

    • Na⁺ + e^- → Na

    • Na + H₂O → Na⁺ + OH^- + ½H₂

    • water is preferentially reduced to hydrogen

  • anode: water can be oxidized to oxygen

    • 2H₂O_(l) → 4H⁺_(aq) + O_2(g) + 4e^-

• when a solute M⁺A^- is in an aqueous solution, there is more than one redox reaction possible at each electrode

  • anode: either A^- or H₂O can be oxidized

  • cathode: either M⁺ or H₂O can be reduced

• discharge of an ion at the electrode is known as selective discharge

  • in the example, water is reduced in preference to sodium because sodium is highly reactive → negative electrode potential

• outcome can be determined generally:

  • relative E^Ꝋ values of ions compared to water

  • relative concentrations of ions in the electrolyte

  • nature of the electrodes

1. identify all ions present in electrolyte, determine which will migrate to which electrode

2. write the half-equation for the possible reactions at each electrode

3. the half-reaction with the higher electrode potential is the oxidation reaction at the cathode (higher electrode potential = gains electrons most readily)

4. the half-reaction with the lower electrode potential is the reduction reaction at the anode (lower electrode potential = loses electrons most readily)

5. to deduce overall reaction, balance the electrons lost and gained at the anode and cathode then add the two half-equations

6. consider what changes would be observed in the cell as a result of the redox reactions (colour changes, precipitation of solid, gas discharge, pH changes)

Electrolysis examples

• electrolysis of water

  • wateris not a good conductor of electricity, so some sulfuric acid or sodium hydroxide is usually added

  • 

• electrolysis of NaCl_(aq) (brine)

  • 

• electrolysis of CuSO_4(aq) with carbon_(graphite) or other ‘inert’ electrodes

  • 

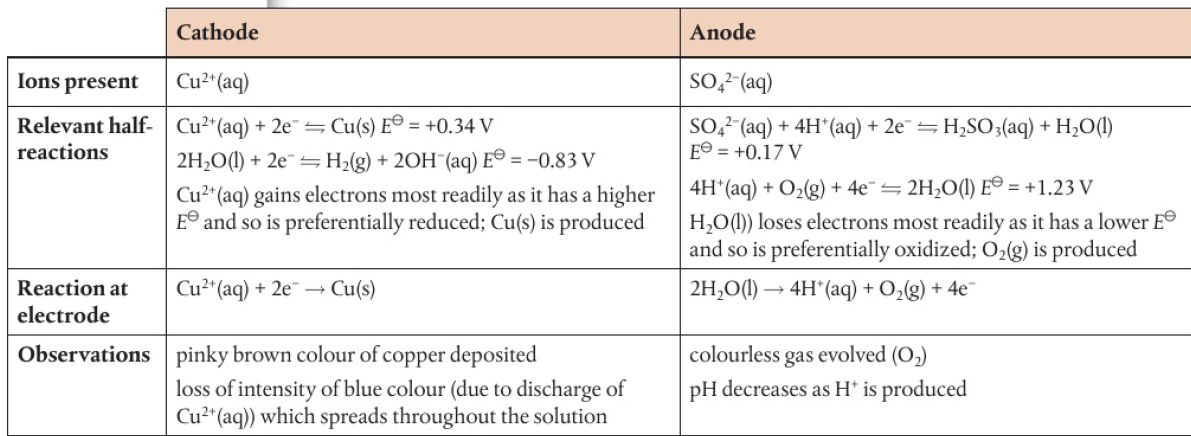
  • electrolysis of CuSO_4(aq) with copper electrodes

    • 

Electroplating

• process of using electrolysis to deposit a layer of metal on top of another metal or other conductive object

• electrolytic cell used for electroplating has following features:

  • object to be plated forms the cathode

  • electrolyte contains the metal ions which are to coat the object

  • anode is sometimes made of the same plating metal → as it is oxidized, it replenishes the ions in the electrolyte which are discharged at the cathode

• reduction of metal ions at the cathode leads to deposition on its surface

  • process can be controlled by altering current and time accordingly to thickness of metal layer desired

• purposes of electroplating:

  • decorative

  • corrosion control: metal covering the surface is oxidized preferentially to metal underneath

  • improvement of function

• 

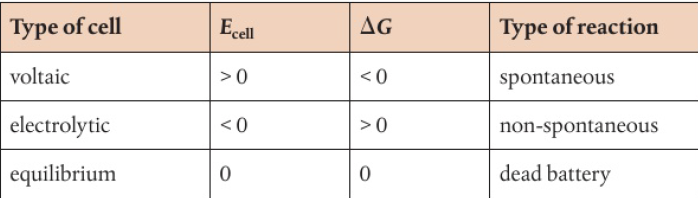
3.3: Electron sharing reactions

Radicals

• radical → chemical species that contains an unpaired electron

  • presence of unpaired electron results in high enthalpy

  • radicals are very reactive → energetically favourable for them to take an electron from other species or combine with other radicals to form a covalent bond (both results in products with lower enthalpy)

• radicals have short lifetimes (reactivity) and do not exist for very long

  • exception: radicals formed in the upper atmosphere, can persist for a significant length of time (low probability of collision)

• radicals can be atoms, molecules, or ions

Homolytic fission

• homolytic fission → covalent bond breaks to form two radicals

  • breaking bonds is endothermic → homolytic fission requires energy

    • amount of energy depends on strength of covalent bond

  • weaker bonds, sufficient energy by heating compound → thermolytic fission

  • stronger bonds, absorption of UV light → photolytic fission

• termination reactions → two radicals combine, sharing unpaired electrons and forming a covalent bond (reverse process of homolytic fission)

• chlorofluorocarbons (CFCs) → when in the stratosphere, the UV radiation breaks them down, releasing chlorine atoms (reactive radicals)

  • chlorine radicals released then catalyse the decomposition of ozone

  • Cl_(g) + O_3(g) → O_2(g) + ClO_(g)

  • ClO_(g) + O_(g) → O_2(g) + Cl_(g)

Radical substitution reactions of alkanes

• alkanes are relatively unreactive compounds → strong C-C and C-H bonds, non-polar, high activation energies

  • at regular temperatures, reactant molecules lack sufficient KE to overcome activation energy, so alkanes are kinetically stable

  • alkane reactants have a higher enthalpy (exothermic) and are thermodynamically unstable compared to the products

• alkanes are saturated, so undergo substitution reactions (another reactant takes the place of a hydrogen atom in the alkane)

  • products between alkane and halogen: halogenoalkane and hydrogen halide

  • once formed, radicals will start a chain reaction → mixture of products including halogenoalkane is formed

• initiation

  • formation of radical species, which can then react with the alkane

• propagation

  • radicals formed in initiation stage react with other species present to form new radicals

  • there are many possible propagation steps, all of which allow the reaction to continue (chain reaction)

• termination

  • radicals are removed when they reach with each other and pair up electrons to form a covalent bond (many possible termination steps)

3.4: Electron-pair sharing reactions

Nucleophiles

• nucleophile → reactant that forms a covalent bond to its reaction partner (the electrophile) by donating both bonding electrons

  • tend to be electron rich, having 1+ lone pairs of electrons and may also carry a negative charge

  • ex: H₂O, NH₃, alcohols, amines, OH^-, F^-, Cl^-

• forms a coordination bond

  • 

Nucleophilic substitution reactions

• halogenoalkanes are polar compounds → halogen atom is more electronegative than carbon, exerts a stronger pull on shared electrons

  • halogen gains a partial negative charge and carbon gains a partial positive charge and is said to be electron deficient

• nucleophiles are attracted to electron-deficient species

  • leads to reactions which substitution of the halogen occurs → nucleophilic substitution

    • CH₃Cl + OH^- → CH₃OH + Cl^-

• overall reaction between a nucleophile and an electron-deficient substrate molecule (like a halogenoalkane):

  • for the nucleophile to donate an electron pair to the substrate molecule and form a new bond, another bond must break

    • leaving group → species that breaks away from the substrate molecule

    • halogens generally make good leaving groups as they form relatively weak bonds with carbon

    • halogens have a higher electronegativity meaning it draws the bonded electrons towards itself, making the carbon atom electron deficient → susceptible to nucleophilic attack

  • the bond that forms and the bond that breaks must both involve the carbon atom that is bonded to the leaving group

• if nucleophile is a neutral species (ex: water), the initial product formed will be positively charged

  • subsequently deprotonates and loses an H+ to give a neutral product

  • if the leaving group was a halide ion, the H+ and halide ion combine to form a hydrogen halide

  • overall equation: H2O + RX → ROH + HX

Heterolytic fission

• bond-breaking in nucleophilic substitution reactions involves heterolytic fission → a covalent bond breaks such that one of the products gains both of the shared electrons

  • formation of oppositely charged ions (the more electronegative atom will gain the negative charge)

  • ex: Cl-Cl → Cl+ + Cl-

  • ex: H-Cl → H+ + Cl-

• bond breakage occurs through movement of an electron pair (use double-headed curly arrows instead)

• opposite process: nucleophile donates a pair of electrons to an electrophile, forming a coordination bond

Electrophiles

• electrophile → reactant that forms a covalent bond to its reaction partner (the nucleophile) by accepting both electrons

  • tend to be electron deficient or contain an electron-deficient region

  • often have a positive charge / partial positive charge

  • ex: hydrogen halides, halogens, halogenoalkanes, H+, NO2+, CH3+, carbocations

Electrophilic addition of alkenes

• alkenes are unsaturated hydrocarbons

  • more reactive than alkanes: double bond has a high electron density which makes it attractive to electrophiles

  • reaction occurs at site of double bond (pi bond is selectively broken) which creates two new bonding positions on the carbon atoms

    • enables addition reactions with electrophiles (range of different saturated products)

• addition of water (hydration)

  • converts alkene into an alcohol

  • water is a poor electrophile, so requires a strong acid catalyst (ex: concentrated sulfuric acid)

  • ex: CH2CH2 →(H2O, H2SO4) CH3CH2OH

• addition of halogens

  • halogens react with alkenes to produce dihalogeno compounds

    • reactions happen quickly at room temperature, accompanied by loss of color of reacting halogen

• addition of halogen halides

  • halogen halides (ex: HCl, HBr) react with alkenes to produce halogenoalkanes

    • reactions take place rapidly in solution at room temperature

  • reactivity is in order of the decreasing strength of the hydrogen halide bond down group 17

    • HI (weakest bond) reacts most readily

Lewis acids and Lewis bases

• a Lewis acid is a lone pair acceptor

  • all Bronsted-Lowry acids are Lewis acids, but Lewis acids also include any species capable of accepting a lone pair of electrons (molecules with an incomplete valence shell)

• a Lewis base is a lone pair donor

  • Lewis bases and Bronsted-Lowry bases are the same group of compounds: must have a lone pair of electrons

• Lewis acid-base reactions result in the formation of a covalent bond (always coordination bond, both electrons come from the base)

• once a coordination bond is formed, it is no different from a regular covalent bond

  • same properties (bond length, bond strength, chemical reactivity)

• Lewis acids are electrophiles, Lewis bases are nucleophiles

  • nucleophile (‘likes nucleus’) is an electron-rich species that donates a lone pair to another reactant to form a new covalent bond

  • an electrophile (‘likes electrons’) is an electron-deficient species that accepts a lone pair from another reactant to form a new covalent bond

Complex ions

• transition metals can act as Lewis acids and accept lone pairs of electrons when they bond with ligands to form complex ions

  • ligand donates both electrons, coordination bond is formed

    • ligands are therefore Lewis bases

• ligands can be neutral molecules/anions but must always contain at least one lone pair of electrons

  • ex: H₂O, NH₃, CO, I^-, Br^-, F^-, OH^-

• transition metals typically bond to more than one ligand when they form complex ions

  • number of coordination bonds between ligands and central ion in the complex ion → coordination number

• 

• charge on complex ion is dependent on:

  • charge on central transition metal ion

  • charge on ligands

  • coordination number

Nucleophilic substitution mechanisms

• shorthand notation: S_N (substitution nucleophilic) is used to describe this type of reaction

  • polar carbon-halogen bond in halogenoalkanes means the carbon atom is electron deficient, thus attacked by nucleophiles (like OH^-)

    • during these reactions, the carbon-halogen bond breaks and the halogen atom is released as a negative ion (halide)

• 

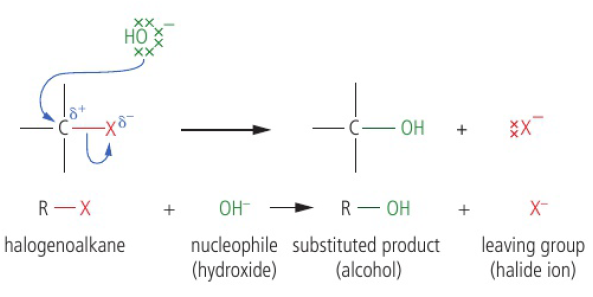
  • exact mechanism of reaction depends on whether the halogenoalkane is primary, secondary, or tertiary

• primary halogenoalkanes react via S_N2

  • primary halogenoalkanes have at least 2 hydrogen atoms attached to the carbon of the carbon-halogen bond

    • ex: chloroethane (C₂H₅Cl)

    • overall reaction with NaOH:

      • C₂H₅Cl + OH^- → C₂H₅OH + Cl^-

    • as hydrogen atoms are small, the carbon atom is relatively open to attack by nucleophile

    • unstable transition state is formed in which carbon is weakly bonded to both halogen and nucleophile

      • carbon-halogen bond then breaks heterolytically (electrons go to the same species)

  • 

  • effectively a one-step concerted reaction with an unstable transition state

  • mechanism is dependent on concentration of both the halogenoalkane and the hydroxide ion, so it is a bimolecular reaction (second order)

    • therefore the mechanism is fully described by S_N2: substitution nucleophilic 2^nd order

• nucleophile attacks the electrophilic carbon on the opposite side from the leaving group, causing an inversion of the arrangement of atoms around the carbon atom

  • 

  • configurations are a result of different spatial arrangements around tetrahedrally bonded carbon atoms

  • S_N2 mechanism → stereospecific, three-dimensional arrangement of reactants determines three-dimensional configuration of products

  • bond formation comes before bond cleavage (breaking) in transition state, so stereochemistry of the carbon attacked is not lost

  • optically active reactants will react via S_N2 to give optically active products (stereospecific)

• tertiary halogenoalkanes react with S_N1

  • tertiary halogenoalkanes have 3 alkyl groups attached to the carbon of the carbon-halogen bond

    • ex: 2-chloro-2-methylpropane

      • overall reaction: (CH₃)₃CCl + OH^- → (CH₃)₃COH + Cl^-

    • presence of 3 alkyl groups causes steric hindrance → bulky groups make it difficult for an incoming group to attack the carbon atom

    • S_N2: nucleophile attacks electron-deficient carbon, S_N1: heterolytic fission of the carbon-halogen bond and halide ion leaving

      • creates a carbocation intermediate → positive charge centered on carbon

      • intermediate is then attacked by the nucleophile in the second step of the reaction, leading to a bond being formed

    • 

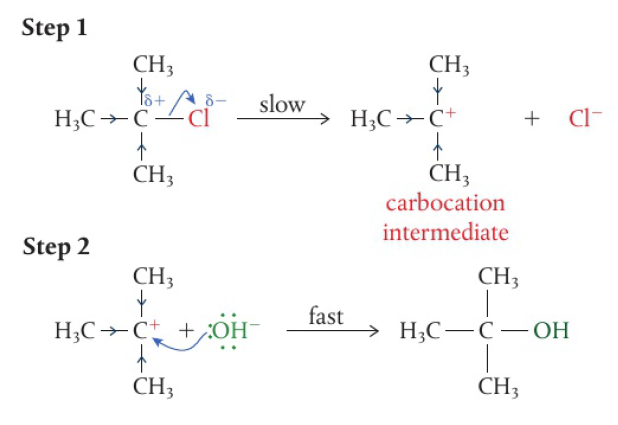
  • slow (rate-determining) step is determined by concentration of only the halogenoalkane → unimolecular reaction, first order

    • S_N1: substitution nucleophilic 1^st order

  • carbocation intermediate has a planar shape → nucleophile can attack from any position

    • S_N1 is not stereospecific, optically active reactants react via S_N1 to form racemic mixtures (50:50 mixture of 2 enantiomers)

  • mechanism is favoured by tertiary halogenoalkanes because carbocation is stabilized by presence of 3 alkyl groups → each alkyl group has an electron-donating/positive inductive effect

    • stabilizing effect helps carbocation to persist long enough for second step to occur

• secondary halogenoalkanes

  • it is not possible to be precise about the mechanism of nucleophilic substitution in secondary halogenoalkanes

  • usually undergo a mixture of both S_N1 and S_N2 mechanisms

  • influence of leaving group (halogen)

    • rate of nucleophilic reactions for halogenoalkanes (with same carbon chain length and branching) is: iodo>bromo>chloro>fluoro

      • the weaker the bond, the easier it is to break and the better the halide is as a leaving group

• S_N1 and S_N2 mechanisms have different rate expressions + reaction profiles

  • 

Electrophilic addition reaction mechanism

• electrophilic addition reactions of alkenes require the breaking of a pi bond

  • carbon atoms of carbon-carbon double bond are sp2 hybridized, forming a planar triangular shape with bond angle 120

    • fairly open structure, relatively easy for incoming groups to attack

  • pi bond represents an area of electron density above and below the plane of bond axis

    • pi bond is attractive to electrophiles (area of electron density)

    • electrons in pi bond are less closely associated with the nuclei so it is a weaker bond than a sigma bond, so breaks more easily

    • when pi bond breaks, reactants attach at each carbon atom through formation of 2 sigma bonds

  • reactions between reagants and alkenes are therefore known as electrophilic addition reactions

• addition reactions of alkenes involve a two-step mechanism

  • ex: ethene + bromine → 1,2-dibromoethane

    • 

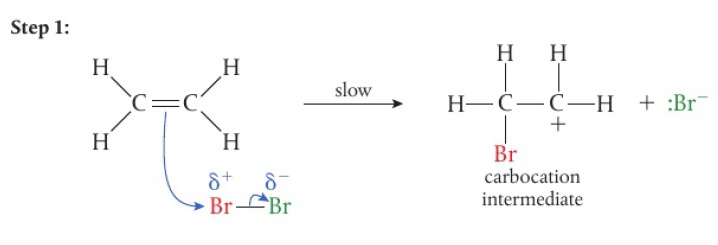
    • bromine is neutral, but becomes polarized due to electron repulsion as it approaches the electron-rich region of the alkene and acts as an electrophile with an electron-deficient bromine atom

    • electrons in pi bond of alkene are attracted to electrophilic bromine so causes Br-Br bond to break heterolytically

    • 

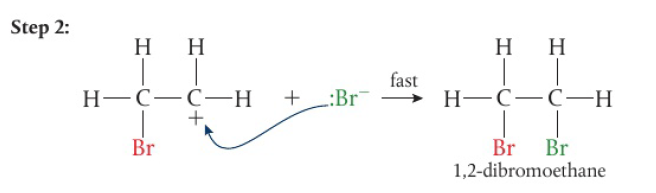
    • this step is slow, resulting in an unstable carbocation intermediate

    • unstable carbocation reacts rapidly with negative bromine ion (Br-), acting as a nucleophile and attacking the positively charged carbon in the carbocation, forming a covalent bond

  • ex: water

    • water is a weak electrophile so does not directly undergo addition reactions with alkenes, but with strong acids (catalysts), H₃O⁺ can act as the electrophile

    • 

    • 

Addition of hydrogen halides to unsymmetrical alkenes

• addition reaction of an unsymmetrical alkene has two potential products but only one is formed exclusively

  • ex: propene + hydrogen bromide

    • 

    • two different carbocation intermediates, one primary (a) and the other secondary (b)

    • alkyl groups around a carbocation stabilize it somewhat due to their positive inductive effects (push electron density away from themselves so lessen the density of the positive charge)

    • (a) only stabilized by one, whereas (b) is stabilized by two, so (b) has greater stabilization

    • more stable carbocation will be more likely to persist, so 2-bromopropane is the main product of the reaction

• Markovnikov’s rule: the hydrogen atom will attach to the carbon that is already bonded to the greater number of hydrogens

  • the more electropositive part of the reacting species bonds to the least highly substituted carbon atom in the alkene (one with smaller number of carbons attached)

Electrophilic substitution of benzene

• benzene molecules contain 3 pi bonds and pi electrons are delocalized due to resonance → minimizes repulsion between pi electrons so benzene molecule is stabilized compared to regular alkenes

  • despite high unsaturation, benzene does not behave like alkenes in its characteristic reactions

  • unusual and highly stable aromatic ring determines that substitution, not addition, is its favoured reaction

• benzene is attractive to electrophiles because its ring is a region of high electron density

  • delocalized cloud of pi electrons attracts electron-deficient species and can form a new bond as a hydrogen atom is lost → electrophilic substitution

  • reaction has high activation energy, proceeds rather slowly (first step)

    • electron pair from benzene is attracted to electrophile leading to a distruption of the delocalized pi system

    • unstable carbocation intermediate that forms has both entering atom/group and leaving hydrogen temporarily bonded to the ring

  • 

  • incomplete circle inside the ring → delocalized pi system disrupted (positive charge distributed over carbon atoms in the ring)

  • heterolytic breaking of C-H bond results in the two electrons moving to regenerate the aromatic ring and thus formation of a neutral substitution product

    • product is more stable

• nitration of benzene

  • substitution of -H by -NO₂ to form nitrobenzene

  • electrophile: NO₂⁺ (nitronium ion), generated using a nitrating mixture (mixture of concentrated nitric acid and concentrated sulfuric acid at 50C)

    • sulfuric acid (stronger acid) protonates nitric acid (nitric acid acts as a Bronsted-Lowry base) and forms (with loss of water) the electrophile

  • NO₂⁺ is a strong electrophile → attracts pi electrons of benzene ring to form carbocation intermediate

    • loss of a proton from intermediate leads to reformation of arene ring in the product nitrobenzene