10. MR Physics & Imaging

Introduction

• MRI is non-ionizing
• MRI can have both anatomical and functional imaging
• MRI uses precession imaging
• NMR is nuclear magnetic resonance, but is not related to radioactive decay (the nucleus is spinning, but there’s no radioactivity)
• MRI offers images of the same object in different contrasts to bring attention to different areas

Physics

• Protons and neutrons are spinning inside the atom, which act like magnets
• Spinning nuclei in an atom ==must have an odd atomic number== or odd mass number to exhibit this behavior
  • If a nuclei has an even atomic number, the magnetic fields cancel out
  • Spinning nuclei possess angular momentum (J) called spin
  • Placed in a magnetic field, the spin of the protons and neutrons assume certain orientations
  • Each spin produces a microscopic magnetization vector (𝜇)
  • 𝜇 = 𝛾 * J where gamma is the gyromagnetic ratio (specific to each material)
    • Gyromagnetic ratio gamma is radians per second*Teslas
    • The gyromagnetic ratio can also be gamma divided by 2pi
• The body is mostly water, which has a lot of hydrogen and is a great source for MRI signal
  • ==Hydrogen== has the highest gyromagnetic ratio
• The net (macroscopic) magnetization of a magnetic field is zero in its natural state
  • A single particle will have its own nonzero magnetic field

Bulk Magnetization Vector

• Once an external magnetic field (B0) is applied…
  • The spins of each particle align in parallel or anti-parallel (a semi majority in parallel)
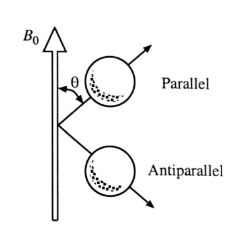
  • The net (bulk) magnetization vector with an external magnetic field is nonzero
  • The bulk magnetization (𝜇 vector) vector is the source of the MR signal (also called M vector)
• B0 is always applied along the z direction (up-down on the body)
  • Field strengths in MRI are commonly 1.5 T or 3 T (Tesla)
  • A 1.5T magnet is about 30,000 times stronger than Earth’s magnetic field
  • RF cage has to be in the MRI room to absorb any signals from outside, otherwise FM radio frequencies would be measured
• The vector 𝜇 precesses (spins) around the z axis st an angular frequency 𝜔 (radians per second)
  • 𝜔0 = 𝛾 * B0
  • Larmor Frequency: The angular frequency at which the bulk magnetization factor precesses around the z-axis
  • f = 𝛾 / (2pi) * B0
  • Larmor frequency depends only on the material (𝛾) and the main magnetic field strength (B0)

Generating Data

• Spin Equilibrium & spin excitation
  • At equilibrium, the net magnetization vector M precesses about the z-axis
  • When M is entirely along the z-axis, only the Mz (longitudinal) component exists, and the transverse component M(xy) = 0
  • If M tips away from the z-axis (during excitation), a component in the x-y plane is generated
  • The signal is always measured in the x-y plane
  • The signal component is always in the transverse x-y plane
• RF excitation
  • An RF pulse moves M away from the equilibrium state (tips away from z-axis), by using energy from the RF pulse onto the magnetic field B1
  • B0 is the magnet by itself, and the magnet is always on
    • The coil with current running through it acts as the magnet, and is surrounded by liquid Helium to keep the coil cool (decreasing resistance and energy lost to heat)
    • Why do we want the maximum signal? To decrease noise
  • B1 is applied from external antennas
    • B1 is along on the x-axis, so B1x is the only non-zero value for B1
  • Resonance comes from B1 (the additional force) being at the same frequency as B0 (𝜔0) to make sure there is maximum energy being delivery
  • As M returns to equilibrium, an RF signal is produced by energy releasing from the absorbed energy that was given to the material from B1
• RF Pulse Shape
  • While oscilating at 𝜔0, the RF pulse can have three shapes, rectangular, sinc, and triangular
• Rotating frame of reference
  • Since the spins and B1 field are both rotating, you can image from a spinning reference
  • The transverse plane (xy) is rotating at 𝜔, which is equal to 𝜔0, so it can be considered a rotating plane (x’-y’)
• Flip angle/tip angle
  • The flip angle depends on the (1) shape of the RF pulse, (2) field strength B1 and (3) duration of the RF pulse 𝜏p (tau p)
  • For a rectangular pulse after 𝜏 seconds, the vector M has rotated at an angle α
  • α = 𝛾 * *B1 ** 𝜏
  • For any B1(t)
  • α (always in radians) is the integration from 0 to 𝜏 over B1(t) with respect to t (in seconds)
  • For the max transverse component Mxy, α is set to pi/2 radians
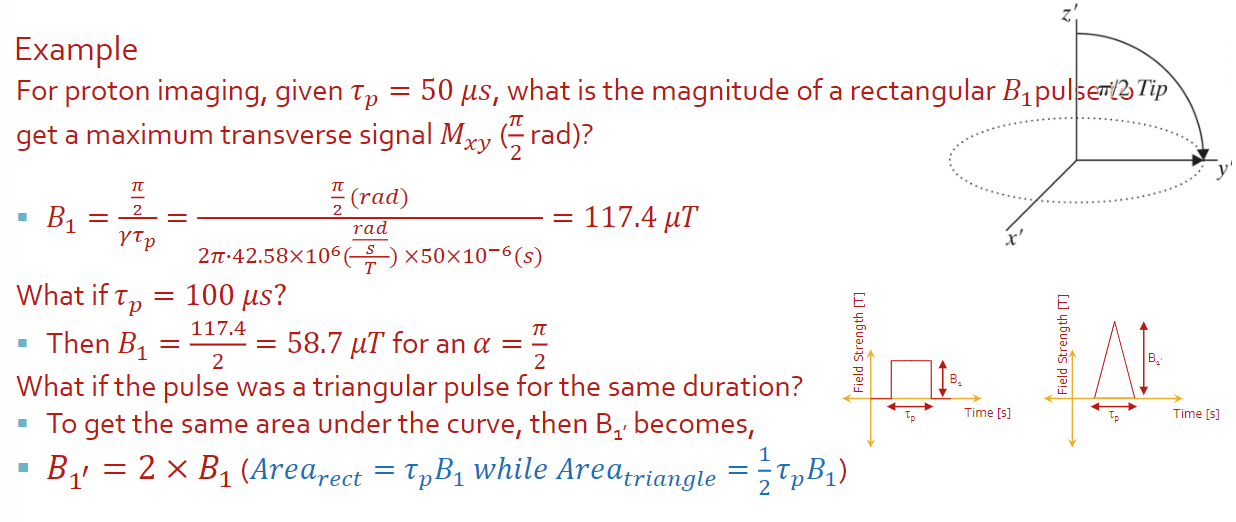
    • α = 𝛾 * *B1 ** 𝜏 = pi/2
• Relaxation: The process by which M returns to steady state configuration after a B1 is done being applied
  • The longitudinal Mz and transverse Mxy components vary as time changes, and are descibed by Bloch Equations
  • 
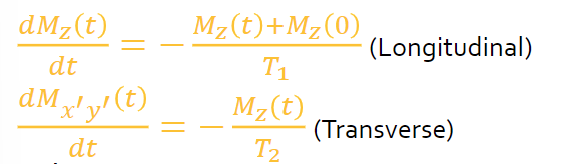
  • Where T1 is the longitudinal relaxation time (spin minus lattice relaxation)
  • T2 is the transvese relaxation time (spin minus spin relaxation)
  • 
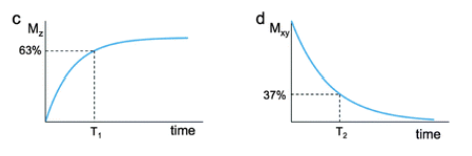
  • The solutions become
  • 
  • Mz^0 is the steady state of Mz
  • Mz(0+) is transverse magnetization Mx’y’ immediately after the RF pulse
  • We always measure the x-y plane and do everything else after
  • T1 is usually a LOT larger that T2
    • The longitudinal time is usually a lot longer than the transverse time (around 10 times more)
  • 
  • 
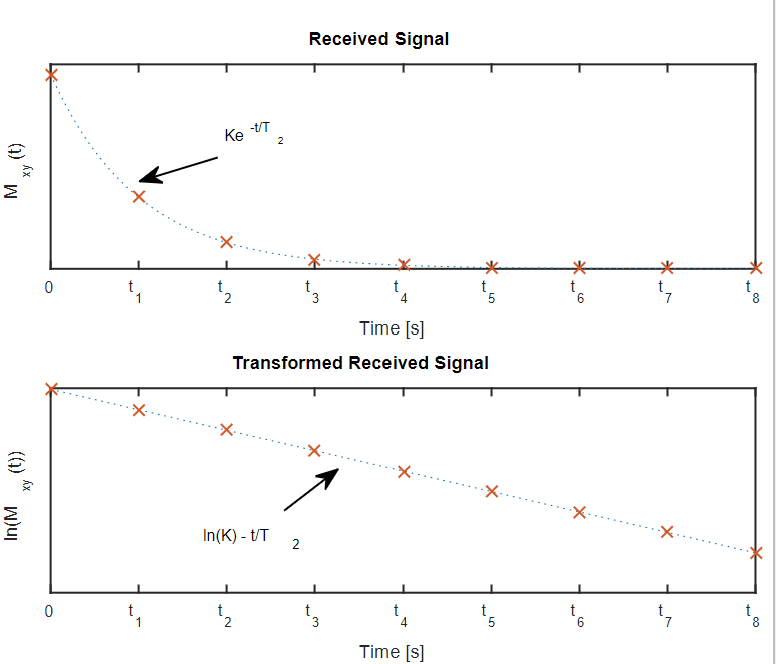
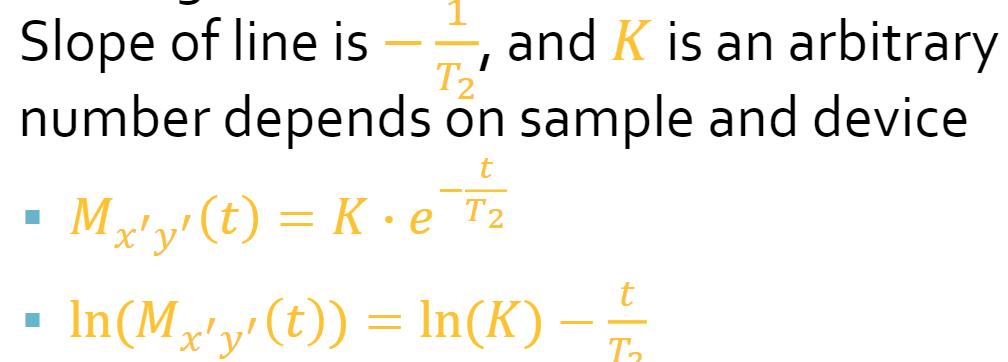
  • Mx’y’ decays at a faster rate (T2* instead of T2) because of signal desync
• Spin Echo
  • To correct for dephasing and loss of Mx’y’ signal, another RF pulse (180 degree pulse) is applied to re-phase the spins
  • Spin echo makes the slower spins catch up with faster oens to sync the signal
• Relaxation times T1 and T2 are dependant on materials (very high for water, really low for muscle, fat, tendons)

Acquisition & Contrast

• Contrast in MRI is the difference between signal intensities generated by different tissues (B0, which is constant, vs gamma, which is material specific)
• The difference in signal intensity is used to discriminate different tissues by representing them as brightness
• Pulse sequence: Carefully timed set of scanner operations used to generate images.
  • Gradient-echo based pulse sequences are
  • Spin-echo based pulse sequences
  • 90 degree pulse
  • 180 degree pulse
  • Wait for relaxation echo
  • Record relaxation echo
  • Repeat

MR Signal Intensity

• MR signal is always recorded in the xy plane
• Signal intensity S of a SE sequence is
  • S = K x [H] x (1 - exp(-1 x TR / T1) x exp(-1 x TE/T2)
  • K is the scaling factor
  • [H] is the proton/spin density
  • Twice the number of spins means you have twice as large a signal
  • TR is the repetition time
  • TE is the echo time
  • T1 is the longitudinal relaxation time
  • T2 is the transverse relaxation time
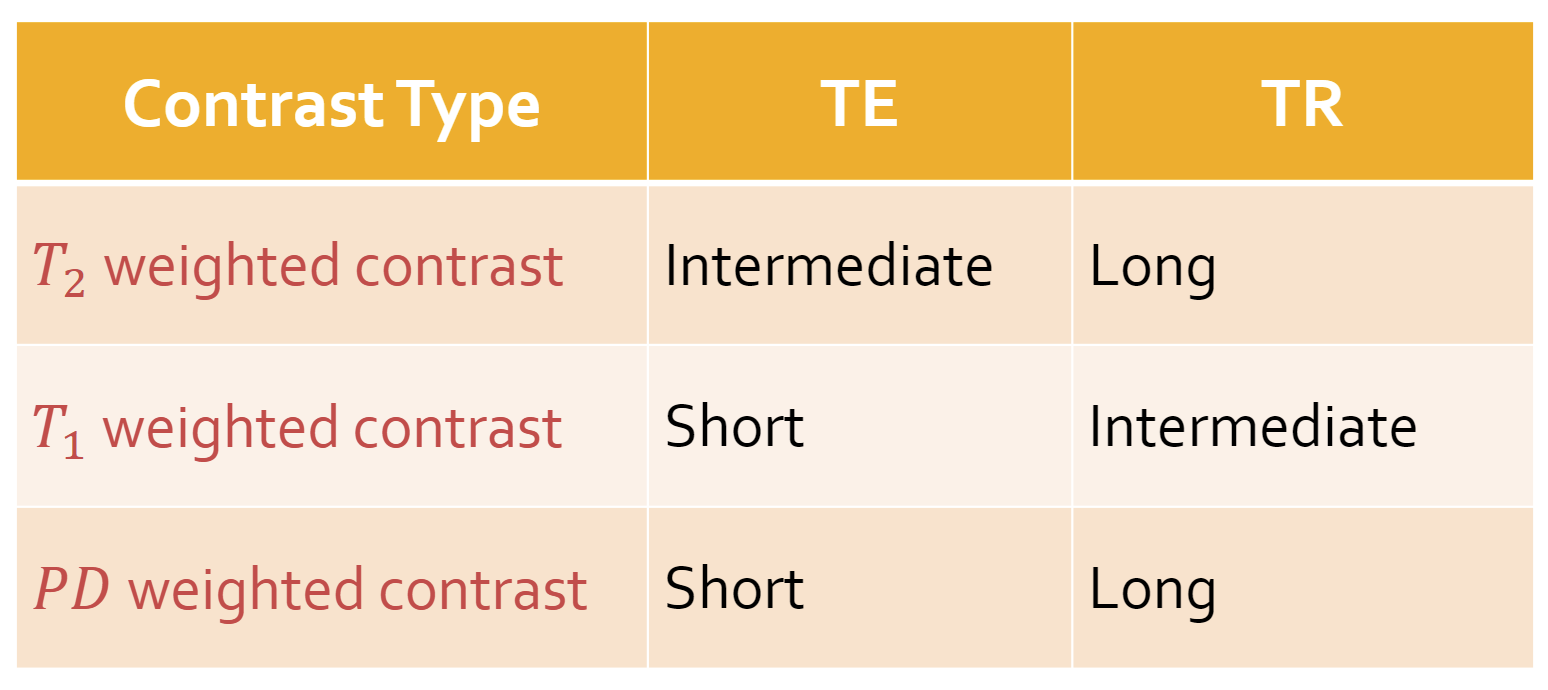
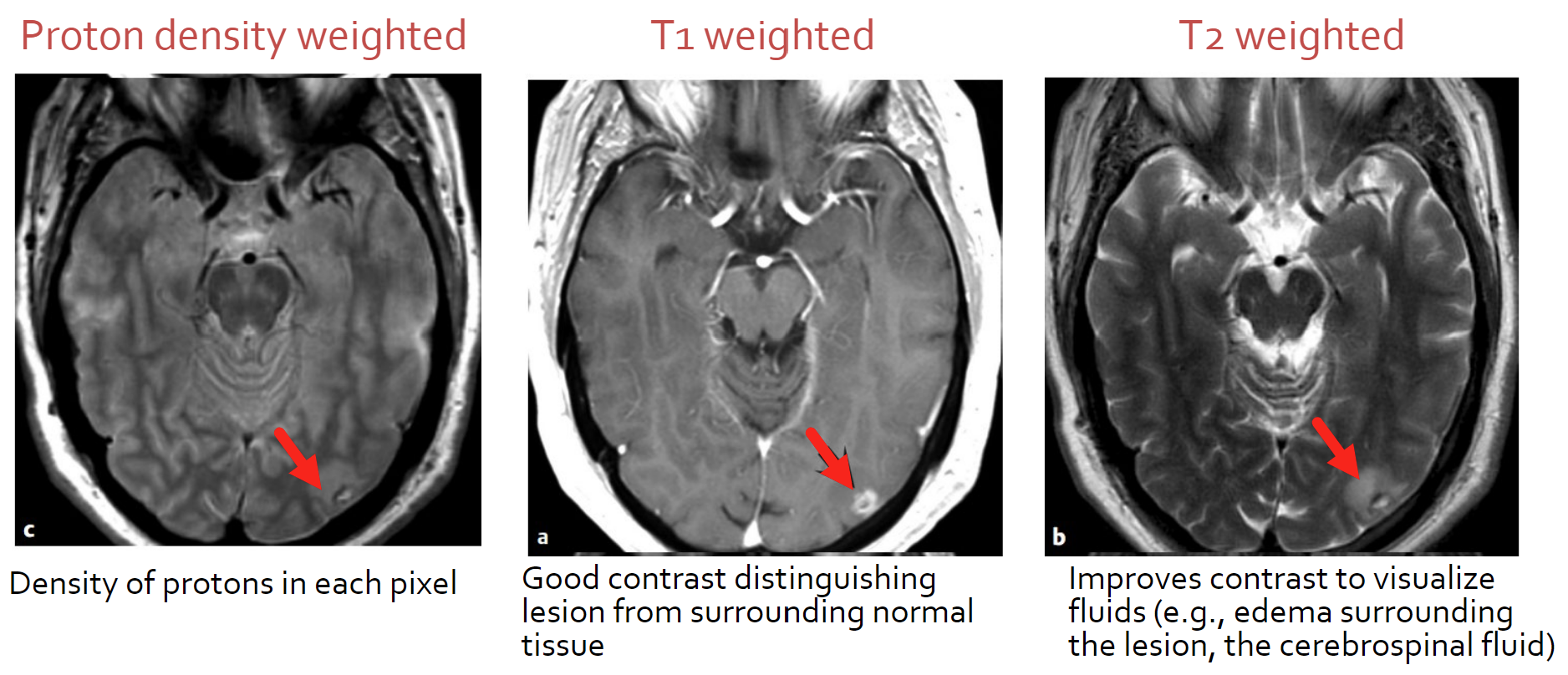
• Weighting
  • Proton density weighting: We want a long TR and a short TE
  • T1 weighting: We want an intermediate TR and a short TE (becomes a constant scaling factor, less noise)
  • T2 weighting: We want ta long TR (minimize T1 differences) and an intermediate TE
  • 
  • 
• Gradients
  • Adding a small gradient field (extra magnetic field) allows the same material to be differentiated if it is at different distances
  • Gradient affects Larmor frequency
  • Gradients added using more coils
  • Gradients are localized in x, y, and z directions
  • Gz is a slice selection
    • z-directional coils are called Maxwell pairs
  • Gx is frequency encoding
    • x-directional coils are called Golay coils
  • Gy is phase encoding
    • y-directional coils are called Golay-type coils
  • Gradients vary the magnetic field strength along the z-direction, which affects the frequency and phase of the spins
• Fourier Transform is used to break a signal into its freuqency components (sum of sinusoids) based on their amplitude and phase
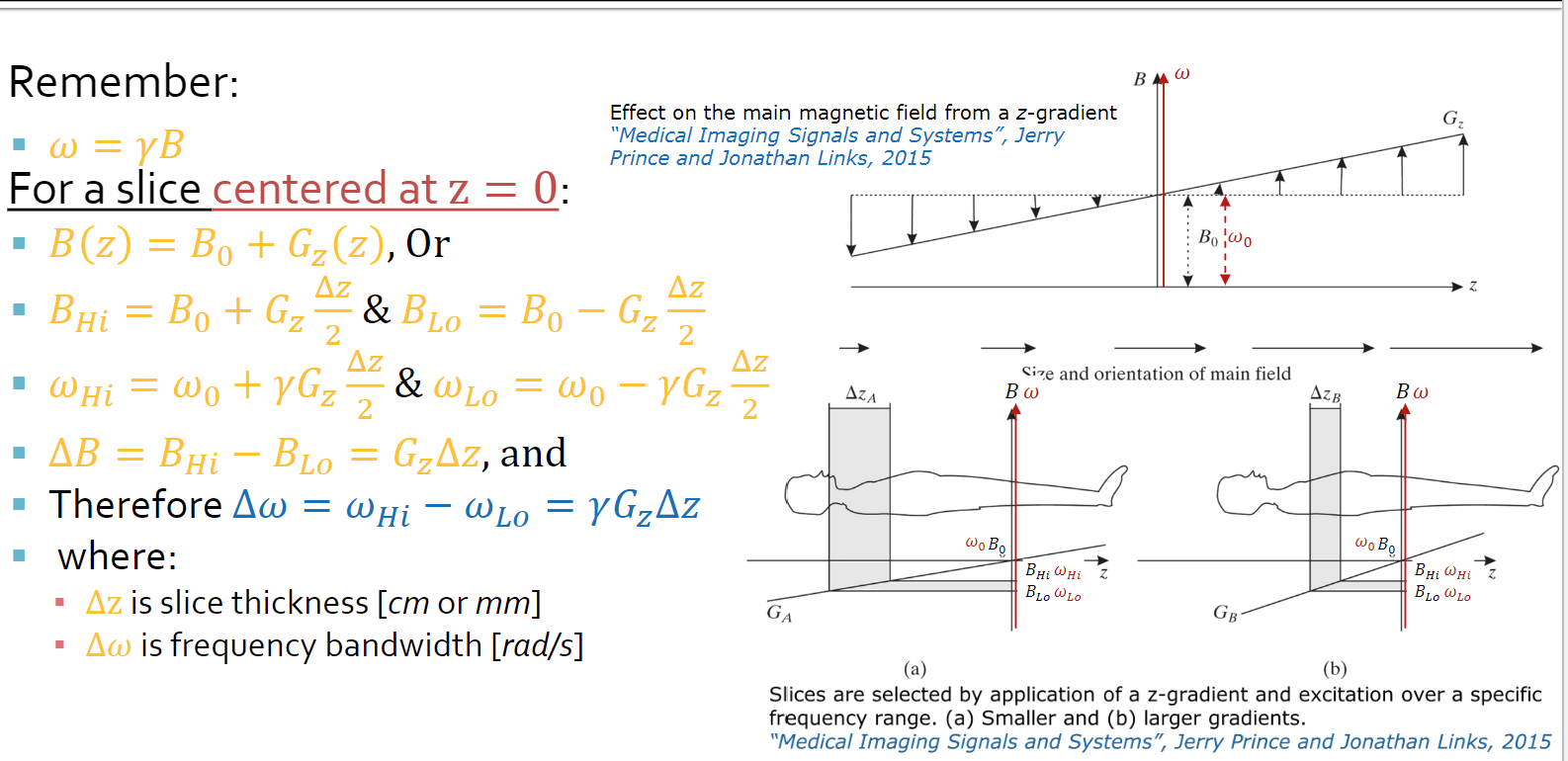
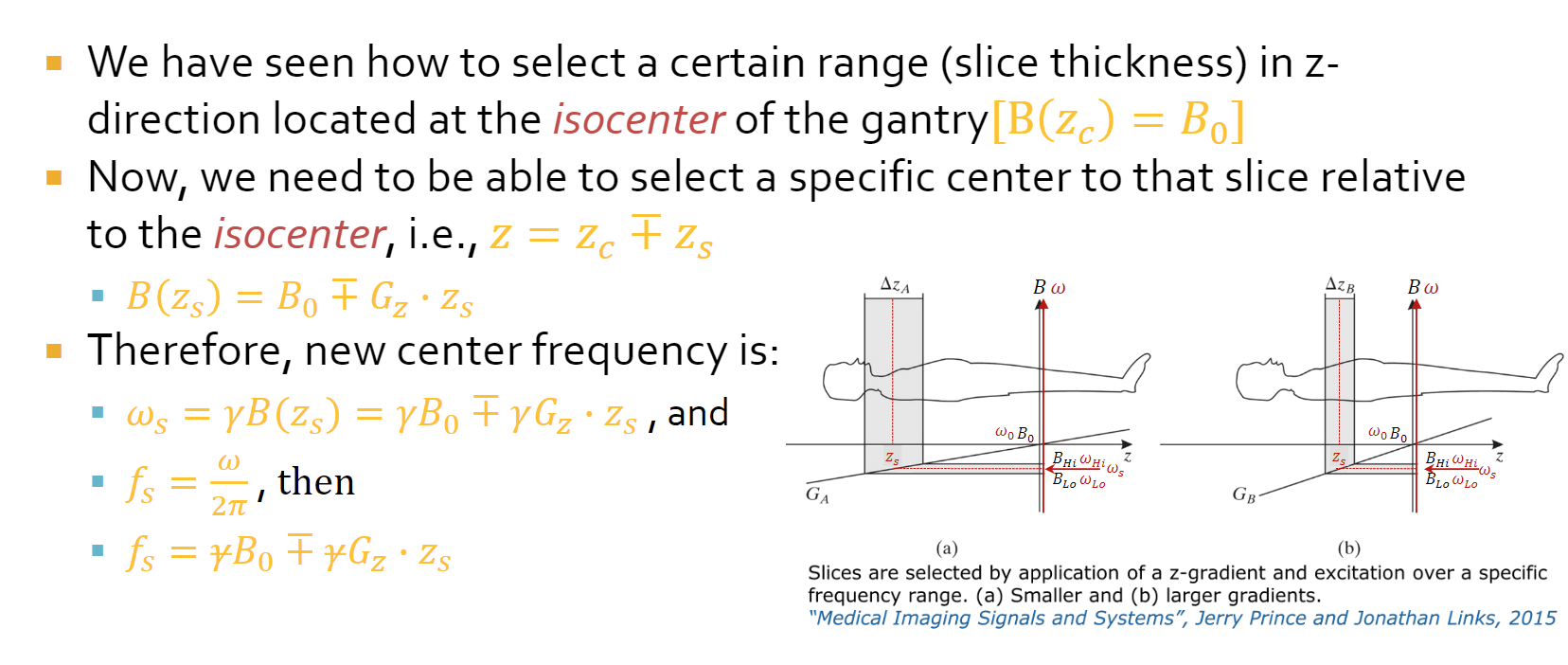
• Slice thickness
  • Centered at z=0 , B(z) = B0 + Gz(z)
  • Gz is gradient strength
  • 
  • 

Overview

• The magnet is used to create polarization (creates the bulk of the ==magnetic== field)
• The RF oil is used to promote excitation (sends RF energy at ==resonance== conditions)
• The gradients are used to generate spatial localization (used to form the ==images==)
• The receiver RF coils are there to target specific organs
• \

\