Unit 6: Thermochemistry

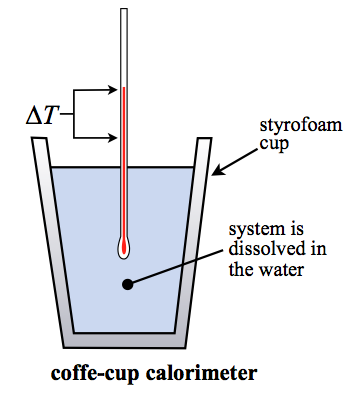
1) Coffee Cup Calorimeter - Insulated system, reaction in a solution

• Constant volume and pressure (ΔV = ΔP = 0)

  • Cannot expand or contract

  • Liquids are incompressible

  • ***If it involved a liquid or a solid, it maintains a constant volume and pressure.

• Work = 0

• ΔH = ΔE = Q

2) Piston Calorimeter - Gas Forming Reactions

• Piston changes volume (variable volume but constant P)

  • Ex: Gasoline engine

  • ΔV≠0, ΔP = 0

• ΔH = ΔE + PΔV = Q - PΔV + PΔV = Q —> ΔH = Q

3) Bomb Calorimeter - Gas forming reaction

• Constant V but varying P

  • ΔV = 0, ΔP ≠ 0

  • Measuring temp (C) of H2O

• ΔE = Q

• ΔH = Q + VΔP

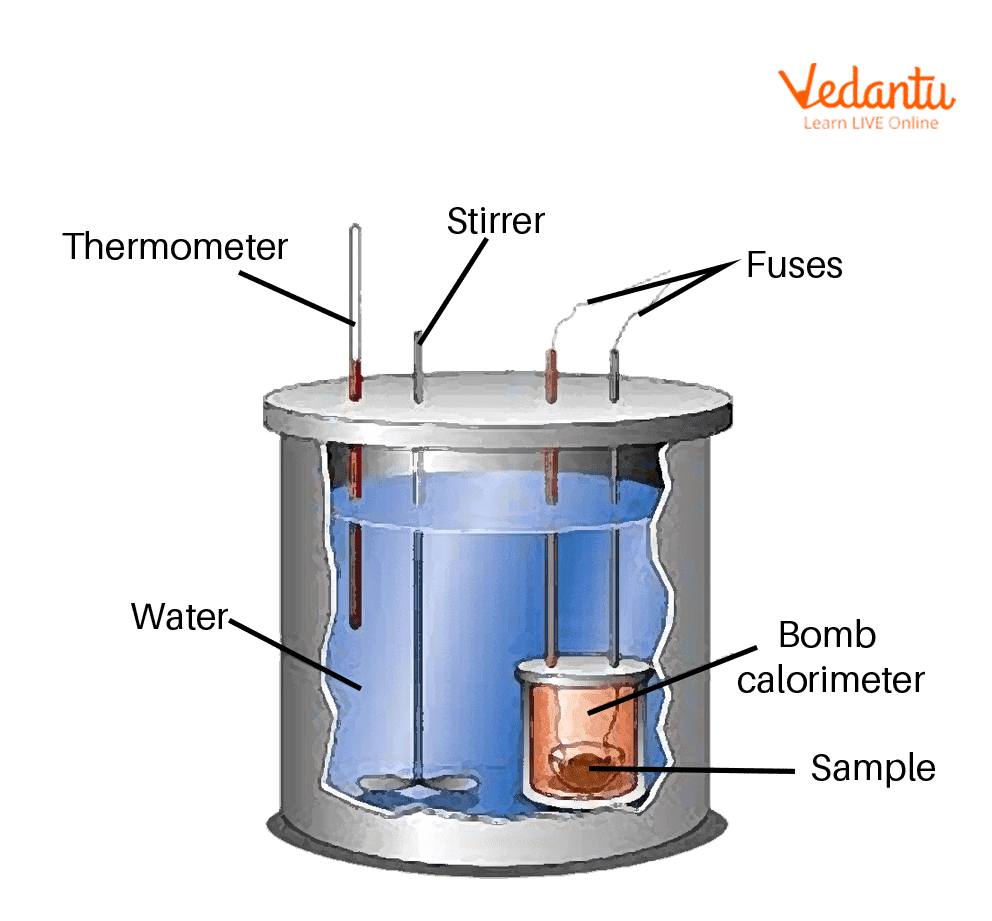
*High Specific Heat = lower thermal conductivity

Example: A 1.000 g sample of octane (C8H18) is burned in a bomb calorimeter containing 1200 grams of water at an initial temperature of 25.00ºC. After the reaction, the final temperature of the water is 33.20ºC. The heat capacity of the calorimeter (also known as the “calorimeter constant”) is 837 J/ºC. The specific heat of water is 4.184 J/g ºC. Calculate the heat of combustion of octane in kJ/mol.

• Qrxn = Qcal + QH2O

• Qcal = 837 J/ºC * (33.20 - 25.00) = 6862.4 J = 6.86 kJ

• Q_H2O = 1200 × 4.184 x (33.20 - 25.00) = 41170.56 J = 41.17 kJ

• - Q rxn = 6.862 + 41.17 = 48.032 kJ (the reaction is negative because it releases heat, as evident by the fact that the water increased in temperature

• 1.000 g C8H18/114.23 g/mol = 0.00875 moles of C8H18

• -48.032/0.00875 moles = -5489.4 kJ/mol

Example: 0.5060 grams of liquid cyclohexanol undergo complete combustion in a bomb calorimeter. The calorimeter assembly has a heat capacity of 827 J/ºC and contains exactly 1000 grams of water. What is the final temperature it the initial water temperature is 24.98 C? The heat of combustion is -3727 kJ/mol

• -(-3727 kJ/mol) = Qcal + QH2O = 827(Tf - 24.98) + 1000 g * 4.184 (Tf - 24.98)

• 0.5060 grams of C6H12OH/100.12 = 0.005054 moles

• 3727 × 0.005054 × 1000 = 827(Tf - 24.98) + 1000 g * 4.184 (Tf - 24.98)

  • Tf = 28.74 C

Example: A coffee-cup calorimeter having a heat capacity of 472 J/ºC is used to measure the heat evolved when the following aqueous solutions, both inititally at 22.6 C are mixed: 100 grams of a solution containing 6.62 g of lead (II) nitrate, and 100.0 grams of solution containing 6.00 grams of sodium iodide. The final temperature is 24.2 C. Assume that the specific heat of the mixture is the same as that for water (4.184 J/gºC). Find the H reaction

1) Balanced equation: Pb(NO3)2 (aq) + 2NaI (aq) —> PbI2(s) + 2NaNO3

Moles of Pb(NO3)2 = 6.62/331.2 = 0.02

Moles of NaI = 6.00/149.89 = 0.04

No Limiting Reactant

-Qrxn = Qcal + QH2O = 472 (24.2 - 22.6) + 200.0 g * 4.184 * (24.2 - 22.6) = 2094.08 J = 2.094 kJ

• Qrxn = -2.094 (negative because it releases energy, as evident by the increase in temperature of the solution/surrounding)

Hrxn = Qrxn/moles = -2.094 kJ/0.02 moles = -104.7 kJ/mol

Heat of Formation

• Formation reaction: synthesis of a compound from its elements in standard conditions (25 C, 1 atm, 1M)

• Example: Formation reaction of Ethanol (C2H5OH)

  • 2 C(s) + 3H2(g) + ½ O2(g) —> C2H5OH (l) ΔHf = -277.7 kJ/mol

  • C2H5OH (l) ΔHf = -277.7 kJ/mol

  • C2H5OH (g) ΔHf = -235.7 kJ/mol

    • Different heat of formation for gas and liquid. It takes energy to turn vaporize the liquid into a gas (Heat of vaporization)

    • Hf (l) + Hvap = Hf(g)

    • -277.7 + x = -235.7 —> x = 42.6 kJ/mol

Hrxn = ∑n_products * H_f - ∑n_reactants * H_f

• Products - Reactants

Solid Ice: C = 2.09

Water: 4.184

Gas: 2.02

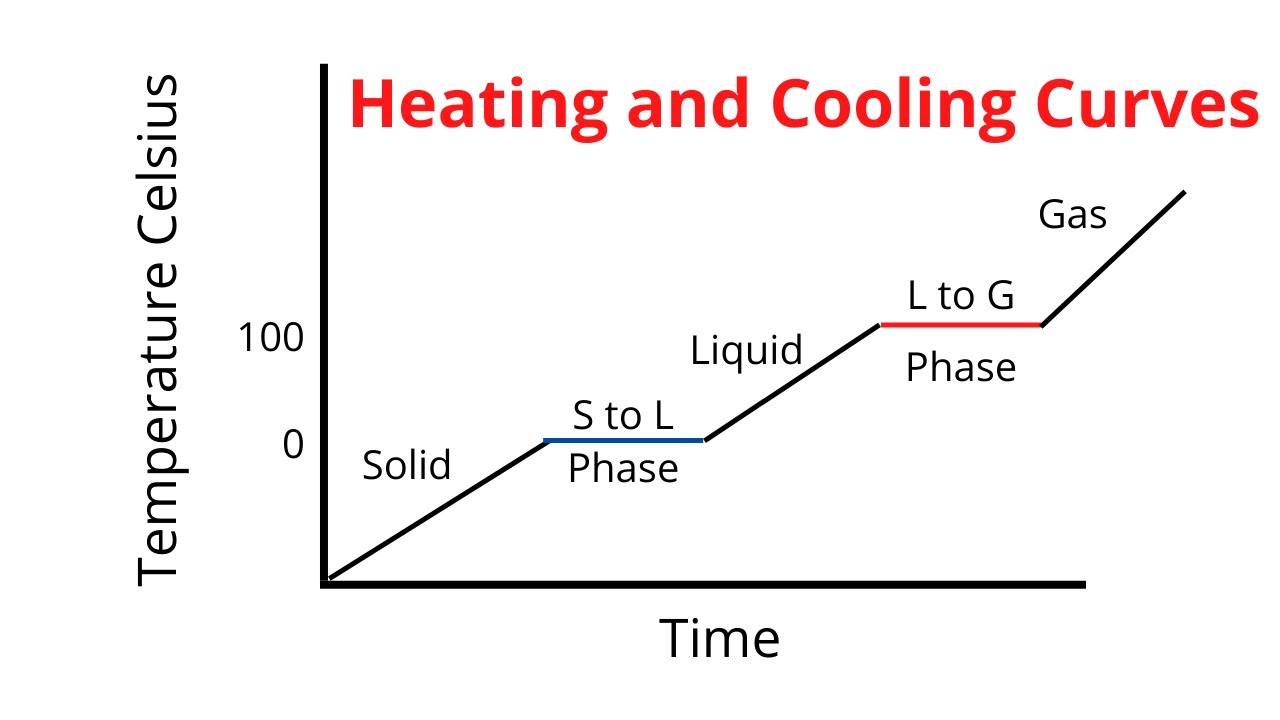
Heat of vaporization: 2260 J/g

Heat of Fusion: 334 J/g

H_vap>H_Fus because it takes more energy to completely break the bonds between water molecule to bring them from a liquid to a gas. When going from solid to liquid, the bonds are only partially broken/loosened.

*Important: For the heat of fusion, it is positive when it is an endothermic process. When a substance freezes, it releases the energy so the correct formula would be -m x H_fus.

For heat of vaporization: it is positive when it is an endothermic process. When vapor condenses, it releases the energy so it would be -m x H_vap

_{Example: How much heat is required to cool 25 g of water vapor from 130 degree C to -5 degree C?}

• Q = mCAT - mHvap + mCAT - mHfus + mCAT

• Q = 25(2.02 * (100-130) - 2260 + 4.184 (0-100) - 334 + 2.09 (-5-0))

  • Q = -77,086.25 J = -77.09 kJ

Energy

• ΔE = Q + (-PΔV)

  

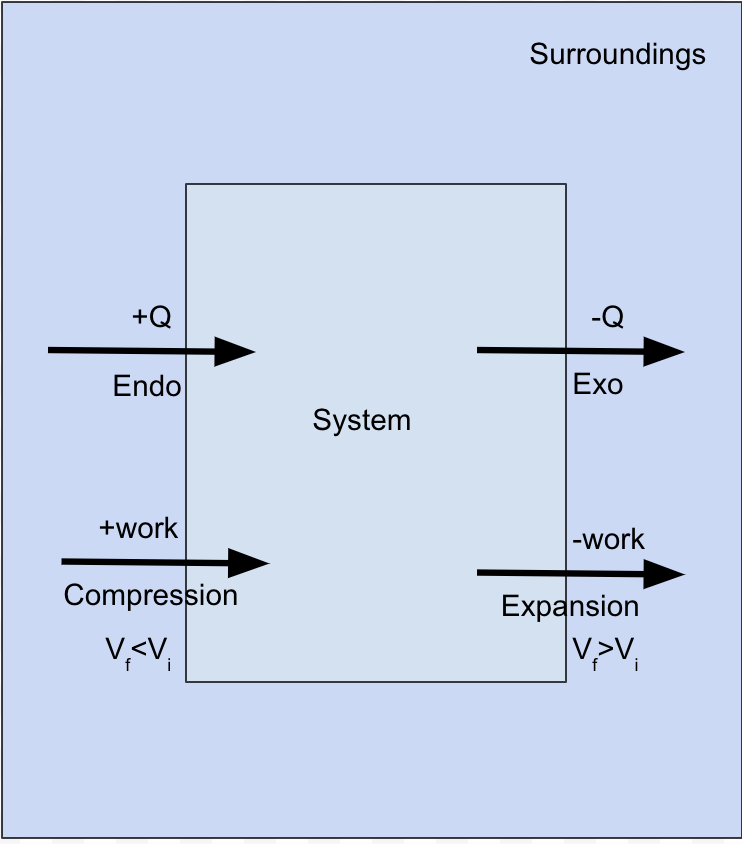
Increase ΔE = putting heat into system and compressing it

Decrease ΔE = releasing heat and expanding

UNITS: 1 L* atm = 101.3 Joules = 101.3 × 10^-3 kJ

Topics covered on the Test:

1) Calorimetry (Cup and Bomb)

• -Qrxn = Q_cal+Q_{H2O/surrounding}

• H_rxn = Q_rxn/moles of limiting reactant

• Q_H2O<Q_Cal because the calorimeter absorbs some of the heat

2) Mass-Heat stoichiometry

3) Hess’s Law, ΔHf, and Bond dissociation energy (use these to calculate Hrxn)

• Hess’s Law: Reversing a reaction —> flip the sign of the Hf

• Using ΔHf: products - reactants

• Using BDE: Bonds broken-Bonds formed

  • Breaking bonds is positive enthalpy and forming bonds is negative enthalpy

4) ΔE = Q + (-PΔV)

• Look at above diagram

5) Potential Energy Diagrams

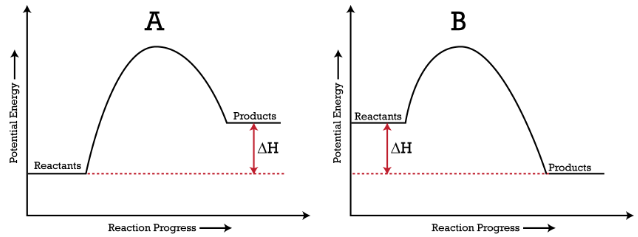
• Diagram A is endothermic (+ΔH) and diagram B is exothermic (-ΔH)

• Reactants to top of the graph is the activation energy

• Adding a catalyst decreases the activation (does not affect the reacts or products)

6) Mixing Hot and Cold

Condensation, freezing = exothermic, melting, freezing = endothermic

**Usually positive means heat absorbed and negative means heat released.

+ΔH = endothermic = treated as reactant

-ΔH = Exothermic = treated as product