Chapter 1: Units, Physical Quantities, and Vectors
Physics is an experimental science.
- Physicists observe the phenomena of nature and try to find patterns that relate these phenomena.
- These patterns are called physical theories or, when they are very well established and widely used, physical laws or principles.
No theory is ever regarded as the final or ultimate truth. The possibility always exists that new observations will require that a theory be revised or discarded.
Problem-Solving Strategy for solving Physics Problems:
- 1**. IDENTIFY** the relevant concepts.
- 2.SET UP the problem.
- Given the concepts you have identified and the known and target quantities, choose the equations that you’ll use to solve the problem and decide how you’ll use them.
- 3.EXECUTE the solution.
- Study the worked examples to see what’s involved in this step.
- 4.EVALUATE your answer
- Compare your answer with your estimates, and reconsider things if there’s a discrepancy.
In physics a model is a simplified version of a physical system that would be too complicated to analyze in full detail.
Any number that is used to describe a physical phenomenon quantitatively is called a physical quantity.
- Example: weight, height etc.
When we measure a quantity, we always compare it with some reference standard.
- Such a standard defines a unit of the quantity.
- The meter is a unit of distance, and the second is a unit of time.
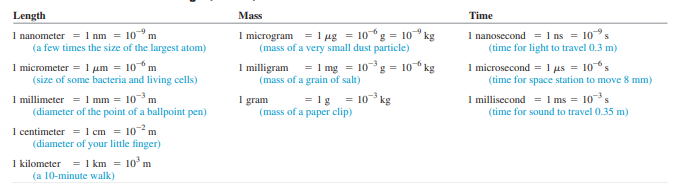
Some units of length, Mass and time:
<<An equation must always be dimensionally consistent.<<
An uncertainty in the measurement is also called the error because it indicates the maximum difference there is likely to be between the measured value and the true value.
- The uncertainty or error of a measured value depends on the measurement technique used.
When a physical quantity is described by a single number, it is a scalar quantity.
In contrast, a vector quantity has both a magnitude and a direction in space.
- The simplest vector quantity is displacement.
- Displacement is simply a change in the position of an object.
- Displacement is a vector quantity because we must state not only how far the object moves but also in what direction
A vector with the same magnitude but in the opposite direction is known as a negative vector.
When two vectors and have opposite directions, whether their magnitudes are the same or not, we say that they are antiparallel.
The magnitude of a vector quantity is a scalar quantity (a number) and is always positive
A vector can never be equal to a scalar because they are different kinds of quantities.
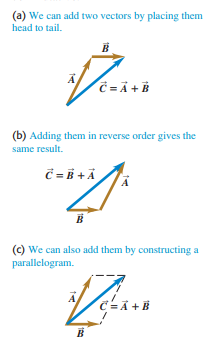
Suppose a particle undergoes a displacement A followed by a second displacement B .
- The final result is the same as if the particle had started at the same initial point and undergone a single displacement C.
- We call displacement the vector sum, or resultant, of displacements and We express this relationship symbolically as
C= A+B
- If we make the displacements and in reverse order, with first B and second A, the result is the same.
Multiplying a vector by a positive scalar changes the magnitude (length) of the vector, but not its direction.
Multiplying a vector by a negative scalar changes its magnitude and reverses its direction.
A vector can be divided into its horizontal component( Ax) and a vertical component (Ay).
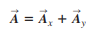
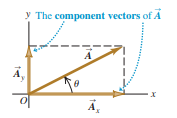
Components are not vectors. The components and of a vector are just numbers; they are not vectors themselves.
Imagine that the vector originally lies along the x axis and that you then rotate it to its correct direction, as indicated by the arrow in figure below on the angle theta.
- If this rotation is from the +x axis toward the +y axis, then theta is positive
- If the rotation is from the +x axis toward the -y axis, theta is negative.
Thus the +y axis is at an angle of 90°, the at 180°, and the at 270° (or If is measured in this way, then from the definition of the trigonometric function).

The trigonometric functions for this vector and theta are:
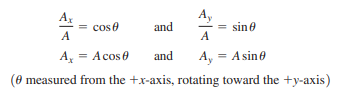
Unit vectors describe directions in space. A unit vector has a magnitude of 1, with no units.
The unit vectors i,j and k aligned with the x-, y-, and z-axes of a rectangular coordinate system, are especially useful.
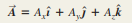
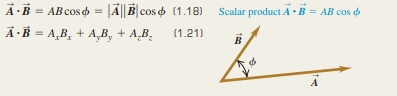
The scalar product C=A.B of two vectors A and B is a scalar quantity.
It can be expressed in terms of the magnitudes of A and B and the angle phi between the two vectors, or in terms of the components of A and B.
The scalar product is commutative.
The scalar product of two perpendicular vectors is zero.
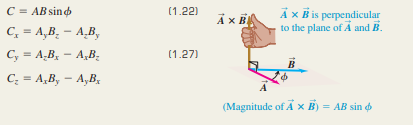
The vector product C=AxB of two vectors A and B is another vector.
The magnitude of depends on the magnitudes of A and B and the angle phi between the two vectors.
==The direction of the vector product is perpendicular to the plane of the two vectors being multiplied, as given by the right-hand rule.==
The components of the vector product can be expressed in terms of the components of A and B.
The vector product is not commutative.
The vector product of two parallel or antiparallel vectors is zero.