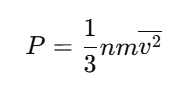Kinetic Theory(PRESSURE EXERTED BY GAS)

PRESSURE EXERTED BY GAS

• An ideal gas is enclosed in a cubical box of side length lll.

• A molecule of gas is moving with a random velocity v, which can be resolved into components:

  

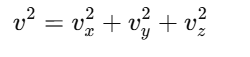
• Momentum along the x-axis for a molecule is px=mvx​, where m is the mass of the molecule.

Change in Momentum During a Collision

• When a molecule collides with a wall parallel to the y-z plane, its velocity component vx is reversed, so:

  

This is the total change in momentum along the x-axis.

Number of Collisions in Time ΔT

• Consider the number density n, which is the number of molecules per unit volume.

• Molecules that can collide with the wall in time ΔT are those within a distance vxΔT of the wall.

• The number of such molecules is:

  

The factor 1/2​ accounts for only those molecules moving toward the wall.

Momentum Transferred to the Wall

• For each collision, the momentum transfer is 2mvx​.

• Total momentum transferred in time ΔT is:

  

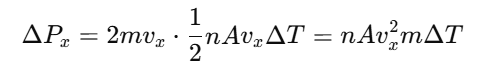
Force on the Wall

• Force is the rate of change of momentum:

  

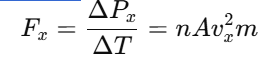
Pressure on the Wall

• Pressure is force per unit area:

  

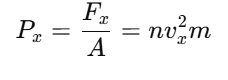
Velocity Averaging

• Since molecules have different velocities, we use the average of v²_x

  

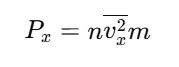
From symmetry of the cube, pressure is the same along all three axes (Px=Py=Pz​):

The pressure of the gas is: