units and measurements

PHYSICAL QUANTITIES

quantities which can be measured. they are of two types;

• fundamental quantities: independent of other quantities. there are 7 fundamental quantities;

  • length, metre (m)

  • mass, kilogram (kg)

  • time, second (s)

  • electric current, ampere (A)

  • thermodynamic temp., kelvin (K)

  • amt. of substance, mole (mol)

  • luminous intensity, candela (cd)

• derived quantities: derived from the fundamental quantities by multiplication/ division. eg: velocity = length/ time

to measure physical quantities, we need two things;

• the unit, u

• the numerical value/ magnitude, n

this gives us,

Q = nu

we know that the magnitude of a quantity remains same, no matter the unit of measurement

eg: 1 kg = 1000g

hence,

Q = n1u1 = n2u2

some common systems of units

• FPS system: foot/ pound/ second

• CGS system: centimeter/ gram/ second

• MKS system: metre/ kilogram/ second

• SI system: metre/ kilogram/ second

NOTE: the FPS system is not a metric system

order of magnitude: expression of very large numbers in the form N x 10^n where N < 10 (eg: 6.4 × 10^7)

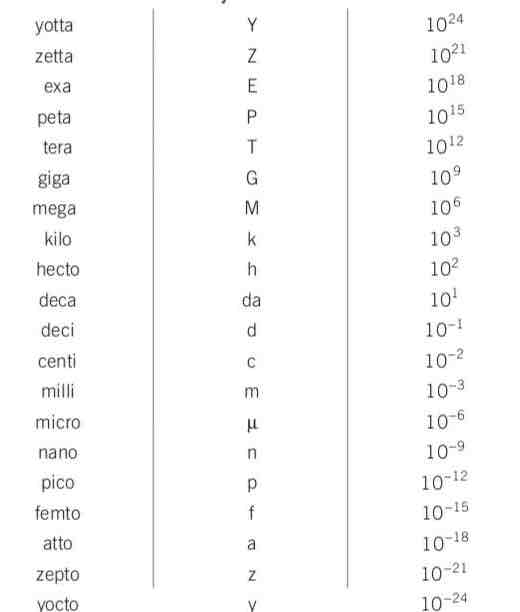
prefixes of powers of 10

SIGNIFICANT FIGURES

a result of measurement includes all digits of the number known reliably plus one which is uncertain. these digits are called significant figures

eg: 374.5m has 4 SFs; 3,7 and 4 are certain while 5 is uncertain

rules to determine no. of SFs

1. all non-zero digits are significant (eg: 1234 → 4 SFs)

2. all zeros between 2 non-zero digits are significant (eg: 1007 & 10.07 → 4 SFs)

3. any zeros before the first non-zero digit is not significant (eg: 0.005704 → 4 SFs)

4. trailing zeros are not significant in non-decimal no.s (eg: 3210 → 3 SFs)

5. trailing zeros are significant in decimal no.s (eg: 3.500 → 4 SFs)

6. multiplying/ dividing factors have infinite no. of SFs (eg: in r = d/2, 2 → infinite SFs)

7. the no. of SFs do not depend on the unit (eg: 16.4cm, 0.164m and 0.000164cm → all have 3 SFs)

rounding off measurements

to round off measurements, the following rules must be followed;

1. if the digit is less than 5, it is dropped (eg: 7.82 → 7.8)

2. if the digit is more than 5, it is dropped & the preceding digit is raised by 1 (eg: 6.87 → 6.9)

3. if the digit is 5 followed by any non-zero digit, they are both dropped and the preceding digit is raised by 1 (eg: 16.351 → 16.4)

4. if the digit is 5 followed by 0, it is dropped and the preceding digit is left unchanged (eg: 3.250 → 3.2)

rules for operations with SFs

• addition & subtraction: the final result should retain as many decimal places as there are in the number with the least decimal places (eg: 2.1m + 1.78m + 2.046m = 5.926m → 5.9m

• multiplication & division: the final result should retain as many SFs as there are in the number with the least SFs (eg: 3.00 × 3.155 = 9.4685 → 9.46 → 9.5 (rounded off))

least count

it is the smallest subdivision on a scale and it is uncertain while measuring (eg: in a cm scale, 1mm is smallest subdivision)

least count of a screw gauge = pitch/ total no. of divisions

NOTE

precision: closeness of two measurements to each other

accuracy: closeness of a measurement to the actual value

DIMENSIONS OF A PHYSICAL QUANTITY

they are the powers to which the units of base quantities are raised to represent a derived unit

as we know, there are 7 base quantities, [L], [M], [T], [A], [K], [Cd] & [mol]

eg: V = [L] x [L] x [L] = [L]³ = [M^0L³T^0]

F = ma = [M] x [L]/[T]² = [M^1L^1T^-2]

the above are called dimensional formulae of a quantity

easy strategy to find dimensional formulae

1. write the formula of the quantity (eg: a = velocity/ time)

2. convert it into fundamental quantities (eg: a = length/ time²)

3. write the symbols for the fundamental quantities (eg: length/ time² = L/T²]

4. convert it into the form [MLT] (eg: L/T² = [M^0L^1T^-2])

DIMENSIONAL ANALYSIS

dimensional analysis helps us to find relations between different physical quantities. it has 3 uses;

• checking the dimensional consistency of equations: the principle of homogeneity of dimension: all terms in a physical quantity equation must have same dimensions (a dimensionally correct equation)

  eg: s = ut + ½ at²

  here, [L] = [L] + [L]

• conversion of units from one system to another: we know,

  Q = n1u1 = n2u2

  which gives,

  n2 = n1u1/u2 (where u1 & u2 are 2 units and n1 and n2 are their respective numerical values)

if u1 has dimensional formula [M1^aL1^bT1^c] and u2 has [M2^aL2^bT2^c], then we get,

n2 = n1 [M1^aL1^bT1^c]/ [M2^aL2^bT2^c]

hence,

n2 = n1[M1/M2]^a [L1/L2]^b [T1/T2]^c

ex Q: find the value of 60J per min on a system that has 100g, 100cm and 1 min as the base units

=> 60J/ 1 min = 60J/60s = 1W

dimensional formula of P = [ML²T^-3]

n1 = 1, M1 = 1000g, L1 = 100cm, T1 = 1s, n2 = ?, M1 = 100g, L2 = 100cm, T2 = 60s

n2 = 1[1000/100]^1 [100/100]² [1/60]^-3

= 2.16 × 10^6

• deducing relation among physical quantities: we can find the dependence of a physical quantity on other quantities

  given by, Q = kq1^aq2^bq3^c

  eg : consider a simple pendulum with a bob that oscillates under gravity. its t depends on mass, length & gravity

  time period T = km^aL^bg^c (k = dimensionless constant of proportionality)

  [M^0L^0T] = M^aL^b[LT^-2]^c

  = [M]^a[L]b+c[T]^-2c

  applying principle of homogeneity, a = 0, b = ½ & c = -1/2

  substituting these values,

  T = km^0l^1/2g^-1/2

  T = k root l/g

  NOTE: there are many limitations to dimensional analysis. it does not tell us if a quantity is scalar/ vector, and we cannot derive formulas with trigonometrical/ logarithmic functions